17.S: Additional Aspects of Aqueous Equilibria (Summary)
- Page ID
- 91302
17.1: The Common-Ion Effect
The common-ion effect argues that the dissociation of a weak electrolyte is decreased by adding a strong electrolyte to the solution that has a common ion with the weak electrolyte.
17.2: Buffered Solutions
Buffers are solutions that resist a change in pH
17.2.1 Composition and Action of Buffered Solutions
- buffers have both acidic and basic species to neutralize H+ and OH- ions
- acid dissociation equilibrium in buffered solution \[ HX(aq) \rightleftharpoons H^+ (aq) + X^-(aq)\] with \[ K_A = \dfrac{[H^+][X^-]}{[HX]}\] or \[[H^+]= K_a \dfrac{[HX]}{[X^-]}\]
- pH determined by: value of Ka AND the ratio of [HX]/[X-]
- if OH- added:
- \[ OH^-(aq) + HX(aq) \rightleftharpoons H_2O(l) + X^-(aq)\]
- Therefore [HX] decreases and [X-] increases
- if amounts of HX and X- are larger than amount of OH-
- ratio [HX]/[X-] doesn’t change much, and pH is small
- when [HX] and [X-] are about the same, buffers are most effective: i.e., when \([H^+] = K_a\)
17.2.2 Buffer Capacity and pH
- buffer capacity – amount of acid or base buffer can neutralize before the pH changes considerably
- capacity depends on amount of acid or base in buffer
- pH depends on Ka for acid and relative concentrations of the acid and base
- Henderson-Hasselbalch Approximation: \[ pH = pK_a + \log_{10} \dfrac{[base]}{[acid]}\]
- [base] and [acid] = concentrations of conjugate acid-base pair
- when [base]=[acid], pH=Ka
- can use initial concentrations of acid and base components of buffer directly into equation
17.2.3 Addition of Strong Acids or Bases to Buffers
reactions between strong acids and bases go to completion
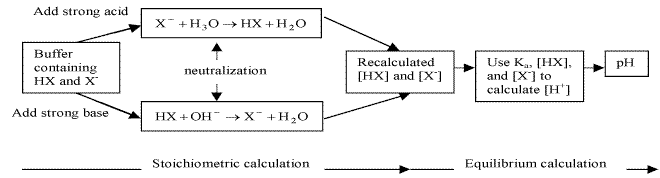
Either strong base or acid assumed to be completely consumed by reaction with buffer if buffering capacity is not exceeded
17.3: Acid-Base Titrations
- solution containing a known [base] added to an acid or acid solution added to base
- acid-base indicators used to signal equivalence point
- titration curve – pH vs Volume
17.3.1 Strong Acid – Strong base Titrations
- pH starts out low ends high
- pH before equivalence point is pH of acid not neutralized by base
- pH at equivalence point is pH of solution
- pH equals 7.00
- for strong base titrations, the pH starts high ends low
17.3.2 The Addition of a Strong Base to a Weak Acid
Reactions between weak acid and strong base goes to completion
- calculating pH before equivalence point
- stoichiometric calculations: allow strong base to react to completion producing a solution containing a weak acid and its conjugate base

- equilibrium calculation: use Ka and equilibrium expression to find equilibrium concentrations of the weak acid and its conjugate base, and H+
17.3.3 Titration Curves for Weak Acids or Weak Bases
Differences between strong acid-strong base titrations
- solution of weak acid as higher initial pH than solution of a strong acid with same concentration
- solution of weak acid rises more rapidly in early part of titration and more slowly as it reached the equivalence point
- pH is not 7.00 at equivalence point
- before equivalence point solution has mixture of weak acid and its salt
- also called the buffer region of curve
- at equivalence point solution contains only salt
- weakly basic due to hydrolysis of anion
- after equivalence point solution has mixture of salt and excess strong base
- pH determined by [base]
17.3.4 Titrations of Polyprotic Acids
- reaction occurs in series of steps
- titration curve shows multiple equivalence points
17.4: Solubility Equilibria
17.4.1 The Solubility-Product Constant, Ksp
- saturated solution – dissolved and undissolved solute are at equilibrium
- expressed by g/L
- molar solubility – moles of solute dissolved to form a liter of saturated solution (mol/L)
- Ksp equilibrium constant for the equilibrium between an ionic solid and its saturated solution
- Solubility of compound (g/L) à molar solubility of compound (mol/L) à [molar] of ions à Ksp of ions
17.5: Factors that Affect Solubility
solubility affected by temperature and presence of other solutes. The solubility of ionic compound affected by:
- the presence of common ions
- pH of solution
- presence of complexing agent
17.5.1 Common-Ion Effect
- solubility of slightly soluble salt decreases when a second solute has a common ion
17.5.2 Solubility and pH
- solubility of any ionic compound affected if solution is acidic or basic
- change only noticeable if both ions are moderately acidic or basic
- solubility of slightly soluble salts containing basic anions increase as [H+] increases (as pH is lowered)
- the more basic an anion is, the greater the solubility will be affected by pH
17.5.3 Formation of Complex Ions
- metal ions act as Lewis acids in water
- complex ion – metal ion and Lewis base bonded together
- Kf – formation constant, equilibrium expression for formation of a complex ion
- Solubility of metal salts increases in acceptable Lewis bases if metal forms a complex base
- Lewis bases: \(NH_3\), \(CN^-\), \(OH^-\)
17.5.4 Amphoterism
- amphoteric substances include hydroxides and oxides of: Al3+, Cr2+, Zn2+, and Sn2+
- dissolve in strongly basic solutions
- formation of complex anions containing, typically four, hydroxides bound to metal ion
- amphoterism also associated with behavior of water molecules that surround and bond to metal ions by Lewis acid-base interactions
17.6: Precipitation and Separation of Ions
Q = ion product
- If Q > Ksp, precipitation occurs until Q = Ksp
- If Q = Ksp, equilibrium exists, have a saturated solution
- If Q < Ksp, solid dissolves until Q = Ksp
17.6.1 Selective Precipitation of Ions
- separation of ions in aqueous solution using a reagent that precipitates only with selected ions
17.7: Qualitative Analysis for Metallic Elements
Qualitative analysis: determines presence or absence of a particular metal ion
- ions separated into broad groups on basis of solubility
- ions separated by dissolving selected members in group
- ions identified by specific tests

