Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

15.4: Heterogeneous Equilibria

Last updated

Sep 8, 2020
Save as PDF
- 15.3: Interpreting & Working with Equilibrium Constants
- 15.5: Calculating Equilibrium Constants

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Learning Objectives

To understand how different phases affect equilibria.

When the products and reactants of an equilibrium reaction form a single phase, whether gas or liquid, the system is a homogeneous equilibrium. In such situations, the concentrations of the reactants and products can vary over a wide range. In contrast, a system whose reactants, products, or both are in more than one phase is a heterogeneous equilibrium, such as the reaction of a gas with a solid or liquid.

Because the molar concentrations of pure liquids and solids normally do not vary greatly with temperature, their concentrations are treated as constants, which allows us to simplify equilibrium constant expressions that involve pure solids or liquids.The reference states for pure solids and liquids are those forms stable at 1 bar (approximately 1 atm), which are assigned an activity of 1. (Recall that the density of water, and thus its volume, changes by only a few percentage points between 0°C and 100°C.)

Consider the following reaction, which is used in the final firing of some types of pottery to produce brilliant metallic glazes:

$CO_{2(g)}+C_{(s)} \rightleftharpoons 2CO_{(g)} \label{Eq14.4.1}$

The glaze is created when metal oxides are reduced to metals by the product, carbon monoxide. The equilibrium constant expression for this reaction is as follows:

$K=\dfrac{[CO]^2}{[CO_2][C]} \label{Eq14.4.2}$

Because graphite is a solid, however, its molar concentration, determined from its density and molar mass, is essentially constant and has the following value:

$[C] =\dfrac{2.26 \cancel{g}/{\cancel{cm^3}}}{12.01\; \cancel{g}/mol} \times 1000 \; \cancel{cm^3}/L = 188 \; mol/L = 188\;M \label{Eq14.4.3}$

We can rearrange Equation $\ref{Eq14.4.3}$ so that the constant terms are on one side:

$K[C]=K(188)=\dfrac{[CO]^2}{[CO_2]} \label{Eq14.4.4}$

Incorporating the constant value of $[C]$ into the equilibrium equation for the reaction in Equation $\ref{Eq14.4.4}$ ,

$K'=\dfrac{[CO]^2}{[CO_2]} \label{Eq14.4.5}$

The equilibrium constant for this reaction can also be written in terms of the partial pressures of the gases:

$K_p=\dfrac{(P_{CO})^2}{P_{CO_2}} \label{Eq14.4.6}$

Incorporating all the constant values into $K′$ or $K_p$ allows us to focus on the substances whose concentrations change during the reaction.

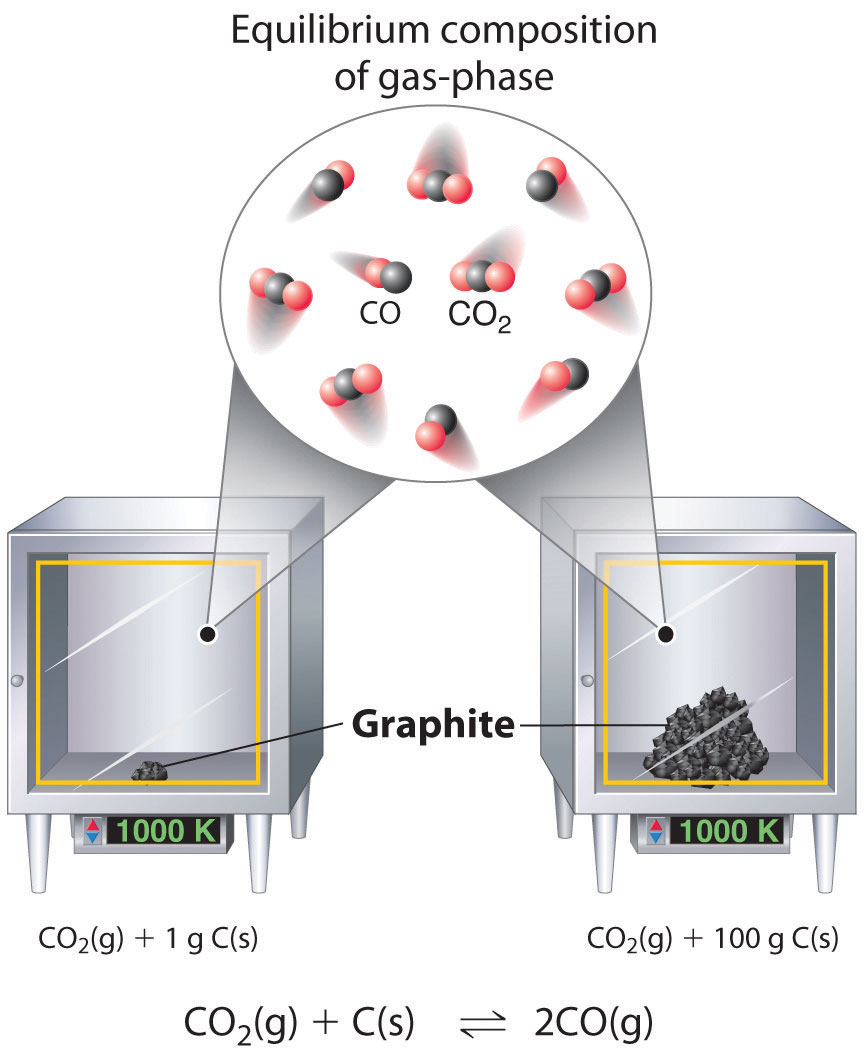
Although the concentrations of pure liquids or solids are not written explicitly in the equilibrium constant expression, these substances must be present in the reaction mixture for chemical equilibrium to occur. Whatever the concentrations of $CO$ and $CO_2$ , the system described in Equation $\ref{Eq14.4.1}$ will reach chemical equilibrium only if a stoichiometric amount of solid carbon or excess solid carbon has been added so that some is still present once the system has reached equilibrium. As shown in Figure $\PageIndex{1}$ , it does not matter whether 1 g or 100 g of solid carbon is present; in either case, the composition of the gaseous components of the system will be the same at equilibrium.

Figure $\PageIndex{1}$ : Effect of the Amount of Solid Present on Equilibrium in a Heterogeneous Solid–Gas System. In the system, the equilibrium composition of the gas phase at a given temperature, 1000 K in this case, is the same whether a small amount of solid carbon (left) or a large amount (right) is present.

Example $\PageIndex{1}$

Write each expression for $K$ , incorporating all constants, and $K_p$ for the following equilibrium reactions.

$PCl_{3(l)}+Cl_{2(g)} \rightleftharpoons PCl_{5(s)}$
$Fe_3O_{4(s)}+4H_{2(g)} \rightleftharpoons 3Fe_{(s)}+4H_2O_{(g)}$

Given: balanced equilibrium equations

Asked for: expressions for $K$ and $K_p$

Strategy:

Find $K$ by writing each equilibrium constant expression as the ratio of the concentrations of the products and reactants, each raised to its coefficient in the chemical equation. Then express $K_p$ as the ratio of the partial pressures of the products and reactants, each also raised to its coefficient in the chemical equation.

Solution:

This reaction contains a pure solid ( $PCl_5$ ) and a pure liquid ( $PCl_3$ ). Their concentrations do not appear in the equilibrium constant expression because they do not change significantly. So

$K=\dfrac{1}{[Cl_2]}$

and

$K_p=\dfrac{1}{P_{Cl_2}}$

This reaction contains two pure solids ( $Fe_3O_4$ and $Fe$ ), which do not appear in the equilibrium constant expressions. The two gases do, however, appear in the expressions:

$K=\dfrac{[H_2O]^4}{[H_2]^4}$

and

$K_p=\dfrac{(P_{H_2O})^4}{(P_{H_2})^4}$

Exercise $\PageIndex{1}$

Write the expressions for $K$ and $K_p$ for the following reactions.

$CaCO_{3(s)} \rightleftharpoons CaO_{(s)}+CO_{2(g)}$
$\underset{glucose}{C_6H_{12}O_{6(s)}} + 6O_{2(g)} \rightleftharpoons 6CO_{2(g)}+6H_2O_{(g)}$

Answer:

$K = [CO_2]$ and $K_p = P_{CO_2}$
$K=\dfrac{[CO_2]^6[H_2O]^6}{[O_2]^6}$ and $K_p=\dfrac{(P_{CO_2})^6(P_{H_2O})^6}{(P_{O_2})^6}$

For reactions carried out in solution, the concentration of the solvent is omitted from the equilibrium constant expression even when the solvent appears in the balanced chemical equation for the reaction. The concentration of the solvent is also typically much greater than the concentration of the reactants or products (recall that pure water is about 55.5 M, and pure ethanol is about 17 M). Consequently, the solvent concentration is essentially constant during chemical reactions, and the solvent is therefore treated as a pure liquid. The equilibrium constant expression for a reaction contains only those species whose concentrations could change significantly during the reaction.

The concentrations of pure solids, pure liquids, and solvents are omitted from equilibrium constant expressions because they do not change significantly during reactions when enough is present to reach equilibrium.

Summary

An equilibrated system that contains products and reactants in a single phase is a homogeneous equilibrium; a system whose reactants, products, or both are in more than one phase is a heterogeneous equilibrium.

Summary

Support Center

How can we help?