Grading on a Curve
- Page ID
- 2891
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In education, grading on a curve is a statistical method of assigning grades designed to yield a pre-determined distribution of grades among the students in a class. The term "curve" refers to the "bell curve," the graphical representation of the probability density of the normal distribution (also called the Gaussian distribution).
The Process
The method of applying a curve in Chem 2C (Spring Quarter) uses these three steps:
- First, numeric scores are assigned to the students.
- In the second step these scores are converted to relative percentiles.
- Finally, the percentile values are transformed to grades according to a division of the percentile scale into intervals, where the interval width of each grade indicates the desired relative frequency for that grade.
Step 1: Absolute Scores
The grading schemes available for the grade in Chem 2C is:
25% midterm1 + 25% midterm2 + 40% final + 10% lab
Step 2: Conversion of Scores to Percentages
Since curving is designed to normalize the class to a known average, the absolute grade for a specific student is not the relevant measure of performance. The proper measure is the deviation from the mean (in factors of standard deviation).
For example: Student A in class #1 may get a 90% on the final score, whereas student B in class #2 may get a 85% Which student gets the higher grade for the class?
- On an absolute grading scale, one would presume student A would get the higher grade, however a grade based on a curve requires more information to answer.
- If the mean for class #1 is 84% and the mean for class #2 is 70%, then student B has a greater deviation from the respective mean of the class (85%-70%=15%) vs. (90%-84%=6%) for student A.
- This is still not enough, since it is necessary to ask how great of a spread of scores around the mean is the distribution. This is quantified via a standard deviation. Hence, if the standard deviation of the scores in class #1 is 6% and for class #2 it is 20%, then student A is 1.0 standard deviations above the mean and student B is 15/20=0.75 standard deviations above the mean. The answer to which student gets the higher grade is student A since he/she is a greater number of deviations above the mean. This is the only meaningful measure for a student's performance with a mean.
The Importance of having a score distribution centered around 50%
Believe it or not, the best grade distribution is centered around 50%. That is, the class mean is in the middle of possible range of scores, which provides students the full range of opportunity to excel (by having a greater opportunity to deviate from the mean). Distributions with higher averages (e.g. 75% to 80%) may initially appear great to the student (mostly by boosting egos), but really limit the students abilities to perform well. For example, if a class had a final average of 80%, and a standard deviation of 15%, then the BEST any student can do (100% absolute score) is to get 20/15= 1.33 x standard deviation above the mean. As shown below, that can mean a potential maximum of a A- for the class. This is a greatly undesired result and does a grave disservice to advance students.
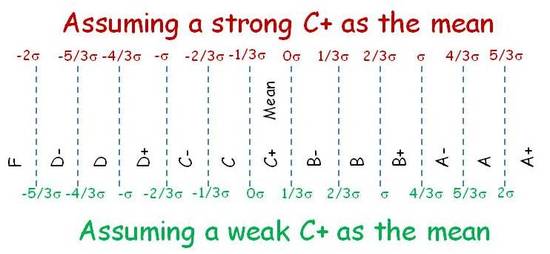
Step 3: Constructing the Curve via the Percentages (or deviations from mean)
The "curve" or grading distribution is based on the below figure shown for two different set points for the mean (a weak or a strong C+). The mean was set to a weak C+ based on the grade outcome and available score augmentations in the class.
Calculate Grades before Contacting Prof. Larsen to Review Final Scores
Before contacting Prof. Larsen regarding scores, you must follow all three steps above. Please calculate the number of standard deviations your final grade is above (or below) the mean. Here are the relevant statistics for the Chem2C (Spring quarter) class to review:
Mean= 72.1164, std. dev.=13.3321
So if a student gets an absolute score of 85.0% for grading Mode 1 and 87.0% for grading Mode 2, then the percentage deviations are
(85.0-72.1164)/13.3321 = 0.96635 std. dev. above the mean
Extra credit may push the student over the edge, but this is calculated in the absolute scores (but not for calculating the mean and std. dev.).
Remember the absolute score is only step 1 of the three steps need to calculate a student's grade. DO NOT EMAIL PROF. LARSEN WITH DISCUSSION OF ABSOLUTE SCORES without completing steps 2 and 3 above.

