Crystal field theory is successful at providing some general insights into the differing energy levels of d orbitals in coordination complexes. That knowledge can help us to understand some of the magnetic properties of these compounds, which are determined by the number of unpaired electrons. It can also help us to understand some of the absorbed wavelengths of light in the UV-visible spectrum, which in some cases depends on the energy difference between different sets of d orbitals.
Ligand field theory picks up where crystal field theory left off by taking into consideration the importance of orbital overlap for covalent bonding. That factor adds another level of detail to our model of metal complex behavior, and it provides additional nuance to our understanding of why two related complexes may have very different properties.
We can start by taking another look at octahedral coordination geometry. That’s probably the most common coordination geometry. It’s also the one we looked at when we considered crystal field theory. In this geometry, all six ligands are located along the x, y, or z axes.
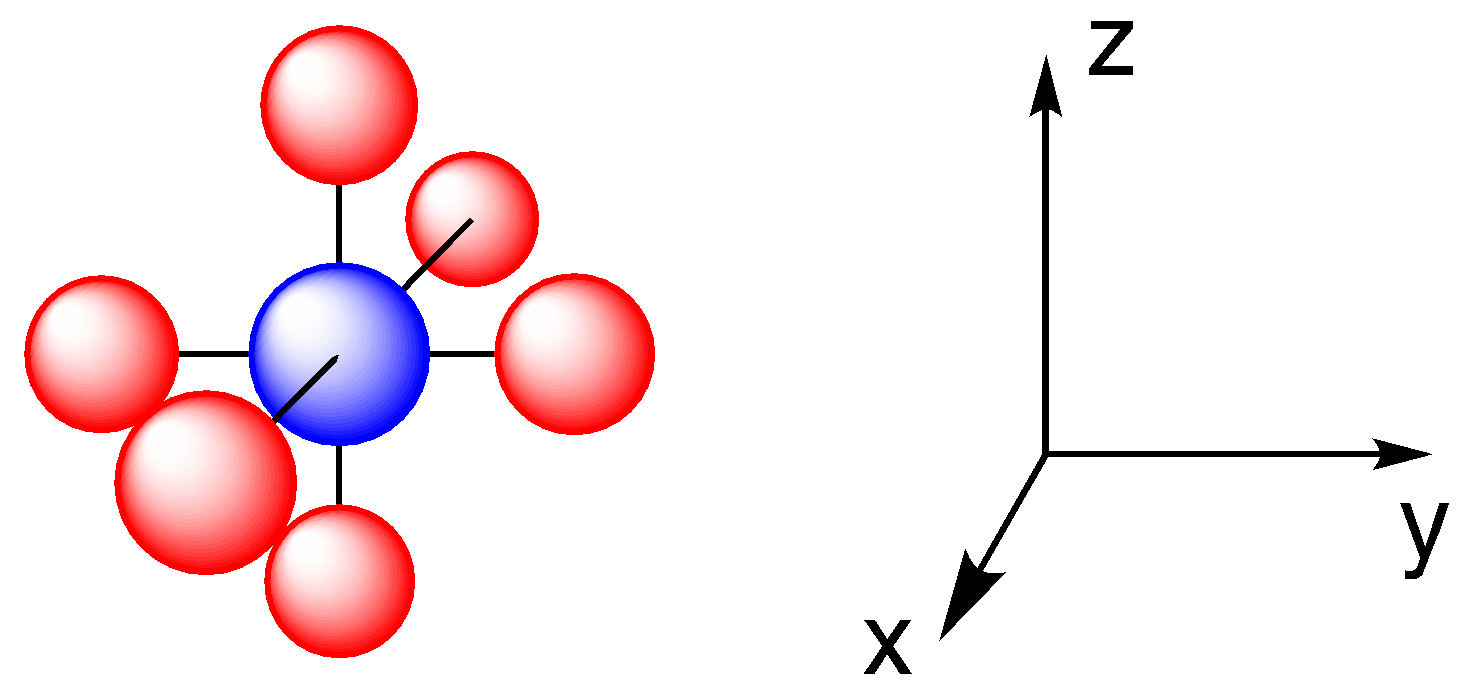
Once again, only two of the valence d orbitals are located along those axes. In crystal field theory, we considered that orientation as an opportunity for electron-electron repulsion between ligand lone pairs (or charges on neighboring anions) and the d electrons. This time, we’ll think about the opportunity for covalent bonding that exists between ligand orbitals and the d orbitals along these axes.
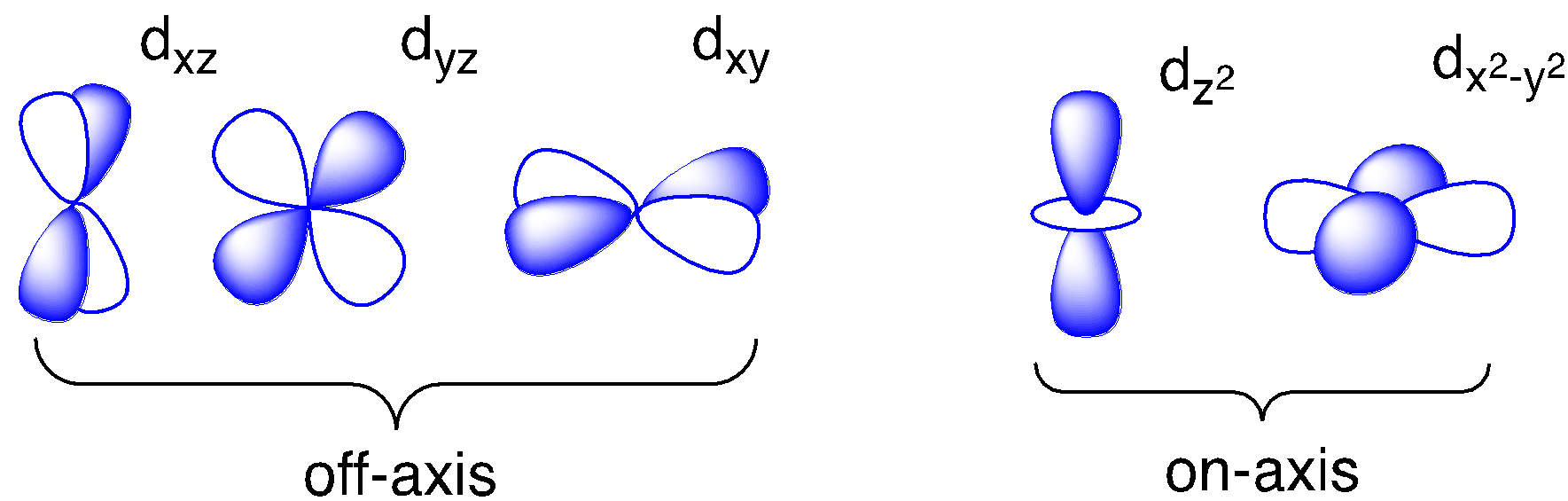
In addition to the d orbitals, we should also think about the potential for bonding with the metal’s valence s and p orbitals. All three p orbitals are located along the axes where we find the ligands. They are ideally located for bonding with ligand orbitals along those axes. The s orbital is different: it is non-directional. No matter where a ligand is located, if it has sigma bonding, it will have the same overlap with that spherical s orbital. That means the s orbital is a good candidate for forming a covalent sigma bond with any ligand, no matter what the geometry.
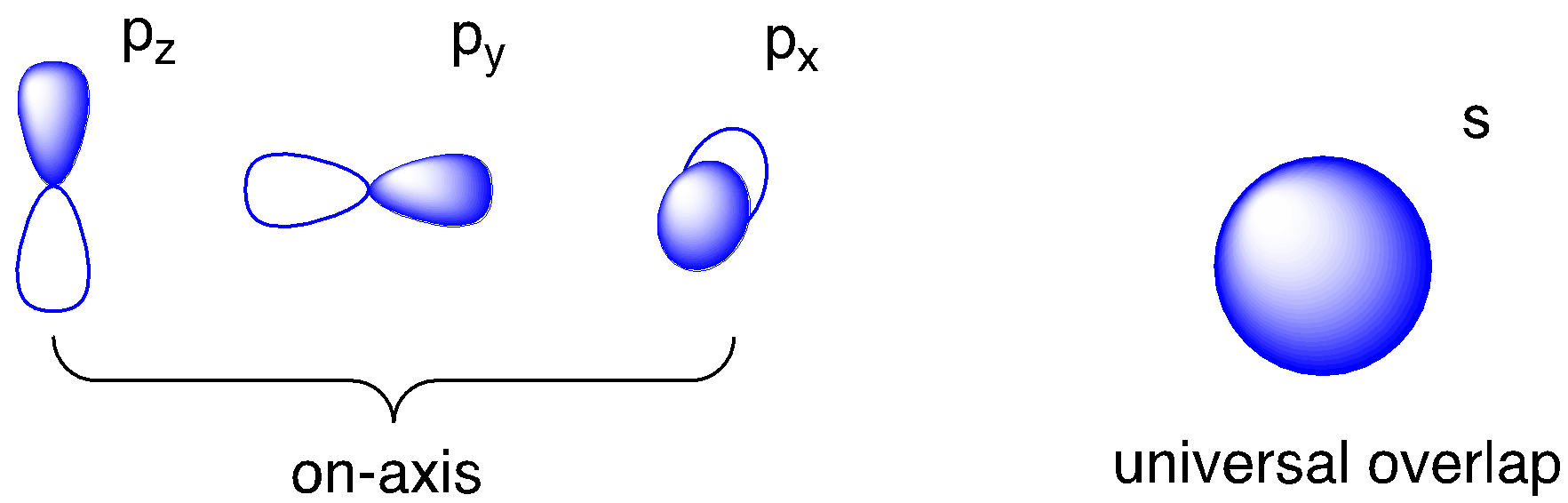
That leaves us with six possible metal orbitals that could potentially overlap with the six ligands. Each metal orbital could potentially interact with any one of a number of these ligands. In the illustration below, we’ve made the assumption that the ligand orbitals are p orbitals. That’s not a bad bet given that we are generally considering p-block elements as donor atoms in most ligands. The s orbital could presumably interact with up to six ligands. The metal p orbitals could interact with ligand orbitals in either direction along the axis. By inspection, it appears that the d orbitals could interact with two or four orbitals.
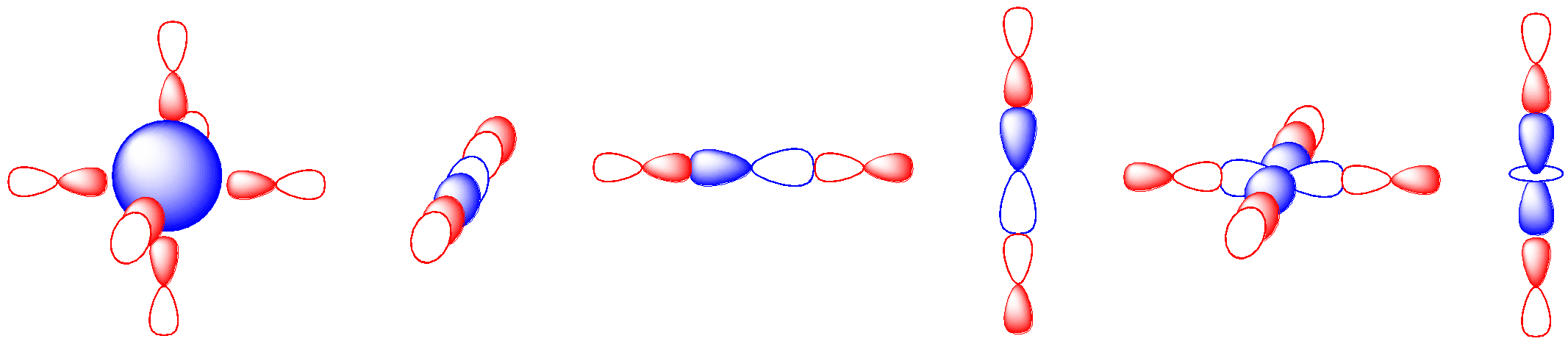
That ratio of six ligand orbitals to six ligand orbitals is coincidental. A one-to-one correspondence between metal orbitals and ligand orbitals isn’t strictly required, but it’s easier to think about that way. Let’s begin by assuming that the s orbital interacts with just one donor orbital. Just like in MO construction for main group diatomics, if we start with two atomic orbitals and combine them, we get two molecular orbitals. One of them is the in-phase combination and the other one is the out-of-phase combination. The number of molecular orbitals we obtain is the same as the number of atomic orbitals we start with.
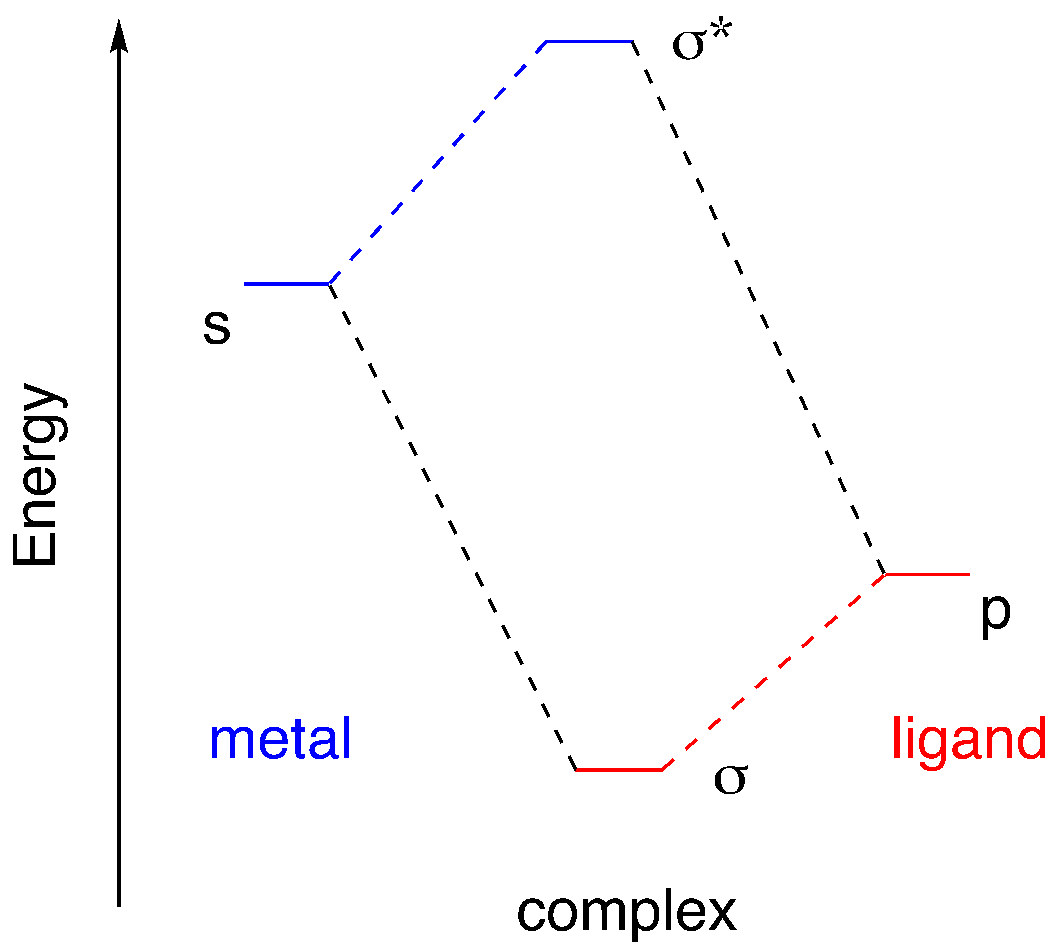
We can think of these two combinations as the addition of two orbitals together, with a positive coefficient if they are in phase and a negative coefficient if they are out of phase.
ψM-L = aψM(s) + bψL(p)
ψ*M-L = cψM(s) - dψL(p)
Notice that the molecular orbital interaction diagram is asymmetric. The ligand orbital is at lower energy than the metal orbital. The donor atom is a p block element; it’s to the right of the transition metals in the periodic table. The donor atom is more electronegative than the transition metal, so its electrons are at lower energy. In a case like that, the coefficients in the orbital combination follow a pattern. In the in-phase combination, the more electronegative element gets a larger coefficient than the less electronegative element (b > a). The opposite is true in the out-of-phase combination (c > d).
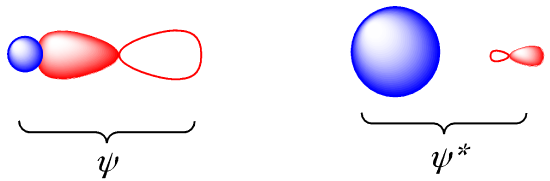
The molecular orbital more closely resembles the atomic orbital to which it is closest in energy, both spatially and energetically. Consequently, we often talk about the bonding orbital, σ, as though it is still a ligand p orbital, and the antibonding orbital, σ, as though it is still a metal s orbital. The bonding orbital is ligand-centered and the antibonding orbital is metal-centered.
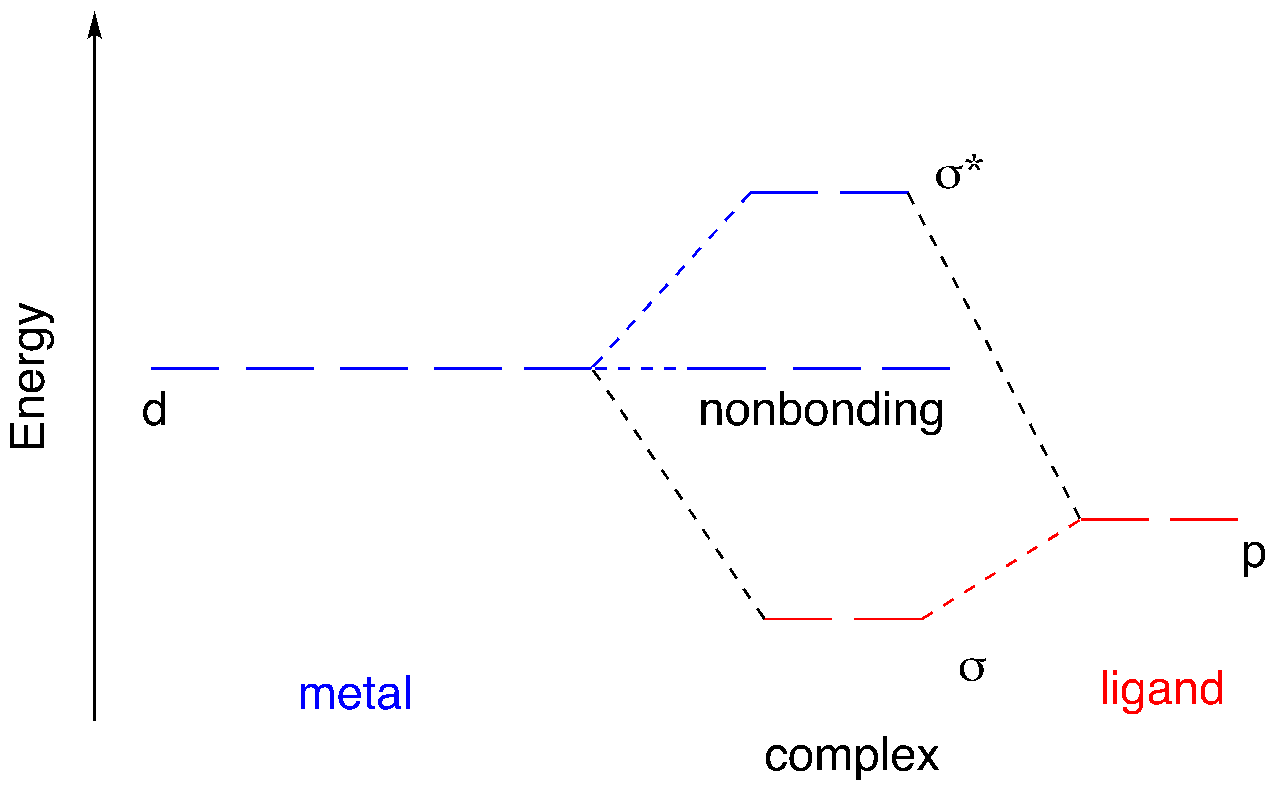
If we consider the three metal p orbitals and their overlap with three of the ligand donor orbitals, we find a very similar outcome. The three σ* orbitals are metal-centered, whereas the three σ orbitals are ligand-centered.

The d orbitals, on the other hand, present a significant variation. Only two of them appear to be capable of overlap with ligand p orbitals. If we pair those two d orbitals off with the remaining two ligand donor orbitals, we have three leftover metal d orbitals. Those three metal orbitals do not overlap with the ligand and so they are non-bonding.
We can superimpose those three pictures to get a look at the full molecular orbital interaction diagram. It is still somewhat simplified. We are ignoring any core orbitals on the metal and we are also ignoring any ligand orbitals that aren’t involved in sigma bonding to the metal. Maybe we have ammine ligands but we are ignoring all of those N-H bonds and focusing solely on the M-N bonds.
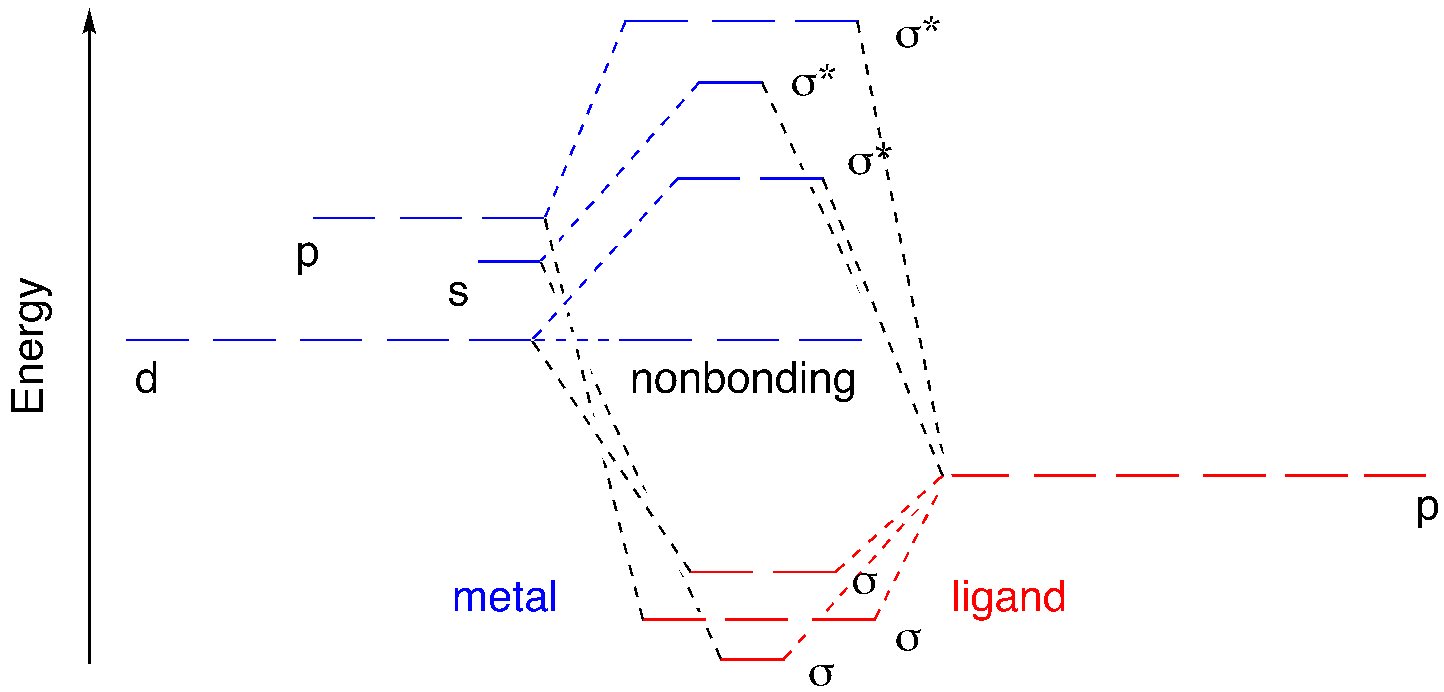
The six donor atoms are each donating a pair of electrons to the metal. Upon covalent bond formation, each of those lone pairs slides down into an energy well; they are stabilized by formation of the metal-ligand bond.
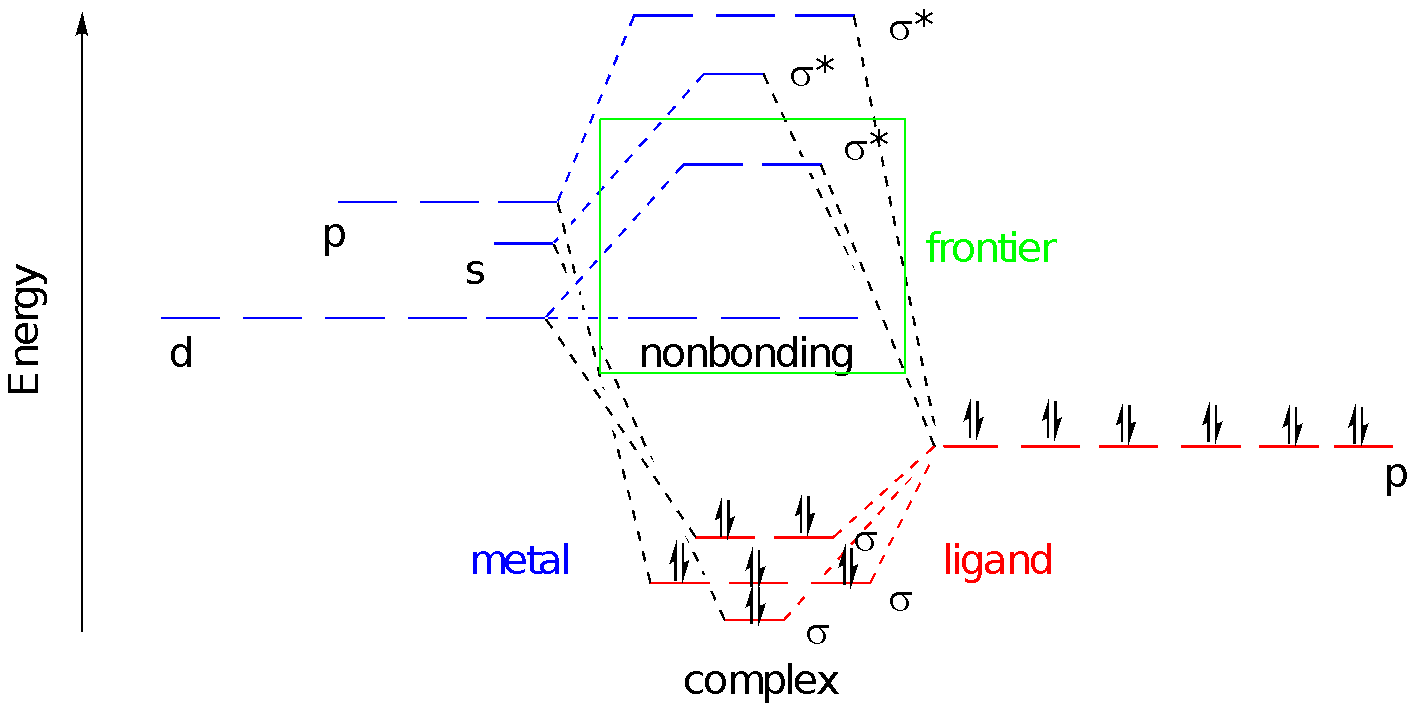
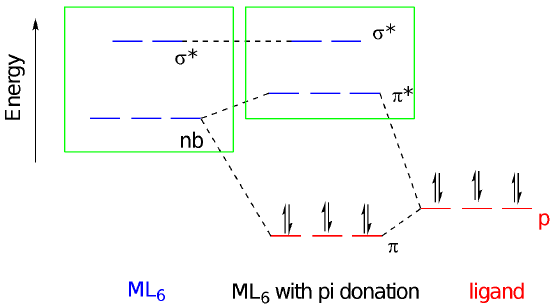
So far, we have ignored the electrons on the metal because we are looking at a general case. There could be anywhere from zero to ten electrons in the atomic d orbitals, depending on the metal and its oxidation state. These electrons will be crucial to many aspects of the behavior of the complex because they are found at the frontier. Frontier orbitals are often the key to understanding reactivity as well as physical properties such as magnetism and interaction with light.
We often consider only these frontier orbitals when we consider the properties of transition metal complexes. We ignore what seems like the most important part of the picture: the bonding orbitals. We do that because those bonding orbitals are not frontier orbitals. Furthermore, the stability of those bonding orbitals is reflected in the antibonding level. The lower the bonding electrons sink, the higher the antibonding levels rise. One immediate consequence of that connection is that the bond strength may control the gap between nonbonding and antibonding d orbitals. Strong sigma donors provide a large d-d gap, whereas weak sigma donors give a smaller d-d gap. Understanding donor strength can be a difficult task, but remember for example that more basic ligands are often stronger donors.
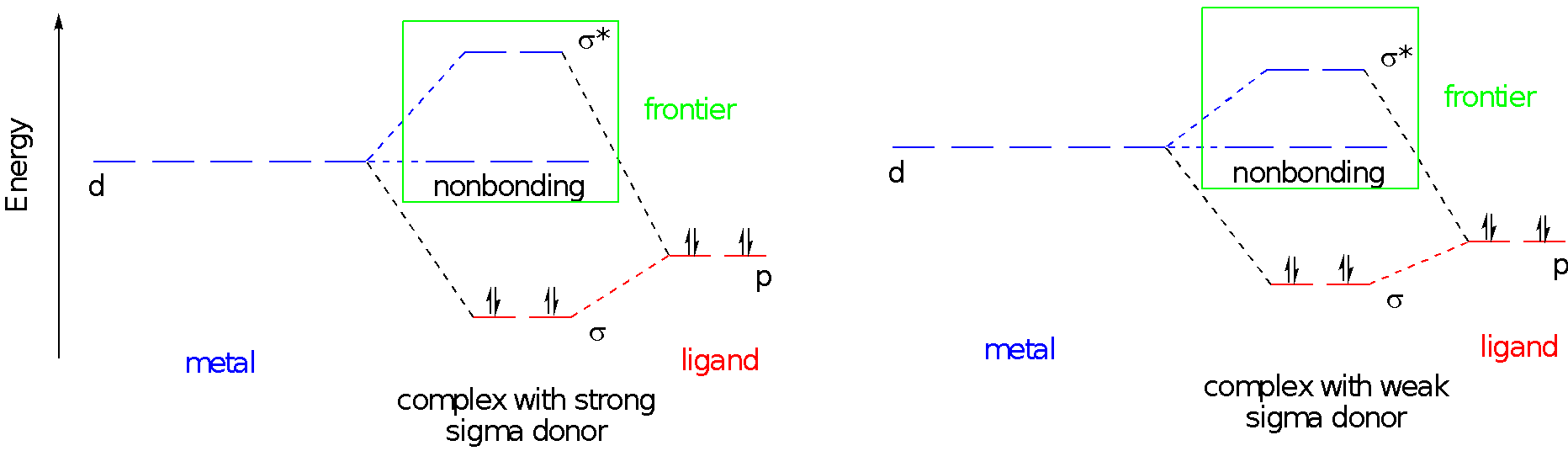
If we think about a specific case of metal ion, we can add some d electrons to the picture. Suppose we have a d4 metal ion. Maybe it’s a Cr2+ ion. This ion can take on two different electron configurations in an octahedral environment. With stronger donors, the d-d gap is potentially too large to promote a fourth electron into the next energy level, so the complex is left with two unpaired electrons. With weaker donors, the relatively small d-d gap may be smaller than the energy it takes to put two electrons into the same orbital, so there are four unpaired electrons. We’ll return to the consequences of these situations on an upcoming page.
Pi Donor Ligands
The sigma donor strength of the ligand can have an appreciable effect on the d orbital splitting in the complex, and that might influence the properties of the complex. That’s not something that we considered in crystal field theory, but thinking about bonding interactions has provided another layer of information. What other variations in ligand donation might play a role in the d orbital splitting?
Suppose a donor atom had more than one lone pair. Could it donate a second? On paper, that makes another bond between the ligand and the metal. Bond formation is energy-releasing and stabilizing, although sometimes we get in trouble when we draw too many bonds, because we’ve run out of orbitals to interact with each other.
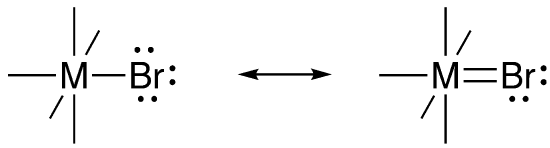
When we draw a pair of bonds between two atoms, we usually think of the first bond as a sigma bond and the second one as a pi bond. The question here is, can bromine form a pi bond with a transition metal? When we learn about pi bonds, we start by looking at two nitrogen atoms or two carbon atoms using parallel p orbitals to bond with each other. The resulting pi bond avoids the sigma bond because it is above and below the bond axis. We have already established that the metal can use its p orbitals for bonding. On paper, a metal p orbital in an octahedral complex could pi bond with four different ligands.
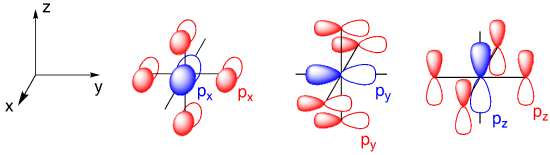
The trouble with that scheme is that we are already using these metal p orbitals for sigma bonding. We have other metal orbitals that could overlap with these ligand p orbitals, however, and we aren’t using them for sigma bonding. These are the dxy, dxz, and dyz orbitals. This set looks even more promising for pi bonding; the d orbitals reach out towards the ligand p orbitals, maximizing overlap. Once again, each d orbital could potentially form pi bonds with up to four ligands.
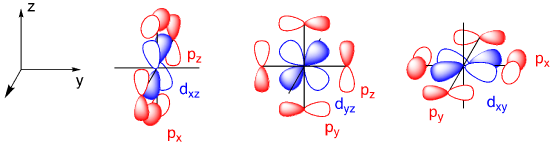
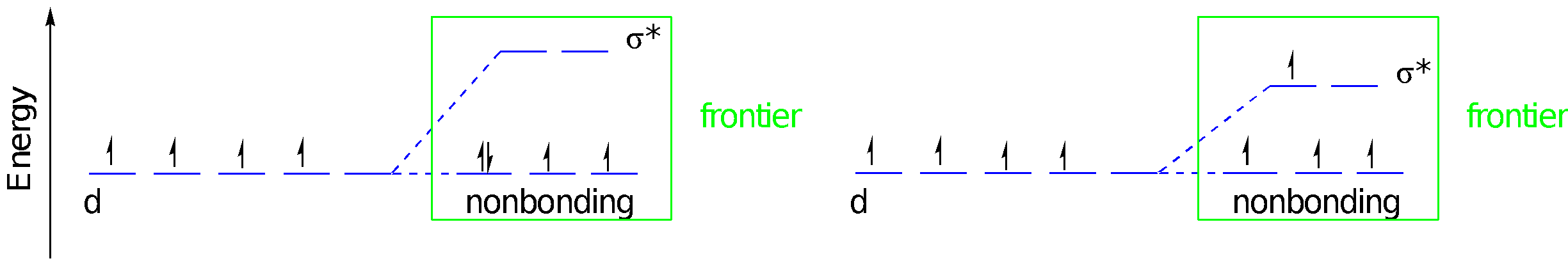
Let’s consider the consequences of pi bond formation using the molecular orbital interaction diagram. This time we’re just considering lone pairs on three ligands that could donate to the three different d orbitals of appropriate symmetry: the dxy, dyz, and dxz. We get a bonding and an antibonding combination, but the three ligand pairs all go down in energy. As long as there are fewer than six d orbitals there is a net decrease in energy. Just like before, when these orbitals combine, the bonding combination has more ligand character whereas the antibonding combination has more metal character. The key change in terms of the frontier orbitals is that the d orbital splitting becomes smaller than in the case of the simple sigma bond donor.
Ligand-to-metal donation is just one way to make a metal-ligand double bond. In those cases in which the metal has d electrons, the double bond could also be formed by donation from the metal to the ligand. The d electrons are most likely to occupy the dxy, dxz, and dyz, as those orbitals are the lowest available ones. Those are the same orbitals that were involved in π bonding in ligand-to-metal donation. In addition to d electrons, there must also be an acceptor orbital on the ligand. That is to say, there must be an empty orbital on the ligand with π symmetry. Instead of a lone pair, this acceptor takes the form of a π* orbital on the ligand. That means there has to be a π bond between the donor atom and a second atom within the ligand. Carbon monoxide is the classic example of such a ligand.
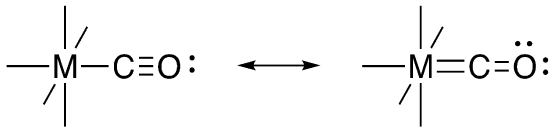
Metal-to-ligand π donation has the effect of breaking a π bond in the ligand because of the fact that electrons are donated into a ligand π* orbital. In terms of Lewis structures, this looks like an interaction that would strengthen the metal-ligand bond but weaken bonds within the ligand itself. When a metal π donates into a carbon monoxide, populating the CO π* orbital, the CO bond gets weaker.
Because we are still looking at π bond formation, the orbital pictures look somewhat similar to the ones for ligand-to-metal π donation, but with π* molecular orbitals instead of p atomic orbitals.
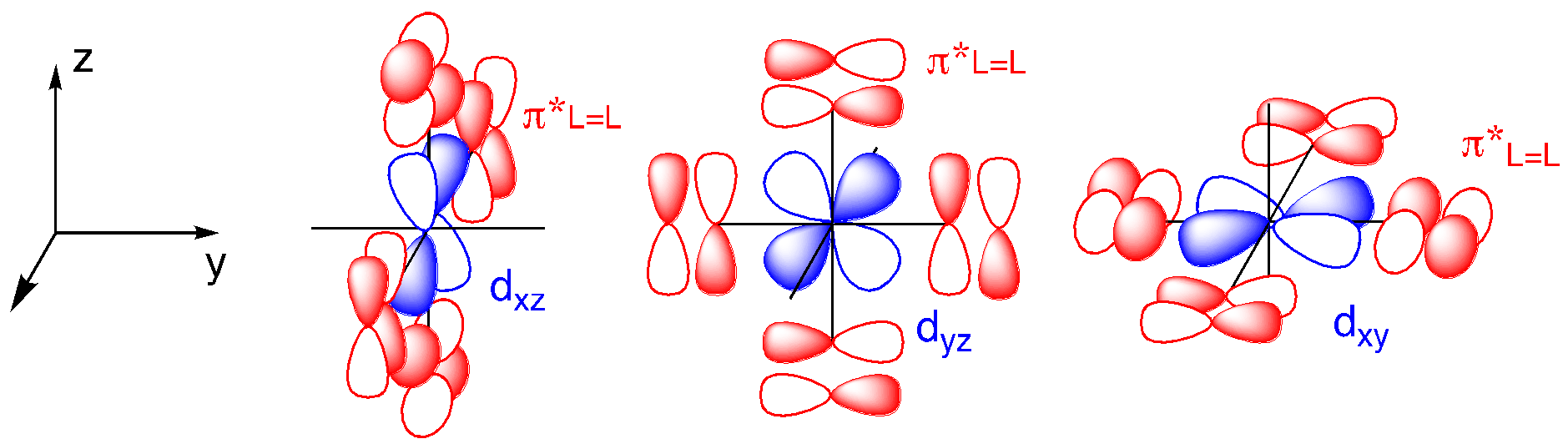
This time, the relevant ligand orbitals are higher in energy than the metal orbitals because they are antibonding. Antibonding orbitals are typically higher in energy than atomic orbitals. That means that when we construct orbital combinations from these pairs, the metal-ligand pi bonding orbital has more metal character. Conversely, the metal-ligand pi antibonding orbital has more ligand character. If there are any d electrons, they will drop into a lower energy well through formation of the pi bond. The ligand π* molecular orbitals are raised in energy, but that change has no energetic consequences since there aren’t any electrons there.
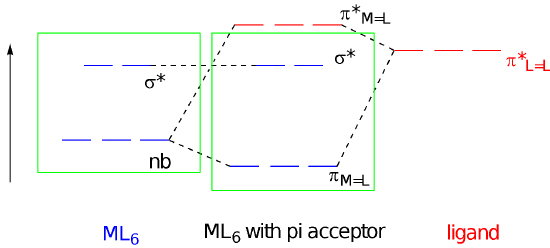
In contrast to ligand-to-metal pi bond donation, metal-to-ligand pi bond formation has the effect of increasing the d orbital splitting. That gives us three different magnitudes of d orbital splitting for three different categories of ligand. The splitting follows the order pi donors < sigma donors < pi acceptors, at least as a rough trend; individual ligands may deviate from this trend for other reasons, such as the strength of sigma donation.
What if the orbital combinations are not obvious? What if you can’t decide by inspection which ligand orbital would overlap with which metal orbital? There is a more general method of evaluating these things using group theory. We should use that approach for the octahedral geometry, because we already have a concrete example of what that should look like. We can use our knowledge of that outcome to build confidence in our results from group theory. Group theory may be a newer approach to us, and so it will be helpful to validate the results.
To get started, we are going to need a character table for an octahedral geometry, and we will need to consider how the bonding orbitals behave under the symmetry operations of this group. You can brush up on point groups and symmetry here.1
The top row of the character table for the octahedral point group organizes the symmetry operations and tells us how many of each operation we can find.
| Oh |
E |
8C3 |
6C2 |
6C4 |
3C2 (= C42) |
i |
6S4 |
8S6 |
3σh |
3σd |
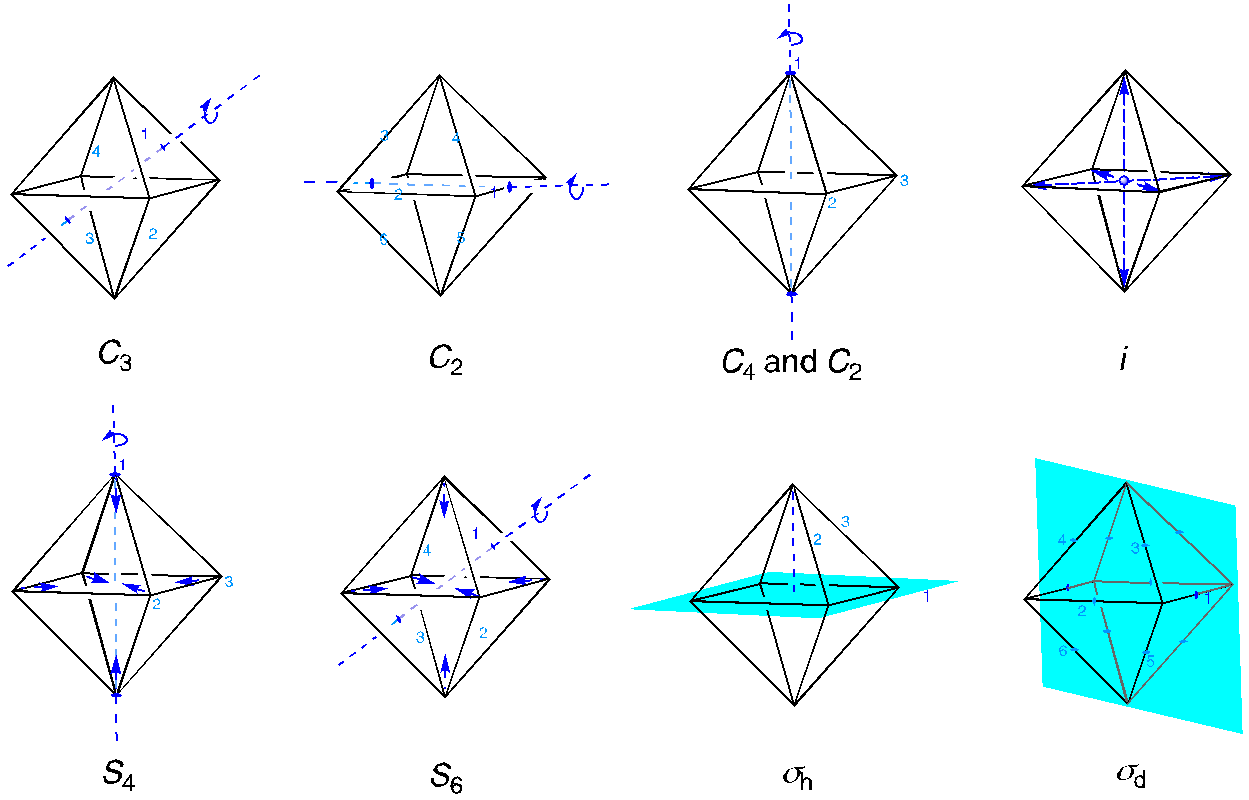
Apart from the identity element, there are ten additional symmetry elements in this point group. The C3 axis passes through the four pairs of opposite faces of the octahedron. We can rotate by either 120 degrees or 240 degrees, making a total of eight operations. Six different C2 axes pass through opposite edges of the octahedron. Additional C2 axes are coincident with the C4 axis passing through opposite corners of the octahedron (because C2 = C42, but not C41 or C43). There is an inversion center at the position of the metal ion. That inversion element makes possible an S4 axis coincident with the C4 axis and an S6 axis coincident with the C3 axis. Finally, there are two sets of mirror planes, one set in the equatorial planes and one set bisecting the faces of the octahedron.
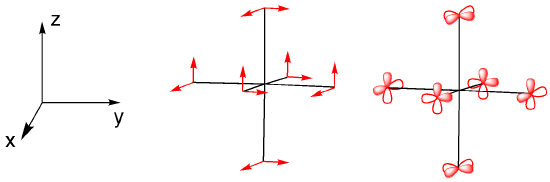
We can use the character table to determine appropriate orbitals for bonding with the ligands. A set of vectors is often used for this purpose but p orbitals can be used just as well. We consider how each of the p orbitals will change under a particular symmetry element.
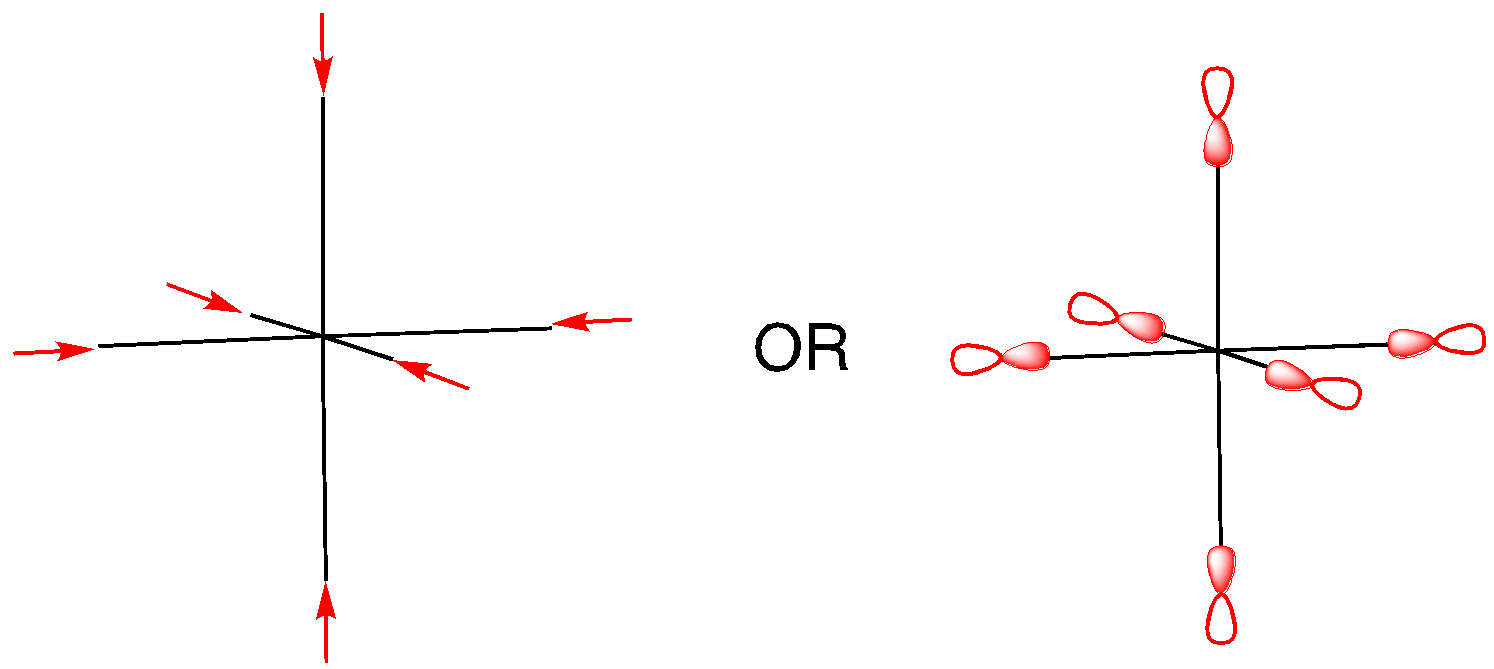
Has a p orbital remained in place, unchanged? That counts as 1. Has it moved into an entirely different position? That counts as 0. Has it remained in place, but with the opposite orientation? That counts as -1.
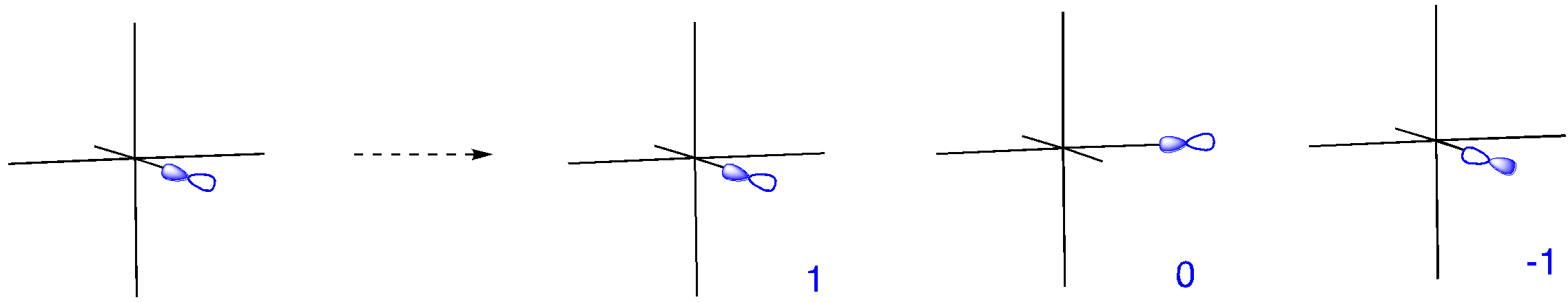
Looking at each of the symmetry elements gives us a reducible representation.
| Oh |
E |
8C3 |
6C2 |
6C4 |
3C2 (= C42) |
i |
6S4 |
8S6 |
3σh |
3σd |
| Γ |
6 |
0 |
0 |
2 |
2 |
0 |
0 |
0 |
4 |
2 |
If we look at the complete character table, we can find the irreducible representations that this reducible representation is composed of.
| Oh |
E |
8C3 |
6C2 |
6C4 |
3C2 (= C42) |
i |
6S4 |
8S6 |
3σh |
3σd |
|
|
| A1g |
1 |
1 |
1 |
1 |
1 |
1 |
1 |
1 |
1 |
1 |
|
x2 + y2 + z2 |
| A2g |
1 |
1 |
-1 |
-1 |
1 |
1 |
-1 |
1 |
1 |
-1 |
|
|
| Eg |
2 |
-1 |
0 |
0 |
2 |
2 |
0 |
-1 |
2 |
0 |
|
(2z2 – x2 – y2, x2 - y2) |
| T1g |
3 |
0 |
-1 |
1 |
-1 |
3 |
1 |
0 |
-1 |
-1 |
(Rx, Ry, Rz) |
|
| T2g |
3 |
0 |
1 |
-1 |
-1 |
3 |
-1 |
0 |
-1 |
1 |
|
(xz, yz, xy) |
| A1u |
1 |
1 |
1 |
1 |
1 |
-1 |
-1 |
-1 |
-1 |
-1 |
|
|
| A2u |
1 |
1 |
-1 |
-1 |
1 |
-1 |
1 |
-1 |
-1 |
1 |
|
|
| Eu |
2 |
-1 |
0 |
0 |
2 |
-1 |
0 |
1 |
-2 |
0 |
|
|
| T1u |
3 |
0 |
-1 |
1 |
-1 |
-3 |
-1 |
0 |
1 |
1 |
(x, y, z) |
|
| T2u |
3 |
0 |
1 |
-1 |
-1 |
-3 |
1 |
0 |
1 |
-1 |
|
|
We find that Γ = A1g + T1u + Eg. If we add together the characters for each of the symmetry elements in those three representations, we get the characters in our reducible representation for the ligand p orbitals.
The A1g representation corresponds to the metal s orbital. The T1u representation corresponds to the three metal p orbitals. The Eg representation corresponds to two of the d orbitals: 2z2 – x2 – y2 (usually abbreviated as z2) and x2 – y2. The other three d orbitals (dxy, dxz, and dyz) have T1u symmetry and are not a match for the set of ligand orbitals that we examined. This is exactly the outcome that we established by looking at metal orbitals and ligand orbitals and deciding which ones would overlap. The match between the two approaches should provide some confidence about the use of character tables to make decisions about symmetry-appropriate bonding.
References
(1) Point Groups https://chem.libretexts.org/@go/page/269925 (accessed Jul 2, 2021).
Example \(\PageIndex{1}\)
The formula for decomposing a reducible representation is based on taking the dot product and normalizing:
\[ai = 1h QN∙ χ(R)∙χ(R)Q \nonumber \]
in which ai is the number of times the irreducible representation appears in the reducible representation; h is the order of the point group (the total number of symmetry operations); N is the number of operations for a given symmetry element, Q; χ(R)is the character of the reducible representation and χ(R)is the character of the irreducible representation.
Use the formula to confirm the finding that, for sigma bonding in an octahedral geometry:
\[ Γ = A_{1g} + T_{1u} + E_g. \nonumber \]
Solution
ai = 1h QN∙ χ(R)∙χ(R)Q
A1g: ai = 148 [1∙6∙1+8∙0∙1+6∙0∙1+6∙2∙1+3∙2∙1+ 1∙0∙1+6∙0∙1 + 8∙0∙1+3∙4∙1+6∙2∙1] = 148 (6+12+ 6+12+12)= 148∙48=1
A2g: ai = 148 [1∙6∙1+8∙0∙1+6∙0∙(-1)+6∙2∙(-1)+3∙2∙1+ 1∙0∙1+6∙0∙(-1) + 8∙0∙1+3∙4∙1+6∙2∙(-1)] = 148 (6-12+ 6+12-12)= 148∙0=0
Eg: ai = 148 [1∙6∙2+8∙0∙(-1)+6∙0∙0+6∙2∙0+3∙2∙2+ 1∙0∙2+6∙0∙0 + 8∙0∙(-1)+3∙4∙2+6∙2∙0] = 148 (12+12+ 24)= 148∙48=1
T1g: ai = 148 [1∙6∙3+8∙0∙0+6∙0∙(-1)+6∙2∙1+3∙2∙(-1)+ 1∙0∙3+6∙0∙1 + 8∙0∙0+3∙4∙(-1)+6∙2∙(-1)] = 148 (18+12- 6-12-12)= 148∙0=0
T2g: ai = 148 [1∙6∙3+8∙0∙0+6∙0∙1+6∙2∙(-1)+3∙2∙(-1)+ 1∙0∙3+6∙0∙(-1) + 8∙0∙0+3∙4∙(-1)+6∙2∙1] = 148 (18-12- 6-12+12)= 148∙0=0
A1u: ai = 148 [1∙6∙1+8∙0∙1+6∙0∙1+6∙2∙1+3∙2∙1+ 1∙0∙(-1)+6∙0∙(-1) + 8∙0∙(-1)+3∙4∙(-1)+6∙2∙(-1)] = 148 (6+12+ 6-12-12)= 148∙0=0
A2u: ai = 148 [1∙6∙1+8∙0∙1+6∙0∙(-1)+6∙2∙(-1)+3∙2∙1+ 1∙0∙(-1)+6∙0∙1 + 8∙0∙(-1)+3∙4∙(-1)+6∙2∙1] = 148 (6-12+ 6-12+12)= 148∙0=0
Eu: ai = 148 [1∙6∙2+8∙0∙(-1)+6∙0∙0+6∙2∙0+3∙2∙2+ 1∙0∙(-2)+6∙0∙0 + 8∙0∙1+3∙4∙(-2)+6∙2∙0] = 148 (12+12- 24)= 148∙0=0
T1u: ai = 148 [1∙6∙3+8∙0∙0+6∙0∙(-1)+6∙2∙1+3∙2∙(-1)+ 1∙0∙(-3)+6∙0∙(-1) + 8∙0∙0+3∙4∙1+6∙2∙1] = 148 (18+12- 6+12+12)= 148∙48=1
T2u: ai = 148 [1∙6∙3+8∙0∙0+6∙0∙1+6∙2∙(-1)+3∙2∙(-1)+ 1∙0∙(-3)+6∙0∙1 + 8∙0∙0+3∙4∙1+6∙2∙(-1)] = 148 (18-12- 6+12-12)= 148∙0=0
Γ = A1g + T1u + Eg.
Example \(\PageIndex{1}\)
Determine the reducible representation for the symmetry of the pi-bonding orbitals in an octahedral geometry. You can use simple vectors to represent the bias of the p orbitals.
Solution
E: All 12 vectors remain in same place in same orientation. The character is 12.
C3: An off-axis rotation, so all vectors have moved. The character is 0.
C2: An off-axis rotation, so all vectors have moved. The character is 0.
C4: An on-axis rotation, but the vectors are off-axis. Note how the labeled vectors make the movement out of position clear. The character is 0.
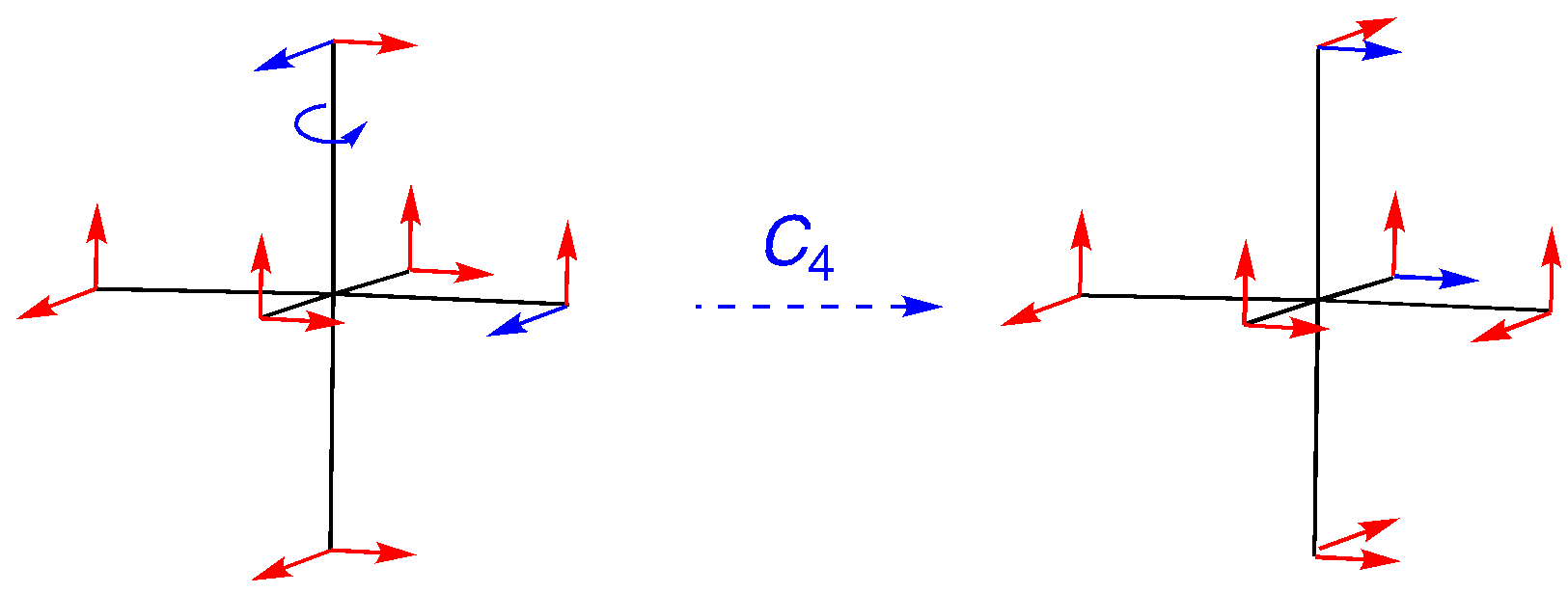
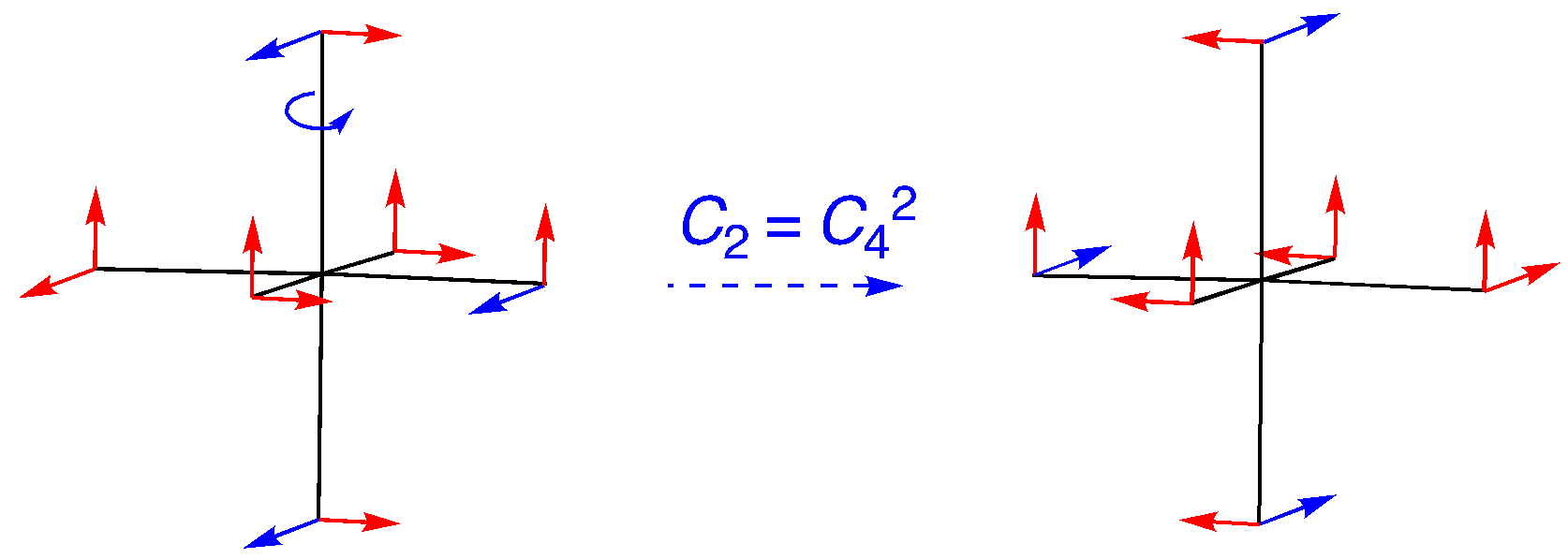
C2 (= C42): An on-axis rotation, but this time the four vectors located along the rotational axis just switch bias. The others move out of position. The character is -4.
i: All 12 vectors move to the opposite side of the structure. The character is 0.
S4: An on-axis rotation, followed by inversion. Note how the labeled vectors make the movement out of position clear. The character is 0.
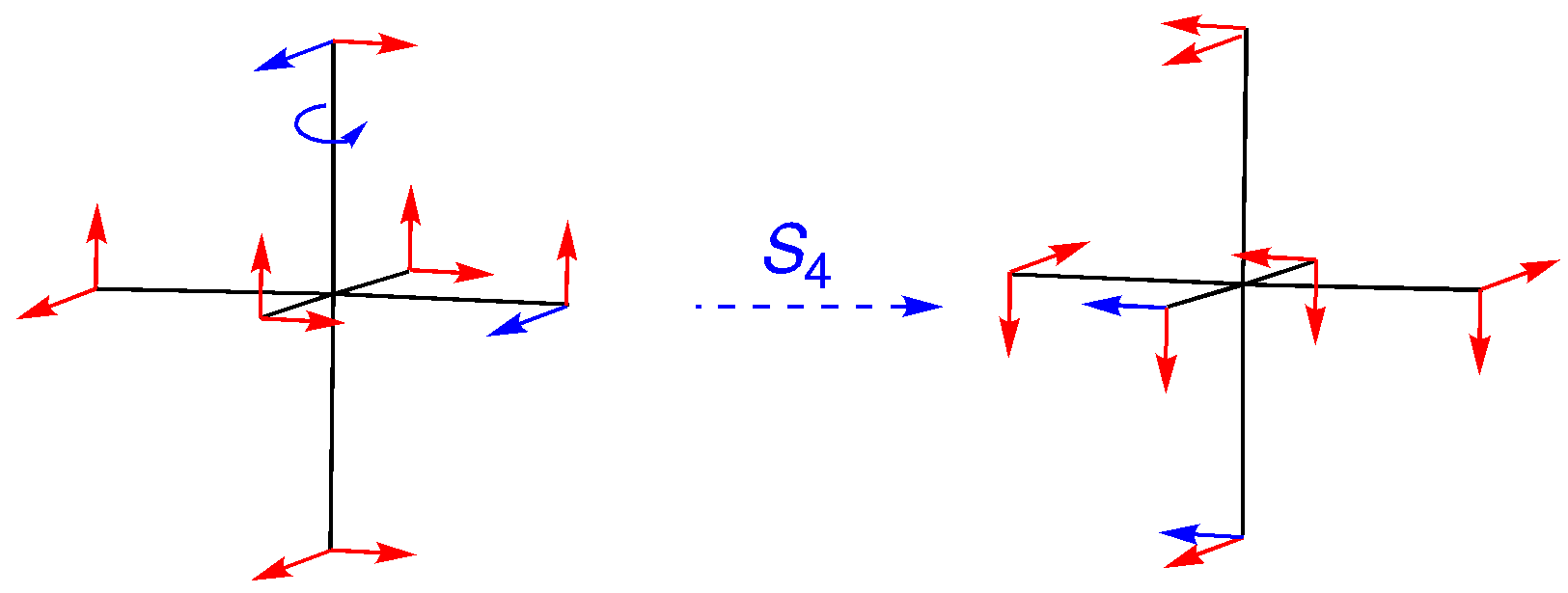
S6: An off-axis rotation, so all vectors have moved. The character is 0.
σh: Four vectors change position (character is 0); four vectors remain in position and keep original bias (character is 4); four vectors remain in position and switch bias (character is -4); net character is 0.
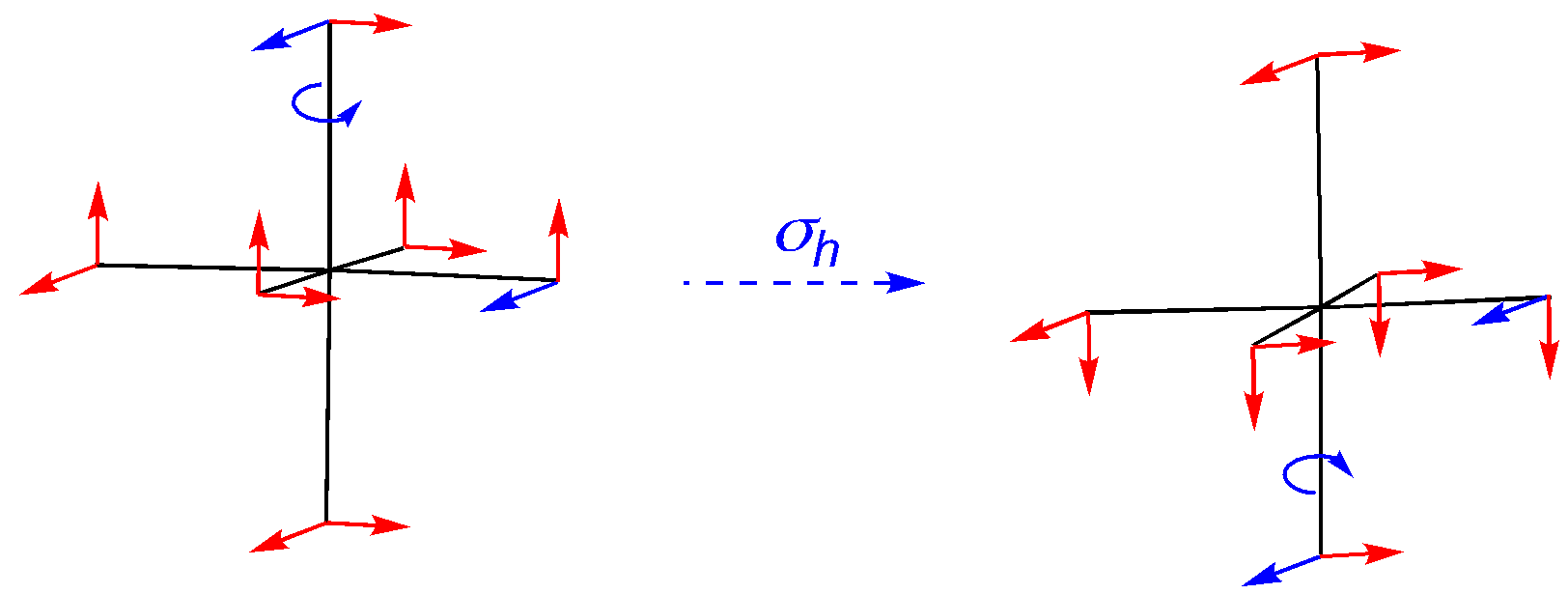
σd: An off-axis reflection, so all vectors have moved. The character is 0.
| Oh |
E |
8C3 |
6C2 |
6C4 |
3C2 (= C42) |
i |
6S4 |
8S6 |
3σh |
3σd |
| Γ |
12 |
0 |
0 |
0 |
-4 |
0 |
0 |
0 |
0 |
0 |


