7.5: Nuclear Fission and Nuclear Fusion

Learning Objectives

• Explain nuclear fission and fusion processes
• Relate the concepts of critical mass and nuclear chain reactions

Nuclear fusion and nuclear fission are two different types of energy-releasing reactions in which energy is released from high-powered atomic bonds between the particles within the nucleus. The main difference between these two processes is that fission is the splitting of an atom into two or more smaller ones while fusion is the fusing of two or more smaller atoms into a larger one.

Protons and neutrons make up a nucleus, which is the foundation of nuclear science. Fission and fusion involves the dispersal and combination of elemental nucleus and isotopes, and part of nuclear science is to understand the process behind this phenomenon. Adding up the individual masses of each of these subatomic particles of any given element will always give you a greater mass than the mass of the nucleus as a whole. The missing idea in this observation is the concept called nuclear binding energy. Nuclear binding energy is the energy required to keep the protons and neutrons of a nucleus intact, and the energy that is released during a nuclear fission or fusion is nuclear power. There are some things to consider however. The mass of an element's nucleus as a whole is less than the total mass of its individual protons and neutrons.

To calculate the energy released during mass destruction in both nuclear fission and fusion, we use Einstein’s equation that equates energy and mass:

\[ E=mc^2 \label{1} \]

with \(m\) is mass (kilograms), \(c\) is speed of light (meters/sec) and \(E\) is energy (Joules).

Nuclear Fission

Many heavier elements with smaller binding energies per nucleon can decompose into more stable elements that have intermediate mass numbers and larger binding energies per nucleon—that is, mass numbers and binding energies per nucleon that are closer to the “peak” of the binding energy graph near 56. Sometimes neutrons are also produced. This decomposition is called fission, the breaking of a large nucleus into smaller pieces. The breaking is rather random with the formation of a large number of different products. Fission usually does not occur naturally, but is induced by bombardment with neutrons. The first reported nuclear fission occurred in 1939 when three German scientists, Lise Meitner, Otto Hahn, and Fritz Strassman, bombarded uranium-235 atoms with slow-moving neutrons that split the U-238 nuclei into smaller fragments that consisted of several neutrons and elements near the middle of the periodic table. Since then, fission has been observed in many other isotopes, including most actinide isotopes that have an odd number of neutrons. A typical nuclear fission reaction is shown in Figure \(\PageIndex{2}\).

Figure \(\PageIndex{2}\): When a slow neutron hits a fissionable U-235 nucleus, it is absorbed and forms an unstable U-236 nucleus. The U-236 nucleus then rapidly breaks apart into two smaller nuclei (in this case, Ba-141 and Kr-92) along with several neutrons (usually two or three), and releases a very large amount of energy.

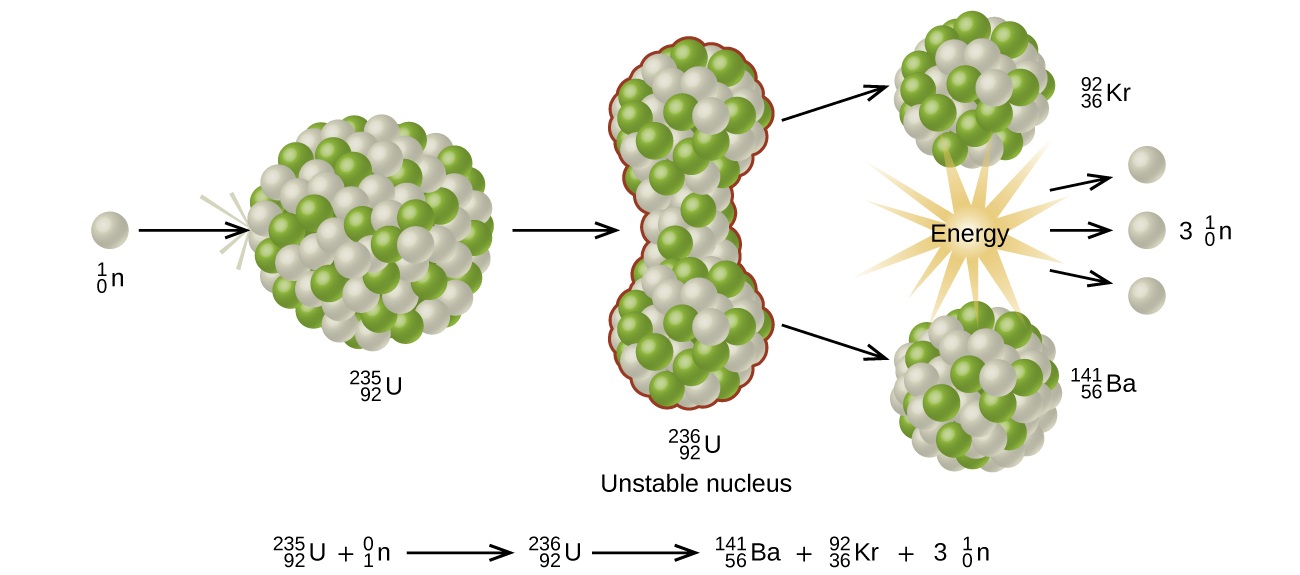
Among the products of Meitner, Hahn, and Strassman’s fission reaction were barium, krypton, lanthanum, and cerium, all of which have nuclei that are more stable than uranium-235. Since then, hundreds of different isotopes have been observed among the products of fissionable substances. A few of the many reactions that occur for U-235, and a graph showing the distribution of its fission products and their yields, are shown in Figure \(\PageIndex{3}\). Similar fission reactions have been observed with other uranium isotopes, as well as with a variety of other isotopes such as those of plutonium.

Figure \(\PageIndex{3}\): (a) Nuclear fission of U-235 produces a range of fission products. (b) The larger fission products of U-235 are typically one isotope with a mass number around 85–105, and another isotope with a mass number that is about 50% larger, that is, about 130–150.

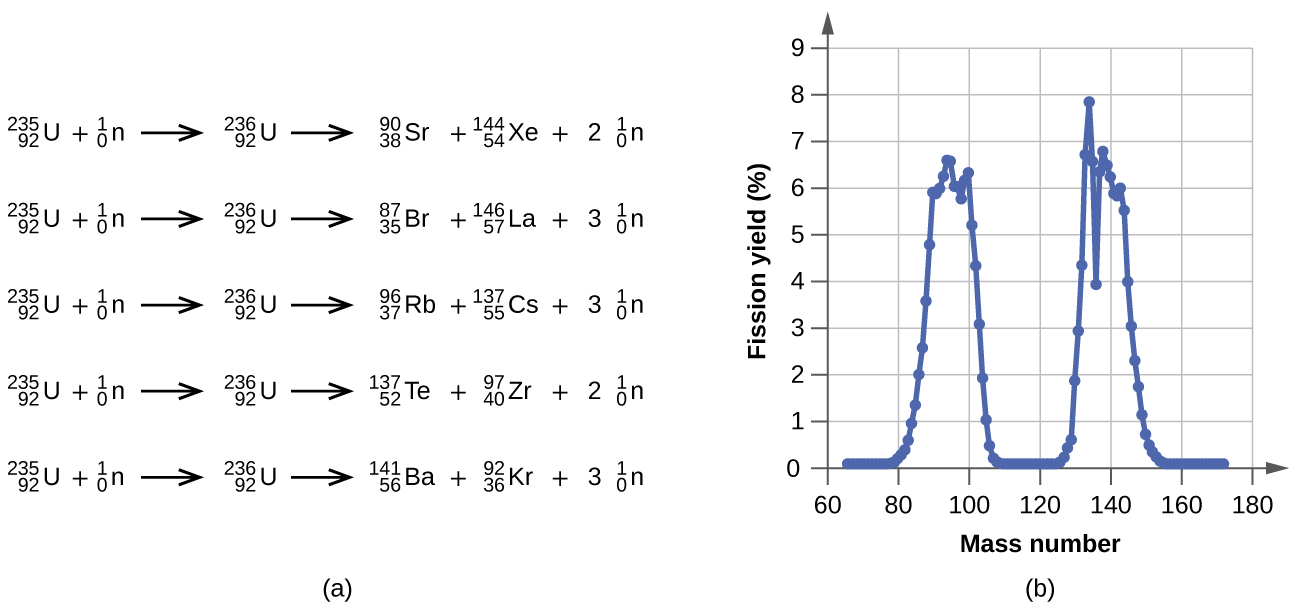
A tremendous amount of energy is produced by the fission of heavy elements. For instance, when one mole of U-235 undergoes fission, the products weigh about 0.2 grams less than the reactants; this “lost” mass is converted into a very large amount of energy, about 1.8 × 10¹⁰ kJ per mole of U-235. Nuclear fission reactions produce incredibly large amounts of energy compared to chemical reactions. The fission of 1 kilogram of uranium-235, for example, produces about 2.5 million times as much energy as is produced by burning 1 kilogram of coal.

As described earlier, when undergoing fission U-235 produces two “medium-sized” nuclei, and two or three neutrons. These neutrons may then cause the fission of other uranium-235 atoms, which in turn provide more neutrons that can cause fission of even more nuclei, and so on. If this occurs, we have a nuclear chain reaction (Figure \(\PageIndex{4}\)). On the other hand, if too many neutrons escape the bulk material without interacting with a nucleus, then no chain reaction will occur.

Figure \(\PageIndex{4}\): The fission of a large nucleus, such as U-235, produces two or three neutrons, each of which is capable of causing fission of another nucleus by the reactions shown. If this process continues, a nuclear chain reaction occurs.

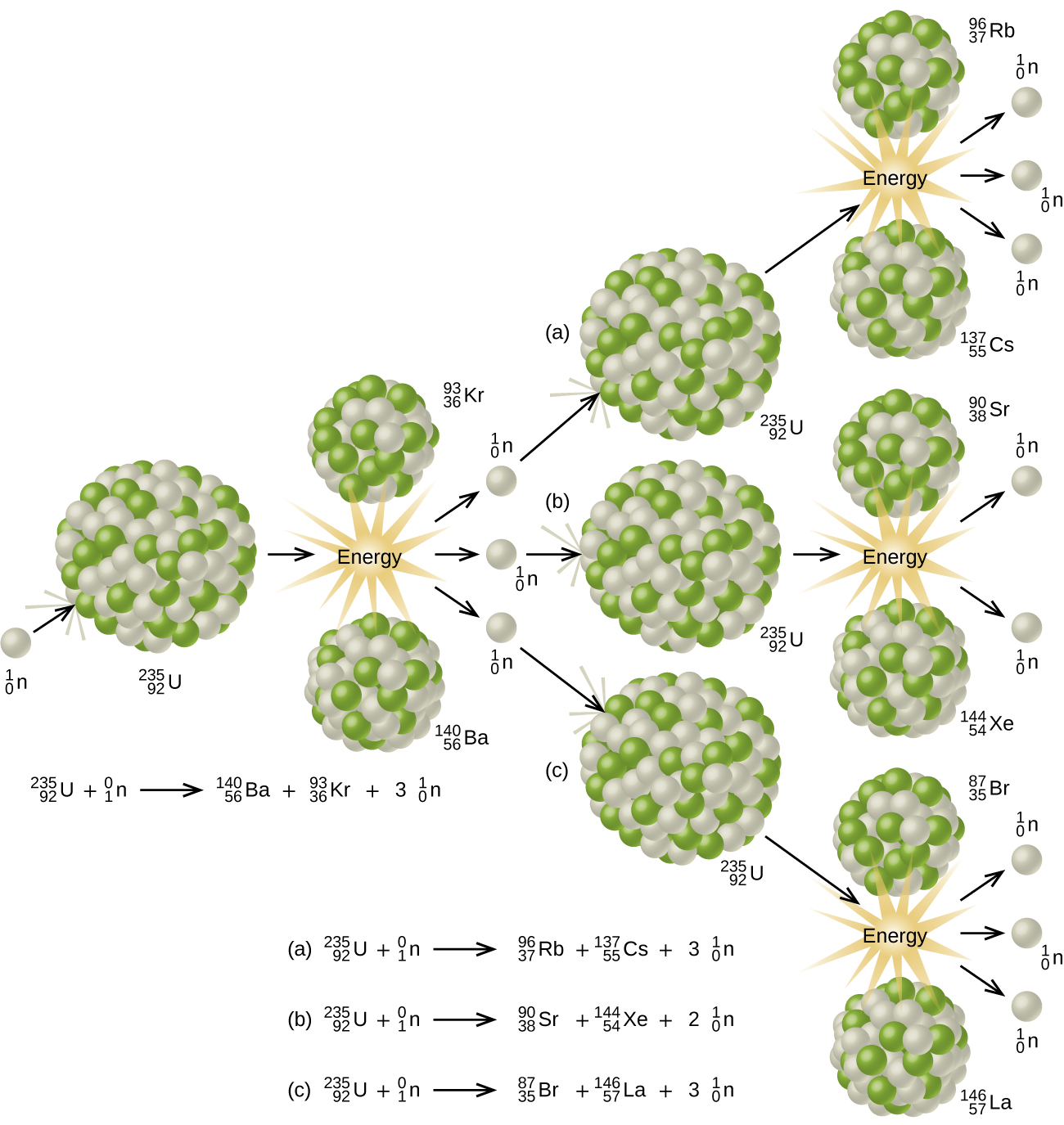
Material that can sustain a nuclear fission chain reaction is said to be fissile or fissionable. (Technically, fissile material can undergo fission with neutrons of any energy, whereas fissionable material requires high-energy neutrons.) Nuclear fission becomes self-sustaining when the number of neutrons produced by fission equals or exceeds the number of neutrons absorbed by splitting nuclei plus the number that escape into the surroundings. The amount of a fissionable material that will support a self-sustaining chain reaction is a critical mass. An amount of fissionable material that cannot sustain a chain reaction is a subcritical mass. An amount of material in which there is an increasing rate of fission is known as a supercritical mass. The critical mass depends on the type of material: its purity, the temperature, the shape of the sample, and how the neutron reactions are controlled (Figure \(\PageIndex{5}\)).

Nuclear power plants are designed in such a way that they cannot form a supercritical mass of fissionable material and therefore cannot create a nuclear explosion. But as history has shown, failures of systems and safeguards can cause catastrophic accidents, including chemical explosions and nuclear meltdowns (damage to the reactor core from overheating). The following Chemistry in Everyday Life feature explores three infamous meltdown incidents.

The energy produced by a reactor fueled with enriched uranium results from the fission of uranium as well as from the fission of plutonium produced as the reactor operates. As discussed previously, the plutonium forms from the combination of neutrons and the uranium in the fuel. In any nuclear reactor, only about 0.1% of the mass of the fuel is converted into energy. The other 99.9% remains in the fuel rods as fission products and unused fuel. All of the fission products absorb neutrons, and after a period of several months to a few years, depending on the reactor, the fission products must be removed by changing the fuel rods. Otherwise, the concentration of these fission products would increase and absorb more neutrons until the reactor could no longer operate.

Spent fuel rods contain a variety of products, consisting of unstable nuclei ranging in atomic number from 25 to 60, some transuranium elements, including plutonium and americium, and unreacted uranium isotopes. The unstable nuclei and the transuranium isotopes give the spent fuel a dangerously high level of radioactivity. The long-lived isotopes require thousands of years to decay to a safe level. The ultimate fate of the nuclear reactor as a significant source of energy in the United States probably rests on whether or not a politically and scientifically satisfactory technique for processing and storing the components of spent fuel rods can be developed.

Nuclear Fusion

The process of converting very light nuclei into heavier nuclei is also accompanied by the conversion of mass into large amounts of energy, a process called fusion. The principal source of energy in the sun is a net fusion reaction in which four hydrogen nuclei fuse and produce one helium nucleus and two positrons. This is a net reaction of a more complicated series of events:

\[\ce{4^1_1H ⟶ ^4_2He + 2^0_{+1}}\]

A helium nucleus has a mass that is 0.7% less than that of four hydrogen nuclei; this lost mass is converted into energy during the fusion. This reaction produces about 3.6 × 10¹¹ kJ of energy per mole of \(\ce{^4_2He}\) produced. This is somewhat larger than the energy produced by the nuclear fission of one mole of U-235 (1.8 × 10¹⁰ kJ), and over 3 million times larger than the energy produced by the (chemical) combustion of one mole of octane (5471 kJ).

It has been determined that the nuclei of the heavy isotopes of hydrogen, a deuteron, \(^2_1\) and a triton, \(^3_1\), undergo fusion at extremely high temperatures (thermonuclear fusion). They form a helium nucleus and a neutron:

\[\ce{^2_1H + ^3_1H ⟶ ^4_2He + 2^1_0n}\]

This change proceeds with a mass loss of 0.0188 amu, corresponding to the release of 1.69 × 10⁹ kilojoules per mole of \(\ce{^4_2He}\) formed. The very high temperature is necessary to give the nuclei enough kinetic energy to overcome the very strong repulsive forces resulting from the positive charges on their nuclei so they can collide.

Useful fusion reactions require very high temperatures for their initiation—about 15,000,000 K or more. At these temperatures, all molecules dissociate into atoms, and the atoms ionize, forming plasma. These conditions occur in an extremely large number of locations throughout the universe—stars are powered by fusion. Humans have already figured out how to create temperatures high enough to achieve fusion on a large scale in thermonuclear weapons. A thermonuclear weapon such as a hydrogen bomb contains a nuclear fission bomb that, when exploded, gives off enough energy to produce the extremely high temperatures necessary for fusion to occur.

Contributors and Attributions

• Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).