10.1: Overview of Spectroscopy

The focus of this chapter is on the interaction of ultraviolet, visible, and infrared radiation with matter. Because these techniques use optical materials to disperse and focus the radiation, they often are identified as optical spectroscopies. For convenience we will use the simpler term spectroscopy in place of optical spectroscopy; however, you should understand that we are considering only a limited part of a much broader area of analytical techniques.

Despite the difference in instrumentation, all spectroscopic techniques share several common features. Before we consider individual examples in greater detail, let’s take a moment to consider some of these similarities. As you work through the chapter, this overview will help you focus on similarities between different spectroscopic methods of analysis. You will find it easier to understand a new analytical method when you can see its relationship to other similar methods.

\(\PageIndex{1}\) What is Electromagnetic Radiation

Electromagnetic radiation—light—is a form of energy whose behavior is described by the properties of both waves and particles. Some properties of electromagnetic radiation, such as its refraction when it passes from one medium to another (Figure \(\PageIndex{1}\)), are explained best by describing light as a wave. Other properties, such as absorption and emission, are better described by treating light as a particle. The exact nature of electromagnetic radiation remains unclear, as it has since the development of quantum mechanics in the first quarter of the 20^th century.¹ Nevertheless, the dual models of wave and particle behavior provide a useful description for electromagnetic radiation.

Figure \(\PageIndex{1}\): The Golden Gate bridge as seen through rain drops. Refraction of light by the rain drops produces the distorted images. Source: Mila Zinkova (commons.wikipedia.org).

Wave Properties of Electromagnetic Radiation

Electromagnetic radiation consists of oscillating electric and magnetic fields that propagate through space along a linear path and with a constant velocity. In a vacuum electromagnetic radiation travels at the speed of light, c, which is 2.997 92 × 10⁸ m/s. When electromagnetic radiation moves through a medium other than a vacuum its velocity, v, is less than the speed of light in a vacuum. The difference between v and c is sufficiently small (<0.1%) that the speed of light to three significant figures, 3.00 × 10⁸ m/s, is accurate enough for most purposes.

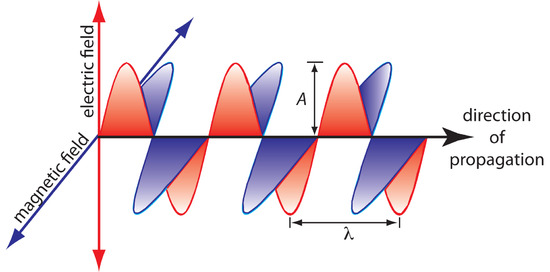
The oscillations in the electric and magnetic fields are perpendicular to each other, and to the direction of the wave’s propagation. Figure \(\PageIndex{2}\) shows an example of plane-polarized electromagnetic radiation, consisting of a single oscillating electric field and a single oscillating magnetic field.

Figure \(\PageIndex{2}\): Plane-polarized electromagnetic radiation showing the oscillating electric field in red and the oscillating magnetic field in blue. The radiation’s amplitude, A, and its wavelength, λ, are shown. Normally, electromagnetic radiation is unpolarized, with oscillating electric and magnetic fields present in all possible planes perpendicular to the direction of propagation.

An electromagnetic wave is characterized by several fundamental properties, including its velocity, amplitude, frequency, phase angle, polarization, and direction of propagation.² For example, the amplitude of the oscillating electric field at any point along the propagating wave is

\[A_\ce{t} = A_\ce{e}\sin(2πνt + Φ)\]

where A_t is the magnitude of the electric field at time t, A_e is the electric field’s maximum amplitude, ν is the wave’s frequency—the number of oscillations in the electric field per unit time—and Φ is a phase angle, which accounts for the fact that A_t need not have a value of zero at t = 0. The identical equation for the magnetic field is

\[A_\ce{t} =A_\ce{m}\sin(2πνt + Φ)\]

where A_m is the magnetic field’s maximum amplitude.

Other properties also are useful for characterizing the wave behavior of electromagnetic radiation. The wavelength, λ, is defined as the distance between successive maxima (see Figure \(\PageIndex{2}\)). For ultraviolet and visible electromagnetic radiation the wavelength is usually expressed in nanometers (1 nm = 10^–9 m), and for infrared radiation it is given in microns (1 μm = 10^–6 m). The relationship between wavelength and frequency is

\[λ = \dfrac{c}{ν}\]

Note

When electromagnetic radiation moves between different media—for example, when it moves from air into water—its frequency, ν, remains constant. Because its velocity depends upon the medium in which it is traveling, the electromagnetic radiation’s wavelength, λ, changes. If we replace the speed of light in a vacuum, c, with its speed in the medium, v, then the wavelength is

\[λ = \dfrac{v}{ν}\]

This change in wavelength as light passes between two media explains the refraction of electromagnetic radiation shown in Figure \(\PageIndex{1}\).

Another unit useful unit is the wavenumber, ν, which is the reciprocal of wavelength

\[\overline{ν} = \dfrac{1}{λ}\]

Wavenumbers are frequently used to characterize infrared radiation, with the units given in cm^–1.

Example \(\PageIndex{1}\)

In 1817, Josef Fraunhofer studied the spectrum of solar radiation, observing a continuous spectrum with numerous dark lines. Fraunhofer labeled the most prominent of the dark lines with letters. In 1859, Gustav Kirchhoff showed that the D line in the sun’s spectrum was due to the absorption of solar radiation by sodium atoms. The wavelength of the sodium D line is 589 nm. What are the frequency and the wavenumber for this line?

Solution

The frequency and wavenumber of the sodium D line are

\[ν = \dfrac{c}{λ} = \mathrm{\dfrac{3.00×10^8\: m/s}{589×10^{−9}\: m} = 5.09×10^{14}\: s^{−1}}\]

\[\overline{ν} = \dfrac{1}{λ} = \mathrm{\dfrac{1}{589×10^{−9}\: m} × \dfrac{1\: m}{100\: cm} = 1.70×10^4\: cm^{−1}}\]

Exercise 10.1

Another historically important series of spectral lines is the Balmer series of emission lines form hydrogen. One of the lines has a wavelength of 656.3 nm. What are the frequency and the wavenumber for this line?

Click here to review your answer to this exercise.

Particle Properties of Electromagnetic Radiation

When matter absorbs electromagnetic radiation it undergoes a change in energy. The interaction between matter and electromagnetic radiation is easiest to understand if we assume that radiation consists of a beam of energetic particles called photons. When a photon is absorbed by a sample it is “destroyed,” and its energy acquired by the sample.³ The energy of a photon, in joules, is related to its frequency, wavelength, and wavenumber by the following equalities

\[E = hν = \dfrac{hc}{λ} =hc\overline{ν}\]

where h is Planck’s constant, which has a value of 6.626 × 10^–34 J⋅s.

Example \(\PageIndex{2}\)

What is the energy of a photon from the sodium D line at 589 nm?

Solution

The photon’s energy is

\[E = \dfrac{hc}{λ} = \mathrm{\dfrac{(6.626×10^{−34}\: J⋅s)(3.00×10^8\: m/s)}{589×10^{−9}\: m} = 3.37×10^{−19}\: J}\]

Exercise 10.2

What is the energy of a photon for the Balmer line at a wavelength of 656.3 nm?

Click here to review your answer to this exercise.

The Electromagnetic Spectrum

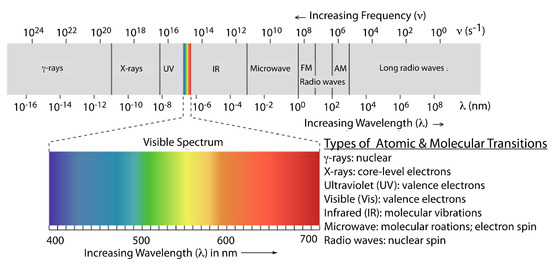
The frequency and wavelength of electromagnetic radiation vary over many orders of magnitude. For convenience, we divide electromagnetic radiation into different regions—the electromagnetic spectrum—based on the type of atomic or molecular transition that gives rise to the absorption or emission of photons (Figure \(\PageIndex{3}\)). The boundaries between the regions of the electromagnetic spectrum are not rigid, and overlap between spectral regions is possible.

Figure \(\PageIndex{3}\): The electromagnetic spectrum showing the boundaries between different regions and the type of atomic or molecular transition responsible for the change in energy. The colored inset shows the visible spectrum. Source: modified from Zedh (www.commons.wikipedia.org).

\(\PageIndex{2}\) Photons as a Signal Source

In the previous section we defined several characteristic properties of electromagnetic radiation, including its energy, velocity, amplitude, frequency, phase angle, polarization, and direction of propagation. A spectroscopic measurement is possible only if the photon’s interaction with the sample leads to a change in one or more of these characteristic properties. We can divide spectroscopy into two broad classes of techniques. In one class of techniques there is a transfer of energy between the photon and the sample. Table \(\PageIndex{1}\) provides a list of several representative examples.

Table \(\PageIndex{1}\): Examples of Spectroscopic Techniques Involving an Exchange of Energy Between a Photon and the Sample

Type of Energy Transfer	Region of Electromagnetic Spectrum	Spectroscopic Technique^a
absorption	γ-ray	Mossbauer spectroscopy
	X-ray	X-ray absorption spectroscopy
	UV/Vis	UV/Vis spectroscopy atomic absorption spectroscopy
	IR	infrared spectroscopy raman spectroscopy
	Microwave	microwave spectroscopy
	Radio wave	electron spin resonance spectroscopy nuclear magnetic resonance spectroscopy
emission (thermal excitation)	UV/Vis	atomic emission spectroscopy
photoluminescence	X-ray	X-ray fluorescence
	UV/Vis	fluorescence spectroscopy phosphorescence spectroscopy atomic fluorescence spectroscopy
chemiluminescence	UV/Vis	chemiluminescence spectroscopy

^a Techniques discussed in this text are shown in italics.

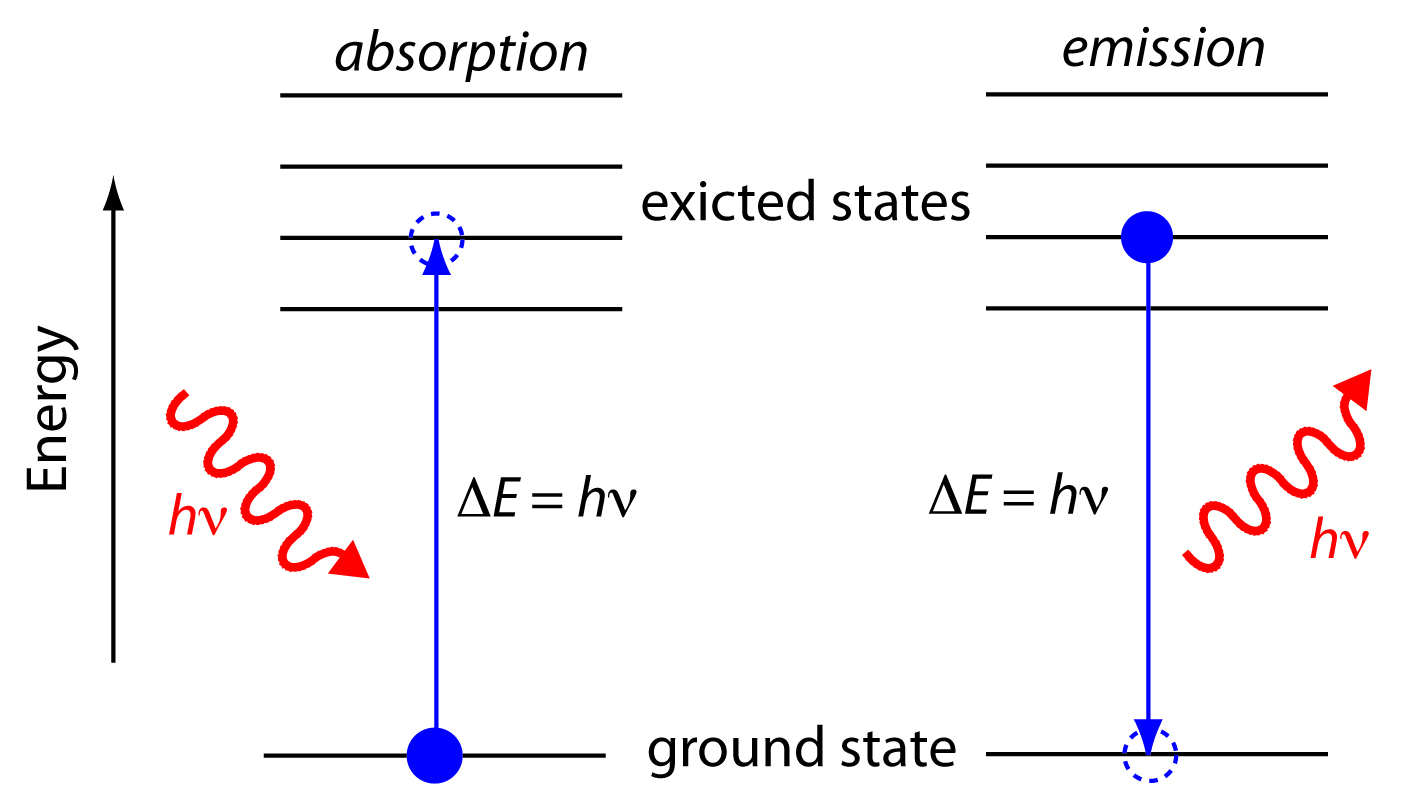
In absorption spectroscopy a photon is absorbed by an atom or molecule, which undergoes a transition from a lower-energy state to a higher-energy, or excited state (Figure \(\PageIndex{4}\)). The type of transition depends on the photon’s energy. The electromagnetic spectrum in Figure \(\PageIndex{3}\), for example, shows that absorbing a photon of visible light promotes one of the atom’s or molecule’s valence electrons to a higher-energy level. When an molecule absorbs infrared radiation, on the other hand, one of its chemical bonds experiences a change in vibrational energy.

Figure \(\PageIndex{4}\): Simplified energy diagram showing the absorption and emission of a photon by an atom or a molecule. When a photon of energy hν strikes the atom or molecule, absorption may occur if the difference in energy, ∆E, between the ground state and the excited state is equal to the photon’s energy. An atom or molecule in an excited state may emit a photon and return to the ground state. The photon’s energy, hν, equals the difference in energy, ∆E, between the two states.

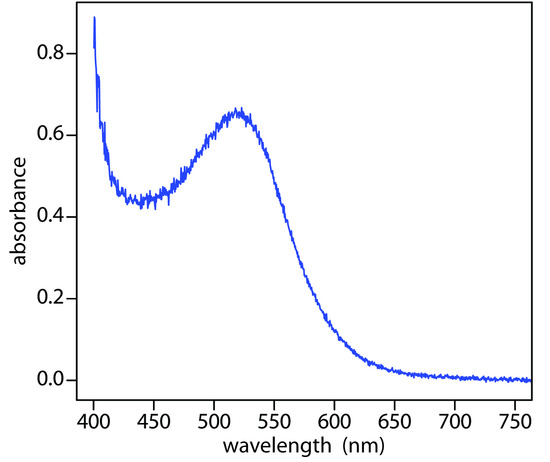
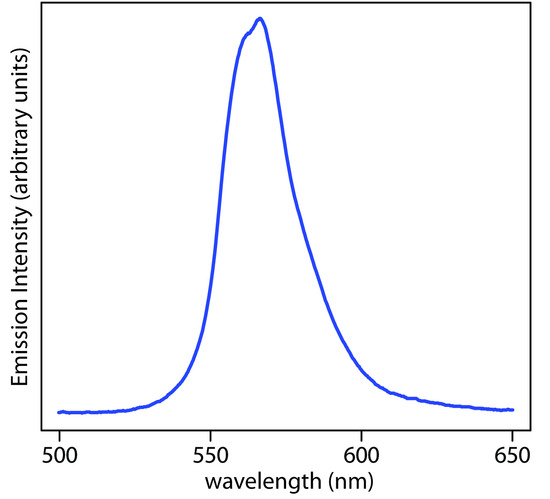
When it absorbs electromagnetic radiation the number of photons passing through a sample decreases. The measurement of this decrease in photons, which we call absorbance, is a useful analytical signal. Note that the each of the energy levels in Figure \(\PageIndex{4}\) has a well-defined value because they are quantized. Absorption occurs only when the photon’s energy, hν, matches the difference in energy, ∆E, between two energy levels. A plot of absorbance as a function of the photon’s energy is called an absorbance spectrum. Figure \(\PageIndex{5}\), for example, shows the absorbance spectrum of cranberry juice.

Figure \(\PageIndex{5}\): Visible absorbance spectrum for cranberry juice. The anthocyanin dyes in cranberry juice absorb visible light with blue, green, and yellow wavelengths (see Figure \(\PageIndex{3}\)). As a result, the juice appears red.

When an atom or molecule in an excited state returns to a lower energy state, the excess energy often is released as a photon, a process we call emission (Figure \(\PageIndex{4}\)). There are several ways in which an atom or molecule may end up in an excited state, including thermal energy, absorption of a photon, or by a chemical reaction. Emission following the absorption of a photon is also called photoluminescence, and that following a chemical reaction is called chemiluminescence. A typical emission spectrum is shown in Figure \(\PageIndex{6}\).

Note

Molecules also can release energy in the form of heat. We will return to this point later in the chapter.

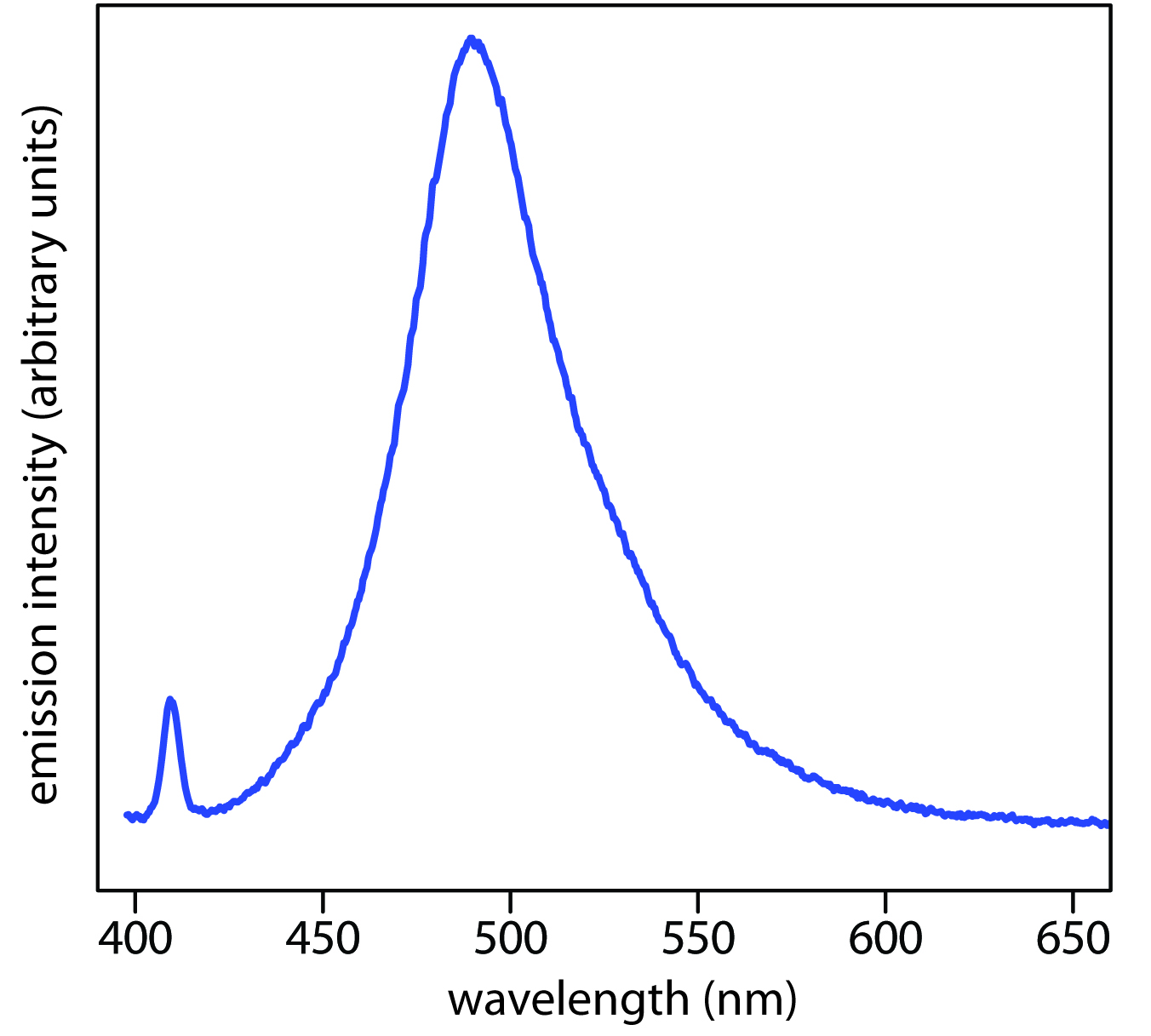
Figure \(\PageIndex{6}\): Photoluminescence spectrum of the dye coumarin 343, which is incorporated in a reverse micelle suspended in cyclohexanol. The dye’s absorbance spectrum (not shown) has a broad peak around 400 nm. The sharp peak at 409 nm is from the laser source used to excite coumarin 343. The broad band centered at approximately 500 nm is the dye’s emission band. Because the dye absorbs blue light, a solution of coumarin 343 appears yellow in the absence of photoluminescence. Its photoluminescent emission is blue-green. Source: data from Bridget Gourley, Department of Chemistry & Biochemistry, DePauw University).

In the second broad class of spectroscopic techniques, the electromagnetic radiation undergoes a change in amplitude, phase angle, polarization, or direction of propagation as a result of its refraction, reflection, scattering, diffraction, or dispersion by the sample. Several representative spectroscopic techniques are listed in Table \(\PageIndex{2}\).

Table \(\PageIndex{2}\): Examples of Spectroscopic Techniques That Do Not Involve an Exchange of Energy Between a Photon and the Sample

Region of Electromagnetic Spectrum	Type of Interaction	Spectroscopic Technique^a
X-ray	diffraction	X-ray diffraction
UV/Vis	refraction	refractometry
	scattering	nephelometry turbidimetry
	dispersion	optical rotary dispersion

^a Techniques discussed in this text are shown in italics.

\(\PageIndex{3}\) Basic Components of Spectroscopic Instruments

The spectroscopic techniques in Table \(\PageIndex{1}\) and Table \(\PageIndex{2}\) use instruments that share several common basic components, including a source of energy, a means for isolating a narrow range of wavelengths, a detector for measuring the signal, and a signal processor that displays the signal in a form convenient for the analyst. In this section we introduce these basic components. Specific instrument designs are considered in later sections.

Note

You will find a more detailed treatment of these components in the additional resources for this chapter.

Sources of Energy

All forms of spectroscopy require a source of energy. In absorption and scattering spectroscopy this energy is supplied by photons. Emission and photoluminescence spectroscopy use thermal, radiant (photon), or chemical energy to promote the analyte to a suitable excited state.

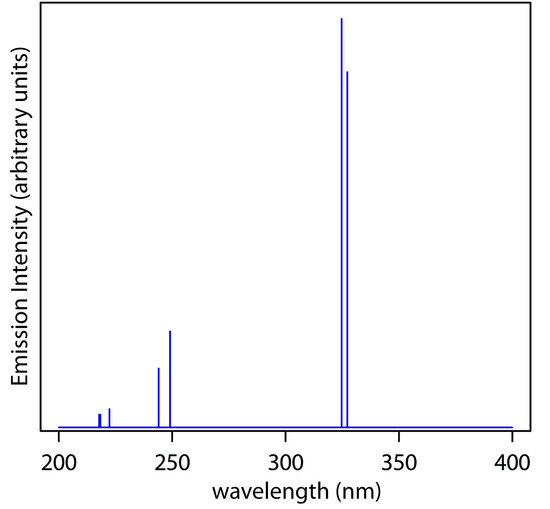
Sources of Electromagnetic Radiation. A source of electromagnetic radiation must provide an output that is both intense and stable. Sources of electromagnetic radiation are classified as either continuum or line sources. A continuum source emits radiation over a broad range of wavelengths, with a relatively smooth variation in intensity (Figure \(\PageIndex{7}\)). A line source, on the other hand, emits radiation at selected wavelengths (Figure \(\PageIndex{8}\)). Table \(\PageIndex{3}\) provides a list of the most common sources of electromagnetic radiation.

Figure \(\PageIndex{7}\): Spectrum showing the emission from a green LED, which provides continuous emission over a wavelength range of approximately 530–640 nm.

Figure \(\PageIndex{8}\) Emission spectrum from a Cu hollow cathode lamp. This spectrum consists of seven distinct emission lines (the first two differ by only 0.4 nm and are not resolved in this spectrum). Each emission line has a width of approximately 0.01 nm at ½ of its maximum intensity.

Table \(\PageIndex{3}\): Common Sources of Electromagnetic Radiation

Source	Wavelength Region	Useful for...
H2 and D2 lamp	continuum source from 160–380 nm	molecular absorption
tungsten lamp	continuum source from 320–2400 nm	molecular absorption
Xe arc lamp	continuum source from 200–1000 nm	molecular fluorescence
nernst glower	continuum source from 0.4–20 μm	molecular absorption
globar	continuum source from 1–40 μm	molecular absorption
nichrome wire	continuum source from 0.75–20 μm	molecular absorption
hollow cathode lamp	line source in UV/Visible	atomic absorption
Hg vapor lamp	line source in UV/Visible	molecular fluorescence
laser	line source in UV/Visible/IR	atomic and molecular absorption, fluorescence, and scattering

Sources of Thermal Energy. The most common sources of thermal energy are flames and plasmas. Flames sources use the combustion of a fuel and an oxidant to achieve temperatures of 2000–3400 K. Plasmas, which are hot, ionized gases, provide temperatures of 6000–10 000 K.

Chemical Sources of Energy. Exothermic reactions also may serve as a source of energy. In chemiluminescence the analyte is raised to a higher-energy state by means of a chemical reaction, emitting characteristic radiation when it returns to a lower-energy state. When the chemical reaction results from a biological or enzymatic reaction, the emission of radiation is called bioluminescence. Commercially available “light sticks” and the flash of light from a firefly are examples of chemiluminescence and bioluminescence.

Wavelength Selection

In Nessler’s original colorimetric method for ammonia, described at the beginning of the chapter, the sample and several standard solutions of ammonia are placed in separate tall, flat-bottomed tubes. As shown in Figure \(\PageIndex{9}\), after adding the reagents and allowing the color to develop, the analyst evaluates the color by passing natural, ambient light through the bottom of the tubes and looking down through the solutions. By matching the sample’s color to that of a standard, the analyst is able to determine the concentration of ammonia in the sample.

Figure \(\PageIndex{9}\) Nessler’s original method for comparing the color of two solutions. Natural light passes upwards through the samples and standards and the analyst views the solutions by looking down toward the light source. The top view, shown on the right, is what the analyst sees. To determine the analyte’s concentration, the analyst exchanges standards until the two colors match.

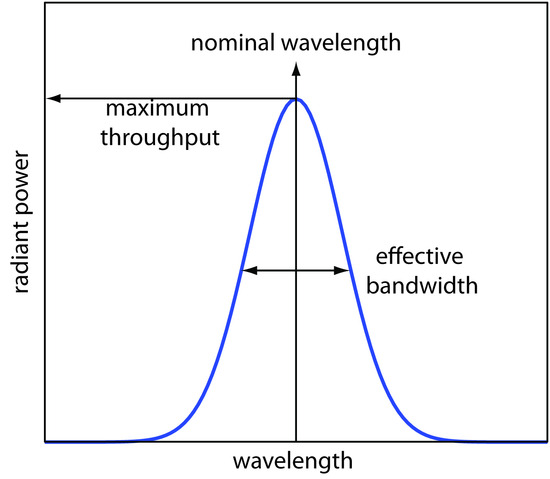
In Figure \(\PageIndex{9}\) every wavelength of light from the source passes through the sample. If there is only one absorbing species, this is not a problem. If two components in the sample absorbs different wavelengths of light, then a quantitative analysis using Nessler’s original method becomes impossible. Ideally we want to select a wavelength that only the analyte absorbs. Unfortunately, we can not isolate a single wavelength of radiation from a continuum source. As shown in Figure \(\PageIndex{1}\)0, a wavelength selector passes a narrow band of radiation characterized by a nominal wavelength, an effective bandwidth and a maximum throughput of radiation. The effective bandwidth is defined as the width of the radiation at half of its maximum throughput.

Figure \(\PageIndex{1}\)0 Radiation exiting a wavelength selector showing the band’s nominal wavelength and its effective bandwidth.

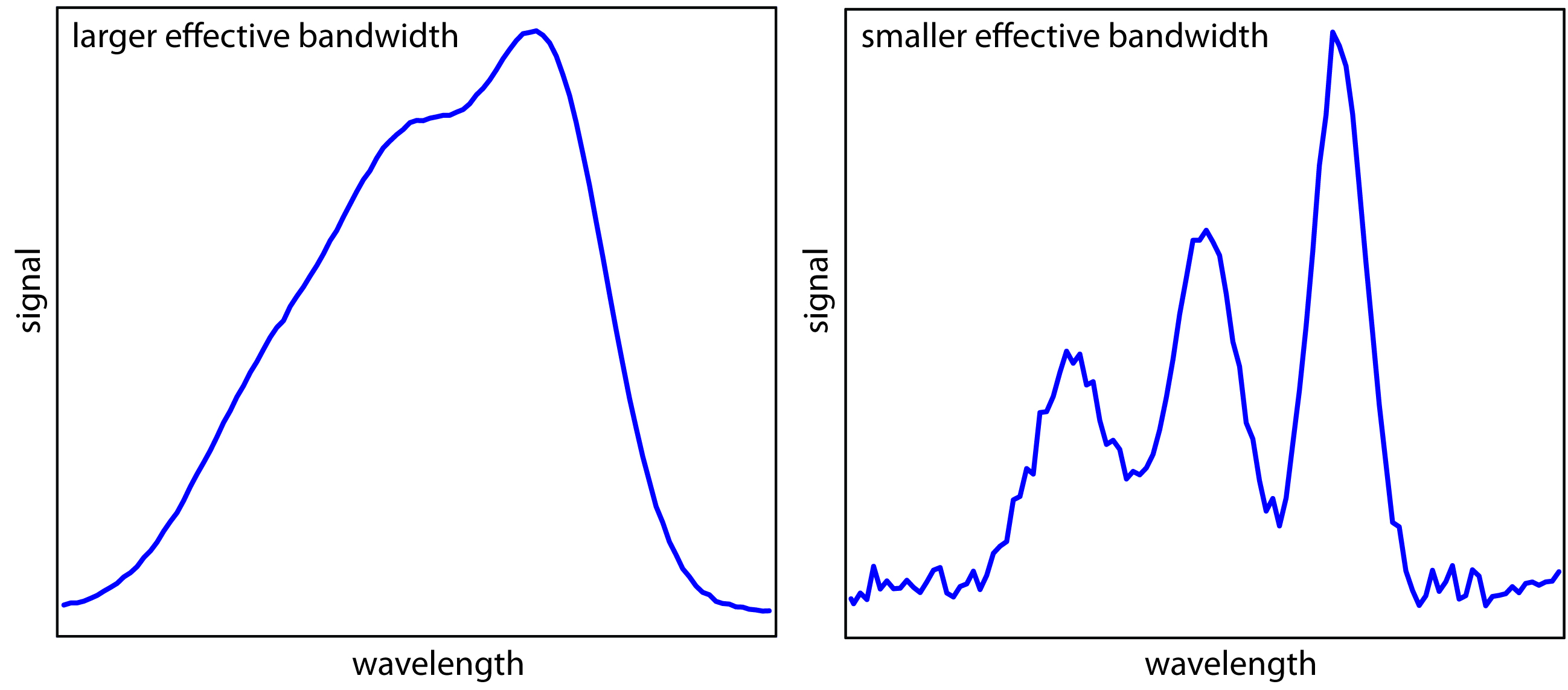
The ideal wavelength selector has a high throughput of radiation and a narrow effective bandwidth. A high throughput is desirable because more photons pass through the wavelength selector, giving a stronger signal with less background noise. A narrow effective bandwidth provides a higher resolution, with spectral features separated by more than twice the effective bandwidth being resolved. As shown in Figure \(\PageIndex{1}\)1, these two features of a wavelength selector generally are in opposition. Conditions favoring a higher throughput of radiation usually provide less resolution. Decreasing the effective bandwidth improves resolution, but at the cost of a noisier signal.⁴ For a qualitative analysis, resolution is usually more important than noise, and a smaller effective bandwidth is desirable. In a quantitative analysis less noise is usually desirable.

Figure \(\PageIndex{1}\)1: Example showing the effect of the wavelength selector’s effective bandwidth on resolution and noise. The spectrum with the smaller effective bandwidth has a better resolution, allowing us to see the presence of three peaks, but at the expense of a noisier signal. The spectrum with the larger effective bandwidth has less noise, but at the expense of less resolution between the three peaks.

Wavelength Selection Using Filters. The simplest method for isolating a narrow band of radiation is to use an absorption or interference filter. Absorption filters work by selectively absorbing radiation from a narrow region of the electromagnetic spectrum. Interference filters use constructive and destructive interference to isolate a narrow range of wavelengths. A simple example of an absorption filter is a piece of colored glass. A purple filter, for example, removes the complementary color green from 500–560 nm. Commercially available absorption filters provide effective bandwidths of 30–250 nm, although the throughput may be only 10% of the source’s emission intensity at the low end of this range. Interference filters are more expensive than absorption filters, but have narrower effective bandwidths, typically 10–20 nm, with maximum throughputs of at least 40%.

Wavelength Selection Using Monochromators. A filter has one significant limitation—because a filter has a fixed nominal wavelength, if you need to make measurements at two different wavelengths, then you need to use two different filters. A monochromator is an alternative method for selecting a narrow band of radiation that also allows us to continuously adjust the band’s nominal wavelength.

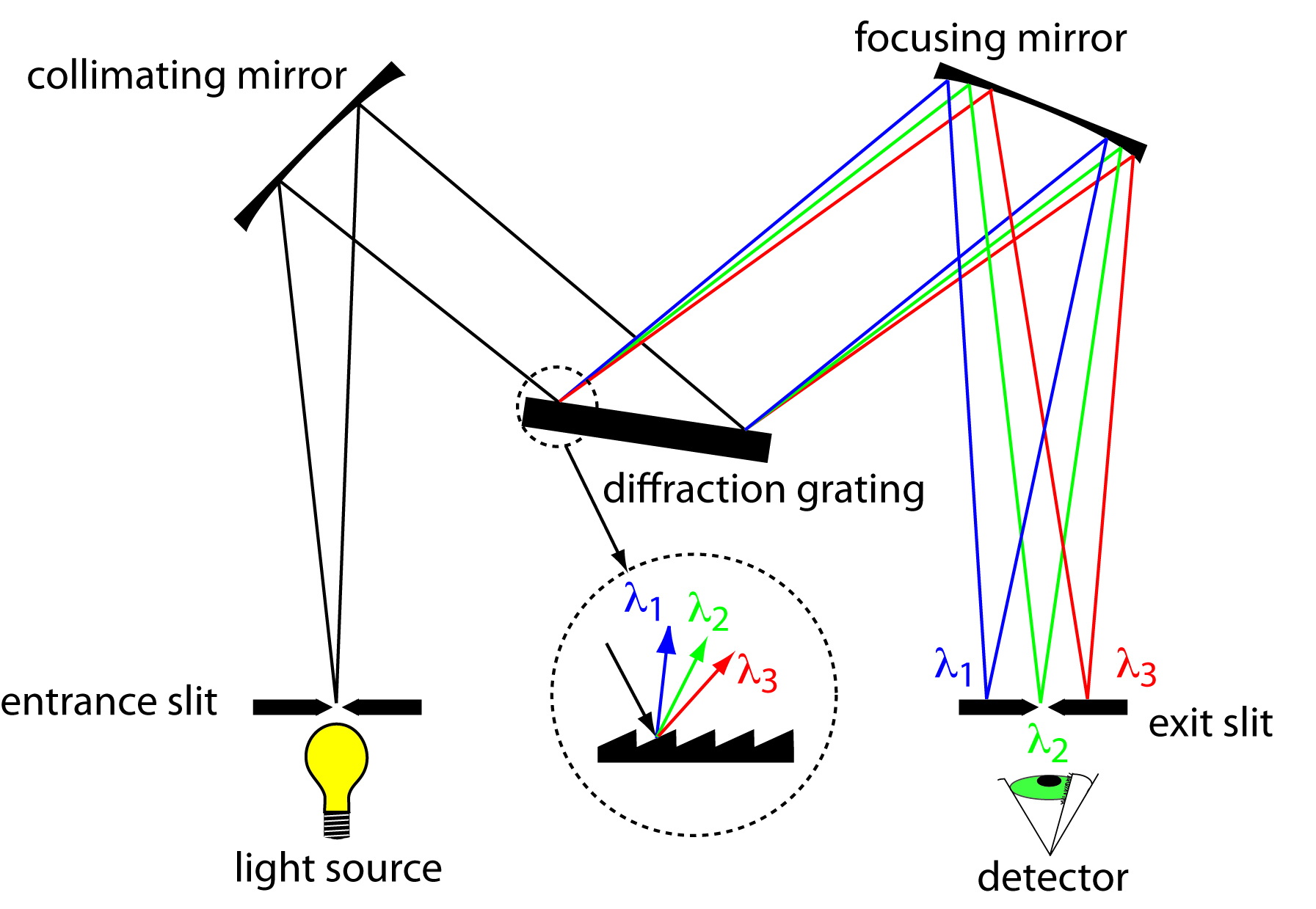
The construction of a typical monochromator is shown in Figure \(\PageIndex{1}\)2. Radiation from the source enters the monochromator through an entrance slit. The radiation is collected by a collimating mirror, which reflects a parallel beam of radiation to a diffraction grating. The diffraction grating is an optically reflecting surface with a large number of parallel grooves (see insert to Figure \(\PageIndex{1}\)2). The diffraction grating disperses the radiation and a second mirror focuses the radiation onto a planar surface containing an exit slit. In some monochromators a prism is used in place of the diffraction grating.

Figure \(\PageIndex{1}\)2 Schematic diagram of a monochromator that uses a diffraction grating to disperse the radiation.

Radiation exits the monochromator and passes to the detector. As shown in Figure \(\PageIndex{1}\)2, a monochromator converts a polychromatic source of radiation at the entrance slit to a monochromatic source of finite effective bandwidth at the exit slit. The choice of which wavelength exits the monochromator is determined by rotating the diffraction grating. A narrower exit slit provides a smaller effective bandwidth and better resolution, but allows a smaller throughput of radiation.

Note

• Polychromatic means many colored. Polychromatic radiation contains many different wavelengths of light.
• Monochromatic means one color, or one wavelength. Although the light exiting a monochromator is not strictly of a single wavelength, its narrow effective bandwidth allows us to think of it as monochromatic.

Monochromators are classified as either fixed-wavelength or scanning. In a fixed-wavelength monochromator we select the wavelength by manually rotating the grating. Normally a fixed-wavelength monochromator is used for a quantitative analysis where measurements are made at one or two wavelengths. A scanning monochromator includes a drive mechanism that continuously rotates the grating, allowing successive wavelengths to exit from the monochromator. Scanning monochromators are used to acquire spectra, and, when operated in a fixed-wavelength mode, for a quantitative analysis.

Interferometers. An interferometer provides an alternative approach for wavelength selection. Instead of filtering or dispersing the electromagnetic radiation, an interferometer allows source radiation of all wavelengths to reach the detector simultaneously (Figure \(\PageIndex{1}\)3). Radiation from the source is focused on a beam splitter that reflects half of the radiation to a fixed mirror and transmits the other half to a movable mirror. The radiation recombines at the beam splitter, where constructive and destructive interference determines, for each wavelength, the intensity of light reaching the detector. As the moving mirror changes position, the wavelengths of light experiencing maximum constructive interference and maximum destructive interference also changes. The signal at the detector shows intensity as a function of the moving mirror’s position, expressed in units of distance or time. The result is called an interferogram, or a time domain spectrum. The time domain spectrum is converted mathematically, by a process called a Fourier transform, to a spectrum (also called a frequency domain spectrum) showing intensity as a function of the radiation’s energy.

Note

The mathematical details of the Fourier transform are beyond the level of this textbook. You can consult the chapter’s additional resources for additional information.

Figure \(\PageIndex{1}\)3 Schematic diagram of an interferometers.

In comparison to a monochromator, an interferometer has two significant advantages. The first advantage, which is termed Jacquinot’s advantage, is the higher throughput of source radiation. Because an interferometer does not use slits and has fewer optical components from which radiation can be scattered and lost, the throughput of radiation reaching the detector is 80–200× greater than that for a monochromator. The result is less noise. The second advantage, which is called Fellgett’s advantage, is a savings in the time needed to obtain a spectrum. Because the detector monitors all frequencies simultaneously, an entire spectrum takes approximately one second to record, as compared to 10–15 minutes with a scanning monochromator.

Detectors

In Nessler’s original method for determining ammonia (Figure \(\PageIndex{9}\)) the analyst’s eye serves as the detector, matching the sample’s color to that of a standard. The human eye, of course, has a poor range—responding only to visible light—nor is it particularly sensitive or accurate. Modern detectors use a sensitive transducer to convert a signal consisting of photons into an easily measured electrical signal. Ideally the detector’s signal, S, is a linear function of the electromagnetic radiation’s power, P,

\[S = kP + D\]

where k is the detector’s sensitivity, and D is the detector’s dark current, or the background current when we prevent the source’s radiation from reaching the detector.

Note

Transducer is a general term that refers to any device that converts a chemical or physical property into an easily measured electrical signal. The retina in your eye, for example, is a transducer that converts photons into an electrical nerve impulse.

There are two broad classes of spectroscopic transducers: thermal transducers and photon transducers. Table \(\PageIndex{4}\) provides several representative examples of each class of transducers.

Table \(\PageIndex{4}\): Examples of Transducers for Spectroscopy

Transducer	Class	Wavelength Range	Output Signal
phototube	photon	200–1000 nm	current
photomultiplier	photon	110–1000 nm	current
Si photodiode	photon	250–1100 nm	current
photoconductor	photon	750–6000 nm	change in resistance
photovoltaic cell	photon	400–5000 nm	current or voltage
thermocouple	thermal	0.8–40 μm	voltage
thermistor	thermal	0.8–40 μm	change in resistance
pneumatic	thermal	0.8–1000 μm	membrane displacement
pyroelectric	thermal	0.3–1000 μm	current

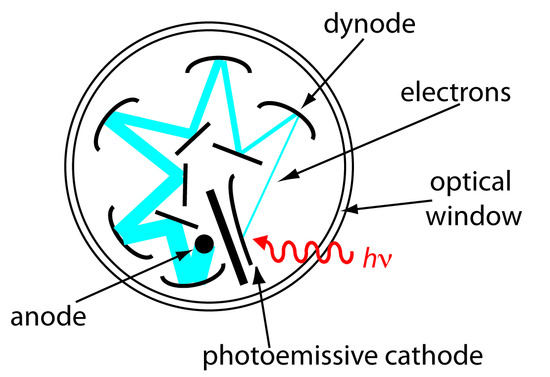
Photon Transducers. Phototubes and photomultipliers contain a photosensitive surface that absorbs radiation in the ultraviolet, visible, or near IR, producing an electrical current proportional to the number of photons reaching the transducer (Figure \(\PageIndex{1}\)4). Other photon detectors use a semiconductor as the photosensitive surface. When the semiconductor absorbs photons, valence electrons move to the semiconductor’s conduction band, producing a measurable current. One advantage of the Si photodiode is that it is easy to miniaturize. Groups of photodiodes may be gathered together in a linear array containing from 64–4096 individual photodiodes. With a width of 25 μm per diode, for example, a linear array of 2048 photodiodes requires only 51.2 mm of linear space. By placing a photodiode array along the monochromator’s focal plane, it is possible to monitor simultaneously an entire range of wavelengths.

Figure \(\PageIndex{1}\)4: Schematic of a photomultiplier. A photon strikes the photoemissive cathode producing electrons, which accelerate toward a positively charged dynode. Collision of these electrons with the dynode generates additional electrons, which accelerate toward the next dynode. A total of 10⁶–10⁷ electrons per photon eventually reach the anode, generating an electrical current.

Thermal Transducers. Infrared photons do not have enough energy to produce a measurable current with a photon transducer. A thermal transducer, therefore, is used for infrared spectroscopy. The absorption of infrared photons by a thermal transducer increases its temperature, changing one or more of its characteristic properties. A pneumatic transducer, for example, is a small tube of xenon gas with an IR transparent window at one end and a flexible membrane at the other end. Photons enter the tube and are absorbed by a blackened surface, increasing the temperature of the gas. As the temperature inside the tube fluctuates, the gas expands and contracts and the flexible membrane moves in and out. Monitoring the membrane’s displacement produces an electrical signal.

Signal Processors

A transducer’s electrical signal is sent to a signal processor where it is displayed in a form that is more convenient for the analyst. Examples of signal processors include analog or digital meters, recorders, and computers equipped with digital acquisition boards. A signal processor also is used to calibrate the detector’s response, to amplify the transducer’s signal, to remove noise by filtering, or to mathematically transform the signal.

Note

If the retina in your eye is a transducer, then your brain is a signal processor.

Contributors

David Harvey (DePauw University)