8.E: Gravimetric Methods (Exercises)

1. Starting with the equilibrium constant expressions for reaction 8.1, and reactions 8.3–8.5, verify that equation 8.7 is correct.

2. Equation 8.7 explains how the solubility of AgCl varies as a function of the equilibrium concentration of Cl^–. Derive a similar equation that describes the solubility of AgCl as a function of the equilibrium concentration of Ag⁺. Graph the resulting solubility function and compare it to that shown in Figure 8.1.

3. Construct a solubility diagram for Zn(OH)₂ that takes into account the following soluble zinc-hydroxide complexes: Zn(OH)⁺, Zn(OH)₃^–, and Zn(OH)₄^2–. What is the optimum pH for quantitatively precipitating Zn(OH)₂? For your solubility diagram, plot log(S) on the y-axis and pH on the x-axis. See the appendices for relevant equilibrium constants.

4. Starting with equation 8.10, verify that equation 8.11 is correct.

5. For each of the following precipitates, use a ladder diagram to identify the pH range where the precipitates has its lowest solubility? See the appendices for relevant equilibrium constants.

a. CaC₂O₄ b. PbCrO₄ c. BaSO₄ d. SrCO₃ e. ZnS

6. Mixing solutions of 1.5 M KNO₃ and 1.5 M HClO₄ produces a white precipitate of KClO₄. If permanganate ions are present, an inclusion of KMnO₄ is possible. Impure precipitates of KClO₄ are purple if an inclusion of KMnO₄ is present. Shown below are descriptions of two experiments in which KClO₄ is precipitated in the presence of MnO₄^–. Explain why the experiments lead to the different results shown in Figure 8.15.

Experiment 1. Place 1 mL of 1.5 M KNO₃ in a test tube, add 3 drops of 0.1 M KMnO₄, and swirl to mix. Add 1 mL of 1.5 M HClO₄ dropwise, agitating the solution between drops. Destroy the excess KMnO₄ by adding 0.1 M NaHSO₃ dropwise. The resulting precipitate of KClO₄ has an intense purple color.

Experiment 2. Place 1 mL of 1.5 M HClO₄ in a test tube, add 3 drops of 0.1 M KMnO₄, and swirl to mix. Add 1 mL of 1.5 M KNO₃ dropwise, agitating the solution between drops. Destroy the excess KMnO₄ by adding 0.1 M NaHSO₃ dropwise. The resulting precipitate of KClO₄ has a pale purple in color.

Figure 8.15 Results for the experiments in Problem 8.6. (a) Experiment 1; (b) Experiment 2.

7. Mixing solutions of Ba(SCN)₂ and MgSO₄ produces a precipitate of BaSO₄. Shown below are the descriptions and results for three experiments using different concentrations of Ba(SCN)₂ and MgSO₄. Explain why these experiments produce different results.

Experiment 1. When equal volumes of 3.5 M Ba(SCN)₂ and 3.5 M MgSO₄ are mixed, a gelatinous precipitate immediately forms.

Experiment 2. When equal volumes of 1.5 M Ba(SCN)₂ and 1.5 M MgSO₄ are mixed, a curdy precipitate immediately forms. Individual particles of BaSO₄ can be seen as points under a magnification of 1500×(a particle size less than 0.2 μm).

Experiment 3. When equal volumes of 0.5 mM Ba(SCN)₂ and 0.5 mM MgSO₄ are mixed, the complete precipitation of BaSO₄ requires 2–3 h. Individual crystals of BaSO₄ obtain lengths of approximately 5 μm.

8. Aluminum is determined gravimetrically by precipitating Al(OH)₃ and isolating Al₂O₃. A sample containing approximately 0.1 grams of Al is dissolved in 200 mL of H₂O, and 5 grams of NH₄Cl and a few drops of methyl red indicator are added (methyl red is red at pH levels below 4 and yellow at pH levels above 6). The solution is heated to boiling and 1:1 NH₃ is added dropwise till the indicator turns yellow, precipitating Al(OH)₃. The precipitate is held at the solution’s boiling point for several minutes before filtering and rinsing with a hot solution of 2% w/v NH₄NO₃. The precipitate is then ignited at 1000–1100^oC, forming Al₂O₃.

(a) Cite two ways in which this procedure encourages the formation of larger particles of precipitate.

(b) The ignition step must be carried out carefully to ensure the quantitative conversion of Al(OH)₃ to Al₂O₃. What is the effect of an incomplete conversion on the %w/w Al?

(c) What is the purpose of adding NH₄Cl and methyl red indicator?

(d) An alternative procedure involves isolating and weighing the precipitate as the 8-hydroxyquinolate, Al(C₉H₆ON)₃. Why might this be a more advantageous form of Al for a gravimetric analysis? Are there any disadvantages?

9. Calcium is determined gravimetrically by precipitating CaC₂O₄•H₂O and isolating CaCO₃. After dissolving a sample in 10 mL of water and 15 mL of 6 M HCl, the resulting solution is heated to boiling and a warm solution of excess ammonium oxalate is added. The solution is maintained at 80^oC and 6 M NH₃ is added dropwise, with stirring, until the solution is faintly alkaline. The resulting precipitate and solution are removed from the heat and allowed to stand for at least one hour. After testing the solution for completeness of precipitation, the sample is filtered, rinsed with 0.1% w/v ammonium oxalate, and dried at 100–120^oC for 1 hour. The precipitate is transferred to a muffle furnace where it is converted to CaCO₃ by drying at 500 ± 25^oC until constant weight.

(a) Why is the precipitate of CaC₂O₄•H₂O converted to CaCO₃?

(b) In the final step, if the sample is heated at too high of a temperature some CaCO₃ may be converted to CaO. What effect would this have on the reported %w/w Ca?

(c) Why is the precipitant, (NH₄)₂C₂O₄, added to a hot, acidic solution instead of a cold, alkaline solution?

10. Iron is determined gravimetrically by precipitating as Fe(OH)₃ and igniting to Fe₂O₃. After dissolving a sample in 50 mL of H₂O and 10 mL of 6 M HCl, any Fe²⁺ is converted Fe³⁺ by oxidizing with 1–2 mL of concentrated HNO₃. The sample is heated to remove the oxides of nitrogen and the solution is diluted to 200 mL. After bringing the solution to a boil, Fe(OH)₃ is precipitated by slowly adding 1:1 NH₃ until the odor of NH₃ is detected. The solution is boiled for an additional minute and the precipitate is allowed to settler. The precipitate is then filtered and rinsed with several portions of hot 1% w/v NH₄NO₃ until no Cl^– is found in the wash water. Finally, the precipitate is ignited to constant weight at 500–550^oC and weighed as Fe₂O₃.

(a) If ignition is not carried out under oxidizing conditions (plenty of O₂ present), the final product may contain Fe₃O₄. What effect would this have on the reported %w/w Fe?

(b) The precipitate is washed with a dilute solution of NH₄NO₃. Why is NH₄NO₃ added to the wash water?

(c) Why does the procedure call for adding NH₃ until the odor of ammonia is detected?

(d) Describe how you might test the filtrate for Cl^–.

11. Sinha and Shome described a gravimetric method for molybdenum in which it is precipitated as MoO₂(C₁₃H₁₀NO₂)₂ using n-benzoylphenylhydroxylamine, C₁₃H₁₁NO₂, as a precipitant.¹² The precipitate is weighed after igniting to MoO₃. As part of their study, the authors determined the optimum conditions for the analysis. Samples containing 0.0770 g of Mo were taken through the procedure while varying the temperature, the amount of precipitant added, and the pH of the solution. The solution volume was held constant at 300 mL for all experiments. A summary of their results are shown in the following table.

Temperature (^oC)	Mass(g) of precipitant	Volume(mL) of 10 M HCl	Mass (g) of MoO3
30	0.20	0.9	0.0675
30	0.30	0.9	0.1014
30	0.35	0.9	0.1140
30	0.42	0.9	0.1155
30	0.42	0.3	0.1150
30	0.42	18.0	0.1152
30	0.42	48.0	0.1160
30	0.42	75.0	0.1159
50	0.42	0.9	0.1156
75	0.42	0.9	0.1158
80	0.42	0.9	0.1129

Considering these results, discuss the optimum conditions for determining Mo by this method. Express your results for the precipitant as the minimum %w/v in excess, needed to ensure a quantitative precipitation.

12. A sample of an impure iron ore is approximately 55% w/w Fe. The amount of Fe in the sample is to be determined gravimetrically by isolating it as Fe₂O₃. What mass of sample do you need to ensure that you isolate at least 1 g of Fe₂O₃?

13. The concentration of arsenic in an insecticide is determined gravimetrically by precipitating MgNH₄AsO₄ and isolating Mg₂As₂O₇. Determine the %w/w As₂O₃ in a 1.627-g sample of insecticide if it yields 106.5 mg of Mg₂As₂O₇.

14. After preparing a sample of alum, K₂SO₄•Al₂(SO₄)₃•24H₂O, a student determined its purity by dissolving a 1.2391-g sample and precipitating the aluminum as Al(OH)₃. After filtering, rinsing, and igniting, 0.1357 g of Al₂O₃ is obtained. What is the purity of the alum preparation?

15. To determine the amount of iron in a dietary supplement, a random sample of 15 tablets weighing a total of 20.505 g was ground into a fine powder. A 3.116-g sample was dissolved and treated to precipitate the iron as Fe(OH)₃. The precipitate was collected, rinsed, and ignited to a constant weight as Fe₂O₃, yielding 0.355 g. Report the iron content of the dietary supplement as g FeSO₄•7H₂O per tablet.

16. A 1.4639-g sample of limestone was analyzed for Fe, Ca, and Mg. The iron was determined as Fe₂O₃ yielding 0.0357 g. Calcium was isolated as CaSO₄, yielding a precipitate of 1.4058 g, and Mg was isolated as 0.0672 g of Mg₂As₂O₇. Report the amount of Fe, Ca, and Mg in the limestone sample as %w/w Fe₂O₃, %w/w CaO, and %w/w MgO.

17. The number of ethoxy groups (CH₃CH₂O–) in an organic compound is determined by the following two reactions.

\[\mathrm{R(CH_2CH_3)}_x+x\mathrm{HI}\rightarrow \mathrm{R(OH)}_x + x\mathrm{CH_3CH_2I}\]

\[\mathrm{CH_3CH_2I+Ag^++H_2O\rightarrow AgI}(s)+\mathrm{CH_3CH_2OH}\]

A 36.92-mg sample of an organic compound with an approximate molecular weight of 176 was treated in this fashion, yielding 0.1478 g of AgI. How many ethoxy groups are there in each molecule of the compound?

18. A 516.7-mg sample containing a mixture of K₂SO₄ and (NH₄)₂SO₄ was dissolved in water and treated with BaCl₂, precipitating the SO₄^2– as BaSO₄. The resulting precipitate was isolated by filtration, rinsed free of impurities, and dried to a constant weight, yielding 863.5 mg of BaSO₄. What is the %w/w K₂SO₄ in the sample?

19. The amount of iron and manganese in an alloy can be determined by precipitating the metals with 8-hydroxyquinoline, C₉H₇NO. After weighing the mixed precipitate, the precipitate is dissolved and the amount of 8-hydroxyquinoline determined by another method. In a typical analysis a 127.3-mg sample of an alloy containing iron, manganese, and other metals was dissolved in acid and treated with appropriate masking agents to prevent an interference from other metals. The iron and manganese were precipitated and isolated as Fe(C₉H₆NO)₃ and Mn(C₉H₆NO)₂, yielding a total mass of 867.8 mg. The amount of 8-hydroxyquinolate in the mixed precipitate was determined to be 5.276 mmol. Calculate the %w/w Fe and %w/w Mn in the alloy.

20. A 0.8612-g sample of a mixture of NaBr, NaI, and NaNO₃ was analyzed by adding AgNO₃ and precipitating a 1.0186-g mixture of AgBr and AgI. The precipitate was then heated in a stream of Cl₂, converting it to 0.7125 g of AgCl. Calculate the %w/w NaNO₃ in the sample.

20. The earliest determinations of elemental atomic weights were accomplished gravimetrically. In determining the atomic weight of manganese, a carefully purified sample of MnBr₂ weighing 7.16539 g was dissolved and the Br^– precipitated as AgBr, yielding 12.53112 g. What is the atomic weight for Mn if the atomic weights for Ag and Br are taken to be 107.868 and 79.904, respectively?

22. While working as a laboratory assistant you prepared 0.4 M solutions of AgNO₃, Pb(NO₃)₂, BaCl₂, KI and Na₂SO₄. Unfortunately, you became distracted and forgot to label the solutions before leaving the laboratory. Realizing your error, you label the solutions A–E and perform all possible binary mixings of the five solutions, obtaining the results shown in Figure 8.16 (key: NP means no precipitate formed, W means a white precipitate formed, and Y means a yellow precipitate formed). Identify solutions A–E.

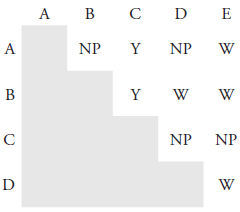
Figure 8.16 Results of the binary mixing of solutions A–E for Problem 8.22.

23. A solid sample has approximately equal amounts of two or more of the following soluble salts: AgNO₃, ZnCl₂, K₂CO₃, MgSO₄, Ba(C₂H₃O₂)₂, and NH₄NO₃. A sample of the solid, sufficient to give at least 0.04 moles of any single salt, was added to 100 mL of water, yielding a white precipitate and a clear solution. The precipitate was collected and rinsed with water. When a portion of the precipitate was placed in dilute HNO₃ it completely dissolved, leaving a colorless solution. A second portion of the precipitate was placed in dilute HCl, yielding a precipitate and a clear solution. Finally, the filtrate from the original precipitate was treated with excess NH₃, yielding a white precipitate. Identify the salts that must be present in the sample, the salts that must be absent and the salts for which there is insufficient information to make this determination.¹³

24. Two methods have been proposed for the analysis of sulfur in impure samples of pyrite, FeS₂. Sulfur can be determined in a direct analysis by oxidizing it to SO₄^2– and precipitating it as BaSO₄. An indirect analysis is possible if the iron is precipitated as Fe(OH)₃ and isolated as Fe₂O₃. Which of these methods will provide a more sensitive determination for sulfur? What other factors should you consider in choosing between these methods?

25. A sample of impure pyrite known to be approximately 90–95% w/w FeS₂ is to be analyzed by oxidizing the sulfur to SO₄^2– and precipitating it as BaSO₄. How many grams of the sample must you take to form at least 1 g of BaSO₄?

26. A series of samples containing any possible combination of KCl, NaCl, and NH₄Cl is to be analyzed by adding AgNO₃ and precipitating AgCl. What is the minimum volume of 5% w/v AgNO₃ necessary to completely precipitate the chloride in any 0.5-g sample?

27. If a precipitate of known stoichiometry does not form, a gravimetric analysis is still feasible if we can establish experimentally the mole ratio between the analyte and the precipitate. Consider, for example, the precipitation gravimetric analysis of Pb as PbCrO₄.¹⁴

(a) For each gram of Pb, how many grams of PbCrO₄ should form?

(b) In a study of this procedure, Grote found that 1.568 g of PbCrO₄ formed for each gram of Pb. What is the apparent stoichiometry between Pb and PbCrO₄?

(c) Does failing to account for the actual stoichiometry lead to a positive determinate error or a negative determinate error?

28. Determine the uncertainty for the gravimetric analysis described in Example 8.1. The expected accuracy for a gravimetric method is 0.1–0.2%. What additional sources of error might account for the difference between your estimated uncertainty and the expected accuracy?

29. A 38.63-mg sample of potassium ozonide, KO₃, was heated to 70^oC for 1 h, undergoing a weight loss of 7.10 mg. A 29.6-mg sample of impure KO₃ experiences a 4.86-mg weight loss when treated under similar condition. What is the %w/w KO₃ in the sample?

30. The water content of an 875.4-mg sample of cheese was determined with a moisture analyzer. What is the %w/w H₂O in the cheese if the final mass was found to be 545.8 mg?

31. Representative Method 8.2 describes a procedure for determining Si in ores and alloys. In this analysis a weight loss of 0.21 g corresponds to 0.1 g of Si. Show that this relationship is correct.

32. The iron in an organometallic compound was determined by treating a 0.4873-g sample with HNO₃ and heating to volatilize the organic material. After ignition, the residue of Fe₂O₃ weighed 0.2091 g.

(a) What is the %w/w Fe in this compound?

(b) The carbon and hydrogen in a second sample of the compound were determined by a combustion analysis. When a 0.5123-g sample was carried through the analysis, 1.2119 g of CO₂ and 0.2482 g of H₂O were collected. What are the %w/w C and %w/w H in this compound and what is the compound’s empirical formula?

33. A polymer’s ash content is determined by placing a weighed sample in a Pt crucible that has been previously brought to a constant weight. The polymer is melted under gentle heating from a Bunsen burner until the volatile vapor ignites. The polymer is allowed to burn until only a non-combustible residue remains. The residue is brought to constant weight at 800^oC in a muffle furnace. The following data were collected during the analysis of two samples of a polymer resin.

Polymer A	g crucible	g crucible + polymer	g crucible + ash
replicate 1	19.1458	21.2287	19.7717
replicate 2	15.9193	17.9522	16.5310
replicate 3	15.6992	17.6660	16.2909

Polymer B	g crucible	g crucible + polymer	g crucible + ash
replicate 1	19.1457	21.0693	19.7187
replicate 2	15.6991	12.8273	16.3327
replicate 3	15.9196	17.9037	16.5110

(a) For each polymer, determine the mean and the standard deviation for the %w/w ash.

(b) Is there any evidence at α = 0.05 for a significant difference between the two polymers? See the appendices for statistical tables.

34. In the presence of water vapor the surface of zirconia, ZrO₂, chemically adsorbs H₂O, forming surface hydroxyls, ZrOH (additional water is physically adsorbed as H₂O). When heated above 200^oC, the surface hydroxyls convert to H₂O(g), releasing one molecule of water for every two surface hydroxyls. Below 200^oC only physically absorbed water is lost. Nawrocki, et al. used thermogravimetry to determine the density of surface hydroxyls on a sample of zirconia that was heated to 700^oC and cooled in a desiccator containing humid N₂.¹⁵ Heating the sample from 200^oC to 900^oC released 0.006 g of H₂O for every gram of dehydroxylated ZrO₂. Given that the zirconia had a surface area of 33 m²/g and that one molecule of H₂O forms two surface hydroxyls, calculate the density of surface hydroxyls in μmol/m².

35. The concentration of airborne particulates in an industrial workplace was determined by pulling the air through a single-stage air sampler equipped with a glass-fiber filter. The air was sampled for 20 min at a rate of 75 m³/h. At the end of the sampling period, the filter’s mass was found to have increased by 345.2 mg. What is the concentration of particulates in the air sample in mg/m³ and mg/L?

36. The fat content of potato chips is determined indirectly by weighing a sample before and after extracting the fat with supercritical CO₂. The following data were obtained for the analysis of potato chips.¹⁶

Sample Number	Initial Mass (g)	Final Mass (g)
1	1.1661	0.9253
2	1.1723	0.9252
3	1.2525	0.9850
4	1.2280	0.9562
5	1.2837	1.0119

(a) Determine the mean and standard deviation for the %w/w fat.

(b) This sample of potato chips is known to have a fat content of 22.7% w/w. Is there any evidence for a determinate error at α = 0.05? See the appendices for statistical tables.

37. Delumyea and McCleary reported results for the %w/w organic material in sediment samples collected at different depths from a cove on the St. Johns River in Jacksonville, FL.¹⁷ After collecting a sediment core, they sectioned it into 2-cm increments. Each increment was treated using the following procedure:

(a) The sediment was placed in 50 mL of deionized water and the resulting slurry filtered through preweighed filter paper;

(b) The filter paper and the sediment were placed in a preweighed evaporating dish and dried to a constant weight in an oven at 110^oC;

(c) The evaporating dish containing the filter paper and the sediment were transferred to a muffle furnace where the filter paper and any organic material in the sample were removed by ashing;

(d) Finally, the inorganic residue remaining after ashing was weighed.

Using the following data, determine the %w/w organic material as a function of the average depth for each increment.

	Mass (g) of...		Mass (g) of Filter Paper, Dish, and Sediment...
Depth (cm)	Filter Paper	Dish	After Drying	After Ashing
0–2	1.590	43.21	52.10	49.49
2–4	1.745	40.62	48.83	46.00
4–6	1.619	41.23	52.86	47.84
6–8	1.611	42.10	50.59	47.13
8–10	1.658	43.62	51.88	47.53
10–12	1.628	43.24	49.45	45.31
12–14	1.633	43.08	47.92	44.20
14–16	1.630	43.96	58.31	55.53
16–18	1.636	43.36	54.37	52.75

38. Yao, et al. recently described a method for the quantitative analysis of thiourea based on its reaction with I₂.¹⁸

\[\mathrm{CS(NH_2)_2+4I_2+6H_2O\rightarrow (NH_4)_2SO_4+8HI+CO_2}\]

The procedure calls for placing a 100-μL aqueous sample containing thiourea in a 60-mL separatory funnel and adding 10 mL of a pH 7 buffer and 10 mL of 12 μM I₂ in CCl₄. The contents of the separatory funnel are shaken and the organic and aqueous layers allowed to separate. The organic layer, which contains the excess I₂, is transferred to the surface of a piezoelectric crystal on which a thin layer of Au has been deposited. After allowing the I₂ to adsorb to the Au, the CCl₄ is removed and the crystal’s frequency shift, ∆f, measured. The following data is reported for a series of thiourea standards.

[thiourea] (M)	∆f(Hz)	[thiourea] (M)	∆f (Hz)
3.00×10^–7	74.6	1.50×10^–6	327
5.00×10^–7	120	2.50×10^–6	543
7.00×10^–7	159	3.50×10^–6	789
9.00×10^–7	205	5.00×10^–6	1089

(a) Characterize this method with respect to the scale of operation shown in Figure 3.5 of Chapter 3.

(b) Using a regression analysis, determine the relationship between the crystal’s frequency shift and the concentration of thiourea.

(c) If a sample containing an unknown amount of thiourea gives a ∆f of 176 Hz, what is the molar concentration of thiourea in the sample?

(d) What is the 95% confidence interval for the concentration of thiourea in this sample assuming one replicate? See the appendices for statistical tables.

8.5.3 Solutions to Practice Exercises

Practice Exercise 8.1

The solubility reaction for CaC₂O₄ is

\[\mathrm{CaC_2O_4}(s)\rightleftharpoons \mathrm{Ca^{2+}}(aq)+\mathrm{C_2O_4^{2-}}(aq)\]

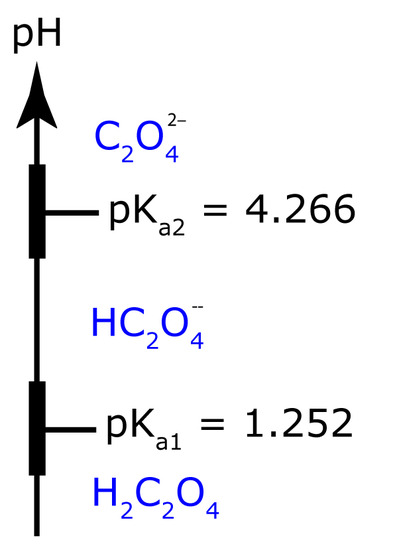
To minimize solubility, the pH needs to be basic enough that oxalate, C₂O₄^2–, does not react to form HC₂O₄^– or H₂C₂O₄. The ladder diagram for oxalic acid, including approximate buffer ranges, is shown in Figure 8.17. Maintaining a pH greater than 5.3 ensures that C₂O₄^2– is the only important form of oxalic acid in solution, minimizing the solubility of CaC₂O₄.

Figure 8.17 pH ladder diagram for oxalic acid, H₂C₂O₄.

Click here to return to the chapter.

Practice Exercise 8.2

A conservation of mass requires that all the zinc in the alloy is found in the final product, Zn₂P₂O₇. We know that there are 2 moles of Zn per mole of Zn₂P₂O₇; thus

\[\mathrm{0.1163\;g\;Zn_2P_2O_7\times\dfrac{2\;mol\;Zn}{304.72\;g\;Zn_2P_2O_7}\times\dfrac{65.39\;g\;Zn}{mol\;Zn}=4.991\times10^{-2}\;g\;Zn}\]

This is the mass of Zn in 25% of the sample (a 25.00 mL portion of the 100.0 mL total volume). The %w/w Zn, therefore, is

\[\mathrm{\dfrac{4.991\times10^{-2}\;g\;Zn\times4}{0.7336\;g\;sample}\times100=27.22\%\;w/w\;Zn}\]

For copper, we find that

\[\mathrm{0.1163\;g\;CuSCN\times\dfrac{1\;mol\;Cu}{121.64\;g\;CuSCN}\times\dfrac{63.55\;g\;Cu}{mol\;Cu}=0.1249\;g\;Cu}\]

\[\mathrm{\dfrac{0.1249\;g\;Cu\times4}{0.7336\;g\;sample}\times100=67.88\%\;w/w\;Cu}\]

Click here to return to the chapter.

Practice Exercise 8.3

The masses of the solids provide us with the following equations

\[\textrm{g NaCl + g KCl = 0.2692 g}\]

\[\mathrm{(g\;KClO_4)_{NaCl}+(g\;KClO_4)_{KCl}=0.5713\;g}\]

where (g KClO₄)_NaCl and (g KClO₄)_KCl are the masses of KClO₄ from the reaction of HClO₄ with NaCl and KCl. With two equations and four unknowns, we need two additional equations to solve the problem. A conservation of mass requires that all the chlorine in NaCl is found in the (KClO₄)_NaCl; thus

\[\mathrm{(g\;KClO_4)_{NaCl}=g\;NaCl\times\dfrac{1\;mol\;Cl}{58.44\;g\;NaCl}\times\dfrac{138.55\;g\;KClO_4}{mol\;Cl}}\]

\[\mathrm{(g\;KClO_4)_{NaCl}=2.3708\times g\;NaCl}\]

Using the same approach for KCl gives

\[\mathrm{(g\;KClO_4)_{KCl}=g\;KCl\times\dfrac{1\;mol\;Cl}{74.55\;g\;KCl}\times\dfrac{138.55\;g\;KClO_4}{mol\;Cl}}\]

\[\mathrm{(g\;KClO_4)_{KCl}=1.8584\times g\;KCl}\]

Substituting the equations for (g KClO₄)_NaCl and (g KClO₄)_KCl into the equation for their combined masses leaves us with two equations and two unknowns.

\[\textrm{g NaCl + g KCl = 0.2692 g}\]

\[\mathrm{2.3708\times g\;NaCl+1.8584\times g\;KCl=0.5713\;g}\]

Multiplying the first equation by 1.8584 and subtracting the second equation gives

\[\mathrm{-0.5124\times g\;NaCl=-0.07102\;g}\]

\[\textrm{g NaCl = 0.1386 g}\]

To report the %w/w Na₂O in the sample, we use a conservation of mass on sodium.

\[\mathrm{0.1386\;g\;NaCl\times\dfrac{1\;mol\;Na}{58.44\;g\;NaCl}\times\dfrac{61.98\;g\;Na_2O}{2\;mol\;Na}=0.07350\;g\;Na_2O}\]

\[\mathrm{\dfrac{0.07350\;g\;Na_2O}{0.8143\;g\;sample}\times100=9.026\%\;w/w\;Na_2O}\]

Click here to return to the chapter.

Practice Exercise 8.4

To find the mass of (NH₄)₃PO₄•12MoO₃ producing 0.600 g of PbMoO₃, we first use a conservation of mass for molybdenum; thus

\[\mathrm{0.600\;g\;PbMoO_3\times\dfrac{1\;mol\;Mo}{351.14\;g\;PbMoO_3}\times\dfrac{1876.38\;g\;(NH_4)_3PO_4\cdot 12MoO_3}{12\;mol\;Mo}=0.2672\;g\;(NH_4)_3PO_4\cdot 12MoO_3}\]

Next, to convert this mass of (NH₄)₃PO₄•12MoO₃ to a mass of Na₃PO₄, we use a conservation of mass on PO₄^3–.

\[\mathrm{0.2672\;g\;(NH_4)_3PO_4\cdot 12MoO_3\times\dfrac{1\;mol\;PO_4^{3-}}{1876.38\;g\;(NH_4)_3PO_4\cdot 12MoO_3}\times\dfrac{163.94\;g\;Na_3PO_4}{mol\;PO_4^{3-}}=0.02335\;g\;Na_3PO_4}\]

Finally, we convert this mass of Na₃PO₄ to the corresponding mass of sample.

\[\mathrm{0.02335\;g\;Na_3PO_4\times\dfrac{100\;g\;sample}{12.5\;g\;Na_3PO_4}=0.187\;g\;sample}\]

A sample of 0.187 g is sufficient to guarantee 0.600 g of PbMoO₃. If a sample contains more than 12.5% Na₃PO₄, then a 0.187-g sample will produce more than 0.600 g of PbMoO₃.

Click here to return to the chapter.

Practice Exercise 8.5

To determine which form has the greatest sensitivity, we use a conservation of mass for iron to find the relationship between the precipitate’s mass and the mass of iron.

\[\mathrm{g\;FeO=g\;Fe\times\dfrac{1\;mol\;Fe}{55.85\;g\;Fe}\times\dfrac{71.85\;g\;FeO}{mol\;Fe}= 1.286\times g\;Fe}\]

\[\mathrm{g\;Fe_2O_3=g\;Fe\times\dfrac{1\;mol\;Fe}{55.85\;g\;Fe}\times\dfrac{159.70\;g\;Fe_2O_3}{2\;mol\;Fe}=1.430\times g\;Fe}\]

\[\mathrm{g\;Fe_3O_4=g\;Fe\times\dfrac{1\;mol\;Fe}{55.85\;g\;Fe}\times\dfrac{231.55\;g\;Fe_3O_4}{3\;mol\;Fe}=1.382\times\;g\;Fe}\]

Of the three choices, the greatest sensitivity is obtained with Fe₂O₃ because it provides the largest value for k.

Click here to return to the chapter.

Practice Exercise 8.6

From 100–250^oC the sample loses 13.8% of its mass, or a loss of

\[\mathrm{0.138\times130.35\;g/mol=18.0\;g/mol}\]

consistent with the loss of H₂O(g), leaving a residue of MgC₂O₄. From 350–550^oC the sample loses 55.23% of its original mass, or a loss of

\[\mathrm{0.5523\times130.35\;g/mol=71.99\;g/mol}\]

This weight loss is consistent with the simultaneous loss of CO(g) and CO₂(g), leaving a residue of MgO.

We can analyze the mixture by heating a portion of the sample to 300^oC, 600^oC, and 1000^oC, recording the mass at each temperature. The loss of mass between 600^oC and 1000^oC, ∆m₂, is due to the loss of CO₂(g) from the decomposition of CaCO₃ to CaO, and is proportional to the mass of CaC₂O₄•H₂O in the sample.

\[\mathrm{g\;CaC_2O_4\cdot H_2O}=\Delta m_2\times\mathrm{\dfrac{1\;mol\;CO_2}{44.01\;g\;CO_2}\times\dfrac{146.11\;g\;CaC_2O_4\cdot H_2O}{mol\;CO_2}}\]

The change in mass between 300^oC and 600^oC, ∆m₁, is due to the loss of CO(g) from CaC₂O₄•H₂O and the loss of CO(g) and CO₂(g) from MgC₂O₄•H₂O. Because we already know the amount of CaC₂O₄•H₂O in the sample, we can calculate its contribution to ∆m₁.

\[(\Delta m_1)\mathrm{_{Ca}=g\;CaC_2O_4\cdot H_2O\times\dfrac{1\;mol\;CO}{146.11\;g\;CaC_2O_4\cdot H_2O}\times\dfrac{28.01\;g\;CO}{mol\;CO}}\]

The change in mass due to the decomposition of MgC₂O₄•H₂O

\[(\Delta m_1)_\textrm{Mg}=(\Delta m_1)-(\Delta m_1)_\textrm{Ca}\]

provides the mass of MgC₂O₄•H₂O in the sample.

\[\mathrm{g\;MgC_2O_4\cdot H_2O}=(\Delta m_1)_\textrm{Mg}\mathrm{\times\dfrac{1\;mol\;(CO + CO_2)}{130.35\;g\;MgC_2O_4\cdot H_2O}\times\dfrac{78.02\;g\;(CO+CO_2)}{1\;mol\;(CO+CO_2)}}\]

Click here to return to the chapter.

Practice Exercise 8.7

In Practice Exercise 8.6 we developed an equation for the mass of CaC₂O₄•H₂O in a mixture of CaC₂O₄•H₂O, MgC₂O₄•H₂O, and inert materials. Adapting this equation to a sample containing CaC₂O₄, CaC₂O, and inert materials is easy; thus

\[\mathrm{g\;CaC_2O_4=(0.1794\;g-0.1294\;g)\times\dfrac{1\;mol\;CO_2}{44.01\;g\;CO_2}\times\dfrac{128.10\;g\;CaC_2O_4}{mol\;CO_2}=0.1455\;g\;CaC_2O_4}\]

The %w/w CaC₂O₄ in the sample is

\[\mathrm{\dfrac{0.1455\;g\;CaC_2O_4}{0.3317\;g\;sample}\times100=43.86\%\;w/w\;CaC_2O_4}\]

Click here to return to the chapter.

Contributors and Attributions

• David Harvey (DePauw University)