1.8: Heat Capacity Ratios for Gases (Cp/Cv)
- Page ID
- 63354
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Measuring the Ratio of the Heat Capacity at Constant Pressure to the Heat Capacity at Constant Volume for a Gas (Cp/Cv)
Introduction
The equipartition theorem states that any quadratic energy term such as kinetic energy contributes equality to the internal energy of a system in thermal equilibrium. This means that for a gas each degree of freedom contributes ½ RT to the internal energy on a molar basis (R is the ideal gas constant)
An atom of a monoatomic gas can move in three independent directions so the gas has three degrees of freedom due to its translational motion. Therefore its internal energy, U, follows the equation U = 3/2 RT. The heat capacity at constant volume, Cv, is the derivative of the internal energy with respect to the temperature, so for our monoatomic gas, Cv = 3/2 R.
The heat capacity at constant pressure can be estimated because the difference between the molar Cp and Cv is R; Cp – Cv = R. Although this is strictly true for an ideal gas it is a good approximation for real gases.
The rotation of gas molecules adds additional degrees of freedom. A linear molecule rotates along two independent axes. Therefore a linear molecule has two rotational degrees of freedom. The total number of degrees of freedom for a linear molecule is 5 so its internal energy is U = 5/2 RT, its molar heat capacity at constant volume is Cv = 5/2 R and its molar heat capacity at constant pressure will be Cp = 7/2 R.
A nonlinear molecule rotates along three independent axes. Therefore a linear molecule has three rotational degrees of freedom. The total number of degrees of freedom for a linear molecule is 6 so its internal energy is U = 3 RT, its molar heat capacity at constant volume is Cv = 3 R and its molar heat capacity at constant pressure will be Cp = 4 R.
Vibrations may add to the heat capacity but only if they are thermally accessible. The vibrational temperature, \(\Theta _{vib}\), is defined by the equation:
\[\Theta _{vib}=\frac{hc\nu}{k} \nonumber \]
where h is Planck's constant, c is the speed of light, v is the frequency and k is Boltzmann's constant. The vibrational contribution to the molar heat capacity from a given vibrational degree of freedom of frequency ν is given by:
\[\left ( C_{v} \right )_{vib}=N\times R\times \left ( \frac{ \Theta _{vib}}{T} \right )^{2}\times \frac{\exp \left ( \Theta _{vib}/T \right )}{ \left \{ \exp \left ( \Theta _{vib}/T \right ) -1 \right \}^{2}} \nonumber \]
where N is the number of moles, R is the ideal gas constant and T is temperature. The total contribution to the heat capacity at constant volume from all vibrational degrees of freedom is:
\[ C_{vib, Total}=\sum \left ( C_{v} \right )_{vib} \nonumber \]
where the summation is over all vibrational degrees of freedom, 3N-5 (linear molecules) or 3N-6 (non-linear molecules)
Experimental
You have a large bottle fitted with a gas inlet and a pressure gauge attached to a stopper in the neck of the bottle, Figure 1.
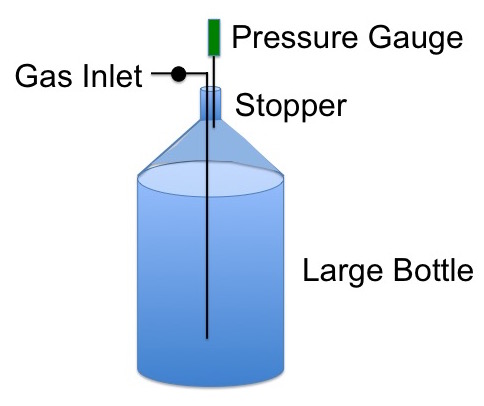
Figure 1. Setup for measuring the ratio of Cp/Cv for gases.
Now you begin with the gas at atmospheric pressure (760 Torr) and then add gas to increase the pressure inside the bottle by a small amount, say 1.5% (11.4 Torr).
Now very quickly pop the stopper from the bottleneck and return it very quickly to its original position, Figure 2.
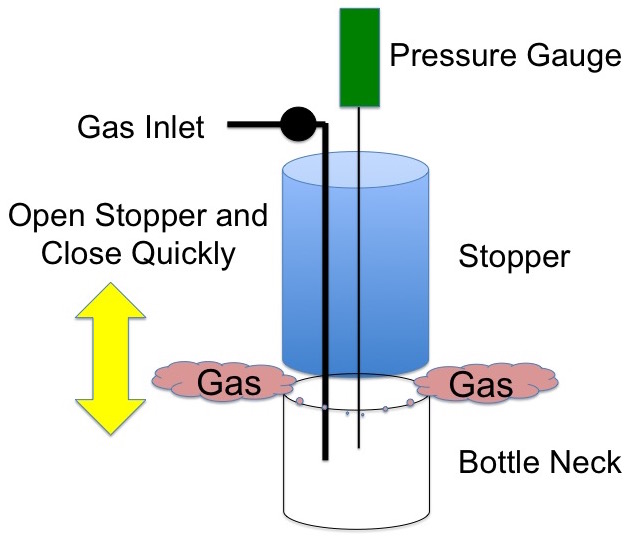
Figure 2. Remove the stopper very quickly.
Before reading further pause for a moment and consider the following two questions. What happens to the pressure and temperature in the bottle when you remove the stopper? How will the pressure and temperature change over the next few minutes? Note that the bottle is not thermally isolated from its surroundings.
First the gas returns to the ambient atmospheric pressure when you remove the stopper from the bottle and gas escapes. Assuming you remove the stopper and return it quickly, this process is adiabatic. An adiabatic process is a process where heat is not transferred (dq = 0). When the gas expands it does work against its surroundings and it cools a little.
Second after a few minutes the gas returns to the original ambient temperature because the bottle is not thermally isolated. What does this do to its pressure? The pressure rises because of the ideal gas law, PV=nRT. Rearranging we have P=nRT/V. As the temperature of the gas returns to ambient the pressure rises.
The extent of the pressure increase is related to the ratio of the gas’s heat capacity at constant pressure to its heat capacity at constant volume. This ratio is given by:
\[\frac{C_{p}}{C_{v}}=\frac{\ln P_{1}-\ln P_{2}}{\ln P_{1}-\ln P_{3}} \nonumber \]
Where P1 is the initial pressure of the gas, P2 is the ambient pressure of the room and P3 is the final pressure reached after the stopper is popped.
Procedure
Construct an apparatus as described above. Gases will be assigned in class and may include nitrogen, oxygen, helium, argon and carbon dioxide. Perform the experiment 10 times for each assign gas. Calculate the mean and standard deviations for your measurements. Compare your measurements of Cp/Cv with available literature values and values calculated from the Equipartition of Energy Theorem.

