4.5: "Component Within:" Equality Pattern and Conversions
- Page ID
- 213286
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Use information in a given phrase or word problem to write "component within" equalities.
- Apply a "component within" conversion factor to convert between the molar quantity of a compound and one of its constituent elements.
- Apply multiple conversion factors to convert between the molar quantity or particle count of a compound and the particle count or molar quantity of one of its constituent elements.
Upon establishing that a "component within" molar relationship is required to solve a problem, a corresponding "component within" equality must be developed. Then, using dimensional analysis, the resultant equality can be applied as a conversion factor, in order to bring about a desired unit transformation.
"Component Within" Equality Pattern
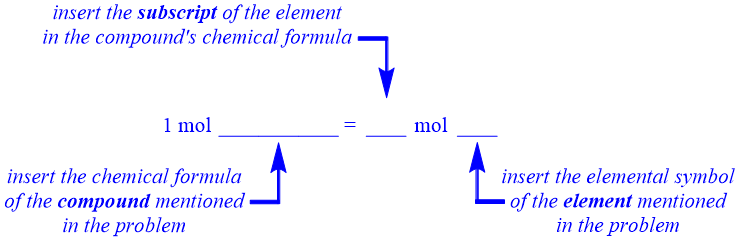
As stated in Section 4.1, an equality pattern contains one number and two units on both sides of an equal sign. One of these units, "mol," is defined on both sides of a "component within" equality pattern, as shown below. Neither secondary unit is specified in this equality pattern. These positions, which are indicated as "blanks" in the equality pattern shown below, should be occupied by units that are relevant to the identities of the specific chemicals that are referenced in a given problem. As indicated below, the secondary unit on the left side of a "component within" equality should be the chemical formula of the compound that is referenced in the given problem, and the final unit position on the right side of the equality should be occupied by the chemical formula of the specific element that is being considered. Therefore, unlike in an Avogadro's number equality, the chemical formulas written on both sides of a "component within" equality do not match. As stated in Section 4.3, a chemical name should not be used in this, or any, equality. Finally, the relative order of the two units on the right side of a "component within" equality should not be interchanged.
As was the case in an Avogadro's number equality, a numerical value of "1" is specified on the left side of a "component within" equality. Recall that Avogadro's number, 6.02 × 1023, occupies the numerical position on the right side of an Avogadro's number equality. However, a "blank" is present in the corresponding position in the "component within" equality pattern that is shown below. A "component within" equality establishes the relative ratios of the elements that are present within a compound, as indicated by the subscripts found on the elemental symbols within that compound's chemical formula. Therefore, the subscript for the particular element being considered should occupy the numerical position on the right side of a "component within" equality.
For example, consider a problem that requires a calculation of how many moles of Ag are present in 11.5 moles of Ag2CO3.
Because both a complete molecule, Ag2CO3, silver carbonate, and a component element found within this molecule, Ag, silver, are referenced in the given problem, a "component within" equality should be developed. Since all chemical information was provided in the form of elemental symbols and/or chemical formulas, the symbols "Ag2CO3" and "Ag" are directly incorporated into the secondary unit positions on the left and right sides, respectively, of the equality that is being developed. Finally, since the subscript for Ag, the particular element that is being considered in this problem, is a "2" in the chemical formula for the compound, Ag2CO3, a 2 is inserted into the numerical position on the right side of this "component within" equality, as shown below.
1 mol Ag2CO3 = 2 mol Ag
Write an equality that could be applied to calculate how many moles of ammonium cyanide contain 57.2 moles of nitrogen.
Solution
Because both a complete molecule, ammonium cyanide, NH4CN, and a component element found within this molecule, nitrogen, N, are referenced in the given problem, a "component within" equality should be developed. In order to recognize the "component within" indicator within this problem, the chemical formulas of each of these chemicals must be determined from the chemical names that were provided. The chemical formula for ammonium cyanide, "NH4CN," is derived by applying the Chapter 3 rules for determining ionic chemical formulas. As chemical names should not be used in this, or any, equality, the chemical formulas for ammonium cyanide, "NH4CN," and nitrogen, "N," are incorporated into the secondary unit positions on the left and right sides, respectively, of the equality that is being developed.
Determining the numerical value that should be inserted into the right side of this equality is moderately challenging, as the specified element, nitrogen, N, appears in two distinct places in the chemical formula of ammonium cyanide, NH4CN. In order to determine the true number of nitrogens that are present within ammonium cyanide, the subscripts on both nitrogens, which are each unwritten "1"s, must be added. Therefore, a 2 is inserted into the numerical position on the right side of this "component within" equality, as shown below.
1 mol NH4CN = 2 mol N
Write an equality that could be applied to calculate how many moles of oxygen are present in 3.08 moles of lead (II) phosphate.
- Answer
- Because both a complete molecule, lead (II) phosphate, Pb3(PO4)2, and a component element found within this molecule, oxygen, O, are referenced in the given problem, a "component within" equality should be developed. In order to recognize the "component within" indicator within this problem, the chemical formulas of each of these chemicals must be determined from the chemical names that were provided. The chemical formula for lead (II) phosphate, "Pb3(PO4)2," is derived by applying the Chapter 3 rules for determining ionic chemical formulas. As chemical names should not be used in this, or any, equality, the chemical formulas for lead (II) phosphate, "Pb3(PO4)2," and oxygen, "O," are incorporated into the secondary unit positions on the left and right sides, respectively, of the equality that is being developed.
Determining the numerical value that should be inserted into the right side of this equality is moderately challenging, as the specified element, oxygen, O, appears inside parentheses within lead (II) phosphate's chemical formula, Pb3(PO4)2. Recall that parentheses are utilized in ionic chemical formulas to offset polyatomic ions as units, and the subscript of "2" that is written outside of the parentheses indicates that 2 phosphate ions are present within this compound. Since each phosphate ion contains 4 oxygens, the subscript on oxygen must be multiplied by the subscript that is written outside of the parentheses, in order to determine the true number of oxygens that are present within lead (II) phosphate. Therefore, an 8 is inserted into the numerical position on the right side of this "component within" equality, as shown below.1 mol Pb3(PO4)2 = 8 mol O
Applying "Component Within" Equalities as Conversion Factors
Once an appropriate "component within" equality has been developed, the information that it contains can be re-written in the form of a conversion factor, which can then be applied to bring about a desired unit transformation. As stated previously, the quantity containing the unit being canceled must be written in the denominator of a conversion factor. This will cause the given unit, which appears in a numerator, to be divided by itself, since the same unit appears in the denominator of the conversion factor. Since any quantity that is divided by itself "cancels," orienting the conversation factor in this way results in the elimination of the undesirable unit. However, remember that both components of the equalities that are developed within this chapter contain two units. Therefore, in order to achieve complete unit cancelation, a conversion factor that results in the simultaneous elimination of both units must be applied.
For example, use a conversion factor based on the equality developed above for Ag to calculate how many moles of Ag are present in 11.5 moles of Ag2CO3.
As stated above, because both a complete molecule, Ag2CO3, and a component element found within this molecule, Ag, are referenced in the given problem, a "component within" equality should be developed and applied to solve this problem. The equality that was generated based on the subscript information that corresponds to Ag in Ag2CO3 is replicated below.
1 mol Ag2CO3 = 2 mol Ag
To create a conversion factor from this equality, the quantity on the left side of the equal sign is written in the numerator of a fraction, and the other quantity is written in the denominator. A second conversion factor can be developed by interchanging where each quantity is written, relative to the fraction bar. Both of the resultant conversion factors are shown below.
\( \dfrac{1 \text{ mol } \ce{Ag_2CO_3}}{2 {\text{ mol }} \text {Ag }} \) and \( \dfrac{2 {\text{ mol }} \text {Ag }}{1 \text{ mol } \ce{Ag_2CO_3}} \)
However, only one of these conversion factors will allow for the complete cancelation of the given unit, "moles of Ag2CO3," since both of the units that are being canceled must be written in the denominator of the conversion factor that should be applied to solve the given problem. Since the intent of this problem is to eliminate the unit "moles of Ag2CO3," the conversion factor on the right must be used. Therefore,
\( {11.5 \; \cancel{\rm{mol} \; \rm{Ag_2CO_3}}} \times\) \( \dfrac{2 \; \rm{mol} \; \rm{Ag}}{1 \; \cancel{\rm{mol} \; \rm{Ag_2CO_3}}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. Recall that, when using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division. In this case,
11.5 × (2 mol Ag ÷ 1) = 23 mol Ag ≈ 23.0 mol Ag
Finally, remember that the correct number of significant figures should be applied to any calculated quantity. Since the math involved in dimensional analysis is multiplication and division, the number of significant figures in each number being multiplied or divided must be counted, and the answer must be limited to the lesser count of significant figures. The numerical quantities within a "component within" equality are exact values, meaning that they are considered to have infinitely-many significant figures and will never limit the number of significant figures in a calculated answer. However, the given number, 11.5, is not exact, and its significant figures must be considered. As this value contains three significant figures, the final answer should be rounded to three significant digits, as shown above.
Use a conversion factor based on the equality developed in Example \(\PageIndex{1}\) to calculate how many moles of ammonium cyanide contain 57.2 moles of nitrogen.
Solution
Because both a complete molecule, ammonium cyanide, NH4CN, and a component element found within this molecule, nitrogen, N, are referenced in the given problem, a "component within" equality should be developed and applied to solve this problem. The equality that was generated based on the subscript information that corresponds to nitrogen, N, in ammonium cyanide, NH4CN, is replicated below.
1 mol NH4CN = 2 mol N
In order to completely eliminate the unit "moles of nitrogen," the quantity on the right side of this equality becomes the denominator in the conversion factor that is applied to solve the given problem. The remaining portion of the "component within" equality is written in the numerator in the resultant conversion factor, as shown below.
\( {\text {57.2}} \) \({\cancel{\rm{mol} \; \rm{N}}} \times\) \( \dfrac{1 \; \rm{mol} \; \rm{NH_4CN}}{2 \; \cancel{\rm{mol} \; \rm{N}}}\) = \( {\text {28.6}}\) \({\rm{mol} \; \rm{NH_4CN}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. When using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division. Applying the correct number of significant figures to the calculated quantity results in the final answer that is shown above.
Use a conversion factor based on the equality developed in Exercise \(\PageIndex{1}\) to calculate how many moles of oxygen are present in 3.08 moles of lead (II) phosphate.
- Answer
- Because both a complete molecule, lead (II) phosphate, Pb3(PO4)2, and a component element found within this molecule, oxygen, O, are referenced in the given problem, a "component within" equality should be developed and applied to solve this problem. The equality that was generated based on the subscript information that corresponds to oxygen, O, in lead (II) phosphate, Pb3(PO4)2, is replicated below.
1 mol Pb3(PO4)2 = 8 mol O
In order to completely eliminate the unit "moles of lead (II) phosphate," the quantity on the left side of this equality becomes the denominator in the conversion factor that is applied to solve the given problem. The remaining portion of the equality is written in the numerator in the resultant conversion factor, as shown below.\( {3.08 \; \cancel{\rm{mol} \; \rm{Pb_3(PO_4)_2 }}} \times\) \( \dfrac{8 \; \rm{mol} \; \rm{O}}{1 \; \cancel{\rm{mol} \; \rm{Pb_3(PO_4)_2}}}\) = \( {\text {24.64}}\) \({\rm{mol} \; \rm{O}}\) ≈ \( {\text {24.6}}\) \({\rm{mol} \; \rm{O}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. When using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division. Applying the correct number of significant figures to the calculated quantity results in the final answer that is shown above.
Consider a problem that requires a calculation of how many atoms of carbon are present in 0.3692 moles of tricarbon octachloride.
- Identify the indicator information in the given problem, and state which molar standard is associated with each indicator.
- Write an equality pattern that corresponds to each of the indicators identified in Part (a).
- Using dimensional analysis, apply the equalities that were developed in Part (b) as conversion factors, in order to bring about the desired unit transformation and solve the given problem.
- Answer
- Indicator Information
The word "atoms" indicates that an Avogadro's number equality should be developed and applied to solve this problem.
Additionally, because both a complete molecule, tricarbon octachloride, C3Cl8, and a component element found within this molecule, carbon, C, are referenced in the given problem, a "component within" equality should also be developed and applied to solve this problem. In order to recognize the "component within" indicator, the chemical formulas of each of these chemicals must be determined from the chemical names that were provided. The subscripts in the chemical formula for tricarbon octachloride, C3Cl8, are determined based on the numerical prefixes in the given chemical name, in alignment with the Chapter 3 rules for determining covalent chemical formulas.
Equality Patterns
Avogadro's Number Equality Pattern
The word "atoms" indicates that an Avogadro's number equality should be developed. Furthermore, since "atoms" is an indicator word, this word is inserted as the second unit on the right side of this type of equality. As multiple chemicals are referenced within this problem, the chemical that is written in closest physical proximity to the indicator word "atoms" is selected for incorporation into the Avogadro's number equality. The chemical that is most closely associated with the word "atoms" in the given problem is "carbon." However, as chemical names should not be used in this, or any, equality, the corresponding elemental symbol, "C," is incorporated into both of the secondary unit positions in the equality that is being developed. The resultant Avogadro's number equality is shown below.1 mol C = 6.02 × 1023 C atoms
Because the given chemical information is an elemental name, the indicator word "atoms" appropriately corresponds to the chemical formula that is applied in this equality.
"Component Within" Equality Pattern
Because both a complete molecule, tricarbon octachloride, C3Cl8, and a component element found within this molecule, carbon, C, are referenced in the given problem, a "component within" equality should also be developed. As chemical names should not be used in this, or any, equality, the chemical formulas for tricarbon octachloride, "C3Cl8," and carbon, "C," are incorporated into the secondary unit positions on the left and right sides, respectively, of the "component within" equality being developed. Finally, since the subscript for carbon, C, the particular element that is being considered in this problem, is a "3" in the chemical formula of tricarbon octachloride, C3Cl8, a 3 is inserted into the numerical position on the right side of the resultant "component within" equality, as shown below.1 mol C3Cl8 = 3 mol C
Dimensional Analysis
In order to completely eliminate the given unit, "moles of tricarbon octachloride," a conversion factor based on the "component within" equality must be applied first. Specifically, the quantity on the left side of this equality becomes the denominator in the first conversion factor that is applied to solve the given problem. The remaining portion of the equality is written in the numerator in the resultant conversion factor.
However, the unit that results upon the cancelation of "moles of tricarbon octachloride" is "mol C," which is not the desired final unit. Therefore, the Avogadro's number equality must be applied as a second conversion factor. In order to completely cancel the intermediate unit of "mol C," the quantity on the left side of the Avogadro's number equality becomes the denominator in the second conversion factor that is applied to solve the given problem. The remaining portion of the equality is written in the numerator in the resultant conversion factor. While reversing the order of the two units on the right side of an Avogadro's number equality is not absolutely necessary, doing so more clearly illustrates that the answer will ultimately be expressed in the desired unit, as shown below.\( {0.3692 \; \cancel{\rm{mol} \; \rm{C_3Cl_8}}} \times\) \( \dfrac{3 \; \bcancel{\rm{mol} \; \rm{C}}}{1 \; \cancel{\rm{mol} \; \rm{C_3Cl_8}}}\) × \( \dfrac{6.02 \times 10^{23} \; \rm{atoms} \; \rm{C}}{1 \; \bcancel{\rm{mol} \; \rm{C}}}\) = \( {\text {6.667752}} \times {\text{10}^{23}}\) \({\rm{atoms} \; \rm{C}}\)
≈ \( {\text {6.67}} \times {\text{10}^{23}}\) \({\rm{atoms} \; \rm{C}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. When using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division, and any quantity that is expressed in scientific notation should be offset by an additional set of parentheses. Applying the correct number of significant figures to the calculated quantity results in the final answer that is shown above.

