3.3: Ionic Bonding: Cation Formation, Symbolism, and Nomenclature

Learning Objectives

• Define cation.
• Explain how neutral atoms ionize to form cations.
• Determine the charges achieved when main group metals ionize.
• Symbolize and name main group cations.

As stated previously, electrons are most stable when they exist in pairs.  Only the noble gases naturally have an octet configuration, meaning that they possess eight, fully-paired valence electrons.  The remaining elements must, therefore, gain or lose up to three valence electrons, in order to achieve an octet configuration.  Gaining or losing more than this number of electrons is energetically-unfavorable and will not occur.  Finally, note that inner shell electrons are always fully-paired and, therefore, are considered stable.  As a result, inner shell electrons do not participate in the ionization process.

By changing the number of electrons that are contained within a particle, an imbalance is created between the number of positively-charged protons and negatively-charged electrons that it possesses.  As a result, this new particle will no longer be neutral, but rather will bear a net charge.  Thus, the term "atom" no longer applies to this new particle, since an atom must be net-neutral, by definition.  The resultant particle is instead is classified as an ion, as it has become a charged particle.

Cations

As stated previously, cations are positively-charged ions that are most often formed when metals, which are found on the left side of the periodic table, lose valence electrons. 

For example, consider calcium (Ca).

An atom of calcium contains 20 protons, because its atomic number is 20, and 20 electrons, in order to be net-neutral.  Recall that the number of neutrons present in an atom can vary, depending on which isotope of calcium is being considered.  The overall charge of a particle is not influenced by the number of neutrons that are present, as neutrons are uncharged, by definition.  Therefore, the neutron count for this particular calcium atom is irrelevant to the analysis that will be performed in the following paragraphs and will not be discussed further.

Calcium's electron configuration, which was first determined in Exercise 2.6.1, is shown below.  

1s²2s²2p⁶3s²3p⁶4s²

Calcium has 2 valence electrons, as determined either by totaling the electrons found within the orbitals in the highest occupied energy level, which, in this case, is the 4s orbital, or by identifying the "A/B System" group number for the column in which the element is found.

In order to be stable, a particle must possess an octet, or eight, fully-paired valence electrons.  Therefore, calcium would need to gain 6 electrons in order to achieve an octet configuration.  However, as stated above, gaining more than three electrons is energetically-unfavorable and will not occur.  Unfortunately, the valence electron count of all metals renders them unable to gain enough electrons to achieve an octet configuration.

Alternatively, an atom can lose its valence electrons, in order to achieve an octet configuration.  Because calcium only has two valence electrons, losing both of them is possible, as doing so would not exceed the maximum loss-limit of three electrons.  Therefore, calcium will lose both of its valence electrons.

Consider the impact that this will have on calcium's electron configuration.  If both of the valence electrons in the 4s orbital are lost, as shown below,

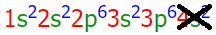
the following electron configuration results:

1s²2s²2p⁶3s²3p⁶

In this new electron configuration, the highest occupied energy level is no longer indicated by a 4.  Instead, the 3^rd energy level is now the valence shell.  Therefore, in order to determine the number of valence electrons contained in this new particle, only those electrons associated with an energy level/orbital combination beginning with a 3 need to be considered.  Since two energy level/orbital combinations begin with a 3, both orbitals are selected for further consideration:

3s²3p⁶

The superscripts associated with these orbitals total to 8.  Therefore, this new particle has 8 valence electrons and has achieved, through the process described above, a highly-stable octet configuration.

Note that the number of protons was unchanged in the process described above, so this new particle still contains 20 protons.  Since the identity of an element is defined by the number of protons that it contains, due to the association between atomic number and proton-count, this new particle is still a form of calcium.  However, since this new particle was formed through the loss of two valence electrons, it now only has 18 (20 – 2) electrons.  Therefore, this new particle is no longer a calcium (Ca) atom which, as stated above, contains 20 electrons. 

This particle is now an ion, a charged particle, as a result of the imbalance between the number of positively-charged protons (+20) and negatively-charged electrons (–18) that it possesses.  This particle now contains two more positively-charged protons than negatively-charged electrons; therefore, it bears an overall net +2 charge.  Additionally, since this ion is positively-charged, it is classified as a cation. 

The notation used to symbolize this particle must change, in order to distinguish an elemental symbol, which represents a neutral atom, from an ion symbol, which represents a charged particle.  An ion symbol incorporates the charge of the ion as a superscript on the corresponding elemental symbol.  As stated above, this ion is still a form of calcium (Ca).  However, because this particle is now an ion, its specific charge (+2) must be included as a superscript, and an ion symbol of "Ca⁺²" results.

Finally, this particle is referred to as a "calcium ion."  The word "ion" is now a necessary part of this particle's name, so that it can be distinguished from a calcium atom, which is often referred to simply as "calcium."

Finally, note that all metals will form cations, as indicated previously, because they will only be able to achieve octet configurations by losing their valence electrons.  The resultant ions will, therefore, always contain more positively-charged protons than negatively-charged electrons and will bear a net-positive overall charge.

Exercise \(\PageIndex{1}\)

Consider each of the following neutral elements.

1. Aluminum
2. Potassium

For each,

1. describe its ionization process,
2. determine the charge that will result upon its ionization,
3. provide the ion symbol for the resultant ion, and
4. provide the ion name for the resultant ion.

Answer a

An atom of aluminum contains 13 protons and 13 electrons, 3 of which can be classified as valence electrons, as determined either by using the electron configuration written below, or by recognizing that aluminum is located in Group 3A on the periodic table. 

1s²2s²2p⁶3s²3p¹

Therefore, aluminum would need to gain 5 electrons in order to achieve an octet configuration.  However, gaining more than three electrons is energetically-unfavorable and will not occur.  Recall that the valence electron count of all metals renders them unable to gain enough electrons to achieve an octet configuration.

Alternatively, an atom can lose its valence electrons, in order to achieve an octet configuration.  As aluminum has three valence electrons, losing all of them is possible, as doing so would meet, but not exceed, the maximum loss-limit of three electrons.  Therefore, aluminum will lose all three of its valence electrons.

If all of the valence electrons in the 3^rd energy level, which includes both the 3s and 3p orbitals, are lost, as shown below,

the following electron configuration results:

1s²2s²2p⁶

In this new electron configuration, the highest occupied energy level is no longer indicated by a 3.  Instead, the 2^nd energy level is now the valence shell.  Therefore, the number of valence electrons contained in this new particle are determined by totaling the electrons found within the the 2s and 2p orbitals.

2s²2p⁶

The superscripts associated with these orbitals total to 8.  Therefore, this new particle has 8 valence electrons and has achieved, through the process described above, a highly-stable octet configuration.

The number of protons was unchanged in the process described above, so this new particle still contains 13 protons.  Since the identity of an element is defined by the number of protons that it contains, this new particle is still a form of aluminum.  However, since this new particle was formed through the loss of three valence electrons, it now only has 10 (13 – 3) electrons.  Therefore, this new particle is no longer an aluminum (Al) atom which, as stated above, contains 13 electrons.

This particle is now an ion, a charged particle, as a result of the imbalance between the number of positively-charged protons (+13) and negatively-charged electrons (–10) that it possesses.  This particle now contains three more positively-charged protons than negatively-charged electrons; therefore, it bears an overall net +3 charge.  Since this ion is positively-charged, it is classified as a cation.

An ion symbol incorporates the charge of the ion as a superscript on the corresponding elemental symbol.  As stated above, this ion is still a form of aluminum (Al).  However, because this particle is now an ion, its specific charge (+3) must be included as a superscript, and an ion symbol of "Al⁺³" results. Finally, this particle is referred to as an "aluminum ion."  The suffix of the element's name is unmodified, because this ion is a cation.

Answer b

An atom of potassium contains 19 protons and 19 electrons, 1 of which can be classified as a valence electron, as determined either by using the electron configuration written below, or by recognizing that potassium is located in Group 1A on the periodic table. 

1s²2s²2p⁶3s²3p⁶4s¹

Therefore, potassium would need to gain 7 electrons in order to achieve an octet configuration, but gaining more than three electrons is energetically-unfavorable and will not occur.  Again, the valence electron count of all metals renders them unable to gain enough electrons to achieve an octet configuration.

Alternatively, an atom can lose its valence electrons, in order to achieve an octet configuration.  As potassium has only one valence electron, losing it is possible, as doing so would not exceed the maximum loss-limit of three electrons.  Therefore, potassium will lose its valence electron.

If the valence electron in the 4s orbital is lost, as shown below,

the following electron configuration results:

1s²2s²2p⁶3s²3p⁶

In this new electron configuration, the highest occupied energy level is no longer indicated by a 4.  Instead, the 3^rd energy level is now the valence shell.  Therefore, the number of valence electrons contained in this new particle are determined by totaling the electrons found within the the 3s and 3p orbitals.

3s²3p⁶

The superscripts associated with these orbitals total to 8.  Therefore, this new particle has 8 valence electrons and has achieved, through the process described above, a highly-stable octet configuration.

The number of protons was again unchanged in the process described above, so this new particle still contains 19 protons and is, therefore, still a form of potassium.  However, since this new particle was formed through the loss of a valence electron, it now only has 18 (19 – 1) electrons.

This particle is now an ion, as a result of the imbalance between the number of positively-charged protons (+19) and negatively-charged electrons (–18) that it possesses.  This particle now contains one more positively-charged proton than negatively-charged electrons; therefore, it bears an overall net +1 charge.  Since this ion is positively-charged, it is classified as a cation.

Because this particle is now an ion, its specific charge (+1) must be included as a superscript on the corresponding elemental symbol, and an ion symbol of "K⁺¹" results. Finally, this particle is referred to as a "potassium ion."  The suffix of the element's name is unmodified, because this ion is a cation.