Objectives
After completing this section, you should be able to
- account for the greater stability of the equatorial conformers of monosubstituted cyclohexanes compared to their axial counterparts, using the concept of 1,3‑diaxial interaction.
- compare the gauche interactions in butane with the 1,3‑diaxial interactions in the axial conformer of methylcyclohexane.
- arrange a given list of substituents in increasing or decreasing order of 1,3‑diaxial interactions.
Key Terms
Make certain that you can define, and use in context, the key term below.
Study Notes
1,3-Diaxial interactions are steric interactions between an axial substituent located on carbon atom 1 of a cyclohexane ring and the hydrogen atoms (or other substituents) located on carbon atoms 3 and 5.
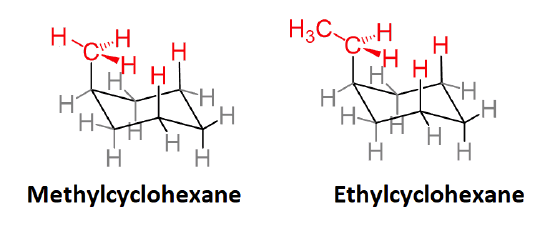
Be prepared to draw Newman-type projections for cyclohexane derivatives as the one shown for methylcyclohexane. Note that this is similar to the Newman projections from chapter 3 such as n-butane.

Newman projections of methylcyclohexane and n‑butane
When a substituent is added to a cyclohexane ring, the two possible chair conformations created during a ring flip are not equally stable. In the example of methylcyclohexane the conformation where the methyl group is in the equatorial position is more stable than the axial conformation by 7.6 kJ/mol at 25o C. The percentages of the two different conformations at equilibrium can be determined by solving the following equation for K (the equilibrium constant): ΔE = -RTlnK. In this equation ΔE is the energy difference between the two conformations, R is the gas constant (8.314 J/mol•K), T is the temperature in Kelvin, and K is the equilibrium constant for the ring flip conversion. Using this equation, we can calculate a K value of 21 which means about 95% methylcyclohexane molecules have the methyl group in the equatorial position at 25o C.

The energy difference between the two conformations comes from strain, called 1,3-diaxial interactions, created when the axial methyl group experiences steric crowding with the two axial hydrogens located on the same side of the cyclohexane ring. Because axial bonds are parallel to each other, substituents larger than hydrogen experience greater steric crowding when they are oriented axial rather than equatorial. Consequently, substituted cyclohexanes will preferentially adopt conformations in which the larger substituents are in the equatorial orientation. When the methyl group is in the equatorial position this strain is not present which makes the equatorial conformer more stable and favored in the ring flip equilibrium.

Actually, 1,3-diaxial steric strain is directly related to the steric strain created in the gauche conformer of butane discussed in Section: 3-7. When butane is in the gauche conformation 3.8 kJ/mol of strain was created due the steric crowding of two methyl group with a 60o dihedral angle. When looking at the a Newman projection of axial methylcyclohexane the methyl group is at a 60o dihedral angle with the ring carbon in the rear. This creates roughly the same amount of steric strain as the gauche conformer of butante. Given that there is actually two such interactions in axial methylcyclohexane, it makes sense that there is 2(3.8 kJ/mol) = 7.6 kJ/mol of steric strain in this conformation. The Newman projection of equatorial methylcyclohexane shows no such interactions and is therefore more stable.

Newman projections of methyl cyclohexane and butane showing similarity of 1,3-diaxial and gauche interactions.
Strain values for other cyclohexane substituents can also be considered. The relative steric hindrance experienced by different substituent groups oriented in an axial versus equatorial location on cyclohexane determined the amount of strain generated. The strain generated can be used to evaluate the relative tendency of substituents to exist in an equatorial or axial location. Looking at the energy values in this table, it is clear that as the size of the substituent increases, the 1,3-diaxial energy tends to increase, also. Note that it is the size and not the molecular weight of the group that is important. Table 4.7.1 summarizes some of these strain values values.
Table 4.7.1: A Selection of ΔG° Values for the Change from Axial to Equatorial Orientation of Substituents for Monosubstituted Cyclohexanes
| Substituent |
-ΔG° (kcal/mol) |
Substituent |
-ΔG° (kcal/mol) |
| \(\ce{CH_3\bond{-}}\) |
1.7 |
\(\ce{O_2N\bond{-}}\) |
1.1 |
| \(\ce{CH_2H_5\bond{-}}\) |
1.8 |
\(\ce{N#C\bond{-}}\) |
0.2 |
| \(\ce{(CH_3)_2CH\bond{-}}\) |
2.2 |
\(\ce{CH_3O\bond{-}}\) |
0.5 |
| \(\ce{(CH_3)_3C\bond{-}}\) |
\(\geq 5.0\) |
\(\ce{HO_2C\bond{-}}\) |
0.7 |
| \(\ce{F\bond{-}}\) |
0.3 |
\(\ce{H_2C=CH\bond{-}}\) |
1.3 |
| \(\ce{Cl\bond{-}}\) |
0.5 |
\(\ce{C_6H_5\bond{-}}\) |
3.0 |
| \(\ce{Br\bond{-}}\) |
0.5 |
|
|
| \(\ce{I\bond{-}}\) |
0.5 |
|
|
Exercises
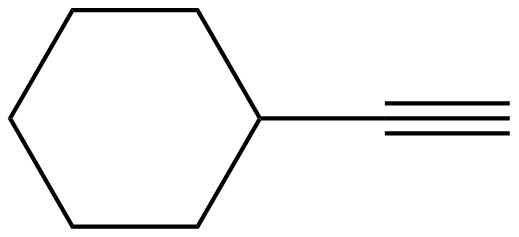
1) In the molecule, cyclohexyl ethyne there is little steric strain, why?
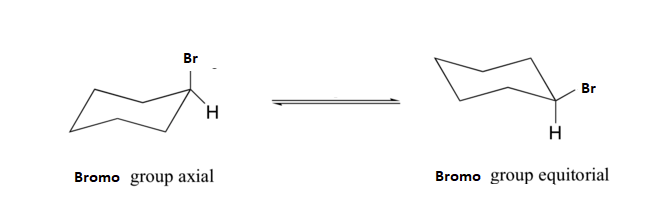
2) Calculate the energy difference between the axial and equatorial conformations of bromocyclohexane?
3) Using your answer from Question 2) estimate the percentages of axial and equatorial conformations of bromocyclohexane at 25o C.
4) There very little in 1,3-diaxial strain when going from a methyl substituent (3.8 kJ/mol) to an ethyl substituent (4.0 kJ/mol), why? It may help to use molecular model to answer this question.
Solutions
1) The ethyne group is linear and therefore does not affect the hydrogens in the 1,3 positions to say to the extent as a bulkier or a bent group (e.g. ethene group) would. This leads to less of a strain on the molecule.
![]()
2) The equatorial conformation of bromocyclohexane will have two 1,3 diaxial interactions. The table above states that each interaction accounts for 1.2 kJ/mol of strain. The total strain in equatorial bromocyclohexane will be 2(1.2 kJ/mol) = 2.4 kJ/mol.
3) Remembering that the axial conformation is higher in energy, the energy difference between the two conformations is ΔE = (E equatorial - E axial) = (0 - 2.4 kJ/mol) = -2.4 kJ/mol. After converting oC to Kelvin and kJ/mol to J/mol we can use the equation ΔE = -RT lnK to find that -ΔE/RT = lnK or (2.4 x 103 J/mol) / (8.313 kJ/mol K • 298 K) = lnK. From this we calculate that K = 2.6. Because the ring flip reaction is an equilibrium we can say that K = [Equatorial] / [Axial]. If assumption is made that [Equatorial] = X then [Axial] must be 1-X. Plugging these values into the equilibrium expression produces K = [X] / [1-X]. After plugging in the calculated value for K, X can be solved algebraically. 2.6 = [X] / [1-X] → 2.6 - 2.6X = X → 2.6 = 3.6X → 2.6/3.6 = X = 0.72. This means that bromocyclohexane is in the equatorial position 72% of the time and in the axial position 28% of the time.

4) The fact that C-C sigma bonds can freely rotate allows the ethyl subsistent to obtain a conformation which places the bulky CH3 group away from the cyclohexane ring. This forces the ethyl substituent to have only have 1,3- diaxial interactions between hydrogens, which only provides a slight difference to a methyl group.