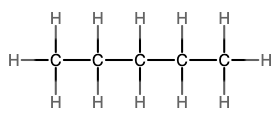Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

12.3: Alkanes

Last updated

Apr 13, 2023
Save as PDF
- 12.2: Hydrocarbons
- 12.4: Branched Alkanes

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

⚙️ Learning Objectives

Recognize straight-chain alkanes up to ten carbons in length.
Convert between molecular formulas, structural formulas, condensed structural formulas, and line formulas for straight-chain alkanes.

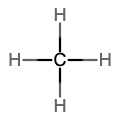
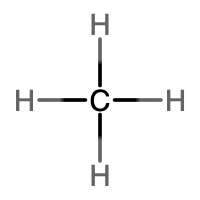
An alkane is a hydrocarbon in which there are only single covalent bonds. The simplest alkane is methane, with the molecular formula CH₄. The carbon is the central atom and makes four single bonds to hydrogen atoms. Organic compounds are often represented by a structural formula. The easiest way to think of a structural formula is to think of it as a Lewis structure minus the lone pairs. The structural formula shows the layout of all of the atoms and bonds in a molecule.

Figure

$\PageIndex{1}$ : Methane is the simplest hydrocarbon and is shown using a structural formula, a ball-and-stick model, and a space-filling model.

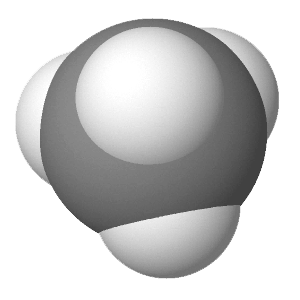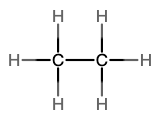
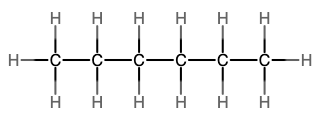
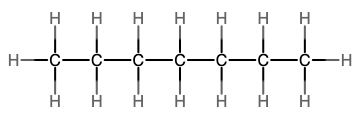
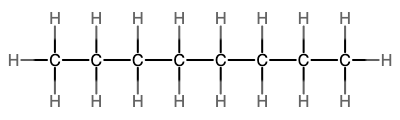
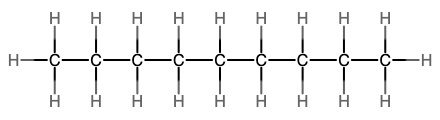
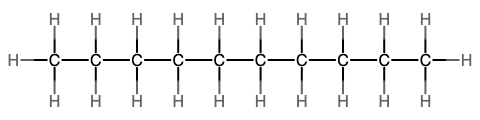
The next simplest alkane is called ethane, C₂H₆, and consists of two carbon atoms with a single covalent bond between them. Each carbon is then able to bond to three hydrogen atoms. The alkane series progresses from there (see Table $\PageIndex{1}$ ), increasing the length of the carbon chain by one carbon at a time. These alkanes are called straight-chain alkanes because the carbon atoms are connected in one continuous chain with no branches. Naming and writing structural and molecular formulas for the straight-chain alkanes is straightforward. The name of each alkane consists of a prefix that specifies the number of carbon atoms and has a suffix of -ane.

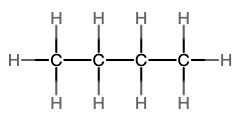
Notice that with the addition of each carbon atom, two additional hydrogen atoms added to the molecule. This pattern may be reflected by writing a general formula for the alkanes as C_nH_2n₊₂ where n represents the number of carbon atoms in the chain. This table also shows that the boiling points of the alkanes steadily increase as the length of the carbon chain increases. This is due to an increase in the strength of the intermolecular attractive forces and is a general feature of other organic molecules as well.

Table $\PageIndex{1}$ : First Ten Members of the Alkane Series
Name	Molecular Formula	Boiling Point (°C)
methane	CH₄	-161
ethane	C₂H₆	-89
propane	C₃H₈	-42
butane	C₄H₁₀	0.5
pentane	C₅H₁₂	36
hexane	C₆H₁₄	69
heptane	C₇H₁₆	99
octane	C₈H₁₈	126
nonane	C₉H₂₀	151
decane	C₁₀H₂₂	174

Simplifying Structural Formulas

The structural formulas in Table $\PageIndex{1}$ take up more space and show an increasing amount repetition as the length of the chain of carbon atoms grows. Chemists often use condensed structural formulas to abbreviate the structures of organic molecules. A condensed structural formula has the appearance of a structure from which most or all of the bond symbols have been removed. Covalent bonds are understood to exist between each carbon atom and their associated hydrogen atoms, as well as between carbon atoms.

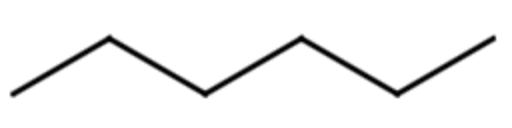
Line formulas are also used to abbreviate the structures of organic molecules. In a line formula, carbon atoms are represented by each end of a line or bend in a line. Hydrogen atoms are not drawn if they are attached to a carbon. Other atoms besides carbon and hydrogen are represented by their elemental symbols.

Table $\PageIndex{2}$ shows the ball-and-stick models and space-filling models of propane and hexane followed by three different ways to draw each structure. Most of the organic molecules drawn in this text will use condensed structural formulas or line formulas.

Table $\PageIndex{2}$ : Models and Formulas of Propane and Hexane
	Propane	Hexane
Ball-and-Stick Model
Space-Filling Model
Structural Formula
Condensed Structural Formula	CH₃CH₂CH₃	CH₃CH₂CH₂CH₂CH₂CH₃ or CH₃(CH₂)₄CH₃
Line Formula

✅ Example $\PageIndex{1}$ :

Consider a straight-chain alkane that has 14 carbon atoms.

What is its molecular formula?
Write the condensed structural formula for this alkane.

Solution

The general formula for an alkane is C_nH_2n₊₂. An alkane that has 14 C atoms has 2(14) + 2 = 30 H atoms. The chemical formula is C₁₄H₃₀.
C atoms on each end are bonded to three H atoms, since they are only bonded to one other C atom. C atoms that are in between are all bonded to two other C atoms, so they only have room for two H atoms each. Therefore, the condensed structural formula is CH₃CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₃ or CH₃(CH₂)₁₂CH₃.

A quick check confirms that the condensed structural formula has 14 C atoms and 30 H atoms.

✅ Example $\PageIndex{2}$ : Writing Chemical Formulas

Give the molecular formula for the molecule represented with the line formula:

Line structure of an alkane with 12 bumps (10 interior bumps and two ends)

Solution

Each end or bend represents a carbon atom. There are 12 ends or bends, so this molecule has 12 C atoms. Alkanes have a general formula of C_nH_2n₊₂, meaning there are 2(12) + 2 = 26 H atoms. This yields a molecular formula of C₁₂H₂₆.

✏️ Exercise $\PageIndex{1}$

Consider a straight-chain alkane that has 11 carbon atoms.

What is its molecular formula?
Write the condensed structural formula for this alkane.
Write the line formula for this alkane.

Answer A: C₁₁H₂₄

Answer B: CH₃CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₂CH₃ or CH₃(CH₂)₉CH₃

Answer C

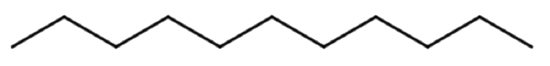
Summary

An alkane is a hydrocarbon in which there are only single covalent bonds.
Alkanes have a general formula of C_nH_2n₊₂.
Condensed structural formulas and line formulas are often used to simplify the structures of organic compounds.

This page is shared under a CK-12 license and was authored, remixed, and/or curated by Lance S. Lund (Anoka-Ramsey Community College). Original source: https://www.ck12.org/c/chemistry/.

LICENSED UNDER

Simplifying Structural Formulas

Summary

Support Center

How can we help?