22.2: Metallic Bonding

Most metals have very compact crystal structures involving either the body-centered cubic, face-centered cubic, or hexagonal closest-packed lattices. Thus every atom in a metal is usually surrounded by 8 or 12 equivalent nearest neighbors. How can each atom be bonded to so many of its fellow atoms? Although there are plenty of electropositive atoms to donate electrons, there are no electronegative atoms to receive them, and so ionic bonding seems unlikely. Ordinary covalent bonding can also be ruled out. Each covalent bond would require one electron from each atom, and no metal has 12 valence electrons.

A valuable clue to the nature of bonding in metals is provided by their ability to conduct electricity. Electrons can be fed into one end of a metal wire and removed from the other end without causing any obvious change in the physical and chemical properties of the metal. To account for this freedom of movement modern theories of metallic bonding assume that the valence electrons are completely delocalized; that is, they occupy molecular orbitals belonging to the metallic crystal as a whole. These delocalized electrons are often referred to as an electron gas or an electron sea. Positive metal ions produced by the loss of these valence electrons can then be thought of as “floating” in this three-dimensional sea. Each ion is held in place by the attraction of the negatively charged electron sea and the repulsion of its fellow positive ions.

In order to see how MO theory can be applied to metals, let us first consider the simplest case, lithium. If two lithium atoms are brought together, the 1s core electrons remain essentially unchanged since there is virtually no overlap between them. The 2s orbitals, by contrast, overlap extensively and produce both a bonding and an antibonding orbital. Only the bonding orbital will actually be occupied by the two electrons, as shown in Figure 1. Somewhat higher than these two orbitals are a group of six unoccupied orbitals produced by the overlap of six 2p atomic orbitals (three on each atom). Suppose now we add a third atom to the two already considered so that we form a triangular molecule of formula Li₃. As shown in the figure, the overlap of three 2s orbitals produces a lower group of three orbitals, while the overlap of three times three 2p orbitals produces a higher group of nine orbitals. Again the total number of molecular orbitals is equal to the number of atomic orbitals from which they are derived.

Continuing to add lithium atoms in this fashion, we soon attain a cluster of 25 lithium atoms. The energy-level situation for a cluster this size is a lower group of 25 MO’s, all deriving from 2s atomic orbitals, and a higher group of 75 MO’s, all deriving from 2p atomic orbitals. Note how closely spaced these energy levels have become. This is in line with the tendency for the energy levels to get closer the greater the degree of delocalization.

Finally, if we add enough lithium atoms to our cluster to make a visible, weigh-able sample of lithium, say 10²⁰ atoms, the energy spacing between the molecular orbitals becomes so small it is impossible to indicate in the figure or even to measure. In effect an electron jumping among these levels can have any energy within a broad band from the lowest to highest. In consequence this view of electronic structure in solids is often referred to as the band theory of solids.

Figure \(\PageIndex{1}\): Molecular-orbital energies corresponding to delocalization of valence electrons over increasing numbers of Li atoms. A 1-mg sample of Li would contain nearly 10²⁰ atoms. The corresponding orbital energies are so closely spaced that they constitute essentially continuous bands.

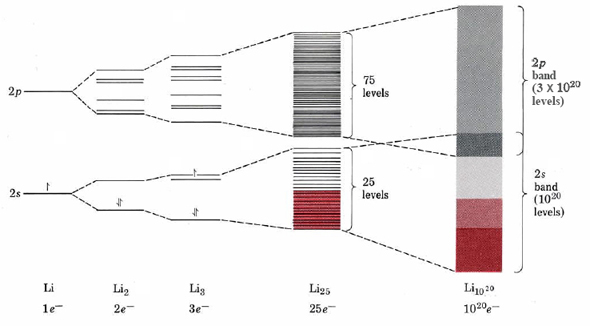
It should also be clear from Figure \(\PageIndex{1}\) that all the available molecular orbitals need not be completely filled with electrons. In the case of lithium, for example, a sample containing 10²⁰ atoms would have 10²⁰ valence electrons. Since each atom would have a single 2s orbital as well as three 2p orbitals, there would be 1 × 10²⁰ MO’s in the 2s band and 3 × 10²⁰ MO’s in the 2p band. If all electrons were paired, only the 0.5 × 10²⁰ MO’s of lowest energy in the 2s band would be required to hold them. Note that there is a nice correspondence between the half-filled 2s band of the macroscopic sample and the half-filled 2s orbital of an individual Li atom.

According to the band theory, it is this partial filling which accounts for the high electrical and thermal conductance of metals. If an electric field is applied to a metallic conductor, some electrons can be forced into one end, occupying slightly higher energy levels than those already there. As a consequence of delocalization this increased electronic energy is available throughout the metal. It therefore can result in an almost instantaneous flow of electrons from the other end of the conductor.

A similar argument applies to the transfer of thermal energy. Heating a small region in a solid amounts to increasing the energy of motion of atomic nuclei and electrons in that region. Since the nuclei occupy specific lattice positions, conduction of heat requires that energy be transferred among nearest neighbors. Thus when the edge of a solid is heated, atoms in that region vibrate more extensively about their average lattice positions.

They also induce their neighbors to vibrate, eventually transferring heat to the interior of the sample. This process can be speeded up enormously if some of the added energy raises electrons to higher energy MO’s within an incompletely filled band. Electron delocalization permits rapid transfer of this energy to other atomic nuclei, some of which may be quite far from the original source.

When an energy band is completely filled with electrons, the mechanism just described for electrical and thermal conduction can no longer operate. In such a case we obtain a solid which is a very poor conductor of electricity, or an insulator. At first glance we might expect Be, Mg, and other alkaline earths to be insulators like this. Since atoms of these elements all contain filled 2s subshells, we would anticipate a filled 2s band in the solid for all of them. That this is not the case is due to the relatively small energy difference between the 2s and 2p levels in these atoms. As you can see from Figure \(\PageIndex{1}\), this small separation results in an overlap between the 2s and 2p bands. Thus electrons can move easily from the one band to the other and provide a mechanism for conduction.

Figure \(\PageIndex{2}\): Band structures of conductors, semiconductors, and insulators. Note that conductors may have a partially filled band or a filled band which overlaps an empty one. In semiconductors and insulators, band separation becomes progressively larger containing electrons are color-coded.)

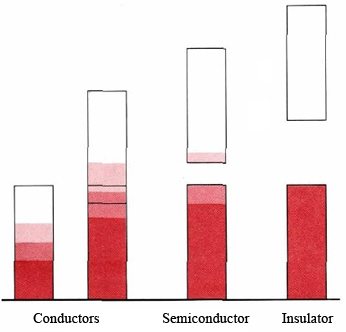
Figure \(\PageIndex{2}\) shows four different possibilities for band structure in a solid. For a solid to be a conductor, a band must be either partially filled or must overlap a higher unfilled band. When there is a very large energy gap between bands and the lower band is filled, we have an insulator. If the gap is quite small, we get an intermediate situation and the solid is a semiconductor. All the semimetals found along the stairstep diagonal in the periodic table, notably germanium, have a band structure of this type.

In a semiconductor we find that collisions among atoms and electrons in the crystal are occasionally energetic enough to excite an electron into the top band. As a result there are always a small number of electrons in this band and an equal number of holes (orbitals from which electrons have been removed) in the lower-energy band. The excited electrons can carry electrical current because many different energy levels are available to them. So can the holes—other electrons from the nearly filled band can move up or down into them, a process which decreases or increases the energy of the hole.

In a metal, electrical conductivity decreases as the temperature is raised because the nuclei vibrate farther from their rest positions and therefore get in the way of moving valence electrons more often. Exactly the opposite behavior is found for semiconductors. With increasing temperature, more and more electrons are excited to the higher-energy conduction band so that more current can be carried. Excitation of electrons to the conduction band can also be accomplished by a photon, a phenomenon known as photoconduction. Selenium metal is often used in this way as a photocell in light meters and “electric eyes.”

The electron-sea model of metals not only explains their electrical properties but their malleability and ductility as well. When one layer of ions in an electron sea moves along one space with respect to the layer below it, a process we can represent pictorially as:

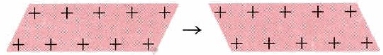
The final situation is much the same as the initial. Thus if we hit a metal with a hammer, the crystals do not shatter, but merely change their shape, This is very different from the behavior of ionic crystals.

The electron-sea model also enables us to explain, at least partially, why the metallic bond is noticeably stronger for some metals than others. While the alkali metals and some of the alkaline-earth metals can be cut with a knife, metals like tungsten are hard enough to scratch the knife itself. A good indication of how the strength of the metallic bonding varies with position in the periodic table is given by the melting point.

Figure \(\PageIndex{3}\): Melting points of the metals of the fourth, fifth, and sixth periods

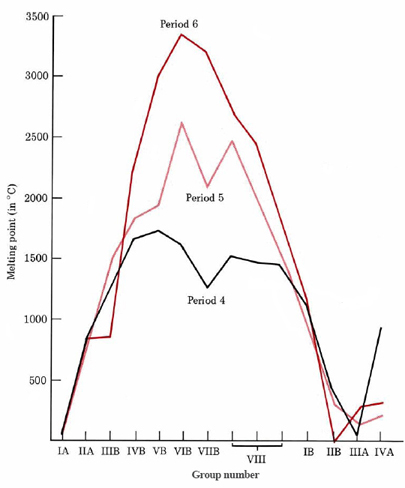
As can be seen from Figure \(\PageIndex{3}\), if the melting point of the metals is plotted against the group number for the three long periods, there is a sharp increase from group IA to group VB or VIB, after which there is a leveling off. Finally the melting point again drops to quite low values. A similar behavior is found for other properties such as boiling point, enthalpy of fusion, density, and hardness.

The initial increases in the strength of metallic bonding as we move from group IA to VIB can be explained in terms of the number of valence electrons the metal is capable of contributing to the electron sea. The more electrons an atom loses, the larger will be the charge of the positive ion embedded in the electron sea and the greater will be the electron probability density of the electron sea itself. Thus the more electrons which are lost, the more tightly the ions will be held together. Chromium with six valence electrons is thus much harder than sodium with one.

This trend cannot continue indefinitely, however. The more electrons that are removed from an atom, the more energy it takes to remove the next electron. Eventually we find that more energy is needed to remove an electron from a metal nucleus than is liberated by placing it in the electron sea. The strength of the bonding thus begins to level off and eventually to drop. It should be pointed out that metallic bonding strength is not solely dependent on the number of valence electrons (or the periodic group number) of an element. Other factors such as atomic radius and type of crystal lattice are also important. Nevertheless it is useful to remember that melting points and other properties related to metallic bond strength reach their maximum at about the middle of each transition series.