Even and Odd Functions (Worksheet)
- Page ID
- 39618
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Name: ______________________________
Section: _____________________________
Student ID#:__________________________
Work in groups on these problems. You should try to answer the questions without referring to your textbook. If you get stuck, try asking another group for help.
Symmetry in 1-D
In mathematics, even functions and odd functions are functions which satisfy particular symmetry relations. A function \(f\) is even if the graph of \(f\) is symmetric with respect to the y-axis. Algebraically, \(f\) is even if and only if \(f(-x) = f(x)\) for all \(x\) in the domain of \(f\). A function \(f\) is odd if the graph of \(f\) is symmetric with respect to the origin. Sometimes the form of a function helps us to solve problems. This is particularly true for even or odd functions.
Q1
An even function is one where \(f(x)=f(-x)\). An odd function is one where \(f(x)=-f(-x)\).
The function, \(f(x)=x^2\) is shown below.
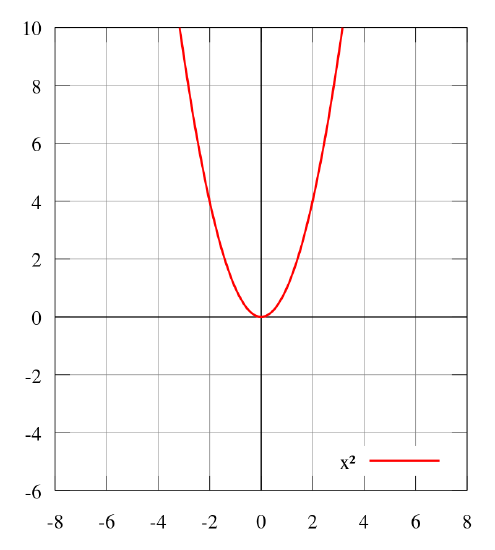
Is \(f(x)\) even or odd?
Describe the symmetry of the plot of the even functions in words?
Q2
\(f(x)=x^3\) is shown below.
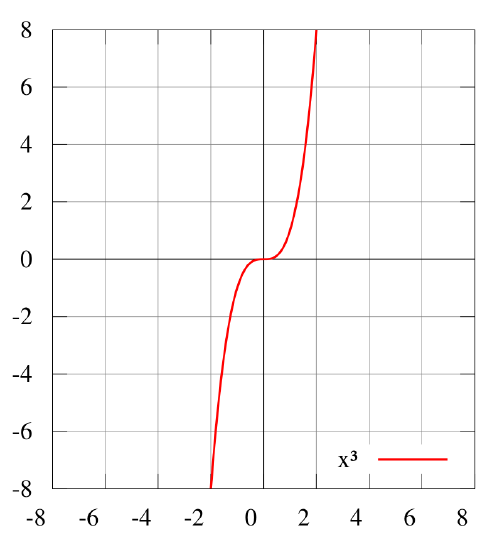
Is \(f(x)\) even or odd?
Describe the symmetry of the plot of the odd function.
Q3
Draw a sketch of \(f(x)=e^{-x^2/2a^2}\) on the axes below.
Is \(f(x)=e^{-x^2/2a^2}\) even or odd?
If \(\displaystyle \int_{0}^{\infty}e^{-ax^2}=(\frac{\pi}{4a})^{1/2}\), what is \(\displaystyle \int_{-\infty}^{\infty}f(x)dx\)? Use your sketch of \(f(x)=e^{-x^2/2a^2}\) to help you find the answer.
Q4
What fundamental property of \(f(x)=e^{-x^2/2a^2}\) did you consider to evaluate the integral \(\int_{-\infty}^{\infty}f(x)dx\)?
From the integral of \(f(x)=e^{-x^2/2a^2}\) over an even interval, e.g., \(-\infty\) to \(\infty\), what can you conclude about the integral of an even function over an even interval compared to the integral of the same function over one half that interval, e.g., \(-\infty\) to 0 or 0 to \(\infty\)?
From the plot of \(f(x)=x^3\) shown above, what is the value of \(\displaystyle \int_{-\infty}^{\infty}f(x)dx\)? Why?
Extrapolating from this function, what can you say about the integral of an odd function over an even interval, such as from \(-\infty\) to \(\infty\)?
For \(f(x)=x\) is \(f(x)\) even or odd?
Draw a sketch of \(f(x)\) on the axes below.

Q5
If \(f(x)=xe^{-x^2/2a^2}\), is \(f(x)\) even or odd?
Sketch \(f(x)\) on the axes below.

What does this tell you about the product of an even and an odd function with each other?
Is the product of two even functions even or odd?
Is the product of two odd functions even or odd (do an example to convince yourself)?
Q6
The eigenfunctions for the lowest for levels of the harmonic oscillator \((v=1-7)\) are below.
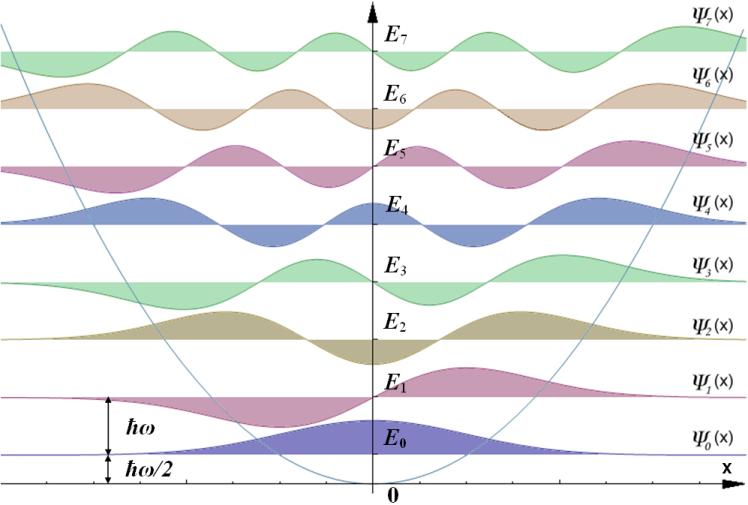
Which levels are even functions?
Which are odd functions?
Q7
The probability densities for the lowest four levels of the harmonic oscillator \((v=1-7\)) are below.
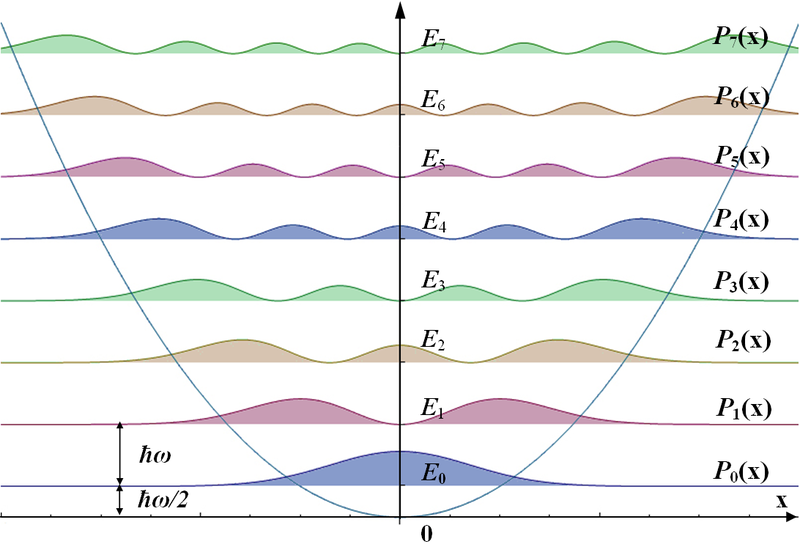
Which levels are even functions?
Which levels are odd functions?
How does this compare to your earlier statements about products of functions?
Consider the graphs of the harmonic oscillator probability densities. Without calculating an integral, what is \(\langle x\rangle\)?
How do you explain your answer in terms of the probability density graphs?
How do you explain your answer in terms of the even/odd character of the probability densities?


