Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

Home
Learning Objects
Worksheets
Worksheets: Physical Chemistry
Harmonic Oscillator and IR Absorption of Diatomics (Worksheet)

Harmonic Oscillator and IR Absorption of Diatomics (Worksheet)

Last updated

Jun 11, 2021
Save as PDF
- Particle in a Box Probability (Worksheet)
- Rigid Rotor (Worksheet)

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Name: ______________________________

Section: _____________________________

Student ID#:__________________________

Work in groups on these problems. You should try to answer the questions without referring to your textbook. If you get stuck, try asking another group for help.

The Quantum Harmonic Oscillator and Molecular Vibrations

At the turn of the nineteenth century Sir William Herschel discovered invisible radiation beyond the red end of the visible region of the electromagnetic spectrum. This radiation appropriately is called infrared, meaning “beneath the red,” and it encompasses the wavelength region from $10^3 \: \text{nm}$ to $10^6 \: \text{nm}$ . Infrared spectroscopy has become the most widely used spectroscopic technique for investigating organic structures. Absorption of infrared radiation causes transitions between vibrational eigenstates of a molecule. A simple diatomic molecule, such as $\ce{H-Cl}$ , has only one vibrational mode available to it, a stretching vibration somewhat like balls on the ends of a spring:

alt

Table $\PageIndex{1}$ gives data about the fundamental vibrational frequencies, force constants and bond lengths for some diatomic molecules.

Table

$\PageIndex{1}$ : Fundamental Vibrational Frequencies of Select Diatomics

Molecule	frequency/cm $^{-1}$	force constant/(N/m)	bond length/(pm)	molecular mass $(g/mol$ )
$H_2$	4401	510	74.1	2
$D_2$	2990	527	74.1	4
$H^{35}$ Cl	2886	478	127.5	36
$H^{79}$ Br	2630	408	141.5	80
$H^{127}$ I	2230	291	160.9	128
$^{35}Cl^{35}Cl$	554	319	198.8	70
$^{79}$ Br $^{79}$ Br	323	240	228.4	158
$^{127}I^{127}l$	213	170	266.7	254
$^{16}O^{16}O$	1556	1142	120.7	32
$^{12}C^{16}O$	2143	1857	112.8	28
$^{14}$ N $^{16}$ O	1876	1550	115.1	30

Use the data in Table $\PageIndex{1}$ to answer and justify your answers for the questions below. These are phenomenological conclusions that will motivate the comparison to the quantum harmonic oscillator results. Your answers should express the relationship explicitly (e.g., linearly proportional).

Q1

How does the vibrational frequency depend on the force constant of the molecule's bond?

Q2

How does the vibrational frequency depend on the bond length of the molecules?

Q3

How does the vibrational frequency depend on total mass of the molecules?

Q4

The reduced mass $\mu_{AB}$ for a diatomic is given by

$\mu_{12}=\dfrac{m_1\, m_2}{m_1+m_2} \label{Reduced}$ where

$m_1$ and

$m_2$ are the respective masses of atoms 1 and 2, respectively. How does the vibrational frequency depend on the reduced mass of the molecules?

Q5

Why is the vibrational frequency lower for $\ce{D_2}$ than it is for $\ce{H_2}$ ?

Quantum Mechanics Harmonic Oscillator

The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can usually be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics. Furthermore, it is one of the few quantum-mechanical systems for which an exact, analytical solution is known. The quantum harmonic oscillator is a good starting point for describing molecular vibrations.

The absorption of IR radiation by a molecule can be likened to two atoms attached to each other by a massless spring. Considering simple diatomic molecules, only one vibration is possible. The Hook's law potential on the other hand is based on an ideal spring

$V(r) = \dfrac{1}{2} k(r-r_{eq}) \label{3}$

Solving the Schrödinger equation for the harmonic oscillator potential results in the energy levels

$E_m = \left(v+\dfrac{1}{2}\right)h\nu_m \label{4}$

where $v$ is the relevant quantum number and range from $0$ to $\infty$ and $m$ is the vibration of interest (only one vibration in diatomics). $\nu_m$ is the natural frequency of the mechanical oscillator

$\nu_m = \dfrac{1}{2\pi} \sqrt{\dfrac{k}{m}} \label{13}$

This depends on the force constant of the spring and the mass of the attached body and independent of energy imparted on the system. For diatomic vibrations, i.e., when there are two masses involved in the system, then the mass used in Equation $\ref{13}$ is the reduced mass $\ref{Reduced}$ introduced above:

$\nu_m = \dfrac{1}{2\pi} \sqrt{\dfrac{k}{\mu_{12}}} \label{14}$

Q5

The molecular masses for $\ce{H^{79}Br}$ is higher than that of $\ce{^{35}Cl^{35}Cl}$ . Why is the vibrational frequency lower for the lighter molecule?

Q6

How does mass of the atoms comprising the molecule affect the vibrational frequency?

Q7

Speculate and predict vibrational frequencies (in cm^-1) for the molecules below:

$D^{35}Cl$
$H^{37}Cl$
$D^{37}Cl$
$^{35}Cl^{35}Cl$

Q8

What assumptions have you made to predict the frequencies?

Q9

What factors do you think are most important in determining molecular vibrational frequency?

Connecting the Quantum Harmonic Oscillator to Infrared Spectroscopy

Transitions between vibrational energy levels can be induced about by absorption or emission of radiation. To understand this, knowledge of both the initial and final eigenstates is is needed. Transitions in vibrational energy levels can be brought about by absorption of radiation, provided the energy of the radiation exactly matches the difference in energy levels between the vibrational quantum states and provided the vibration causes a change in dipole moment. This can be expressed as

${\Delta E} = E_{final}-E_{initial} = h \nu_m = \dfrac{h}{2\pi} \sqrt{\dfrac{k}{\mu}} \label{5.5.18}$

The majority of molecules are in the ground state $v = 0$ at room temperature, so let's only consider this as the initial eigenstate, then from Equation $\ref{13}$

$E_{initial} = \dfrac{1}{2}hv_m \label{5.5.19}$

when a molecule absorbs energy to be promoted to the first excited state ( $v=1$ ) then

$E_{final} = \dfrac{3}{2} hv_m \label{5.5.20}$

and $\Delta E$ is then

$\left(\dfrac{3}{2} hv_m - \dfrac{1}{2} hv_m \right) = hv_m \label{5.5.21}$

The frequency of radiation $\nu$ that will bring about this change is identical to the classical vibrational frequency of the bond $v_m$ and can be expressed as

$E_{radiation} = hv = {\triangle E} = hv_m = \dfrac{h}{2\pi} \sqrt{\dfrac{k}{\mu}} \label{5.5.22}$

The cm^-1 is the wavenumber scale and it can also be defined as 1/wavelength in cm. A linear wavenumber is often used due to its direct relationship with both frequency and energy. The frequency of the absorbed radiation causes the molecular vibrational frequency for the absorption process:

$\underbrace{\widetilde{\nu}}_{\text{in units of cm}^{-1}} = \dfrac{1}{\lambda(\mu m)} \times 10^4 \left(\dfrac{\mu m}{cm}\right) = \dfrac{v(Hz)}{c(cm/s)} \label{5.5.28}$

Equation $\ref{5.5.22}$ can be modified so that the radiation can be expressed in wavenumbers

$\widetilde{\nu} = \dfrac{1}{2\pi c} \sqrt{\dfrac{k}{\mu}} \label{5.5.23}$

where

$c$ is the velocity of light (cm s^-1) and
$\widetilde{\nu}$ is the wave number of an absorption maximum (cm^-1)

Q10

For the following harmonic oscillator potential energy curve, draw the first four energy levels of corresponding eigenstates. Hint: you can use any value of $nu_m$ you like, but make sure you indicate the x- and y-axis units. The equilibrium length is set to zero for convenience.

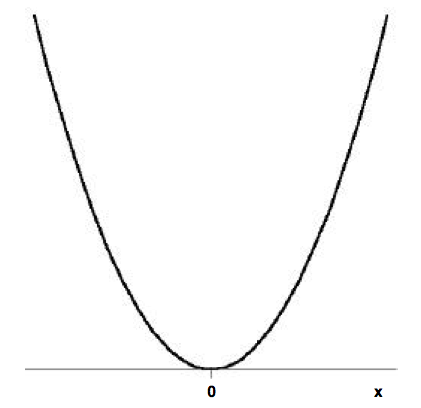
Screen_shot_2014-02-19_at_8.26.05_PM.png

Q11

Draw the transition associated with the $v=0$ to $v=1$ and what is the energy of this transition (again, this depends on the $nu_m$ you used)?

Q12

The frequency of the transition draw in Q11 is the "fundamental frequency" of the vibration and that resolved in IR spectroscopy (which directly correlates to the frequencies in Table $\PageIndex{1}$ . Draw the "first overtone" that is associated with the $v=0$ to $v=2$ transition on the potential above. What is the energy of this transition?

Q13

While one may envision a infinite number of possible transitions from harmonic oscillator (e.g., fundamental and overtone), there are "selection rules" that limit which ones you can actually observe. For example, the first overtone (or any other overtone) cannot be observed in IR spectroscopy. Another example is that for a vibration to be observed in IR spectroscopy, the vibration MUST results in a changing dipole moment. If not, then the vibration is "IR inactive", but it doesn't mean it does not exist. Which of the molecules in Table $\PageIndex{1}$ have vibrations that are IR inactive?

Template:HideTOC

The Quantum Harmonic Oscillator and Molecular Vibrations

Q1

Q2

Q3

Q4

Q5

Quantum Mechanics Harmonic Oscillator

Q5

Q6

Q7

Q8

Q9

Connecting the Quantum Harmonic Oscillator to Infrared Spectroscopy

Q10

Q11

Q12

Q13

Support Center

How can we help?