12.15: Polyenes: Delocalization in Polyenes
- Page ID
- 140078
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Bonding in ozone can be explained with conjugation. In conjugation, several p orbitals can interact with each other to create a delocalized pi bond.
Conjugation can also occur in polyunsaturated alkenes or olefins. Alkenes are compounds that contain carbon-carbon double bonds. A carbon-carbon double bond is sometimes described as unsaturated. This term distinguishes alkenes from alkanes or paraffins, which have only carbon-carbon single bonds. Alkanes can be made from alkenes through the addition of hydrogen gas in the presence of a transition metal catalyst. Whereas unsaturated alkenes can take up additional hydrogens to add to double-bonded carbons, alkanes are already full: they are saturated with hydrogen and can accept no more.
The conversion of unsaturated carbon chains into saturated ones is very common. A familiar example concerns cooking fats and oils. Many vegetable oils contain unsaturated fatty acids, which contain long chains of carbon atoms having at least one carbon-carbon double bond. These double bonds produce a kink in the carbon chain, making these compounds oils at room temperature. When dairy products were rationed during World War II, vegetable oils were converted into saturated fats through catalytic hydrogenation. Saturated fats have a regularly repeating, zig-zag shape in their carbon chains and they are solids at room temperature. The product of this conversion was called oleo, or later, margarine. Margarine remains a common substitute for butter today.
Problem MO15.1.
Why does a kink in the carbon chain make a compound an (liquid) oil, when otherwise it would be a (solid) fat or wax?
- Polyunsaturated alkenes, or polyenes, are compounds that have several carbon-carbon double bonds.
- The double bonds can be spaced out along the carbon chain, with several carbon-carbon single bonds separating them. In this case they are called isolated polyenes.
- Two double bonds could be attached to the same carbon, so that there are two double bonds in a row, but this situation is rare. This compound would be called a cumulene.
- Two double bonds could be separated by one single bond. Alternatively, a number of double bonds could be separated by single bonds, so that a carbon chain had alternating double and single bonds. This compound is a conjugated polyene.
Conjugated polyenes have a few unusual properties.
- First of all, they are slightly more difficult to hydrogenate than are other polyenes. Their double bonds somehow resist taking up hydrogen.
- Second, these compounds have "high rotational barriers" around their carbon-carbon single bonds.
Normally, single bonds between atoms are not difficult to rotate, and the atoms on one end of a bond can spin with respect to the other end. Double bonds, on the other hand, do not rotate easily, because the p orbitals forming the pi bond need to remain parallel to each other in order to bond. The single bonds in conjugated polyenes do not rotate freely; they behave a little more like double bonds.
- Finally, conjugated polyenes show a pronounced "red shift" in their ultraviolet-visible spectra.
When alkenes absorb ultraviolet light, an electron is promoted to a higher energy level. In alkenes, one of the electronic transitions that can occur involves the promotion of an electron from a p to a p * level. The frequency necessary to induce this electronic transition is usually in the ultraviolet range. However, increasing the number of conjugated double bonds moves this transition to lower and lower frequency, as shown in the table below. Some molecules, such as carotene, have many conjugated double bonds, and absrob visible light. Carotene absorbs blue and green light, reflecting red and orange. It is one of the pigments responsible for the orange and red colour of leaves in the fall.
|
Compound |
Absorption wavelength (nm) |
|
CH2=CH2 |
180 |
|
CH2=CH-CH=CH2 |
210 |
|
CH2=CH-CH=CH-CH=CH2 |
240 |
All of these properties of polyenes can be explained using quantitative molecular orbital calculations. We can also get an idea of where some of the results come from by taking a very approximate qualitative MO approach.
Start with the first entry in the table above.
- Ethene, CH2=CH2, contains two trigonal carbons.
- As an approximation, we will say that these two planar carbons each use a set of sp2 orbitals to bond to the hydrogens and each other.
- That leaves a leftover p orbital on each carbon to form a pi bond.
We aren't going to bother drawing a complete molecular orbital energy level diagram for ethene. We will only draw a Huckel diagram, which shows only the portion of the molecule involved in pi bonding.
- In the Huckel diagram, there is a bonding combination and an antibonding combination.
- In the absence of a complete calculation, we will guess from the Lewis structure and say that there is one pair of electrons to populate this set of orbital.
- They will go into the lower, bonding orbital.
- These electrons are delocalized over two carbons, with a half wavelength stretching over both carbons. A full wavelength would stretch to four carbons long.
For comparison, we can look at the next entry in the table. It is butadiene, CH2=CH-CH=CH2.
- As an approximation, we will say that these four planar carbons each use a set of sp2 orbitals to bond to the hydrogens and each other.
- That leaves a leftover p orbital on each carbon to form a pi bond.
- In the Huckel diagram, there is a fully bonding combination (all in phase with their neighbours) and a fully antibonding combination (all out of phase with their neighbours, with three nodes).
There must be two other combinations, because we started with four p orbitals.
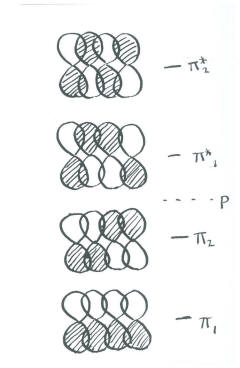
- The two pairs of orbitals on each end could be in phase, but out of phase in the middle. This combination contains one node.
- The opposite could be true: The two in the middle could be in phase, but out of phase with both ends. This final combination contains two nodes.
Notice that the number of nodes increases one by one, from the all-bonding combination to the all-antibonding.
- We will guess from the Lewis structure and say that there are two pairs of electrons to populate this set of orbitals.
- They will go into the lower two orbitals.
- The lowest energy electrons are delocalized over four carbons. A full wavelength would stretch to eight carbons long.
This pair of low-energy electrons must be at least partly responsible for the stability of polyenes. These electrons are much lower in energy than they would be in two separate double bonds, which would be similar in energy to the double bonds in ethene.
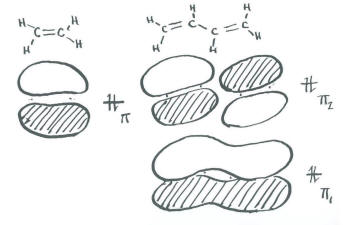
In addition, this orbital explains the difficulty of rotating around the single bonds in butadiene. In a sense, there are no single bonds in butadiene. There are only double bonds.
The spectroscopy results require more attention to subtleties. Note that the next pair of electrons goes into an orbital that contains a node. In a quantitative MO calculation, the presence of this antibonding interaction in an otherwise bonding orbital raises its energy slightly above the energy of the pi bond in ethene. Also, the lowest lying empty orbital has a bonding interaction, lowering its energy slightly below that of the purely antibonding orbital in ethene. The energy gap between the p to a p * levels is slightly smaller in butadiene, requiring a lower-energy photon to promote an electron.
Problem MO15.2.
Construct a Huckel MO diagram for 1,3,5-hexatriene, CH2=CH-CH=CH-CH=CH2.
Problem MO15.3.
Another possible UV-visible absorption leads to n to p * transition in oxygen-containing compounds; the n refers to a nonbonding electron in a lone pair on oxygen. Construct Huckel MO diagrams for CH3-CH2-CHO (the last C is bonded to an H and also to an oxygen, as well as the next carbon) and CH2=CH-CHO. Include the oxygen nonbonding energy levels in your diagrams. Compare the n to p * energy gaps in these two compounds. How do you expect their absorption wavelengths will compare?
Polyenes, and other compounds in which orbitals are conjugated, form very predictable Huckel MO patterns. If there is no conjugation, then we just have one orbital, all by itself. If two orbitals are conjugated, then there are two combinations
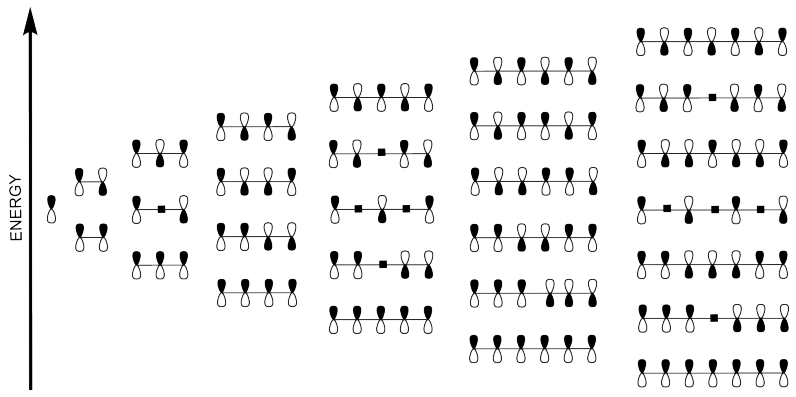
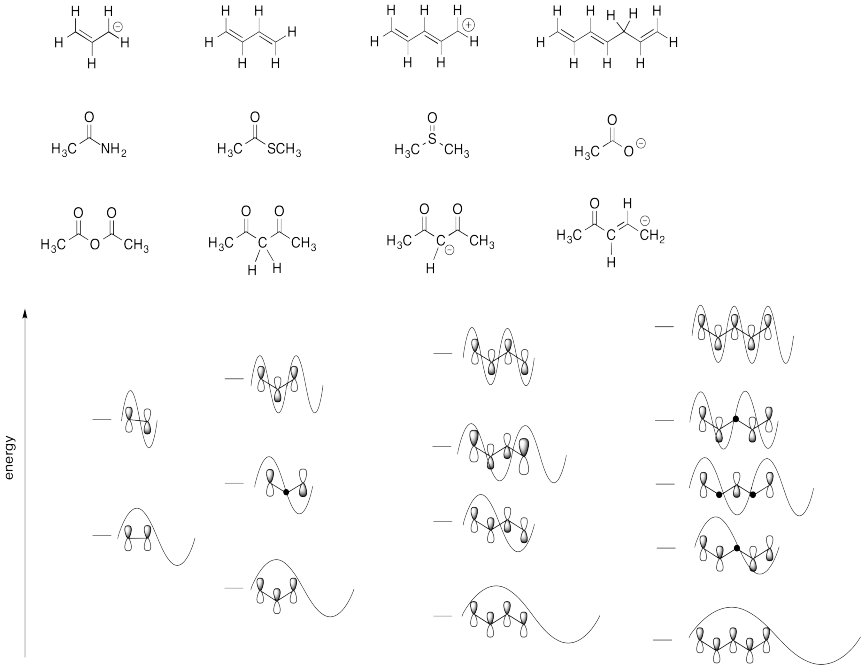
Problem MO15.4.
Match each of the structures below to the correct Huckel MO diagram.