2.3: Quantum-Mechanical Tunneling
- Last updated
- Jan 6, 2020
- Save as PDF
- Page ID
- 202887
( \newcommand{\kernel}{\mathrm{null}\,}\)
Tunneling is a quantum mechanical phenomenon when a particle is able to penetrate through a potential energy barrier that is higher in energy than the particle’s kinetic energy. This amazing property of microscopic particles play important roles in explaining several physical phenomena including radioactive decay. Additionally, the principle of tunneling leads to the development of Scanning Tunneling Microscope (STM) which had a profound impact on chemical, biological and material science research.
Violating Classical Mechanics
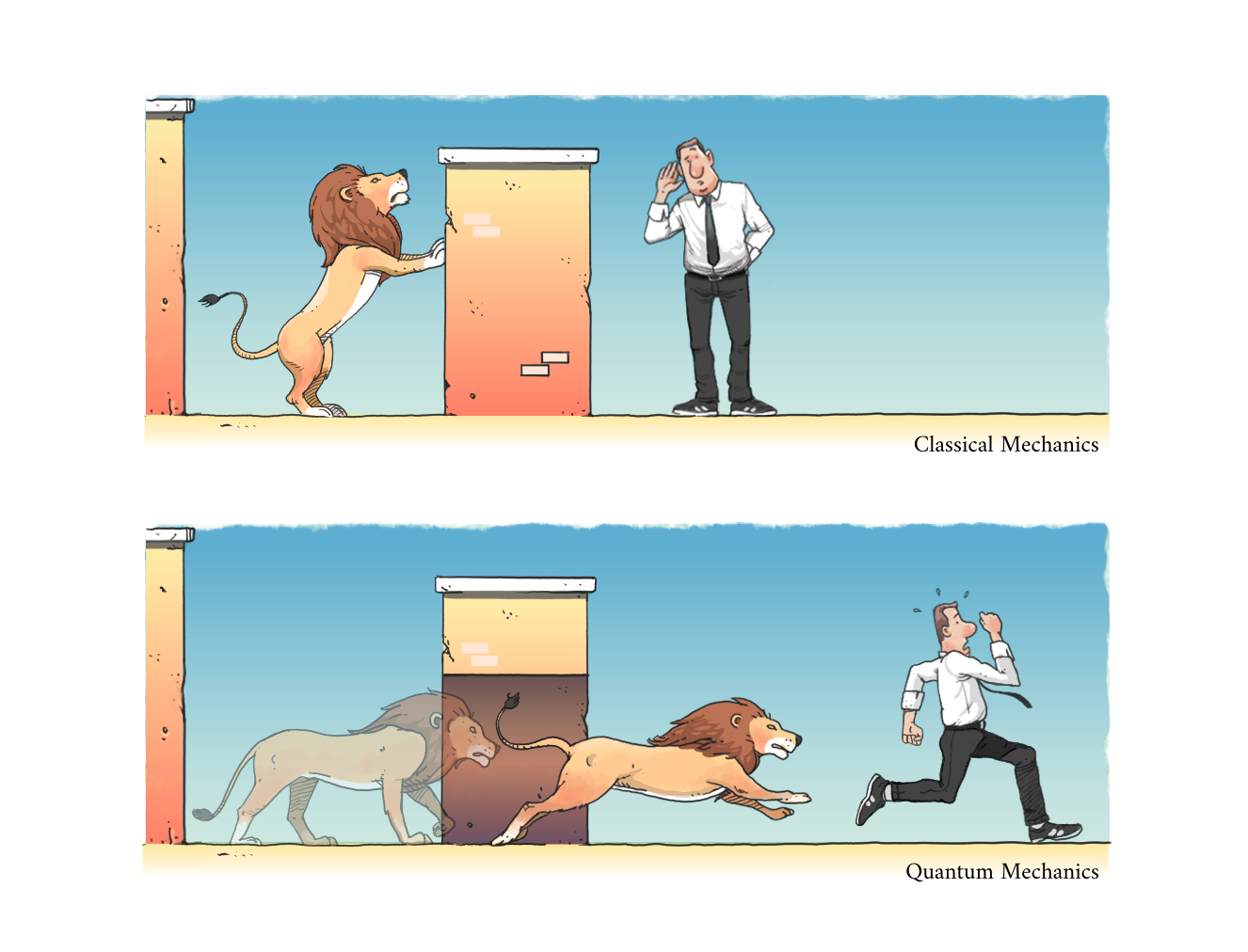
Consider a ball rolling from one valley to another over a hill (Figure 2.3.1). If the ball has enough energy (E) to overcome the potential energy (V) at the top of the barrier between each valley, then it can roll from one valley to the other. This is the classical picture and is controlled by the simple Law of Conservation of Energy approach taught in beginning physics courses. However, If the ball does not have enough kinetic energy (E<V), to overcome the barrier it will never roll from one valley to the other. In contrast, when quantum effects are taken into effect, the ball can "tunnel" through the barrier to the other valley, even if its kinetic energy is less than the potential energy of the barrier to the top of one of the hills.

The Heisenberg Uncertainty Principle in Action
The reason for the difference between classical and quantum motion comes from wave-particle nature of matter. One interpretation of this duality involves the Heisenberg uncertainty principle, which defines a limit on how precisely the position and the momentum of a particle can be known at the same time. This implies that there are no solutions with a probability of exactly zero (or one), though a solution may approach infinity if, for example, the calculation for its position was taken as a probability of 1, the other, i.e. its speed, would have to be infinity. Hence, the probability of a given particle's existence on the opposite side of an intervening barrier is non-zero, and such particles will appear on the 'other' (a semantically difficult word in this instance) side with a relative frequency proportional to this probability.
Microscopic particles such as protons, or electrons would behave differently as a consequence of wave-particle duality. Consider a particle with energy E that is confined in a box which has a barrier of height V. Classically, the box will prevent these particles from escaping due to the insufficiency in kinetic energy of these particles to get over the barrier. However, if the thickness of the barrier is thin, the particles have some probability of penetrating through the barrier without sufficient energy and appear on the other side of the box (Figure 2.3.2).

When it reaches a barrier it cannot overcome, a particle's wave function changes from sinusoidal to exponentially diminishing in form. The solution for the Schrödinger equation in such a medium (Figure 2.3.2; blue region) is:
ψ=Ne−βx
where
- N is a normalization constant and
- β=√2m(V−E)ℏ2
For a quantum particle to appreciably tunnel through a barrier three conditions must be met (Figure 2.3.2):
- The height of the barrier must be finite and the thickness of the barrier should be thin.
- The potential energy of the barrier exceeds the kinetic energy of the particle (E<V).
- The particle has wave properties because the wavefunction is able to penetrate through the barrier. This suggests that quantum tunneling only apply to microscopic objects such protons or electrons and does not apply to macroscopic objects.
If these conditions are met, there would be some probability of finding the particles on the other side of the barrier. Beginning as a sinusoidal wave, a particle begins tunneling through the barrier and goes into exponential decay until it exits the barrier and gets transmitted out the other side as a final sinusoidal wave with a smaller amplitude. The act of tunneling decreases the wave amplitude due the reflection of the incident wave when it comes into the contact with the barrier but does not affect the wave equation.
The probability, P, of a particle tunneling through the potential energy barrier is derived from the Schrödinger Equation and is described as,
P=exp(−4aπh√2m(V−E))
with E<V
where
- V is the potential barrier,
- E is the kinetic energy possessed by the particle, and
- a is the thickness of the barrier.
- m is mass of the particle
- h is Planks Constant (6.6260×10−34m2kg/s)
Therefore, the probability of an object tunneling through a barrier decreases with the object's increasing mass and with the increasing gap between the energy of the object and the energy of the barrier. And although the wave function never quite reaches 0 (as can be determined from the functionality), this explains how tunneling is frequent on nanoscale, but negligible at the macroscopic level. Equation ??? argues that the probability of tunneling decreases exponentially with the square root of the particles mass (particles with a small mass can effectively tunnel through barriers more easily than those with larger mass) and the thickness of the barrier (a)
Example 2.3.1
An electron having total kinetic energy E of 4.50 eV approaches a rectangular energy barrier with V=5.00eV and L=950pm. Classically, the electron cannot pass through the barrier because E<V. Calculate probability of tunneling of this electron through the barrier.
Solution
This is a straightforward application of Equation ???.
P=exp(−4aπh√2m(V−E))
The electronvolt (eV) is a unit of energy that is equal to approximately 1.6×10−19J, which is the conversion used below.
The mass of an electron is 9.10×10−31kg
P=exp[(−(4)(950×10−12m)(π)6.6260×10−34m2kg/s)√(2)(9.10×10−31kg)(5.00−4.50eV)(1.60×10−19J/eV)]=exp−6.88=1.03×10−3
There is a ~0.1% probability of the electrons tunneling though the barrier.
Alpha Decay Radioactivity
Protons and neutrons in a nucleus have kinetic energy, but it is about 8 MeV less than that needed to get out from attractive nuclear potential (Figure 2.3.4). Hence, they are bound by an average of 8 MeV per nucleon. The slope of the hill outside the bowl is analogous to the repulsive Coulomb potential for a nucleus, such as for an α particle outside a positive nucleus. In α decay, two protons and two neutrons spontaneously break away as a 4He unit. Yet the protons and neutrons do not have enough kinetic energy to classically get over the rim.

The α article tunnels through a region of space it is forbidden to be in, and it comes out of the side of the nucleus. Like an electron making a transition between orbits around an atom, it travels from one point to another without ever having been in between (Figure 2.3.5). The wave function of a quantum mechanical particle varies smoothly, going from within an atomic nucleus (on one side of a potential energy barrier) to outside the nucleus (on the other side of the potential energy barrier). Inside the barrier, the wave function does not become zero but decreases exponentially, and we do not observe the particle inside the barrier. The probability of finding a particle is related to the square of its wave function, and so there is a small probability of finding the particle outside the barrier, which implies that the particle can tunnel through the barrier.

Scanning Tunneling Microscopy (STM)
A metal tip usually made out of tungsten is placed between a very small distance above a conducting or semiconducting surface. This distance acts as a potential barrier for tunneling. The space between the tip and the surface normally is vacuum. When electrons tunnel from the metal tip to the surface, a current is created and monitored by a computer (Figure 2.3.6). The current depends on the distance between the tip and the surface, which is controlled by a piezoelectric cylinder. If there is a strong current, the tip will move away from the surface. The increase of the potential barrier will decrease the probability of tunneling and decrease the current. If the current becomes too weak, the tip moves closer to the surface. The potential barrier will be reduced and the current will increase. The variations in the current as the tip moves over the sample are reconstructed by the computer to produce topological image of the scanned surface.

References
- Chang, Raymond. Physical Chemistry for the Biosciences. Sansalito, CA: University Science, 2005. Print.
- Engel, Thomas. Quantum Chemistry and Spectroscopy.Upper Saddle River, NJ. Pearson, 2006. Print.
Contributors and Attributions
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).

