5.11: Chiral Molecules

Objectives

After completing this section, you should be able to

1. use molecular models to show that only a tetrahedral carbon atom satisfactorily accounts for the lack of isomerism in molecules of the type CH₂XY, and for the existence of optical isomerism in molecules of the type CHXYZ.
2. determine whether two differently oriented wedge-and-broken-line structures are identical or represent a pair of enantiomers.

Key Terms

Make certain that you can define, and use in context, the key term below.

• enantiomer

Study Notes

Stereoisomers are isomers that differ in spatial arrangement of atoms, rather than order of atomic connectivity. One of the most interesting types of isomer is the mirror-image stereoisomer, a non-superimposable set of two molecules that are mirror images of one another. The existence of these molecules are determined by a a concept known as chirality. The word “chiral” was derived from the Greek word for hand, because our hands are a good example of chirality since they are non-superimposable mirror images of each other.

Chiral Molecules

The term chiral, from the Greek work for 'hand', refers to anything which cannot be superimposed on its own mirror image. Certain organic molecules are chiral meaning that they are not superimposable on their mirror image. Chiral molecules contain one or more chiral centers, which are almost always tetrahedral (sp³-hybridized) carbons with four different substituents. Consider the molecule A below: a tetrahedral carbon, with four different substituents denoted by balls of four different colors.

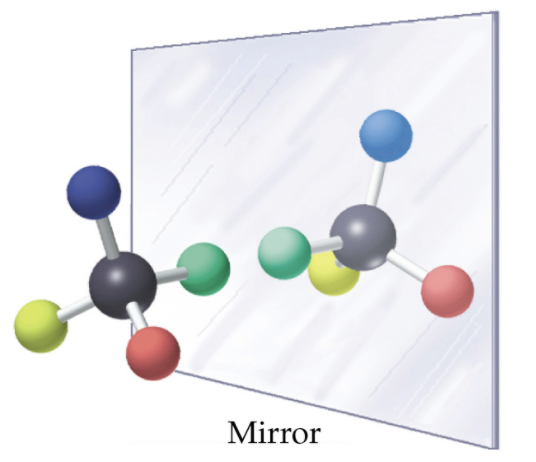
The mirror image of A, which we will call B, is drawn on the right side of the figure, and an imaginary mirror is in the middle. Notice that every point on A lines up through the mirror with the same point on B: in other words, if A looked in the mirror, it would see B looking back.

Now, if we flip compound A over and try to superimpose it point for point on compound B, we find that we cannot do it: if we superimpose any two colored balls, then the other two are misaligned.

A is not superimposable on its mirror image (B), thus by definition A is a chiral molecule. It follows that B also is not superimposable on its mirror image (A), and thus it is also a chiral molecule.

A and B are called stereoisomers or optical isomers: molecules with the same molecular formula and the same bonding arrangement, but a different arrangement of atoms in space. Enantiomers are pairs of stereoisomers which are mirror images of each other: thus, A and B are enantiomers. It should be self-evident that a chiral molecule will always have one (and only one) enantiomer: enantiomers come in pairs. Enantiomers have identical physical properties (melting point, boiling point, density, and so on). However, enantiomers do differ in how they interact with polarized light (we will learn more about this soon) and they may also interact in very different ways with other chiral molecules - proteins, for example. We will begin to explore this last idea in later in this chapter, and see many examples throughout the remainder of our study of biological organic chemistry.

The Many Synonyms of the Chiral Carbon

Be aware - all of the following terms can be used to describe a chiral carbon.

chiral carbon = asymmetric carbon = optically active carbon = stereo carbon = stereo center = chiral center

Let's apply our chirality discussion to real molecules.

Consider 2-butanol, drawn in two dimensions below.

Carbon #2 is a chiral center: it is sp³-hybridized and tetrahedral (even though it is not drawn that way above), and the four substituents attached to is are different: a hydrogen (H) , a methyl (-CH₃) group, an ethyl (-CH₂CH₃) group, and a hydroxyl (OH) group. If the bonding at C₂ of 2-butanol is drawn in three dimensions and this structure called A. Then the mirror image of A can be drawn to form structure B.

When we try to superimpose A onto B, we find that we cannot do it. Because structure A and B are not superimposable on their mirror image they are both chiral molecules. Because A and B are different due only to the arrangement of atoms in space they are stereoisomers. Because A and B are mirror images of each other they are also enantiomers. When looking at simplified line structures is clear that there are two distinct ways of drawing 2-butanol which only differ in their spatial arrangement around a chiral carbon.

The 3D Structures of the Two Enantiomers of 2-Butanol

For comparison, 2-propanol, is an achiral molecule because is lacks a chiral carbon. Carbon #2 is bonded to two identical substituents (methyl groups), and so it is not a chiral carbon. Being achiral means that 2-propanol should be superimposable on its mirror image which is shown in the figure below. A more detailed explaination on why 2-propanol is achiral will be given in the next section.

2-propanol is achiral:

Stereoisomers

Stereoisomers have been defined as molecules with the same connectivity but different arrangements of the atoms in space. It is important to note that there are two types of stereoisomers: geometric and optical.

Optical isomers are molecules whose structures are mirror images but cannot be superimposed on one another in any orientation. Optical isomers have identical physical properties, although their chemical properties may differ in asymmetric environments. Molecules that are nonsuperimposable mirror images of each other are said to be chiral. 25.7.1a" id="MathJax-Element-1-Frame" role="presentation" style="position:relative;" tabindex="0">

Geometric isomers differ in the relative position(s) of substituents in a rigid molecule. Simple rotation about a C–C σ bond in an alkene, for example, cannot occur because of the presence of the π bond. The substituents are therefore rigidly locked into a particular spatial arrangement. Thus a carbon–carbon multiple bond, or in some cases a ring, prevents one geometric isomer from being readily converted to the other. The members of an isomeric pair are identified as either cis or trans, and interconversion between the two forms requires breaking and reforming one or more bonds. Because their structural difference causes them to have different physical and chemical properties, cis and trans isomers are actually two distinct chemical compounds.mers have the same connectivity, but different arrangements of atoms in space. Geometric isomers will be discussed in more detain in Sections 7.4 and 7.5.

Exercise \(\PageIndex{1}\)

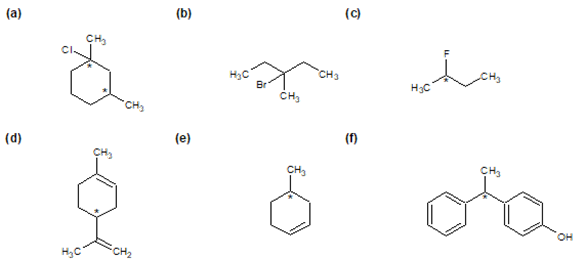
Identify the following molecules as chiral or achiral.

Answer

a) chiral (4 different groups off C)

b) achiral (2 identical -CH₃ substituents off central C)

c) achiral (2 identical -CH₂CH₃ substituents off central C)

d) achiral (2 identical CH₃ substituents off carbon 2)

e) chiral (4 different groups off carbon 2)

f) achiral (2 identical CH₃ substituents off central C)

Exercise \(\PageIndex{2}\)

Determine if the following sets of compounds in each group are enantiomers or the same compound.

Answer

a) enantiomers – non superimposable mirror images

b) same compound – when you rotate the molecule on the right it is identical to the one on the left

c) enantiomers – non superimposable mirror images

d) enantiomers – non superimposable mirror images

Objectives

After completing this section, you should be able to

1. determine whether or not a compound is chiral, given its Kelulé, condensed or shorthand structure, with or without the aid of molecular models.
2. label the chiral centres (carbon atoms) in a given Kelulé, condensed or shorthand structure.

Key Terms

Make certain that you can define, and use in context, the key terms below.

• achiral
• chiral
• chiral (stereogenic) centre
• plane of symmetry

Symmetry and Chirality

Molecules that are nonsuperimposable mirror images of each other are said to be chiral (pronounced “ky-ral,” from the Greek cheir, meaning “hand”). Examples of some familiar chiral objects are your hands. Your left and right hands are nonsuperimposable mirror images. (Try putting your right shoe on your left foot—it just doesn’t work.) An achiral object is one that can be superimposed on its mirror image, as shown by the superimposed flasks 25.7.1b in the figure below. 25.7.1b.

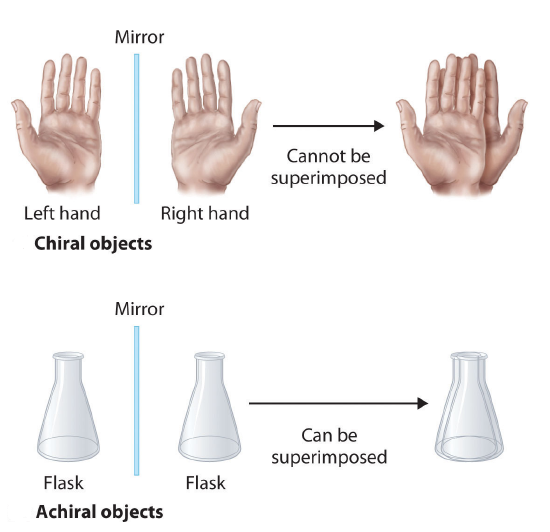
An an important questions is why is one chiral and the other not? The answer is that the flask has a plane of symmetry and your hand does not. A plane of symmetry is a plane or a line through an object which divides the object into two halves that are mirror images of each other. When looking at the flask, a line can be drawn down the middle which separates it into two mirror image halves. However, a similar line down the middle of a hand separates it into two non-mirror image halves. This idea can be used to predict chirality. If an object or molecule has a plane of symmetry it is achiral. If if lacks a plane of symmetry it is chiral.

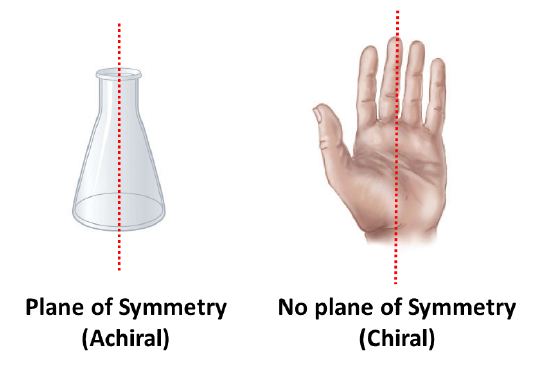
Symmetry can be used to explain why a carbon bonded to four different substituents is chiral. When a carbon is bonded to fewer than four different substituents it will have a plane of symmetry making it achiral. A carbon atom that is bonded to four different substituents loses all symmetry, and is often referred to as an asymmetric carbon. The lack of a plane of symmetry makes the carbon chiral. The presence of a single chiral carbon atom sufficient to render the molecule chiral, and modern terminology refers to such groupings as chiral centers or stereo centers.

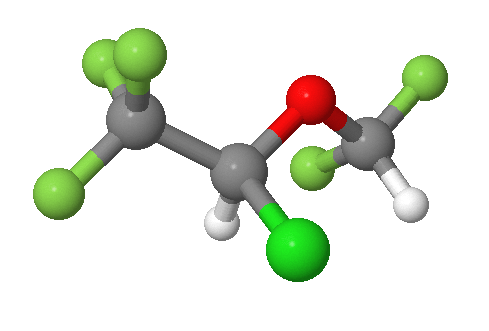
An example is shown in the bromochlorofluoromethane molecule shown in part (a) of the figure below. This carbon, is attached to four different substituents making it chiral. which is often designated by an asterisk in structural drawings. If the bromine atom is replaced by another chlorine to make dichlorofluoromethane, as shown in part (b) below, the molecule and its mirror image can now be superimposed by simple rotation. Thus the carbon is no longer a chiral center. Upon comparison, bromochlorofluoromethane lacks a plane of symmetry while dichlorofluoromethane has a plane of symmetry.

Identifying Chiral carbons

Identifying chiral carbons in a molecule is an important skill for organic chemists. The presence of a chiral carbon presents the possibility of a molecule having multiple stereoisomers. Most of the chiral centers we shall discuss in this chapter are asymmetric carbon atoms, but it should be recognized that other tetrahedral or pyramidal atoms may become chiral centers if appropriately substituted. Also, when more than one chiral center is present in a molecular structure, care must be taken to analyze their relationship before concluding that a specific molecular configuration is chiral or achiral. This aspect of stereoisomerism will be treated later. Because an carbon requires four different substituents to become asymmertric, it can be said, with few exceptions, that sp² and sp hybridized carbons involved in multiple bonds are achiral. Also, any carbon with more than one hydrogen, such as a -CH₃ or -CH₂- group, are also achiral.

Looking for planes of symmetry in a molecule is useful, but often difficult in practice. It is difficult to illustrate on the two dimensional page, but you will see if you build models of these achiral molecules that, in each case, there is at least one plane of symmetry, where one side of the plane is the mirror image of the other. In most cases, the easiest way to decide whether a molecule is chiral or achiral is to look for one or more stereocenters - with a few rare exceptions, the general rule is that molecules with at least one stereocenter are chiral, and molecules with no stereocenters are achiral.

Determining if a carbon is bonded to four distinctly different substituents can often be difficult to ascertain. Remember even the slightest difference makes a substituent unique. Often these difference can be distant from the chiral carbon itself. Careful consideration and often the building of molecular models may be required. A good example is shown below. It may appear that the molecule is achiral, however, when looking at the groups directly attached to the possible chiral carbon, it is clear that they all different. The two alkyl groups are differ by a single -CH₂- group which is enough to consider them different.

Example \(\PageIndex{1}\)

Predict if the following molecule would be chiral or achiral:

Answer

Achiral. When determining the chirality of a molecule, it best to start by locating any chiral carbons. An obvious candidate is the ring carbon attached to the methyl substituent. The question then becomes: does the ring as two different substituents making the substituted ring carbon chiral? With an uncertainty such as this, it is then helpful try to identify any planes of symmetry in the molecule. This molecule does have a plane of symmetry making the molecule achiral. The plane of symmetry would be easier see if the molecule were view from above. Typically, monosubstituted cycloalkanes have a similar plane of symmetry making them all achiral.

Exercise 5.2.1

Determine if each of the following molecules are chiral or achiral. For chiral molecules indicate any chiral carbons.

Answer

Explanation

Structures F and G are achiral. The former has a plane of symmetry passing through the chlorine atom and bisecting the opposite carbon-carbon bond. The similar structure of compound E does not have such a symmetry plane, and the carbon bonded to the chlorine is a chiral center (the two ring segments connecting this carbon are not identical). Structure G is essentially flat. All the carbons except that of the methyl group are sp² hybridized, and therefore trigonal-planar in configuration. Compounds C, D & H have more than one chiral center, and are also chiral.

Note

In the 1960’s, a drug called thalidomide was widely prescribed in the Western Europe to alleviate morning sickness in pregnant women.

Thalidomide had previously been used in other countries as an antidepressant, and was believed to be safe and effective for both purposes. The drug was not approved for use in the U.S.A. It was not long, however, before doctors realized that something had gone horribly wrong: many babies born to women who had taken thalidomide during pregnancy suffered from severe birth defects.

Researchers later realized the problem lay in the fact that thalidomide was being provided as a mixture of two different isomeric forms.

One of the isomers is an effective medication, the other caused the side effects. Both isomeric forms have the same molecular formula and the same atom-to-atom connectivity, so they are not constitutional isomers. Where they differ is in the arrangement in three-dimensional space about one tetrahedral, sp³-hybridized carbon. These two forms of thalidomide are stereoisomers. If you make models of the two stereoisomers of thalidomide, you will see that they too are mirror images, and cannot be superimposed.

As a historical note, thalidomide was never approved for use in the United States. This was thanks in large part to the efforts of Dr. Frances Kelsey, a Food and Drug officer who, at peril to her career, blocked its approval due to her concerns about the lack of adequate safety studies, particularly with regard to the drug's ability to enter the bloodstream of a developing fetus. Unfortunately, though, at that time clinical trials for new drugs involved widespread and unregulated distribution to doctors and their patients across the country, so families in the U.S. were not spared from the damage caused.

Very recently a close derivative of thalidomide has become legal to prescribe again in the United States, with strict safety measures enforced, for the treatment of a form of blood cancer called multiple myeloma. In Brazil, thalidomide is used in the treatment of leprosy - but despite safety measures, children are still being born with thalidomide-related defects.

Example 5.2.2

Label the molecules below as chiral or achiral, and locate all stereocenters.

Answer

Exercise 5.2.2

1) For the following compounds, star (*) each chiral center, if any.

2) Explain why the following compound is chiral.

3) Determine which of the following objects is chiral.

a) A Glove.

b) A nail.

c) A pair of sunglasses.

d) The written word "Chiral".

4) Place an "*" by all of the chrial carbons in the following molecules.

a)

Erythrose, a four carbon sugar.

b) Isoflurane, an anestetic. Bright green = Chlorine, Pale green = Fluorine.

Answer

1)

2) Though the molecule does not contain a chiral carbon, it is chiral as it is non-superimposable on its mirror image due to its twisted nature (the twist comes from the structure of the double bonds needing to be at 90° angles to each other, preventing the molecule from being planar).

3)

a) Just as hands are chiral a glove must also be chiral.

b) A nail has a plane of symmetry which goes down the middle making it a achiral.

c) A pair of sunglasses has a plane of symmetry which goes through the nose making it achiral.

d) Most written words are chiral. Look one in a mirror to confirm this.

4

a)

b)

Exercise 5.2.3

Circle all of the carbon stereocenters in the molecules below.

Answer

Exercise 5.2.4

Circle all of the carbon stereocenters in the molecules below.

Answer

Here are some more examples of chiral molecules that exist as pairs of enantiomers. In each of these examples, there is a single stereocenter, indicated with an arrow. (Many molecules have more than one stereocenter, but we will get to that that a little later!)

Here are some examples of molecules that are achiral (not chiral). Notice that none of these molecules has a stereocenter.

It is difficult to illustrate on the two dimensional page, but you will see if you build models of these achiral molecules that, in each case, there is at least one plane of symmetry, where one side of the plane is the mirror image of the other. Chirality is tied conceptually to the idea of asymmetry, and any molecule that has a plane of symmetry cannot be chiral. When looking for a plane of symmetry, however, we must consider all possible conformations that a molecule could adopt. Even a very simple molecule like ethane, for example, is asymmetric in many of its countless potential conformations – but it has obvious symmetry in both the eclipsed and staggered conformations, and for this reason it is achiral.

Looking for planes of symmetry in a molecule is useful, but often difficult in practice. In most cases, the easiest way to decide whether a molecule is chiral or achiral is to look for one or more stereocenters - with a few rare exceptions (see section 3.7B), the general rule is that molecules with at least one stereocenter are chiral, and molecules with no stereocenters are achiral. Carbon stereocenters are also referred to quite frequently as chiral carbons.

When evaluating a molecule for chirality, it is important to recognize that the question of whether or not the dashed/solid wedge drawing convention is used is irrelevant. Chiral molecules are sometimes drawn without using wedges (although obviously this means that stereochemical information is being omitted). Conversely, wedges may be used on carbons that are not stereocenters – look, for example, at the drawings of glycine and citrate in the figure above. Just because you see dashed and solid wedges in a structure, do not automatically assume that you are looking at a stereocenter.

Other elements in addition to carbon can be stereocenters. The phosphorus center of phosphate ion and organic phosphate esters, for example, is tetrahedral, and thus is potentially a stereocenter.

We will see in chapter 10 how researchers, in order to investigate the stereochemistry of reactions at the phosphate center, incorporated sulfur and/or ¹⁷O and ¹⁸O isotopes of oxygen (the ‘normal’ isotope is ¹⁶O) to create chiral phosphate groups. Phosphate triesters are chiral if the three substituent groups are different.

Asymmetric quaternary ammonium groups are also chiral. Amines, however, are not chiral, because they rapidly invert, or turn ‘inside out’, at room temperature.

Exercise 5.2.5

Label the molecules below as chiral or achiral, and circle all stereocenters.

a) fumarate (a citric acid cycle intermediate)

b) malate (a citric acid cycle intermediate)

b) malate (a citric acid cycle intermediate)

Answer

a) achiral (no stereocenters)

b) chiral

c) chiral

Exercise 5.2.6

Label the molecules below as chiral or achiral, and circle all stereocenters.

a) acetylsalicylic acid (aspirin)

b) acetaminophen (active ingredient in Tylenol)

c) thalidomide (drug that caused birth defects in pregnant mothers in the 1960’s)

Answer

a) achiral (no stereocenters)

b) achiral (no stereocenters)

c) chiral

Exercise 5.2.7

Draw both enantiomers of the following chiral amino acids.

a) Cysteine

b) Proline

Answer

Exercise 5.2.8

Draw both enantiomers of the following compounds from the given names.

a) 2-bromobutane

b) 2,3-dimethyl-3-pentanol

Answer

Exercise 5.2.9

Which of the following body parts are chiral?

a) Hands b) Eyes c) Feet d) Ears

Answer

a) Hands- chiral since the mirror images cannot be superimposed (think of the example in the beginning of the section)

b) Eyes- achiral since mirror images that are superimposable

c) Feet- chiral since the mirror images cannot be superimposed (Does your right foot fit in your left shoe?)

d) Ears- chiral since the mirror images cannot be superimposed

Exercise 5.2.10

Circle the chiral centers in the following compounds.

Answer

Exercise 5.2.11

Identify the chiral centers in the following compounds.

Answer