4.3: Avogadro's Number: Equality Pattern and Conversions
- Page ID
- 216910
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Use information in a given phrase or word problem to write Avogadro's number equalities.
- Apply an Avogadro's number conversion factor to convert between a molar quantity and a chemical particle count.
Upon establishing that Avogadro's number is required to solve a problem, a corresponding Avogadro's number equality must be developed. Then, using dimensional analysis, the resultant equality can be applied as a conversion factor, in order to bring about a desired unit transformation.
Avogadro's Number Equality Pattern
As stated in Section 4.1, an equality pattern contains one number and two units on both sides of an equal sign. On the left side of the Avogadro's number equality pattern shown below, which contains a numerical value of "1," one of these units, "mol," is defined. Neither unit is specified on the right side of this equality pattern, which utilizes Avogadro's number as its numerical quantity. The remaining positions, which are indicated as "blanks" in the equality pattern shown below, should be occupied by units that are relevant to the identity of the specific chemical that is referenced in a given problem. As indicated below, the secondary unit on the left side and the first unit on the right side of an Avogadro's number equality should be the chemical formula of the chemical that is being considered. A chemical name should not be used in this, or any, equality. As the desired unit transformations in this chapter become increasingly complex, multiple chemicals will be referenced within a single problem. In these scenarios, the formula for the chemical that is written in closest physical proximity to the Avogadro's number indicator word should be selected and incorporated into the equality. The remaining unit on the right side of an Avogadro's number equality should be the indicator word that corresponds to the chemical formula that is written. Usually, this indicator word will also be explicitly-written within an Avogadro's number problem. Finally, note that the relative order of the two units on the right side of an Avogadro's number equality can be interchanged.
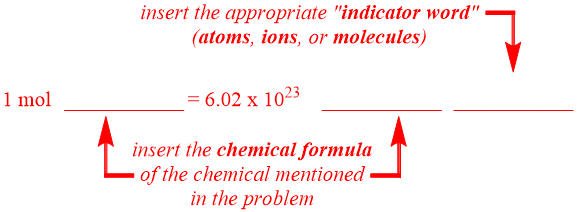
For example, consider the phrase "ions of Ca+2."
The word "ions" indicates that an Avogadro's number equality should be developed. Furthermore, since "ions" is an indicator word, this word is inserted as the second unit on the right side of the Avogadro's number equality that is being created. The chemical that is referenced in the given statement is "Ca+2." As this chemical information was given as a chemical formula, the symbol "Ca+2" is directly incorporated into the remaining unit positions in the equality. The resultant Avogadro's number equality is shown below.
1 mol Ca+2 = 6.02 × 1023 Ca+2 ions
Note that the given chemical formula is actually an ion symbol, as evidenced by the charge that is written as a superscript on the elemental symbol. Therefore, the indicator word "ions" appropriately corresponds to the chemical identified in the given statement.
Write an equality appropriate to the phrase "atoms of silicon."
Solution
The word "atoms" indicates that an Avogadro's number equality should be developed. Furthermore, since "atoms" is an indicator word, this word is inserted as the second unit on the right side of the Avogadro's number equality that is being created. The chemical that is referenced in the given statement is "silicon." However, this information was given in the form of a chemical name, which should not be used in this, or any, equality. Instead, the corresponding elemental symbol, "Si," is incorporated into the remaining unit positions in the equality that is being developed. The resultant Avogadro's number equality is shown below.
1 mol Si = 6.02 × 1023 Si atoms
Because the given chemical name is an elemental name, the indicator word "atoms" appropriately corresponds to the chemical identified in the given statement.
Write an equality appropriate to the phrase "molecules of magnesium acetate."
- Answer
- The word "molecules" indicates that an Avogadro's number equality should be developed. Furthermore, since "molecules" is an indicator word, this word is inserted as the second unit on the right side of the Avogadro's number equality that is being created. The chemical that is referenced in the given statement is "magnesium acetate." However, this information was given in the form of a chemical name, which should not be used in this, or any, equality. Instead, the corresponding chemical formula, "Mg(C2H3O2)2," which is derived by applying the Chapter 3 rules for determining ionic chemical formulas, is incorporated into the remaining unit positions in the equality that is being developed. The resultant Avogadro's number equality is shown below.
1 mol Mg(C2H3O2)2 = 6.02 × 1023 Mg(C2H3O2)2 molecules
Because the given chemical information is the name of an ionic compound, the indicator word "molecules" appropriately corresponds to the chemical identified in the given statement.
Applying Avogadro's Number Equalities as Conversion Factors
Once an appropriate Avogadro's number equality has been developed, the information that it contains can be re-written in the form of a conversion factor, which can then be applied to bring about a desired unit transformation. Recall that the quantity containing the unit being canceled must be written in the denominator of a conversion factor. This will cause the given unit, which appears in a numerator, to be divided by itself, since the same unit appears in the denominator of the conversion factor. Since any quantity that is divided by itself "cancels," orienting the conversation factor in this way results in the elimination of the undesirable unit. However, remember that both components of the equalities that are developed in this chapter contain two units. Therefore, in order to achieve complete unit cancelation, a conversion factor that results in the simultaneous elimination of both units must be applied.
For example, use a conversion factor based on the equality developed above for Ca+2 to calculate how many ions of Ca+2 are present in 9.74 moles of Ca+2.
As stated above, the word "ions" indicates that an Avogadro's number equality pattern should be developed and applied to solve this problem. The equality that was generated to correspond with the phrase "ions of Ca+2" is replicated below.
1 mol Ca+2 = 6.02 × 1023 Ca+2 ions
To create a conversion factor from this equality, the quantity on the left side of the equal sign is written in the numerator of a fraction, and the other quantity is written in the denominator. A second conversion factor can be developed by interchanging where each quantity is written, relative to the fraction bar. Both of the resultant conversion factors are shown below.
\( \dfrac{ \text{1 mol Ca}^{+2}}{6.02 × 10^{23} {\text{ Ca}^{+2}} \text { ions }} \) and \( \dfrac{6.02 × 10^{23} {\text{ Ca}^{+2}} \text { ions }}{ \text{1 mol Ca}^{+2}} \)
However, only one of these conversion factors will allow for the complete cancelation of the given unit, "moles of Ca+2," since both of the units that are being canceled must be written in the denominator of the conversion factor that should be applied to solve the given problem. Since the intent of this problem is to eliminate the unit "moles of Ca+2," the conversion factor on the right must be used. As stated above, the relative order of the two units on the right side of an Avogadro's number equality can be interchanged. While reversing the order of these units is not absolutely necessary, doing so more clearly illustrates that the answer will ultimately be expressed in the desired unit. Therefore,
\( {9.74 \; \cancel{\rm{mol} \; \rm{Ca^{+2} }}} \times\) \( \dfrac{6.02 \times 10^{23} \; \rm{ions} \; \rm{Ca}^{+2}}{1 \; \cancel{\rm{mol} \; \rm{Ca}^{+2}}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. Recall that, when using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division. Furthermore, any quantity that is expressed in scientific notation should be offset by an additional set of parentheses when entered into a calculator. In this case,
9.74 × ((6.02 × 1023) ions Ca+2 ÷ 1) = 5.86348 × 1024 ions Ca+2 ≈ 5.86 × 1024 ions Ca+2
Finally, remember that the correct number of significant figures should be applied to any calculated quantity. Since the math involved in dimensional analysis is multiplication and division, the number of significant figures in each number being multiplied or divided must be counted, and the answer must be limited to the lesser count of significant figures. Neither the given number nor the rounded version of Avogadro's number that was utilized above are exact numbers. As each of these values contains three significant figures, the final answer should be rounded to three significant digits, as shown above.
Use a conversion factor based on the equality developed in Example \(\PageIndex{1}\) to calculate how many moles of silicon contain 4.3 × 1025 atoms of silicon.
Solution
The word "atoms" indicates that an Avogadro's number equality should be developed and applied to solve this problem. The Avogadro's number equality that was generated to correspond with the phrase "atoms of silicon" is replicated below.
1 mol Si = 6.02 × 1023 Si atoms
In order to completely eliminate the unit "atoms of silicon," the quantity on the right side of this equality becomes the denominator in the conversion factor that is applied to solve the given problem. Again, the relative order of the two units on the right side of an Avogadro's number equality can be interchanged. While reversing the order of these units is not absolutely necessary, doing so more clearly illustrates the desired unit cancelation for this particular problem. The remaining portion of the Avogadro's number equality is written in the numerator in the resultant conversion factor, as shown below.
\( {\text {4.3}} \times {\text{10}^{25}}\) \({\cancel{\rm{atoms } \; \rm{Si}}} \times\) \( \dfrac{1 \; \rm{mol} \; \rm{Si}}{6.02 \times 10^{23} \; \cancel{\rm{atoms} \; \rm{Si}}}\) = \( {\text {71.4285714...}}\) \({\rm{mol} \; \rm{Si}}\) ≈ \( {\text {71}}\) \({\rm{mol} \; \rm{Si}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. When using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division, and any quantity that is expressed in scientific notation should be offset by an additional set of parentheses. Applying the correct number of significant figures to the calculated quantity results in the final answer that is shown above.
Use a conversion factor based on the equality developed in Exercise \(\PageIndex{1}\) to calculate how many molecules of magnesium acetate are present in 0.5177 moles of magnesium acetate.
- Answer
- The word "molecules" indicates that an Avogadro's number equality pattern should be developed and applied to solve this problem. The Avogadro's number equality that was generated to correspond with the phrase "molecules of magnesium acetate" is replicated below.
1 mol Mg(C2H3O2)2 = 6.02 × 1023 Mg(C2H3O2)2 molecules
In order to completely eliminate the unit "moles of magnesium acetate," the quantity on the left side of this equality becomes the denominator in the conversion factor that is applied to solve the given problem. The remaining portion of the equality is written in the numerator in the resultant conversion factor, as shown below. While reversing the order of the two units on the right side of an Avogadro's number equality is not absolutely necessary, doing so more clearly illustrates that the answer will ultimately be expressed in the desired unit.\( {0.5177 \; \cancel{\rm{mol} \; \rm{Mg(C_2H_3O_2)_2 }}} \times\) \( \dfrac{6.02 \times 10^{23} \; \rm{molecules} \; \rm{Mg(C_2H_3O_2)_2}}{1 \; \cancel{\rm{mol} \; \rm{Mg(C_2H_3O_2)_2}}}\) = \( {\text {3.116554}} \times {\text{10}^{23}}\) \({\rm{molecules} \; \rm{Mg(C_2H_3O_2)_2}}\)
≈ \( {\text {3.12}} \times {\text{10}^{23}}\) \({\rm{molecules} \; \rm{Mg(C_2H_3O_2)_2}}\)
The solution is calculated by multiplying the given number by the value in each numerator, and then dividing by the quantity in each denominator. When using a calculator, each conversion factor should be entered in parentheses, or the "=" key should be used after each division, and any quantity that is expressed in scientific notation should be offset by an additional set of parentheses. Applying the correct number of significant figures to the calculated quantity results in the final answer that is shown above.

