2.1 Experiment 1 and 2 Background
- Page ID
- 212012
Absorbance is measured using a spectrophotometer. The intensity I of the light that is transmitted through the sample of a known thickness l is compared to the “reference” intensity I0 of light that did not go through the sample. In practical spectroscopy, the power absorption labeled P(ω) in the discussion of fundamentals above is often called the absorbance and labeled A(). The absorption of light in a suitable concentration range can be described by Lambert-Beer’s law,
\[ A(\nu) = -log(\frac{I}{I_o}) = \epsilon(\nu) * c * l\]
where \(\epsilon\) is the extinction coefficient and \(c\) is the sample concentration. In order to get the dependence of the absorbance on the wavelength, the sample is irradiated by light composed of all the wavelengths of interest, and the transmitted light is then dispersed using a grating. The separated frequency components are measured in different parts of a CCD detector.
Exercise 5
Derive Beer’s Law—it’s easy to find in textbooks and online.
In one experiment, we will use the relationship between absorbance and concentration to determine the concentration of an absorbing molecule of interest. One measures the absorbance of a series of solutions of known concentrations to determine the relationship between the absorbance and concentration (that is, to determine the value of ), usually through a linear fit. Once this is known, then from measurement of the absorbance of a solution of unknown concentration, the concentration can be determined. The measurement error can be minimized by adjusting the concentrations and/or sample length such that the absorbance is between 0.3 and 1, ensuring that the absorption is strong enough to yield a significant difference between \(I_o\) and \(I\), but not so strong that there is too little transmitted light to measure.
Quantum mechanical particle-in-a-box model of electronic excited state energies
We’ve seen how absorption spectra have peaks at the resonance frequencies \(\omega_o\) of electronic, vibrational, or spin states of molecules. Can we calculate the values of the frequencies from first principles? The answer is yes – if we can calculate the energies of the quantum mechanical electronic, vibrational, and spin states. In general this may require sophisticated numerical calculations, but in some cases we can use simple models to get approximate values of the energies.
You will record UV-Vis absorption spectra of electronic transitions in a series of aromatic hydrocarbons illustrated below.
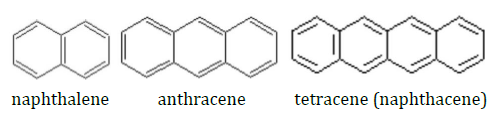
The pi electrons can move freely throughout the molecules. A very simple model that describes the electrons is a quantum mechanical “particle-in-a-box.” In this model, the potential is infinitely high outside the box and zero inside the box. The model is applied to the molecules by assuming that the molecule size is the box size. For a 1-dimensional particle in a box, the energy of the \(n^{th}\) level is given by
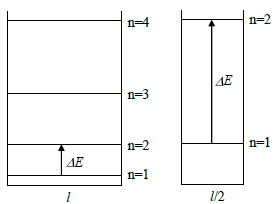
\[ E_n = \frac{n^2 h^2}{8 m l^2}, \text{ } n = 1,2,3,... \tag{8} \]
where m is the mass of the particle and l is the length of the box. The smaller the box, the larger the spacing between the energy levels. Therefore, a smaller box requires light with a higher photon energy (and higher frequency) to induce transitions between the ground state (level 1) and the first excited state (level 2) as shown in Fig. 5. You should observe a shift in the absorption spectral peaks toward lower frequencies, described approximately by Eq. (8), as the number of rings increases.