4.3: Acid-Base Reactions
- Page ID
- 152447
Skills to Develop
-
To know the characteristic properties of acids and bases.
Acid–base reactions are essential in both biochemistry and industrial chemistry. Moreover, many of the substances we encounter in our homes, the supermarket, and the pharmacy are acids or bases. For example, aspirin is an acid (acetylsalicylic acid), and antacids are bases. In fact, every amateur chef who has prepared mayonnaise or squeezed a wedge of lemon to marinate a piece of fish has carried out an acid–base reaction. Before we discuss the characteristics of such reactions, let’s first describe some of the properties of acids and bases.
Definitions of Acids and Bases
We can define acids as substances that dissolve in water to produce H+ ions, whereas bases are defined as substances that dissolve in water to produce OH− ions. In fact, this is only one possible set of definitions. Although the general properties of acids and bases have been known for more than a thousand years, the definitions of acid and base have changed dramatically as scientists have learned more about them. In ancient times, an acid was any substance that had a sour taste (e.g., vinegar or lemon juice), caused consistent color changes in dyes derived from plants (e.g., turning blue litmus paper red), reacted with certain metals to produce hydrogen gas and a solution of a salt containing a metal cation, and dissolved carbonate salts such as limestone (CaCO3) with the evolution of carbon dioxide. In contrast, a base was any substance that had a bitter taste, felt slippery to the touch, and caused color changes in plant dyes that differed diametrically from the changes caused by acids (e.g., turning red litmus paper blue). Although these definitions were useful, they were entirely descriptive.

The Arrhenius Definition of Acids and Bases
The first person to define acids and bases in detail was the Swedish chemist Svante Arrhenius (1859–1927; Nobel Prize in Chemistry, 1903). According to the Arrhenius definition, an acid is a substance like hydrochloric acid that dissolves in water to produce H+ ions (protons; Equation \(\ref{4.3.1}\)), and a base is a substance like sodium hydroxide that dissolves in water to produce hydroxide (OH−) ions (Equation \(\ref{4.3.2}\)):
\[ \underset{an\: Arrhenius\: acid}{HCl_{(g)}} \xrightarrow {H_2 O_{(l)}} H^+_{(aq)} + Cl^-_{(aq)} \label{4.3.1}\]
\[ \underset{an\: Arrhenius\: base}{NaOH_{(s)}} \xrightarrow {H_2O_{(l)}} Na^+_{(aq)} + OH^-_{(aq)} \label{4.3.2}\]
According to Arrhenius, the characteristic properties of acids and bases are due exclusively to the presence of H+ and OH− ions, respectively, in solution. Although Arrhenius’s ideas were widely accepted, his definition of acids and bases had two major limitations:
- First, because acids and bases were defined in terms of ions obtained from water, the Arrhenius concept applied only to substances in aqueous solution.
- Second, and more important, the Arrhenius definition predicted that only substances that dissolve in water to produce \(H^+\) and \(OH^−\) ions should exhibit the properties of acids and bases, respectively. For example, according to the Arrhenius definition, the reaction of ammonia (a base) with gaseous HCl (an acid) to give ammonium chloride (Equation \(\ref{4.3.3}\)) is not an acid–base reaction because it does not involve \(H^+\) and \(OH^−\):
\[NH_{3\;(g)} + HCl_{(g)} \rightarrow NH_4Cl_{(s)} \label{4.3.3}\]
The Brønsted–Lowry Definition of Acids and Bases
Because of the limitations of the Arrhenius definition, a more general definition of acids and bases was needed. One was proposed independently in 1923 by the Danish chemist J. N. Brønsted (1879–1947) and the British chemist T. M. Lowry (1874–1936), who defined acid–base reactions in terms of the transfer of a proton (H+ ion) from one substance to another.
According to Brønsted and Lowry, an acid (A substance with at least one hydrogen atom that can dissociate to form an anion and an \(H^+\) ion (a proton) in aqueous solution, thereby forming an acidic solution) is any substance that can donate a proton, and a base (a substance that produces one or more hydroxide ions (\(OH^-\) and a cation when dissolved in aqueous solution, thereby forming a basic solution) is any substance that can accept a proton. The Brønsted–Lowry definition of an acid is essentially the same as the Arrhenius definition, except that it is not restricted to aqueous solutions. The Brønsted–Lowry definition of a base, however, is far more general because the hydroxide ion is just one of many substances that can accept a proton. Ammonia, for example, reacts with a proton to form \(NH_4^+\), so in Equation \(\ref{4.3.3}\), \(NH_3\) is a Brønsted–Lowry base and \(HCl\) is a Brønsted–Lowry acid. Because of its more general nature, the Brønsted–Lowry definition is used throughout this text unless otherwise specified.
Polyprotic Acids
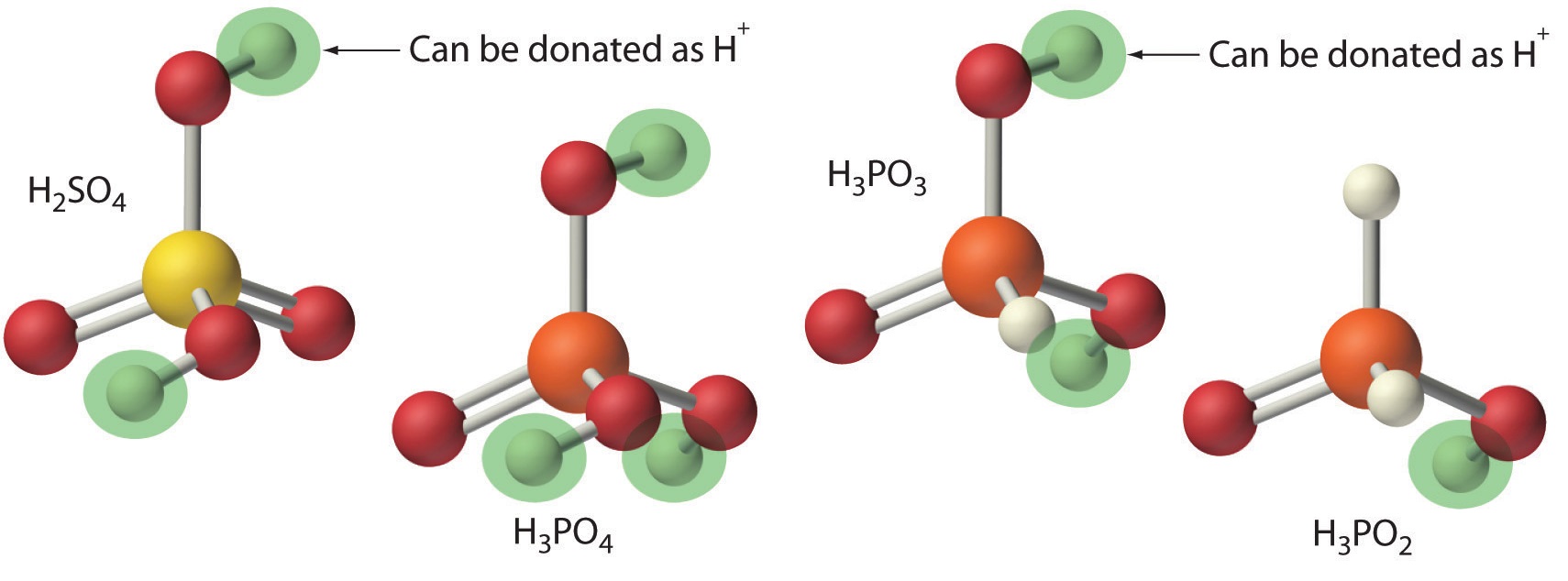
Acids differ in the number of protons they can donate. For example, monoprotic acids (a compound that is capable of donating one proton per molecule) are compounds that are capable of donating a single proton per molecule. Monoprotic acids include HF, HCl, HBr, HI, HNO3, and HNO2. All carboxylic acids that contain a single −CO2H group, such as acetic acid (CH3CO2H), are monoprotic acids, dissociating to form RCO2− and H+. A compound that can donate more than one proton per molecule is known as a polyprotic acid. For example, H2SO4 can donate two H+ ions in separate steps, so it is a diprotic acid (a compound that can donate two protons per molecule in separate steps) and H3PO4, which is capable of donating three protons in successive steps, is a triprotic acid (a compound that can donate three protons per molecule in separate steps), (Equation \(\ref{4.3.4}\), Equation \(\ref{4.3.5}\), and Equation \(\ref{4.3.6}\)):
\[ H_3 PO_4 (l) \overset{H_2 O(l)}{\rightleftharpoons} H ^+ ( a q ) + H_2 PO_4 ^- (aq) \label{4.3.4}\]
\[ H_2 PO_4 ^- (aq) \rightleftharpoons H ^+ (aq) + HPO_4^{2-} (aq) \label{4.3.5}\]
\[ HPO_4^{2-} (aq) \rightleftharpoons H^+ (aq) + PO_4^{3-} (aq) \label{4.3.6}\]
In chemical equations such as these, a double arrow is used to indicate that both the forward and reverse reactions occur simultaneously, so the forward reaction does not go to completion. Instead, the solution contains significant amounts of both reactants and products. Over time, the reaction reaches a state in which the concentration of each species in solution remains constant. The reaction is then said to be in equilibrium (the point at which the rates of the forward and reverse reactions become the same, so that the net composition of the system no longer changes with time).
Strengths of Acids and Bases
We will not discuss the strengths of acids and bases quantitatively until next semester. Qualitatively, however, we can state that strong acids react essentially completely with water to give \(H^+\) and the corresponding anion. Similarly, strong bases dissociate essentially completely in water to give \(OH^−\) and the corresponding cation. Strong acids and strong bases are both strong electrolytes. In contrast, only a fraction of the molecules of weak acids and weak bases react with water to produce ions, so weak acids and weak bases are also weak electrolytes. Typically less than 5% of a weak electrolyte dissociates into ions in solution, whereas more than 95% is present in undissociated form.
In practice, only a few strong acids are commonly encountered: HCl, HBr, HI, HNO3, HClO4, and H2SO4 (H3PO4 is only moderately strong). The most common strong bases are ionic compounds that contain the hydroxide ion as the anion; three examples are NaOH, KOH, and Ca(OH)2. Common weak acids include HCN, H2S, HF, oxoacids such as HNO2 and HClO, and carboxylic acids such as acetic acid. The ionization reaction of acetic acid is as follows:
\[ CH_3 CO_2 H(l) \overset{H_2 O(l)}{\rightleftharpoons} H^+ (aq) + CH_3 CO_2^- (aq) \label{4.3.7}\]
Although acetic acid is very soluble in water, almost all of the acetic acid in solution exists in the form of neutral molecules (less than 1% dissociates). Sulfuric acid is unusual in that it is a strong acid when it donates its first proton (Equation \(\ref{4.3.8}\)) but a weak acid when it donates its second proton (Equation \(\ref{4.3.9}\)) as indicated by the single and double arrows, respectively:
\[ \underset{strong\: acid}{H_2 SO_4 (l)} \xrightarrow {H_2 O(l)} H ^+ (aq) + HSO_4 ^- (aq) \label{4.3.8}\]
\[ \underset{weak\: acid}{HSO_4^- (aq)} \rightleftharpoons H^+ (aq) + SO_4^{2-} (aq) \label{4.3.9}\]
Consequently, an aqueous solution of sulfuric acid contains \(H^+_{(aq)}\) ions and a mixture of \(HSO^-_{4\;(aq)}\) and \(SO^{2−}_{4\;(aq)}\) ions, but no \(H_2SO_4\) molecules. All other polyprotic acids, such as H3PO4, are weak acids.
The most common weak base is ammonia, which reacts with water to form small amounts of hydroxide ion:
\[ NH_3 (g) + H_2 O(l) \rightleftharpoons NH_4^+ (aq) + OH^- (aq) \label{4.3.10}\]
Most of the ammonia (>99%) is present in the form of NH3(g). Amines, which are organic analogues of ammonia, are also weak bases, as are ionic compounds that contain anions derived from weak acids (such as S2−).
There is no correlation between the solubility of a substance and whether it is a strong electrolyte, a weak electrolyte, or a nonelectrolyte.
Table \(\PageIndex{1}\) lists some common strong acids and bases. Acids other than the six common strong acids are almost invariably weak acids. The only common strong bases are the hydroxides of the alkali metals and the heavier alkaline earths (Ca, Sr, and Ba); any other bases you encounter are most likely weak. Remember that there is no correlation between solubility and whether a substance is a strong or a weak electrolyte! Many weak acids and bases are extremely soluble in water.
| Strong Acids | Strong Bases | ||
|---|---|---|---|
| Hydrogen Halides | Oxoacids | Group 1 Hydroxides | Hydroxides of Heavy Group 2 Elements |
| HCl | HNO3 | LiOH | Ca(OH)2 |
| HBr | H2SO4 | NaOH | Sr(OH)2 |
| HI | HClO4 | KOH | Ba(OH)2 |
| RbOH | |||
| CsOH | |||
Example \(\PageIndex{1}\): Acid Strength
Classify each compound as a strong acid, a weak acid, a strong base, a weak base, or none of these.
- CH3CH2CO2H
- CH3OH
- Sr(OH)2
- CH3CH2NH2
- HBrO4
Given: compound
Asked for: acid or base strength
Strategy:
A Determine whether the compound is organic or inorganic.
B If inorganic, determine whether the compound is acidic or basic by the presence of dissociable H+ or OH− ions, respectively. If organic, identify the compound as a weak base or a weak acid by the presence of an amine or a carboxylic acid group, respectively. Recall that all polyprotic acids except H2SO4 are weak acids.
Solution:
- A This compound is propionic acid, which is organic. B It contains a carboxylic acid group analogous to that in acetic acid, so it must be a weak acid.
- A CH3OH is methanol, an organic compound that contains the −OH group. B As a covalent compound, it does not dissociate to form the OH− ion. Because it does not contain a carboxylic acid (−CO2H) group, methanol also cannot dissociate to form H+(aq) ions. Thus we predict that in aqueous solution methanol is neither an acid nor a base.
- A Sr(OH)2 is an inorganic compound that contains one Sr2+ and two OH− ions per formula unit. B We therefore expect it to be a strong base, similar to Ca(OH)2.
- A CH3CH2NH2 is an amine (ethylamine), an organic compound in which one hydrogen of ammonia has been replaced by an R group. B Consequently, we expect it to behave similarly to ammonia (Equation \(\ref{4.3.7}\)), reacting with water to produce small amounts of the OH− ion. Ethylamine is therefore a weak base.
- A HBrO4 is perbromic acid, an inorganic compound. B It is not listed in Table \(\PageIndex{1}\) as one of the common strong acids, but that does not necessarily mean that it is a weak acid. If you examine the periodic table, you can see that Br lies directly below Cl in group 17. We might therefore expect that HBrO4 is chemically similar to HClO4, a strong acid—and, in fact, it is.
Exercise \(\PageIndex{1}\): Acid Strength
Classify each compound as a strong acid, a weak acid, a strong base, a weak base, or none of these.
- Ba(OH)2
- HIO4
- CH3CH2CH2CO2H
- (CH3)2NH
- CH2O
- Answer a
-
strong base
- Answer b
-
strong acid
- Answer c
-
weak acid
- Answer d
-
weak base
- Answer e
-
none of these; formaldehyde is a neutral molecule
The Hydronium Ion
Because isolated protons are very unstable and hence very reactive, an acid never simply “loses” an H+ ion. Instead, the proton is always transferred to another substance, which acts as a base in the Brønsted–Lowry definition. Thus in every acid–base reaction, one species acts as an acid and one species acts as a base. Occasionally, the same substance performs both roles, as you will see later. When a strong acid dissolves in water, the proton that is released is transferred to a water molecule that acts as a proton acceptor or base, as shown for the dissociation of sulfuric acid:
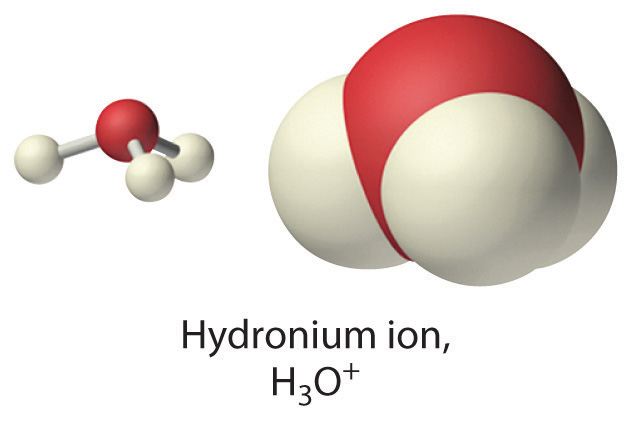
\[ \underset{acid\: (proton\: donor)}{H_2 SO_4 (l)} + \underset{base\: (proton\: acceptor)} {H_2 O(l)} \rightarrow \underset{acid}{H _3 O^+ (aq)} + \underset{base}{HSO_4^- (aq)} \label{4.3.11}\]
Technically, therefore, it is imprecise to describe the dissociation of a strong acid as producing \(H^+_{(aq)}\) ions, as we have been doing. The resulting \(H_3O^+\) ion, called the hydronium ion and is a more accurate representation of \(H^+_{(aq)}\). For the sake of brevity, however, in discussing acid dissociation reactions, we often show the product as \(H^+_{(aq)}\) (as in Equation \(\ref{4.3.7}\)) with the understanding that the product is actually the\(H_3O^+ _{(aq)}\) ion.
Conversely, bases that do not contain the hydroxide ion accept a proton from water, so small amounts of OH− are produced, as in the following:
\[ \underset{base}{NH_3 (g)} + \underset{acid}{H_2 O(l)} \rightleftharpoons \underset{acid}{NH_4^+ (aq)} + \underset{base}{OH^- (aq)} \label{4.3.12}\]
Again, the double arrow indicates that the reaction does not go to completion but rather reaches a state of equilibrium. In this reaction, water acts as an acid by donating a proton to ammonia, and ammonia acts as a base by accepting a proton from water. Thus water can act as either an acid or a base by donating a proton to a base or by accepting a proton from an acid. Substances that can behave as both an acid and a base are said to be amphoteric.
The products of an acid–base reaction are also an acid and a base. In Equation \(\ref{4.3.11}\), for example, the products of the reaction are the hydronium ion, here an acid, and the hydrogen sulfate ion, here a weak base. In Equation \(\ref{4.3.12}\), the products are NH4+, an acid, and OH−, a base. The product NH4+ is called the conjugate acid of the base NH3, and the product OH− is called the conjugate base of the acid H2O. Thus all acid–base reactions actually involve two conjugate acid–base pairs: the Brønsted–Lowry acid and the base it forms after donating its proton, and the Brønsted–Lowry base and the acid it forms after accepting a proton. In Equation \(\ref{4.3.12}\), they are NH4+/NH3 and H2O/OH−.
Neutralization Reactions
A neutralization reaction (a chemical reaction in which an acid and a base react in stoichiometric amounts to produce water and a salt) is one in which an acid and a base react in stoichiometric amounts to produce water and a salt (the general term for any ionic substance that does not have OH− as the anion or H+ as the cation). If the base is a metal hydroxide, then the general formula for the reaction of an acid with a base is described as follows: Acid plus base yields water plus salt. For example, the reaction of equimolar amounts of HBr and NaOH to give water and a salt (NaBr) is a neutralization reaction:
\[ \underset{acid}{HBr(aq)} + \underset{base}{NaOH(aq)} \rightarrow \underset{water}{H_2 O(l)} + \underset{salt}{NaBr(aq)} \label{4.3.13}\]
Acid plus base yields water plus salt.
If we write the complete ionic equation for the reaction in Equation \(\ref{4.3.13}\), we see that \(Na^+_{(aq)}\) and \(Br^−_{(aq)}\) are spectator ions and are not involved in the reaction:
\[ H^+ (aq) + \cancel{Br^- (aq)} + \cancel{Na^+ (aq)} + OH^- (aq) \rightarrow H_2 O(l) + \cancel{Na^+ (aq)} + \cancel{Br^- (aq)} \label{4.3.14}\]
The overall reaction is therefore simply the combination of H+(aq) and OH−(aq) to produce H2O, as shown in the net ionic equation:
\[H^+(aq) + OH^-(aq) \rightarrow H_2O(l)\label{4.3.15}\]
The net ionic equation for the reaction of any strong acid with any strong base is identical to Equation \(\ref{4.3.15}\).
The strengths of the acid and the base generally determine whether the reaction goes to completion. The reaction of any strong acid with any strong base goes essentially to completion, as does the reaction of a strong acid with a weak base, and a weak acid with a strong base. Examples of the last two are as follows:
\[ \underset{strong\: acid}{HCl(aq)} + \underset{weak\: base}{NH_3 (aq)} \rightarrow \underset{salt}{NH_4 Cl(aq)} \label{4.3.16}\]
\[ \underset{weak\: acid} {CH_3 CO _2 H(aq)} + \underset{strong\: base}{NaOH(aq)} \rightarrow \underset{salt}{CH _3 CO _2 Na(aq)} + H_2 O(l) \label{4.3.17}\]
Sodium acetate is written with the organic component first followed by the cation, as is usual for organic salts. Most reactions of a weak acid with a weak base also go essentially to completion. One example is the reaction of acetic acid with ammonia:
\[ \underset{weak\: acid}{CH _3 CO _2 H(aq)} + \underset{weak\: base}{NH_3 (aq)} \rightarrow \underset{salt}{CH_3 CO_2 NH_4 (aq)} \label{4.3.18}\]
An example of an acid–base reaction that does not go to completion is the reaction of a weak acid or a weak base with water, which is both an extremely weak acid and an extremely weak base.
Except for the reaction of a weak acid or a weak base with water, acid–base reactions essentially go to completion.
In some cases, the reaction of an acid with an anion derived from a weak acid (such as HS−) produces a gas (in this case, H2S). Because the gaseous product escapes from solution in the form of bubbles, the reverse reaction cannot occur. Therefore, these reactions tend to be forced, or driven, to completion. Examples include reactions in which an acid is added to ionic compounds that contain the HCO3−, CN−, or S2− anions, all of which are driven to completion:
\[ HCO_3^- (aq) + H^+ (aq) \rightarrow H_2 CO_3 (aq) \label{4.3.19}\]
\[ H_2 CO_3 (aq) \rightarrow CO_2 (g) + H_2 O(l) \]
\[ CN^- (aq) + H^+ (aq) \rightarrow HCN(g) \label{4.3.20}\]
\[ S ^{2-} (aq) + H^+ (aq) \rightarrow HS^- (aq) \label{4.3.21}\]
\[ HS^- (aq) + H^+ (aq) \rightarrow H_2 S(g) \]
The reactions in Equations \(\ref{4.3.19}\)-\(\ref{4.3.21}\) are responsible for the rotten egg smell that is produced when metal sulfides come in contact with acids.
Example \(\PageIndex{2}\): Neutralizing Propionic Acid
Calcium propionate is used to inhibit the growth of molds in foods, tobacco, and some medicines. Write a balanced chemical equation for the reaction of aqueous propionic acid (CH3CH2CO2H) with aqueous calcium hydroxide [Ca(OH)2] to give calcium propionate. Do you expect this reaction to go to completion, making it a feasible method for the preparation of calcium propionate?
Given: reactants and product
Asked for: balanced chemical equation and whether the reaction will go to completion
Strategy:
Write the balanced chemical equation for the reaction of propionic acid with calcium hydroxide. Based on their acid and base strengths, predict whether the reaction will go to completion.
Solution:
Propionic acid is an organic compound that is a weak acid, and calcium hydroxide is an inorganic compound that is a strong base. The balanced chemical equation is as follows:
\(2CH_3CH_2CO_2H(aq) + Ca(OH)_2(aq) \rightarrow (CH_3CH_2CO_2)_2Ca(aq) + 2H_2O(l)\)
The reaction of a weak acid and a strong base will go to completion, so it is reasonable to prepare calcium propionate by mixing solutions of propionic acid and calcium hydroxide in a 2:1 mole ratio.
Exercise \(\PageIndex{2}\)
Write a balanced chemical equation for the reaction of solid sodium acetate with dilute sulfuric acid to give sodium sulfate.
- Answer
-
\[2CH_3CO_2Na(s) + H_2SO_4(aq) \rightarrow Na_2SO_4(aq) + 2CH_3CO_2H(aq) \nonumber\]
One of the most familiar and most heavily advertised applications of acid–base chemistry is antacids, which are bases that neutralize stomach acid. The human stomach contains an approximately 0.1 M solution of hydrochloric acid that helps digest foods. If the protective lining of the stomach breaks down, this acid can attack the stomach tissue, resulting in the formation of an ulcer. Because one factor that is believed to contribute to the formation of stomach ulcers is the production of excess acid in the stomach, many individuals routinely consume large quantities of antacids. The active ingredients in antacids include sodium bicarbonate and potassium bicarbonate (NaHCO3 and KHCO3; Alka-Seltzer); a mixture of magnesium hydroxide and aluminum hydroxide [Mg(OH)2 and Al(OH)3; Maalox, Mylanta]; calcium carbonate (CaCO3; Tums); and a complex salt, dihydroxyaluminum sodium carbonate [NaAl(OH)2CO3; original Rolaids]. Each has certain advantages and disadvantages. For example, Mg(OH)2 is a powerful laxative (it is the active ingredient in milk of magnesia), whereas Al(OH)3 causes constipation. When mixed, each tends to counteract the unwanted effects of the other. Although all antacids contain both an anionic base (OH−, CO32−, or HCO3−) and an appropriate cation, they differ substantially in the amount of active ingredient in a given mass of product.

Stomach acid. An antacid tablet reacts with 0.1 M HCl (the approximate concentration found in the human stomach).
Example \(\PageIndex{3}\)
Assume that the stomach of someone suffering from acid indigestion contains 75 mL of 0.20 M HCl. How many Tums tablets are required to neutralize 90% of the stomach acid, if each tablet contains 500 mg of CaCO3? (Neutralizing all of the stomach acid is not desirable because that would completely shut down digestion.)
Given: volume and molarity of acid and mass of base in an antacid tablet
Asked for: number of tablets required for 90% neutralization
Strategy:
- Write the balanced chemical equation for the reaction and then decide whether the reaction will go to completion.
- Calculate the number of moles of acid present. Multiply the number of moles by the percentage to obtain the quantity of acid that must be neutralized. Using mole ratios, calculate the number of moles of base required to neutralize the acid.
- Calculate the number of moles of base contained in one tablet by dividing the mass of base by the corresponding molar mass. Calculate the number of tablets required by dividing the moles of base by the moles contained in one tablet.
Solution:
A We first write the balanced chemical equation for the reaction:
\(2HCl(aq) + CaCO_3(s) \rightarrow CaCl_2(aq) + H_2CO_3(aq)\)
Each carbonate ion can react with 2 mol of H+ to produce H2CO3, which rapidly decomposes to H2O and CO2. Because HCl is a strong acid and CO32− is a weak base, the reaction will go to completion.
B Next we need to determine the number of moles of HCl present:
\( 75\: \cancel{mL} \left( \dfrac{1\: \cancel{L}} {1000\: \cancel{mL}} \right) \left( \dfrac{0 .20\: mol\: HCl} {\cancel{L}} \right) = 0. 015\: mol\: HCl \)
Because we want to neutralize only 90% of the acid present, we multiply the number of moles of HCl by 0.90:
\((0.015\: mol\: HCl)(0.90) = 0.014\: mol\: HCl\)
We know from the stoichiometry of the reaction that each mole of CaCO3 reacts with 2 mol of HCl, so we need
\( moles\: CaCO_3 = 0 .014\: \cancel{mol\: HCl} \left( \dfrac{1\: mol\: CaCO_3}{2\: \cancel{mol\: HCl}} \right) = 0 .0070\: mol\: CaCO_3 \)
C Each Tums tablet contains
\( \left( \dfrac{500\: \cancel{mg\: CaCO_3}} {1\: Tums\: tablet} \right) \left( \dfrac{1\: \cancel{g}} {1000\: \cancel{mg\: CaCO_3}} \right) \left( \dfrac{1\: mol\: CaCO_3} {100 .1\: \cancel{g}} \right) = 0 .00500\: mol\: CaCO_ 3 \)
Thus we need \(\dfrac{0.0070\: \cancel{mol\: CaCO_3}}{0.00500\: \cancel{mol\: CaCO_3}}= 1.4\) Tums tablets.
Exercise \(\PageIndex{3}\)
Assume that as a result of overeating, a person’s stomach contains 300 mL of 0.25 M HCl. How many Rolaids tablets must be consumed to neutralize 95% of the acid, if each tablet contains 400 mg of NaAl(OH)2CO3? The neutralization reaction can be written as follows:
\( NaAl(OH)_2CO_3(s) + 4HCl(aq) \rightarrow AlCl_3(aq) + NaCl(aq) + CO_2(g) + 3H_2O(l) \)
- Answer
-
6.4 tablets
The pH Scale
One of the key factors affecting reactions that occur in dilute solutions of acids and bases is the concentration of H+ and OH− ions. The pH scale provides a convenient way of expressing the hydrogen ion (H+) concentration of a solution and enables us to describe acidity or basicity in quantitative terms. Pure liquid water contains extremely low, but measurable concentrations of H3O+(aq) and OH−(aq) ions produced via an autoionization reaction, in which water acts simultaneously as an acid and as a base:
\[H_2O(l) + H_2O(l) \rightleftharpoons H_3O^+(aq) + OH^-(aq)\label{4.3.22}\]
The concentration of hydrogen ions in pure water is only 1.0 × 10−7 M at 25°C. Because the autoionization reaction produces both a proton and a hydroxide ion, the OH− concentration in pure water is also 1.0 × 10−7 M. Pure water is a neutral solution in which the total positive charge from all the cations is matched by an identical total negative charge from all the anions. At room temperature this is
\[[H^+] = [OH^−] = 1.0 \times 10^{−7}\; M\]
The pH scale describes the hydrogen ion concentration of a solution in a way that avoids the use of exponential notation. pH is often defined as the negative base-10 logarithm of the hydrogen ion concentration; however, pH is actually defined as the negative base-10 logarithm of hydrogen ion activity. As you will learn in a more advanced course, the activity of a substance in solution is related to its concentration. For dilute solutions such as those we are discussing, the activity and the concentration are approximately the same.
\[pH = -\log[H^+]\label{4.3.23}\]
Conversely,
\[[H^+] = 10^{-pH}\label{4.3.24}\]
Because the hydrogen ion concentration is \(1.0 \times 10^{−7}\; M\) in pure water at 25°C, the pH of pure liquid water (and, by extension, of any neutral solution) is
\[pH = -log[1.0 \times 10^{-7}] = 7.00\label{4.3.25}\]
Adding an acid to pure water increases the hydrogen ion concentration and decreases the hydroxide ion concentration because a neutralization reaction occurs, such as that shown in Equation \(\ref{4.3.15}\). Because the negative exponent of [H+] becomes smaller as [H+] increases, the pH decreases with increasing [H+]. For example, a 1.0 M solution of a strong monoprotic acid such as HCl or HNO3 has a pH of 0.00:
\[pH = -log[1.0] = 0.00\label{4.3.26}\]
Note
pH decreases with increasing [H+].
Conversely, adding a base to pure water increases the hydroxide ion concentration and decreases the hydrogen ion concentration. Because the autoionization reaction of water does not go to completion, neither does the neutralization reaction. Even a strongly basic solution contains a detectable amount of H+ ions. For example, a 1.0 M OH− solution has [H+] = 1.0 × 10−14 M. The pH of a 1.0 M NaOH solution is therefore
\[pH = -log[1.0 \times 10^{-14}] = 14.00\label{4.3.27}\]
For practical purposes, the pH scale runs from pH = 0 (corresponding to 1 M H+) to pH 14 (corresponding to 1 M OH−), although pH values less than 0 or greater than 14 are possible.
We can summarize the relationships between acidity, basicity, and pH as follows:
- If pH = 7.0, the solution is neutral.
- If pH < 7.0, the solution is acidic.
- If pH > 7.0, the solution is basic.
Keep in mind that the pH scale is logarithmic, so a change of 1.0 in the pH of a solution corresponds to a tenfold change in the hydrogen ion concentration. The foods and consumer products we encounter daily represent a wide range of pH values, as shown in Figure \(\PageIndex{2}\).
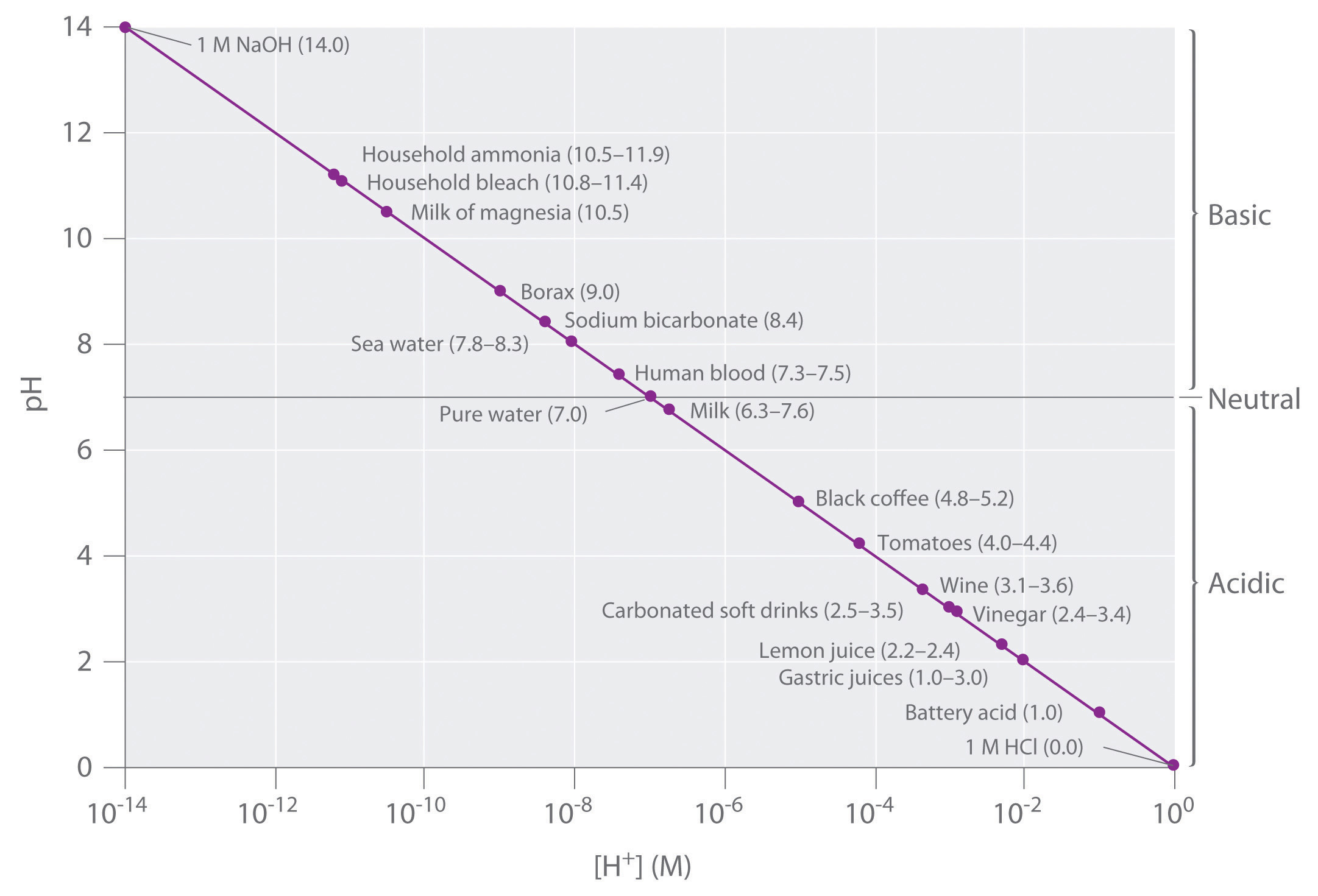
Figure \(\PageIndex{2}\): A Plot of pH versus [H+] for Some Common Aqueous Solutions. Although many substances exist in a range of pH values (indicated in parentheses), they are plotted using typical values.
Example \(\PageIndex{4}\): pH Calculations
- What is the pH of a 2.1 × 10−2 M aqueous solution of HClO4?
- The pH of a vinegar sample is 3.80. What is its hydrogen ion concentration?
Given: molarity of acid or pH
Asked for: pH or [H+]
Strategy:
Using the balanced chemical equation for the acid dissociation reaction and Equation \(\ref{4.3.25}\) or \(\ref{4.3.24}\), determine [H+] and convert it to pH or vice versa.
Solution:
-
HClO4 (perchloric acid) is a strong acid, so it dissociates completely into H+ ions and ClO4− ions:
\[HClO_4(l) \rightarrow H^+(aq) + ClO_4^-(aq)\]
The H+ ion concentration is therefore the same as the perchloric acid concentration. The pH of the perchloric acid solution is thus
\[pH = -log[H^+] = -log(2.1 \times 10^{-2}) = 1.68\]
The result makes sense: the H+ ion concentration is between 10−1 M and 10−2 M, so the pH must be between 1 and 2.
Note: The assumption that [H+] is the same as the concentration of the acid is valid for only strong acids. Because weak acids do not dissociate completely in aqueous solution, a more complex procedure is needed to calculate the pH of their solutions.
-
We are given the pH and asked to calculate the hydrogen ion concentration. From Equation \(\ref{4.3.24}\),
\[10^{-pH} = [H^+]\]
Thus \([H^+] = 10^{-3.80} = 1.6 \times 10^{-4}\: M\).
Exercise \(\PageIndex{4}\)
- What is the pH of a 3.0 × 10−5 M aqueous solution of HNO3?
- What is the hydrogen ion concentration of turnip juice, which has a pH of 5.41?
- Answer a
-
\(pH = 4.52\)
- Answer b
-
\([H^+] = 3.9 \times 10^{-6}\: M\)
Tools have been developed that make the measurement of pH simple and convenient (Figures 4.3.3 and 4.3.4). For example, pH paper consists of strips of paper impregnated with one or more acid–base indicators, which are intensely colored organic molecules whose colors change dramatically depending on the pH of the solution. Placing a drop of a solution on a strip of pH paper and comparing its color with standards give the solution’s approximate pH. A more accurate tool, the pH meter, uses a glass electrode, a device whose voltage depends on the H+ ion concentration (Figure \(\PageIndex{4}\)).
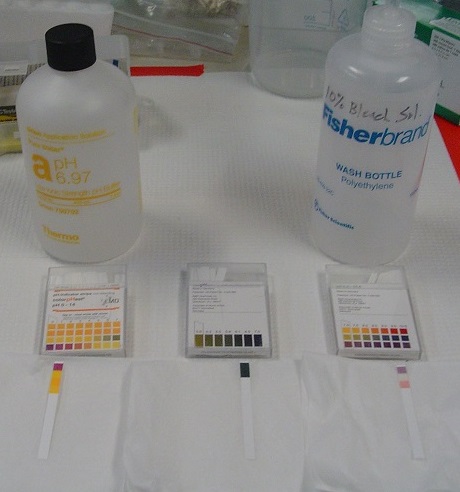

Figure \(\PageIndex{3}\): Ways to measure pH. (left) These pH strips can measure pH in a series of ranges by putting sample on a strip and comparing its color change with colors on the box that correspond to a certain pH. The strip on the left measures pH 0-7 and shows results of a strong acid sample; the center strip is pH range 5-10 and shows results of a 6.97 buffer solution sample; the strip on the right measures a broad range (pH 1-14) and shows results of a 10% bleach water solution sample. This image can be enlarged by clicking on it. (right) These three meters and probes can measure pH (left) and electrical conductivity (center and right). Photos by Monica Bruckner (courtesy of the Science Education Resource Center (SERC) at Carleton College).
Summary
An acidic solution and a basic solution react together in a neutralization reaction that also forms a salt. Acid–base reactions require both an acid and a base. In Brønsted–Lowry terms, an acid is a substance that can donate a proton (H+), and a base is a substance that can accept a proton. All acid–base reactions contain two acid–base pairs: the reactants and the products. Acids can donate one proton (monoprotic acids), two protons (diprotic acids), or three protons (triprotic acids). Compounds that are capable of donating more than one proton are generally called polyprotic acids. Acids also differ in their tendency to donate a proton, a measure of their acid strength. Strong acids react completely with water to produce H3O+(aq) (the hydronium ion), whereas weak acids dissociate only partially in water. Conversely, strong bases react completely with water to produce the hydroxide ion, whereas weak bases react only partially with water to form hydroxide ions. The reaction of a strong acid with a strong base is a neutralization reaction, which produces water plus a salt. The acidity or basicity of an aqueous solution is described quantitatively using the pH scale. The pH of a solution is the negative logarithm of the H+ ion concentration and typically ranges from 0 for strongly acidic solutions to 14 for strongly basic ones. Because of the autoionization reaction of water, which produces small amounts of hydronium ions and hydroxide ions, a neutral solution of water contains 1 × 10−7 M H+ ions and has a pH of 7.0. An indicator is an intensely colored organic substance whose color is pH dependent; it is used to determine the pH of a solution.
Contributors
Modified by Joshua Halpern (Howard University)

