16.4: Quantitative Aspects of Acid–Base Equilibria

The Learning Objective of this Module is to use \(K_a\) and \(K_b\) values to calculate the percent ionization and the pH of a solution of an acid or a base.

This section presents a quantitative approach to analyzing acid–base equilibria. You will learn how to determine the values of \(K_a\) and \(K_b\), how to use \(K_a\) or \(K_b\) to calculate the percent ionization and the pH of an aqueous solution of an acid or a base, and how to calculate the equilibrium constant for the reaction of an acid with a base from the \(K_a\) and \(K_b\) of the reactants.

Determining \(K_a\) and \(K_b\)

The ionization constants \(K_a\) and \(K_b\) are equilibrium constants that are calculated from experimentally measured concentrations, just like the equilibrium constants discussed in Chapter 15. Before proceeding further, it is important to understand exactly what is meant when we describe the concentration of an aqueous solution of a weak acid or a weak base. Suppose, for example, we have a bottle labeled 1.0 M acetic acid or 1.0 M ammonia. As you learned in Chapter 4, such a solution is usually prepared by dissolving 1.0 mol of acetic acid or ammonia in water and adding enough water to give a final volume of exactly 1.0 L. If, however, we were to list the actual concentrations of all the species present in either solution, we would find that none of the values is exactly 1.0 M because a weak acid such as acetic acid or a weak base such as ammonia always reacts with water to some extent. The extent of the reaction depends on the \(K_a\) or the \(K_b\), the concentration of the acid or the base, and the temperature. Consequently, only the total concentration of both the ionized and unionized species is equal to 1.0 M.

The analytical concentration (C) is defined as the total concentration of all forms of an acid or a base that are present in solution, regardless of their state of protonation. Thus a “1.0 M” solution of acetic acid has an analytical concentration of 1.0 M, which is the sum of the actual concentrations of unionized acetic acid (\(CH_3CO_2H\)) and the ionized form (\(CH_3CO_2^−\)):

\[C_{CH_3CO_2H}=[CH_3CO_2H] + [CH_3CO_2^−] \tag{16.38}\]

As we shall see shortly, if we know the analytical concentration and the \(K_a\), we can calculate the actual values of \([CH_3CO_2H]\) and \([CH_3CO_2^−]\).

The equilibrium equations for the reaction of acetic acid and ammonia with water are as follows:

\[K_a=\dfrac{[H^+][CH_3CO_2^−]}{[CH_3CO_2H]} \tag{16.39}\]

\[ K_b=\dfrac{[NH_4^+][OH^−]}{[NH_3]} \tag{16.40}\]

where \(K_a\) and \(K_b\) are the ionization constants for acetic acid and ammonia, respectively. In addition to the analytical concentration of the acid (or the base), we must have a way to measure the concentration of at least one of the species in the equilibrium constant expression to determine the \(K_a\) (or the \(K_b\)). There are two common ways to obtain the concentrations:

1. measure the electrical conductivity of the solution, which is related to the total concentration of ions present, and
2. measure the pH of the solution, which gives \([H^+]\) or \([OH^-]\).

Examples 6 and 7 illustrate the procedure for determining \(K_a\) for a weak acid and \(K_b\) for a weak base. In both cases, we will follow the procedure developed in Chapter 15: the analytical concentration of the acid or the base is the initial concentration, and the stoichiometry of the reaction with water determines the change in concentrations. The final concentrations of all species are calculated from the initial concentrations and the changes in the concentrations. Inserting the final concentrations into the equilibrium constant expression enables us to calculate the \(K_a\) or the \(K_b\).

Example 6: Acetic Acid

Electrical conductivity measurements indicate that 0.42% of the acetic acid molecules in a 1.00 M solution are ionized at 25°C. Calculate \(K_a\) and \(pK_a\) for acetic acid at this temperature. Given: analytical concentration and percent ionization Asked for: \(K_a\) and \(pK_a\) Strategy: Write the balanced equilibrium equation for the reaction and derive the equilibrium constant expression. Use the data given and the stoichiometry of the reaction to construct a table showing the initial concentrations, the changes in concentrations, and the final concentrations for all species in the equilibrium constant expression. Substitute the final concentrations into the equilibrium constant expression and calculate the \(K_a\). Take the negative logarithm of \(K_a\) to obtain the \(pK_a\). Solution: A The balanced equilibrium equation for the dissociation of acetic acid is as follows: \[CH_3CO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+CH3CO^−_{2(aq)}\] and the equilibrium constant expression is as follows: \[K_a=\dfrac{[H^+][CH_3CO_2^−]}{[CH_3CO_2H]}\] B To calculate the \(K_a\), we need to know the equilibrium concentrations of \(CH_3CO_2H\), \(CH_3CO_2^−\), and \(H^+\). The most direct way to do this is to construct a table that lists the initial concentrations and the changes in concentrations that occur during the reaction to give the final concentrations, using the procedure introduced in Chapter 15. The initial concentration of unionized acetic acid ([\(CH_3CO_2H\)]i) is the analytical concentration, 1.00 M, and the initial acetate concentration (\([CH_3CO_2^−]_i\)) is zero. The initial concentration of \(H^+\) is not zero, however; \([H^+]_i\) is \(1.00 \times 10^{−7}\; M\) due to the autoionization of water. The measured percent ionization tells us that 0.42% of the acetic acid molecules are ionized at equilibrium. Consequently, the change in the concentration of acetic acid is \[Δ[CH_3CO_2H] = −(4.2 \times 10^{−3})(1.00\; M) = −0.0042\; M\] Conversely, the change in the acetate concentration is \(Δ[CH_3CO_2^−] = +0.0042\; M\) because every 1 mol of acetic acid that ionizes gives 1 mol of acetate. Because one proton is produced for each acetate ion formed, \(Δ[H^+] = +0.0042\; M\) as well. These results are summarized in the following table. \[CH_3CO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+CH_3CO^−_{2(aq)}\] ICE \([CH_3CO_2H]\) \([H^+]\) \([CH_3CO_2^−]\) Initial 1.00 1.00 × 10−7 0 Change −0.0042 +0.0042 +0.0042 Final The final concentrations of all species are therefore as follows: \([CH_3CO_2H]_f=[CH_3CO_2H]_i+Δ[CH_3CO_2H]=1.00\; M+(−0.0042\; M)=1.00 \;M\) \([CH_3CO_2^−]_f=[CH_3CO_2^−]_i+Δ[CH_3CO_2^−]=0\; M+(+0.0042\; M)=0.0042\; M\) \([H^+]_f=[H^+]_i+Δ[H^+]=1.00 \times 10^{−7} M + (+0.0042\; M)= 0.0042\; M\) C We can now calculate \(K_a\) by inserting the final concentrations into the equilibrium constant expression: \[K_a=\dfrac{[H^+][CH_3CO_2^−]}{[CH_3CO_2H]}=\dfrac{(0.0042)(0.0042)}{1.00}=1.8 \times 10^{−5}\] The \(pK_a\) is the negative logarithm of \(K_a\): \[pK_a = −\log K_a = −\log(1.8 \times 10^{−5}) = 4.74\]

Exercise 6: Picric Acid

Picric acid is the common name for 2,4,6-trinitrophenol, a derivative of phenol (\(C_6H_5OH\)) in which three H atoms are replaced by nitro (\(\ce{–NO_2}\)) groups. The presence of the nitro groups removes electron density from the phenyl ring, making picric acid a much stronger acid than phenol (\(pK_a\) = 9.99). The nitro groups also make picric acid potentially explosive, as you might expect based on its chemical similarity to 2,4,6-trinitrotoluene, better known as TNT. A 0.20 M solution of picric acid is 73% ionized at 25°C. Calculate \(K_a\) and \(pK_a\) for picric acid. Answer: \(K_a = 0.39\) \(pK_a = 0.41\)

Example 7: Ammonia

A 1.0 M aqueous solution of ammonia has a pH of 11.63 at 25°C. Calculate \(K_b\) and \(pK_b\) for ammonia. Given: analytical concentration and pH Asked for: \(K_b\) and \(pK_b\) Strategy: Write the balanced equilibrium equation for the reaction and derive the equilibrium constant expression. Use the data given and the stoichiometry of the reaction to construct a table showing the initial concentrations, the changes in concentrations, and the final concentrations for all species in the equilibrium constant expression. Substitute the final concentrations into the equilibrium constant expression and calculate the \(K_b\). Take the negative logarithm of \(K_b\) to obtain the \(pK_b\). Solution: A The balanced equilibrium equation for the reaction of ammonia with water is as follows: \[NH_{3(aq)}+H_2O_{(l)} \rightleftharpoons NH^+_{4(aq)}+OH^−_{(aq)}\] and the equilibrium constant expression is as follows: \[K_b=\dfrac{[NH_4^+][OH^−]}{[NH_3]}\] Remember that water does not appear in the equilibrium constant expression for \(K_b\). B To calculate \(K_b\), we need to know the equilibrium concentrations of \(NH_3\), \(NH_4^+\), and \(OH^−\). The initial concentration of \(NH_3\) is the analytical concentration, 1.0 M, and the initial concentrations of \(NH_4^+\) and \(OH^−\) are 0 M and \(1.00 \times 10^{−7}\; M\), respectively. In this case, we are given the pH of the solution, which allows us to calculate the final concentration of one species (\(OH^−\)) directly, rather than the change in concentration. Recall that \[pK_w = pH + pOH = 14.00\] at 25°C. Thus \(pOH = 14.00 − pH = 14.00 − 11.63 = 2.37\), and \([OH^−]_f = 10^{−2.37} = 4.3 \times 10^{−3}\; M\). Our data thus far are listed in the following table. \[NH_{3(aq)} \rightleftharpoons NH^+_{4(aq)}+OH^−_{(aq)}\] ICE \([NH_3]\) \([NH_4^+]\) \([OH^−]\) Initial \(1.0\) \(0\) \(1.00 \times 10^{−7}\) Change Final \(4.3 \times 10^{−3}\) The final \([OH^-]\) is much greater than the initial \([H^+]\), so the change in \([OH^-]\) is as follows: \[Δ[OH^−] = (4.3 \times 10^{−3}\; M) − (1.00 \times 10^{−7}\; M) \approx 4.3 \times 10^{−3}\; M\] The stoichiometry of the reaction tells us that 1 mol of \(NH_3\) is converted to \(NH_4^+\) for each 1 mol of \(OH^−\) formed, so \[Δ[NH_4^+] = +4.3 \times 10^{−3}\; M\] and \[Δ[NH_3] = −4.3 \times 10^{−3}\; M\] We can now insert these values for the changes in concentrations into the table, which enables us to complete the table. \[H_2O_{(l)}+NH3_{(aq)} \rightleftharpoons NH^+_{4(aq)}+OH^−_{(aq)}\] ICE \([NH_3]\) \([NH_4^+]\) \([OH^−]\) Initial \(1.0\) \(0\) \(1.00 \times 10^{−7}\) Change \(−4.3 times 10^{−3}\) \(+4.3 \times 10−3\) \(+4.3 \times 10^{−3}\) Final \(1.0\) \(4.3 \times 10−3\) \(4.3 \times 10^{−3}\) C Inserting the final concentrations into the equilibrium constant expression gives \(K_b\): \[K_b=\dfrac{[NH_4^+][OH^−]}{[NH_3]}=\dfrac{(4.3 \times 10^{−3})^2}{1.0}=1.8 \times 10^{−5}\] and \(pK_b = −\log K_b = 4.74\). The \(K_b\) and the \(pK_b\) for ammonia are almost exactly the same as the \(K_a\) and the \(pK_a\) for acetic acid at 25°C. In other words, ammonia is almost exactly as strong a base as acetic acid is an acid. Consequently, the extent of the ionization reaction in an aqueous solution of ammonia at a given concentration is the same as in an aqueous solution of acetic acid at the same concentration.

Exercise 7
The pH of a 0.050 M solution of pyridine (\(C_6H_5N\)) is 8.96 at 25°C. Calculate \(K_b\) and \(pK_b\) for pyridine. Answer \(K_b = 1.7 \times 10^{−9}\); \(pK_b = 8.77\)

Calculating Percent Ionization from \(K_a\) or \(K_b\)

When carrying out a laboratory analysis, chemists frequently need to know the concentrations of all species in solution. Because the reactivity of a weak acid or a weak base is usually very different from the reactivity of its conjugate base or acid, we often need to know the percent ionization of a solution of an acid or a base to understand a chemical reaction. The percent ionization is defined as follows:

\[\text{percent ionization οf acid} =\dfrac{[H^+]}{C_{HA}}×100 \tag{16.41}\]

\[\text{percent ionization οf base}=\dfrac{[OH^−]}{C_B}×100 \tag{16.42}\]

One way to determine the concentrations of species in solutions of weak acids and bases is a variation of the tabular method we used previously to determine \(K_a\) and \(K_b\) values. As a demonstration, we will calculate the concentrations of all species and the percent ionization in a 0.150 M solution of formic acid at 25°C. The data in Table E1 show that formic acid (\(K_a = 1.8 \times 10^{−4}\) at 25°C) is a slightly stronger acid than acetic acid. The equilibrium equation for the ionization of formic acid in water is as follows:

\[HCO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+HCO^−_{2(aq)} \tag{16.43}\]

and the equilibrium constant expression for this reaction is as follows:

\[K_a=\dfrac{[H^+][HCO_2^−]}{[HCO_2H]} \tag{16.44}\]

We set the initial concentration of \(HCO_2H\) equal to 0.150 M, and that of \(HCO_2^−\) is 0 M. The initial concentration of \(H^+\) is \(1.00 \times 10^{−7}\; M\) due to the autoionization of water. Because the equilibrium constant for the ionization reaction is small, the equilibrium will lie to the left, favoring the unionized form of the acid. Hence we can define \(x\) as the amount of formic acid that dissociates.

If the change in \([HCO_2H]\) is \(−x\), then the change in \([H^+]\) and \([HCO_2^−]\) is \(+x\). The final concentration of each species is the sum of its initial concentration and the change in concentration, as summarized in the following ICE table.

\[HCO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+HCO^−_{2(aq)}\]

ICE	\([HCO_2H]\)	\([H^+]\)	\([HCO_2^−]\)
Initial	\(0.150\)	\(1.00 \times 10^{−7}\)	\(0\)
Change	\(−x\)	\(+x\)	\(+x\)
Final	\(0.150 − x\)	\(1.00 \times 10^{−7} + x\)	\(x\)

We can calculate x by substituting the final concentrations from the table into the equilibrium constant expression:

\[K_a=\dfrac{[H^+][HCO_2^−]}{[HCO_2H]}=\dfrac{(1.00 \times 10^{−7}+x) x}{0.150−x}\]

Because the ionization constant \(K_a\) is small, x is likely to be small compared with the initial concentration of formic acid: (0.150 − x) M ≈ 0.150 M. Moreover, \([H^+]\) due to the autoionization of water (1.00 × 10−7 M) is likely to be negligible compared with \([H^+]\) due to the dissociation of formic acid: \((1.00 \times 10^{−7} + x)\; M \approx x \;M\). Inserting these values into the equilibrium constant expression and solving for \(x\),

\[K_a=\dfrac{x^2}{0.150} =1.8 \times 10^{−4}\]

\[x=5.2 \times 10^{−3}\]

We can now calculate the concentrations of the species present in a 0.150 M formic acid solution by inserting this value of x into the expressions in the last line of the table:

\[[HCO_2H]=(0.150−x)\; M=0.145 \;M\]

\[[HCO_2]=x=5.2 \times 10^{−3}\; M\]

\[[H^+]=(1.00×10−7+x) M=5.2 \times 10^{−3} M\]

Thus the pH of the solution is \(–\log(5.2 \times 10^{−3}) = 2.28\). We can also use these concentrations to calculate the fraction of the original acid that is ionized. In this case, the percent ionization is the ratio of \([H^+]\) (or \([HCO_2^−]\)) to the analytical concentration, multiplied by 100 to give a percentage:

\[\text{percent ionization}=\dfrac{[H^+]}{C_{HA}}×100=\dfrac{5.2 \times 10^{−3}\; M}{0.150} \times 100=3.5\%\]

Always check to make sure that any simplifying assumption was valid. As a general rule of thumb, approximations such as those used here are valid only if the quantity being neglected is no more than about 5% of the quantity to which it is being added or from which it is being subtracted. If the quantity that was neglected is much greater than about 5%, then the approximation is probably not valid, and you should go back and solve the problem using the quadratic formula. In the previous demonstration, both simplifying assumptions were justified: the percent ionization is only 3.5%, which is well below the approximately 5% limit, and the \(1.00 \times 10^{−7}\; M \; [H^+]\) due to the autoionization of water is much, much less than the \(5.2 \times 10^{−3}\; M\; [H^+]\) due to the ionization of formic acid.

As a general rule, the \([H^+]\) contribution due to the autoionization of water can be ignored as long as the product of the acid or the base ionization constant and the analytical concentration of the acid or the base is at least 10 times greater than the \([H^+]\) or \([OH^-]\) from the autoionization of water—that is, if

\[K_aC_{HA} \ge 10(1.00 \times 10^{−7}) = 1.0 \times 10^{−6} \tag{16.45}\]

or

\[K_bC_B \ge 10(1.00 \times 10^{−7}) = 1.0 \times 10^{−6} \tag{16.46}\]

By substituting the appropriate values for the formic acid solution into Equation 16.45, we see that the simplifying assumption is valid in this case:

\[K_aC_{HA} = (1.8 \times 10^{−4})(0.150) = 2.7 \times 10^{−5} > 1.0 \times 10^{−6} \tag{16.47}\]

Doing this simple calculation before solving this type of problem saves time and allows you to write simplified expressions for the final concentrations of the species present. In practice, it is necessary to include the \([H^+]\) contribution due to the autoionization of water only for extremely dilute solutions of very weak acids or bases. Example 8 illustrates how the procedure outlined previously can be used to calculate the pH of a solution of a weak base.

Example 8: Ethylamine

Calculate the pH and percent ionization of a 0.225 M solution of ethylamine (\(CH_3CH_2NH_2\)), which is used in the synthesis of some dyes and medicines. The \(pK_b\) of ethylamine is 3.19 at 20°C. Given: concentration and \(pK_b\) Asked for: pH and percent ionization Strategy: Write the balanced equilibrium equation for the reaction and the equilibrium constant expression. Calculate \(K_b\) from \(pK_b\). Use Equation 16.45 to see whether you can ignore \([H^+]\) due to the autoionization of water. Then use a tabular format to write expressions for the final concentrations of all species in solution. Substitute these values into the equilibrium equation and solve for \([OH^-]\). Use Equation 16.42 to calculate the percent ionization. Use the relationship \(K_w = [OH^−][H^+]\) to obtain \([H^+]\). Then calculate the pH of the solution. Solution: A We begin by writing the balanced equilibrium equation for the reaction: \[CH_3CH_2NH_{2(aq)}+H_2O_{(l)} \rightleftharpoons CH_3CH_2NH^+_{3(aq)}+OH^−_{(aq)}\] The corresponding equilibrium constant expression is as follows: \[K_b=\dfrac{[CH_3CH_2NH_3^+][OH^−]}{[CH_3CH_2NH_2}]\] From the \(pK_b\), we have \(K_b = 10−3.19 = 6.5 \times 10^{−4}\). B To calculate the pH, we need to determine the H+ concentration. Unfortunately, \(H^+\) does not appear in either the chemical equation or the equilibrium constant expression. However, \([H^+]\) and \([OH^-]\) in an aqueous solution are related by \(K_w = [H^+][OH^−]\). Hence if we can determine \([OH^-]\), we can calculate \([H^+]\) and then the pH. The initial concentration of \(CH_3CH_2NH_2\) is 0.225 M, and the initial \([OH^-]\) is \(1.00 \times 10^{−7}\; M\). Because ethylamine is a weak base, the extent of the reaction will be small, and it makes sense to let \(x\) equal the amount of \(CH_3CH_2NH_2\) that reacts with water. The change in \([CH_3CH_2NH_2]\) is therefore \(−x\), and the change in both \([CH_3CH_2NH_3^+]\) and \([OH^-]\) is \(+x\). To see whether the autoionization of water can safely be ignored, we substitute \(K_b\) and \(C_B\) into Equation 16.46: \[K_bC_B = (6.5 \times 10^{−4})(0.225) = 1.5 \times 10^{−4} > 1.0 \times 10^{−6}\] Thus the simplifying assumption is valid, and we will not include \([OH^-]\) due to the autoionization of water in our calculations. \[H_2O_{(l)}+CH_3CH_2NH_{2(aq)} \rightleftharpoons CH_3CH_2NH^+_{3(aq)}+OH^−_{(aq)}\] ICE \([CH_3CH_2NH_2]\) \([CH_3CH_2NH_3^+\)] \([OH^−]\) Initial \(0.225\) \(0\) \(1.00 \times 10^{−7}\) Change \(−x\) \(+x\) \(+x\) Final (0.225 − x) \(x\) \(x\) Substituting the quantities from the last line of the table into the equilibrium constant expression, \[K_b=\dfrac{[CH_3CH_2NH_3^+][OH^−]}{[CH_3CH_2NH_2]}=\dfrac{(x)(x)}{0.225−x}=6.5\times 10^{−4}\] As before, we assume the amount of \(CH_3CH_2NH_2\) that ionizes is small compared with the initial concentration, so \([CH_3CH_2NH_2])f = 0.225 − x \approx 0.225\). With this assumption, we can simplify the equilibrium equation and solve for x: \[K_b=\dfrac{x^2}{0.225} =6.5 \times 10^{−4}\] \[x=0.012=[CH_3CH_2NH_3^+]_f=[OH^−]_f\] The percent ionization is therefore \[\text{percent ionization}=\dfrac{[OH^−]}{C_B} \times 100=\dfrac{0.012\; M}{0.225\; M} \times 100=5.4\%\] which is at the upper limit of the approximately 5% range that can be ignored. The final hydroxide concentration is thus 0.012 M. C We can now determine the \([H^+]\) using the expression for \(K_w\): \[K_w=[OH^−][H^+]\] \[1.01 \times 10^{−14} =(0.012 \;M)[H^+]\] \[8.4 \times 10^{−13}\; M=[H^+]\] The pH of the solution is −log(8.4 × 10−13) = 12.08. Alternatively, we could have calculated pOH as −log(0.012) = 1.92 and determined the pH as follows: \[pH + pOH =pKw=14.00\] \[pH=14.00−1.92=12.08\] The two methods are equivalent.

Exercise 8: Analine
Aromatic amines, in which the nitrogen atom is bonded directly to a phenyl ring (\(\ce{−C_6H_5}\)) tend to be much weaker bases than simple alkylamines. For example, aniline (\(C_6H_5NH_2\)) has a \(pK_b\) of 9.13 at 25°C. What is the pH of a 0.050 M solution of aniline? Answer: 8.78

The previous examples illustrate a key difference between solutions of strong acids and bases and solutions of weak acids and bases. Because strong acids and bases ionize essentially completely in water, the percent ionization is always approximately 100%, regardless of the concentration. In contrast, the percent ionization in solutions of weak acids and bases is small and depends on the analytical concentration of the weak acid or base. As illustrated for benzoic acid in Figure 16.16, the percent ionization of a weak acid or a weak base actually increases as its analytical concentration decreases. The percent ionization also increases as the magnitude of \(K_a\) and \(K_b\) increases.

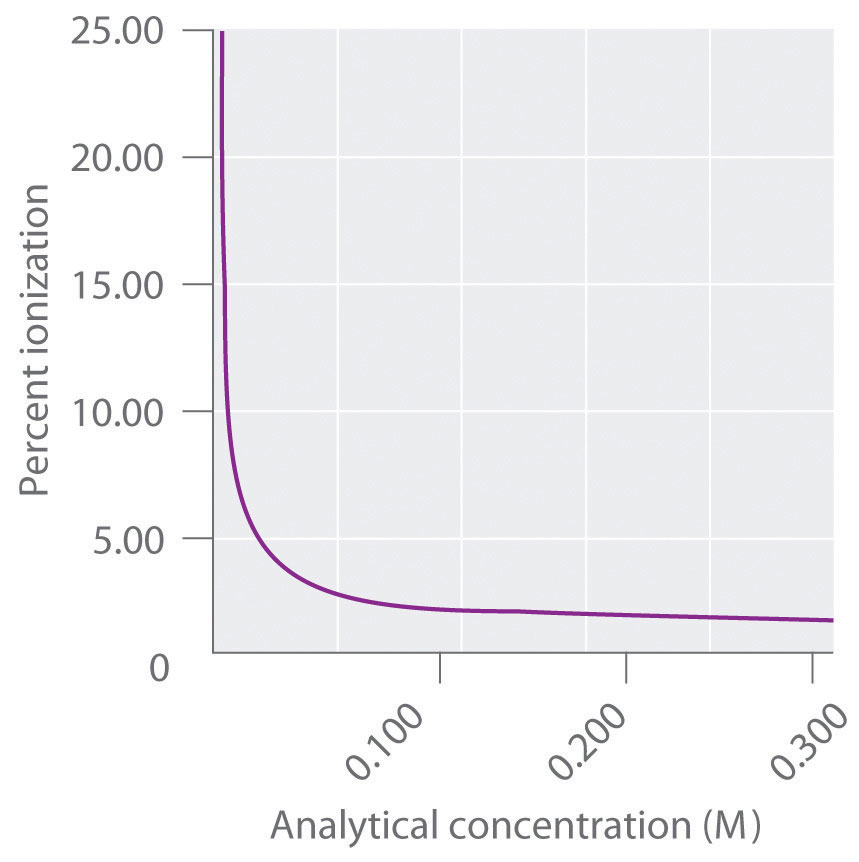
Figure 16.16 The Relationship between the Analytical Concentration of a Weak Acid and Percent Ionization

As shown here for benzoic acid (\(C_6H_5CO_2H\)), the percent ionization decreases as the analytical concentration of a weak acid increases.

Unlike the \(K_a\) or the \(K_b\), the percent ionization is not a constant for weak acids and bases but depends on both the \(K_a\) or the \(K_b\) and the analytical concentration. Consequently, the procedure in Example 8 must be used to calculate the percent ionization and pH for solutions of weak acids and bases. Example 9 and its corresponding exercise demonstrate that the combination of a dilute solution and a relatively large \(K_a\) or \(K_b\) can give a percent ionization much greater than 5%, making it necessary to use the quadratic equation to determine the concentrations of species in solution.

Note
The percent ionization in a solution of a weak acid or a weak base increases as the analytical concentration decreases and as the \(K_a\) or the \(K_b\) increases.

Example 9

Benzoic acid (\(C_6H_5CO_2H\)) is used in the food industry as a preservative and medically as an antifungal agent. Its \(pK_a\) at 25°C is 4.20, making it a somewhat stronger acid than acetic acid. Calculate the percentage of benzoic acid molecules that are ionized in each solution. a 0.0500 M solution a 0.00500 M solution Given: concentrations and \(pK_a\) Asked for: percent ionization Strategy: Write both the balanced equilibrium equation for the ionization reaction and the equilibrium equation (Equation 16.15). Use Equation 16.25 to calculate the \(K_a\) from the \(pK_a\). For both the concentrated solution and the dilute solution, use a tabular format to write expressions for the final concentrations of all species in solution. Substitute these values into the equilibrium equation and solve for \([C_6H_5CO_2^−]_f\) for each solution. Use the values of \([C_6H_5CO_2^−]_f\) and Equation 16.41 to calculate the percent ionization. Solution: A If we abbreviate benzoic acid as \(PhCO_2H\) where \(Ph = \ce{–C_6H_5}\), the balanced equilibrium equation for the ionization reaction and the equilibrium equation can be written as follows: \[PhCO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+PhCO^−_{2(aq)}\] \[K_a=\dfrac{[H^+][PhCO_2^−]}{[PhCO_2H]}\] From the \(pK_a\), we have \(K_a = 10^{−4.20} = 6.3 \times 10^{−5}\). B For the more concentrated solution, we set up our table of initial concentrations, changes in concentrations, and final concentrations: \[PhCO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+PhCO^−_{2(aq)}\] ICE \([PhCO_2H]\) \([H^+]\) \([PhCO_2^−]\) Initial \(0.0500\) \(1.00 \times 10^{−7}\) \(0\) Change \(−x\) \(+x\) \(+x\) Final \(0.0500 − x\) \(1.00 \times 10^{−7} + x\) \(x\) Inserting the expressions for the final concentrations into the equilibrium equation and making our usual assumptions, that \([PhCO_2^−]\) and \([H^+]\) are negligible due to the autoionization of water, \[K_a=\dfrac{[H^+][PhCO_2^−]}{[PhCO_2H]}=\dfrac{(x)(x)}{0.0500−x}=\dfrac{x^2}{0.0500}=6.3 \times 10^{−5}\] \[1.8 \times 10^{-3}=x\] This value is less than 5% of 0.0500, so our simplifying assumption is justified, and \([PhCO_2^−]\) at equilibrium is \(1.8 \times 10^{−3}\; M\). We reach the same conclusion using \[C_{HA}: K_aC_{HA} = (6.3 \times 10^{−5})(0.0500) = 3.2 \times 10^{−6} > 1.0 \times 10^{−6}\] C The percent ionized is the ratio of the concentration of PhCO2− to the analytical concentration, multiplied by 100: \[\text{percent ionized}=\dfrac{[PhCO_2^−]}{C_{PhCO_2H}} \times 100=\dfrac{1.8 \times 10^{−30}}{0.0500} \times 100=3.6\%\] Because only 3.6% of the benzoic acid molecules are ionized in a 0.0500 M solution, our simplifying assumptions are confirmed. B For the more dilute solution, we proceed in exactly the same manner. Our table of concentrations is therefore as follows: \[PhCO_2H_{(aq)} \rightleftharpoons H^+_{(aq)}+PhCO^−_{2(aq)}\] ICE \([PhCO_2H]\) \([H^+]\) \([PhCO_2^−]\) Initial \(0.00500\) \(1.00 \times 10^{−7}\) \(0\) Change \(−x\) \(+x\) \(+x\) Final \(0.00500 − x\) \(1.00 \times 10^{−7} + x\) \(x\) Inserting the expressions for the final concentrations into the equilibrium equation and making our usual simplifying assumptions, \[K_a=\dfrac{[H^+][PhCO_2^−]}{[PhCO_2H]}=\dfrac{(x)(x)}{0.00500−x}=\dfrac{x^2}{0.00500}=6.3 \times 10^{−5}\] \[5.6 \times 10^{−4}=x\] Unfortunately, this number is greater than 10% of 0.00500, so our assumption that the fraction of benzoic acid that is ionized in this solution could be neglected and that \((0.00500 − x) \approx x\) is invalid. Furthermore, we see that \[K_aC_{HA} = (6.3 \times 10^{−5})(0.00500) = 3.2 \times 10^{−7} < 1.0 \times 10^{−6}\] Thus the relevant equation is as follows: \[\dfrac{x^2}{0.00500−x}=6.3 \times 10^{−5}\] which must be solved using the quadratic formula. Multiplying out the quantities, \[x^2 = (6.3 \times 10^{−5})(0.00500 − x) = (3.2 \times 10^{−7}) − (6.3 \times 10^{−5})x\] Rearranging the equation to fit the standard quadratic equation format, \[x^2 + (6.3 \times 10^{−5})x − (3.2 \times 10^{−7}) = 0\] This equation can be solved by using the quadratic formula: \[x=\dfrac{-b \pm \sqrt{b^2 -4ac}}{2a}\] \[x=\dfrac{−(6.3 \times10^{−5}) \pm \sqrt{(6.3 \times 10^{−5})^2−4(1)(−3.2 \times 10^{−7})}}{2(1)}\] \[x=\dfrac{−(6.3 \times 10^{−5}) \pm (1.1 \times 10^{−3})}{2} = 5.3 \times 10^{−4}\] or \[ x= −5.9\times 10^{−4}\] Because a negative \(x\) value corresponds to a negative \([PhCO_2^−]\), which is not physically meaningful, we use the positive solution: \(x = 5.3 \times 10^{−4}\). Thus \([PhCO_2^−] = 5.3 \times 10^{−4}\; M\). C The percent ionized is therefore \[\text{percent ionized}=\dfrac{[PhCO_2^−]C_{PhCO_2H}} \times 100=\dfrac{5.3 \times 10^{−4}}{0.00500} \times 100=11\%\] In the more dilute solution (C = 0.00500 M), 11% of the benzoic acid molecules are ionized versus only 3.6% in the more concentrated solution (C = 0.0500 M). Decreasing the analytical concentration by a factor of 10 results in an approximately threefold increase in the percentage of benzoic acid molecules that are ionized.

Exercise 9: Lactic Acid
Lactic acid (\(CH_3CH(OH)CO_2H\)) is a weak acid with a \(pK_a\) of 3.86 at 25°C. What percentage of the lactic acid is ionized in each solution? 0.10 M solution 0.0020 M solution Answer: 3.7% 23%

Determining \(K_{eq}\) from \(K_a\) and \(K_b\)

In Section 16.2, you learned how to use \(K_a\) and \(K_b\) values to qualitatively predict whether reactants or products are favored in an acid–base reaction. Tabulated values of \(K_a\) (or pKa) and \(K_b\) (or pKb), plus the \(K_w\), enable us to quantitatively determine the direction and extent of reaction for a weak acid and a weak base by calculating K for the reaction. To illustrate how to do this, we begin by writing the dissociation equilibria for a weak acid and a weak base and then summing them:

\[acid:\;\;HA \rightleftharpoons H^++A^− \;\;\; K_a \tag{16.48a}\]

\[\;base:\;\;B + H_2O \rightleftharpoons HB^++OH^− \;\;\; K_b\tag{16.48b}\]

\[sum:\;\;HA + B+ H_2O \rightleftharpoons H^++A^−+HB^++OH^− \;\;\; K_{sum}=K_aK_b \tag{16.48c}\]

The overall reaction has \(H_2O\) on the left and \(H^+\) and \(OH^−\) on the right, which means it involves the autoionization of water (\(H_2O \rightleftharpoons H^++OH^−\)) in addition to the acid–base equilibrium in which we are interested. We can obtain an equation that includes only the acid–base equilibrium by simply adding the equation for the reverse of the autoionization of water (\(H^++OH^− \rightleftharpoons H_2O\)), for which \(K = 1/K_w\), to the overall equilibrium in Equation 16.48 and canceling:

\[HA + B+ \cancel{H_2O} \rightleftharpoons \cancel{H^+} + A^−+HB^++\cancel{OH^−} \;\;\; K_{sum}=K_aK_b \tag{16.49a}\]

\[\cancel{ H^+} + \cancel{OH^−} \rightleftharpoons \cancel{H_2O} \;\;\; 1/K_w \tag{16.49b}\]

\[HA + B \rightleftharpoons A^- + HB^+ \;\;\; K=(K_aK_b)/K_w \tag{16.49c}\]

Thus the equilibrium constant for the reaction of a weak acid with a weak base is the product of the ionization constants of the acid and the base divided by \(K_w\). Example 10 illustrates how to calculate the equilibrium constant for the reaction of a weak acid with a weak base.

Example 10: citric acid and methylamine

Fish tend to spoil rapidly, even when refrigerated. The cause of the resulting “fishy” odor is a mixture of amines, particularly methylamine (\(CH_3NH_2\)), a volatile weak base (\(pK_b = 3.34\)). Fish is often served with a wedge of lemon because lemon juice contains citric acid, a triprotic acid with \(pK_a\) values of 3.13, 4.76, and 6.40 that can neutralize amines. Calculate the equilibrium constant for the reaction of excess citric acid with methylamine, assuming that only the first dissociation constant of citric acid is important. Given: \(pK_b\) for base and \(pK_a\) for acid Asked for: \(K\) Strategy: Write the balanced equilibrium equation and the equilibrium constant expression for the reaction. Convert \(pK_a\) and \(pK_b\) to \(K_a\) and \(K_b\) and then use Equation 16.49 to calculate K. Solution: A If we abbreviate citric acid as \(H_3citrate\), the equilibrium equation for its reaction with methylamine is as follows: \[CH_3NH_{2(aq)}+H_3citrate_{(aq)} \rightleftharpoons CH_3NH^+_{3(aq)}+H_2citrate^−_{(aq)}\] The equilibrium constant expression for this reaction is as follows: \[K=\dfrac{[CH_3NH_3^+][H_2citrate^−]}{[CH_3NH_2][H_3citrate]}\] B Equation 16.49 is \(K = (K_aK_b)/K_w\). Converting \(pK_a\) and \(pK_b\) to \(K_a\) and \(K_b\) gives \(K_a = 10^{−3.13} = 7.4 \times 10^{−4}\) for citric acid and \(K_b = 10^{−3.34} = 4.6 \times 10^{−4}\) for methylamine. Substituting these values into the equilibrium equation, \[K=\dfrac{K_aK_b}{K_w}=\dfrac{(7.4 \times 10^{−4})(4.6 \times 10^{−4})}{1.01 \times 10^{−14}}=3.4 \times 10^7\] The value of pK can also be calculated directly by taking the negative logarithm of both sides of Equation 16.49, which gives \(pK = pK_a + pK_b − pK_w = 3.13 + 3.34 − 14.00 = −7.53\] Thus \(K = 10^{−(−7.53)} = 3.4 \times 10^7\), in agreement with the earlier value. In either case, the \(K\) values show that the reaction of citric acid with the volatile, foul-smelling methylamine lies very far to the right, favoring the formation of a much less volatile salt with no odor. This is one reason a little lemon juice helps make less-than-fresh fish more appetizing.

Exercise 10

Dilute aqueous ammonia solution, often used as a cleaning agent, is also effective as a deodorizing agent. To see why, calculate the equilibrium constant for the reaction of aqueous ammonia with butyric acid (\(CH_3CH_2CH_2CO_2H\)), a particularly foul-smelling substance associated with the odor of rancid butter and smelly socks. The \(pK_b\) of ammonia is 4.75, and the \(pK_a\) of butyric acid is 4.83. Answer: \(2.6 \times 10^4\)

Summary

If the concentration of one or more of the species in a solution of an acid or a base is determined experimentally, \(K_a\) and \(K_b\) can be calculated, and \(K_a\), \(pK_a\), \(K_b\), and \(pK_b\) can be used to quantitatively describe the composition of solutions of acids and bases. The concentrations of all species present in solution can be determined, as can the pH of the solution and the percentage of the acid or base that is ionized. The equilibrium constant for the reaction of a weak acid with a weak base can be calculated from \(K_a\) (or \(pK_a\)), \(K_b\) (or \(pK_b\)), and \(K_w\).

Key Takeaway

For a solution of a weak acid or a weak base, the percent ionization increases as the \(K_a\) or the \(K_b\) increases and as the analytical concentration decreases.

Key Equations

• percent ionization οf acid:

\[=\dfrac{[H^+]}{C_{HA}}×100 \tag{16.41}\]

• percent ionization οf base:

\[\dfrac{[OH^−]}{C_B}×100 \tag{16.42}\]

• Equilibrium constant for reaction of a weak acid with a weak base:

\[K=\dfrac{K_aK_b}{K_w} \tag{16.49}\]

Conceptual Problems

1. Explain why the analytical concentration (C) of \(H_2SO_4\) is equal to \([H)2SO)4] + [HSO)4^−] + [SO)4^{2−}]\).
2. Write an expression for the analytical concentration (C) of \(H_3PO_4\) in terms of the concentrations of the species actually present in solution.
3. For relatively dilute solutions of a weak acid such as acetic acid (\(CH_3CO_2H\)), the concentration of undissociated acetic acid in solution is often assumed to be the same as the analytical concentration. Explain why this is a valid practice.
4. How does dilution affect the percent ionization of a weak acid or a weak base?
5. What is the relationship between the \(K_a\) of a weak acid and its percent ionization? Does a compound with a large \(pK_a\) value have a higher or a lower percent ionization than a compound with a small \(pK_a\) value (assuming the same analytical concentration in both cases)? Explain.
6. For a dilute solution of a weak acid (HA), show that the pH of the solution can be approximated using the following equation (where \(C_{HA}\) is the analytical concentration of the weak acid): \[pH=−\log \sqrt{K_a C_{HA}}\] Under what conditions is this approximation valid?

Numerical Problems

1. The \(pK_a\) of \(NH_3\) is estimated to be 35. Its conjugate base, the amide ion (\(\ce{NH_2−}\)), can be isolated as an alkali metal salt, such as sodium amide (\(NaNH_2\)). Calculate the pH of a solution prepared by adding 0.100 mol of sodium amide to 1.00 L of water. Does the pH differ appreciably from the pH of a \(NaOH\) solution of the same concentration? Why or why not?
2. Phenol is a topical anesthetic that has been used in throat lozenges to relieve sore throat pain. Describe in detail how you would prepare a 2.00 M solution of phenol (\(C_6H_5OH\)) in water; then write equations to show all the species present in the solution. What is the equilibrium constant expression for the reaction of phenol with water? Use the information in Table E1 and Table E2 to calculate the pH of the phenol solution.
3. Describe in detail how you would prepare a 1.50 M solution of methylamine in water; then write equations to show all the species present in the solution. What is the equilibrium constant expression for the reaction of methylamine with water? Use the information in Table E1 and Table E2 to calculate the pH of the solution.
4. A 0.200 M solution of diethylamine, a substance used in insecticides and fungicides, is only 3.9% ionized at 25°C. Write an equation showing the equilibrium reaction and then calculate the \(pK_b\) of diethylamine. What is the \(pK_a\) of its conjugate acid, the diethylammonium ion? What is the equilibrium constant expression for the reaction of diethylammonium chloride with water?
5. A 1.00 M solution of fluoroacetic acid (\(FCH)2CO)2H\)) is 5% dissociated in water. What is the equilibrium constant expression for the dissociation reaction? Calculate the concentration of each species in solution and then calculate the \(pK_a\) of \(FCH_2CO_2H\).
6. The \(pK_a\) of 3-chlorobutanoic acid (\(CH_3CHClCH_2CO_2H\)) is 4.05. What percentage is dissociated in a 1.0 M solution? Do you expect the \(pK_a\) of butanoic acid to be greater than or less than the \(pK_a\) of 3-chlorobutanoic acid? Why?
7. The \(pK_a\) of the ethylammonium ion (\(C_2H_5NH_3^+\)) is 10.64. What percentage of ethylamine is ionized in a 1.00 M solution of ethylamine?
8. The \(pK_a\) of \(Cl_3CCO_2H\) is 0.64. What is the pH of a 0.580 M solution? What percentage of the \(Cl_3CCO_2H\) is dissociated?
9. The pH of a 0.150 M solution of aniline hydrochloride (\(C_6H_5NH_3^+Cl^−\)) is 2.70. What is the \(pK_b\) of the conjugate base, aniline (\(C_6H_5NH_2\))? Do you expect the \(pK_b\) of \((CH_3)_2CHNH_2\) to be greater than or less than the \(pK_b\) of \(C_6H_5NH_2\)? Why?
10. What is the pH of a 0.620 M solution of \(CH_3NH_3^+Br^−\) if the \(pK_b\) of \(CH_3NH_2\) is 10.62?
11. The \(pK_b\) of 4-hydroxypyridine is 10.80 at 25°C. What is the pH of a 0.0250 M solution?

12. The \(pK_a\) values of formic acid and the methylammonium ion are 3.75 and 10.62, respectively. Calculate K for the following reaction: \[HCO^−_{2(aq)} + CH_3NH^+_{3(aq)} \rightleftharpoons HCO_2H_{(aq)}+CH_3NH_{2(aq)}\]
13. The \(pK_a\) values of butanoic acid and the ammonium ion are 4.82 and 9.24, respectively. Calculate K for the following reaction: \[CH_3CH_2CH_2CO^−_{2(aq)}+NH^+_{4(aq)} \rightleftharpoons CH_3CH_2CH_2CO_2H_{(aq)}+NH_{3(aq)}\]
14. Use the information in Table 16.2 "Values of " to calculate the pH of a 0.0968 M solution of calcium formate.
15. Calculate the pH of a 0.24 M solution of sodium lactate. The \(pK_a\) of lactic acid is 3.86.
16. Use the information in Table 16.3 "Values of " to determine the pH of a solution prepared by dissolving 750.0 mg of methylammonium chloride (CH3NH3+Cl−) in enough water to make 150.0 mL of solution.
17. Use the information in Table 16.2 "Values of " to determine the pH of a solution prepared by dissolving 855 mg of sodium nitrite (\(NaNO_2\)) in enough water to make 100.0 mL of solution.

Answers

9. \(pK_b = 9.43\); \((CH_3)_2CHNH_2\) will be a stronger base and have a lower \(pK_b\); aniline is a weaker base because the lone pair on the nitrogen atom can be delocalized on the aromatic ring.

13. \(3.8 \times 10^{−5}\)

17 \(8.18\)