5.2B: Separation Theory
- Last updated
- Save as PDF
- Page ID
- 254941
Raoult's and Dalton's Laws
Distillation of mixtures may or may not produce relatively pure samples. As distillation involves boiling a solution and condensing its vapors, the composition of the distillate is identical to the composition of the vapors. Several equations can be used to describe the composition of vapor produced from a solution.
Raoult's law states that a compound's vapor pressure is lessened when it is part of a solution, and is proportional to its molar composition. Raoult's law is shown in Equation \ref{1}. The equation means that a solution containing \(80 \: \text{mol}\%\) compound "A" and \(20 \: \text{mol}\%\) of another miscible component would at equilibrium produce \(80\%\) as many particles of compound A in the gas phase than if compound A were in pure form.
\[ P_A = P_A^o \chi_A \label{1}\]
where \(P_A^o\) is the vapor pressure3 of a sample of pure A, and \(\chi_A\) is the mole fraction of \(A\) in the mixture.
Dalton's law of partial pressures states that the total pressure in a closed system can be found by addition of the partial pressures of each gaseous component. Dalton's law is shown in Equation \ref{2}.
\[P_\text{total} = P_A + P_B \label{2}\]
A combination of Raoult's and Dalton's laws is shown in Equation \ref{3}, which describes the vapor produced by a miscible two-component system (compounds A + B). This combined law shows that the vapors produced by distillation are dependent on each component's vapor pressure and quantity (mole fraction).
\[P_\text{solution} = P_A^o \chi_A + P_B^o \chi_B \label{3}\]
A compound's vapor pressure reflects the temperature of the solution as well as the compound's boiling point. As temperature increases, a greater percentage of molecules have sufficient energy to overcome the intermolecular forces (IMF's) holding them in the liquid phase. Therefore, a compound's vapor pressure always increases with temperature (see Table 5.2), although not in a linear fashion.
| Compound | Boiling Point (ºC) | Vapor Pressure at 0 ºC | Vapor Pressure at 20 ºC |
|---|---|---|---|
| Diethyl Ether | 34.6 | 183 mmHg | 439 mmHg |
| Methanol | 64.7 | 30 mmHg | 94 mmHg |
| Benzene | 80.1 | 24.5 mmHg | 75 mmHg |
| Toluene | 110.6 | 6.8 mmHg | 22 mmHg |
When comparing two compounds at the same temperature, the compound with weaker IMF's (the one with the lower boiling point) should more easily enter the gas phase. Therefore, at any certain temperature, a compound with a lower boiling point always has a greater vapor pressure than a compound with a higher boiling point (see Table 5.2). This last concept is the cornerstone of distillation theory.
A compound with a lower boiling point always has a greater vapor pressure than a compound with a higher boiling point
Purification Potential
A simple distillation works well to purify certain mixtures, specifically to separate a liquid from non-volatile impurities (e.g. solids or salts), or from small amounts of significantly higher or lower boiling impurities. A general guideline is that a simple distillation is capable of separating components if the difference in boiling point of the components is greater than \(100^\text{o} \text{C}\). A simple distillation does not work well to purify a mixture that contains components with similar boiling points (when the difference in b.p. is \(< 100^\text{o} \text{C}\)).
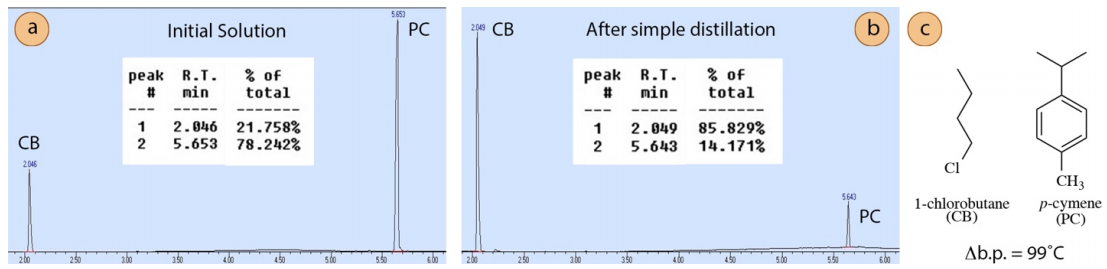
To demonstrate, a mixture containing \(75 \: \text{mol}\%\) 1-chlorobutane (normal b.p. \(78^\text{o} \text{C}\)) and \(25 \: \text{mol}\%\) p-cymene (normal b.p. \(177^\text{o} \text{C}\), Figure 5.10b) was purified using a simple distillation to collect the first two \(\text{mL}\) of distillate. The difference in boiling point of these two components is \(99^\text{o} \text{C}\).
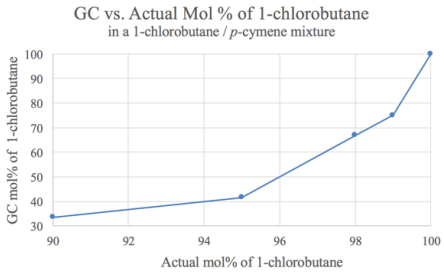
Gas chromatograph (GC) analysis of the initial mixture and distillate allowed for quantitation, although the reported percentages were not proportional to the \(\text{mol}\%\) values because of the detector used (often higher molecular weight compounds have abnormally high integration values with mass spectrometers). A calibration curve was necessary to translate the reported percentages into accurate values (Figure 5.9). To generate the calibration curve, the GC integration values were recorded for several known mixtures of 1-chlorobutane and p-cymene. For example, a sample with \(95 \: \text{mol}\%\) 1-chlorobutane (x-axis) and \(5 \: \text{mol}\%\) p-cymene was reported by the GC instrument to be \(42 \: \text{mol}\%\) 1-chlorobutane (y-axis). This was recorded as the data point (95,42).
| Sample | Component | Recorded GC % | Actual % |
|---|---|---|---|
| Initial Solution | 1-chlorobutane | 22% | 75% |
| Initial Solution | p-cymene | 78% | 25% |
| Distillate | 1-chlorobutane | 86% | 99.5% |
| Distillate | p-cymene | 14% | 0.5% |
GC analysis (Figure 5.10) and a calibration curve (Figure 5.9 showed the distillate to be nearly pure 1-chlorobutane (\(\sim 99.5 \: \text{mol}\%\), Table 5.3). This is an example of a mixture that is purified well through simple distillation.
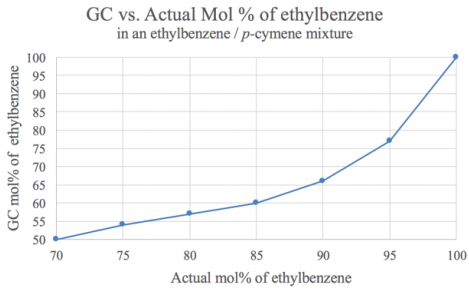
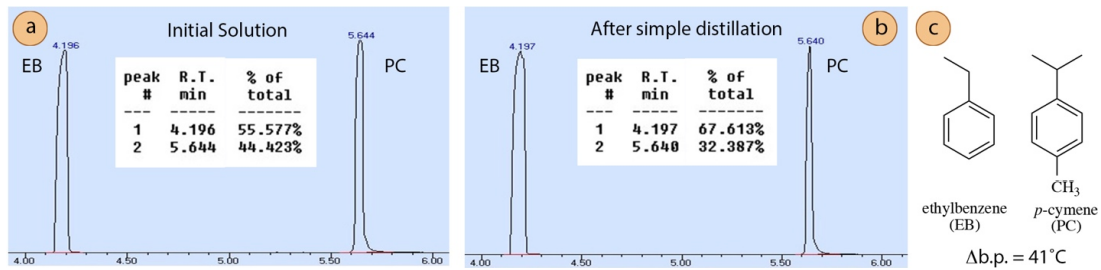
A second distillation was performed in a similar manner using a mixture containing \(75 \: \text{mol}\%\) ethylbenzene (normal b.p. \(136^\text{o} \text{C}\)) and \(25 \: \text{mol}\%\) p-cymene (normal b.p. \(177^\text{o} \text{C}\), Figure 5.12b). The difference in boiling point of these two components is \(41^\text{o} \text{C}\).
| Sample | Component | Recorded GC % | Actual % |
|---|---|---|---|
| Initial Solution | ethylbenzene | 56% | 75% |
| Initial Solution | p-cymene | 44% | 25% |
| Distillate | ethylbenzene | 68% | 90% |
| Distillate | p-cymene | 32% | 10% |
GC analysis (Figure 5.12) and a calibration curve (Figure 5.11) showed the simple distillation did increase the quantity of ethylbenzene in the mixture (from \(75 \: \text{mol}\%\) to \(90 \: \text{mol}\%\), see Table 5.4), but a significant portion of p-cymene remained in the distillate. This is an example of a mixture that is not completely purified by simple distillation.
The two distillations in this section both started with \(75/25 \: \text{mol}\%\) mixtures, and yet the two distillations resulted in distillates with different purity. This result can be explained by analysis of Raoult's and Dalton's laws (Equations \ref{1} and \ref{2}). The equation describing the composition of vapor produced from the \(75 \: \text{mol}\%\) 1-chlorobutane/\(25 \: \text{mol}\%\) p-cymene mixture is shown in Equation \ref{4}:
\[ \begin{align} P_\text{solution} &= P^o_\text{1-chlorobutane} \chi_A + P^o_\text{p-cymene} \chi_B \\[4pt] &= P^o_\text{1-chlorobutane} \left( 0.75 \right) + P^o_\text{p-cymene} \left( 0.25 \right) \label{4} \end{align} \]
In this distillation, the distillate was "enriched" in 1-chlorobutane, as it changed from \(75 \: \text{mol}\%\) 1-chlorobutane in the distilling flask to \(99.5 \: \text{mol}\%\) 1-chlorobutane in the distillate. The distillate contained nearly pure 1-chlorobutane, as it had the greater contribution to the solution's vapor. This is because:
- 1-chlorobutane was present in a greater quantity in the distilling flask (\(75\%\), or had a mole fraction of 0.75)
- 1-chlorobutane had the lower boiling point of the components (\(78^\text{o} \text{C}\) compared to \(177^\text{o} \text{C}\)) and so had a significantly higher vapor pressure than p-cymene \(\left( P^o_\text{1-chlorobutane} \gg P^o_\text{p-cymene} \right)\).
In this distillation, the difference in boiling point of the components was so large (\(\Delta\) b.p. of \(99^\text{o} \text{C}\)), that the vapor pressure of p-cymene (and thus its contribution to the vapor phase) was essentially insignificant during the distillation. The vastly different vapor pressures (along with the differences in mole fraction), were the reason the simple distillation resulted in a nearly pure distillate.
In the distillation of the \(75 \: \text{mol}\%\) ethylbenzene/\(25 \: \text{mol}\%\) p-cymene mixture, the distillate was also enriched in the lower boiling component (ethylbenzene) as it had the greater vapor pressure. However, the distillate still contained \(10 \: \text{mol}\%\) p-cymene, because there was only a \(41^\text{o} \text{C}\) difference in boiling point between the two components. This meant that the higher boiling component (p-cymene) had a smaller but not negligible vapor pressure, leading to a significant quantity of p-cymene in the distillate.
Distillation Curves
Equation (5) describes the vapor produced by a miscible two-component system (compounds A + B). This mathematical equation can be used to generate phase diagrams, or distillation curves, which graphically correlate temperature to the molar composition of the liquid and vapor phases.
\[P_\text{solution} = P^o_A \chi_A + P^o_B \chi_B \label{5}\]
Figure 5.13 shows a generic distillation curve for a two-component system. Molar composition is on the x-axis, with the left side of the plot corresponding to a sample that is pure compound A and the right side of the plot corresponding to a sample of pure compound B. In between the two sides represent mixtures of A and B. Temperature is on the y-axis, and the boiling point of each compound are marked ("bp A" and "bp B").
You may have been previously exposed to phase diagrams for pure compounds (e.g. of water or carbon dioxide), where only a single line would be used to denote a liquid-gas equilibrium. In the distillation of mixtures, there is a difference in composition between the liquid and gas phases, which is the reason for the tear-shaped appearance of the phase diagram. The tear-shaped region represents conditions where both liquid and gas coexist, during boiling of the liquid.
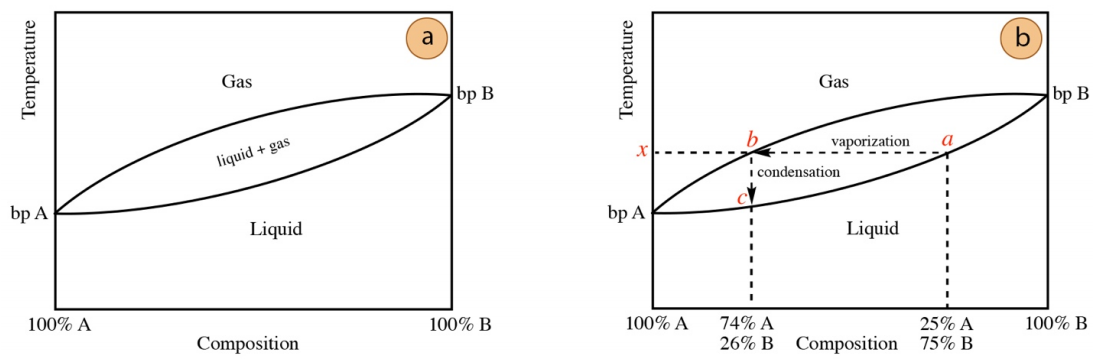
Imagine a \(25 \: \text{mol}\%\) A/\(75 \: \text{mol}\%\) B mixture is to be distilled, and this mixture is described by the distillation curve in Figure 5.13a. When the temperature reaches the lower line of the tear drop (temperature x and point a in Figure 5.13b), the solution begins to boil. Since liquid and gas have the same temperature during boiling, the vaporization process can be thought of as following the horizontal line from position a to b in Figure 5.13b. After vaporization, the gas condenses into the distillate, which can be thought of as following the vertical line from position b to c in Figure 5.13b. Under perfect equilibrating conditions, the distillate in this example would be \(74 \: \text{mol}\%\) A/\(26 \: \text{mol}\%\) B, and is "enriched" in A as it has the lower boiling point and thus the higher vapor pressure.
In a distillation curve, the leftward horizontal "movement" followed by downward vertical "movement" represents one vaporization-condensation event (see Figure 5.13b). This is often referred to as a "theoretical plate", historical terminology related to the collection of distillate onto plates or trays, and represents the purification potential of a simple distillation.
Every mixture has its own distillation curve, reflective of the boiling points of the components. A mixture containing two components whose boiling points are vastly different (\(\Delta\) b.p. \(> 100^\text{o} \text{C}\)) is shown in Figure 5.14.
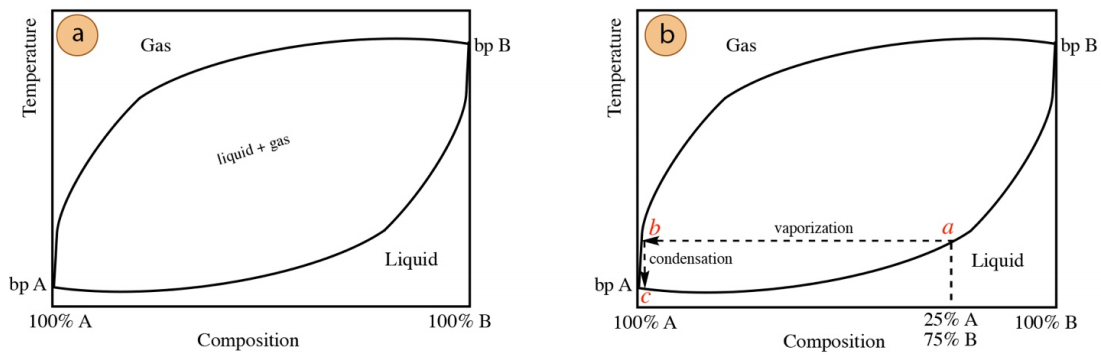
Imagine a \(25 \: \text{mol}\%\) A/\(75 \: \text{mol}\%\) B mixture is to be distilled, and this mixture is described by the distillation curve in Figure 5.14a, where the components have significantly different boiling points. One vaporization-condensation cycle (from a to b to c in Figure 5.14b) will produce a distillate that is nearly \(100\%\) A, the compound with the much lower boiling point. When distilling a mixture with vastly different boiling points, the two components act almost independently and can be easily separated by simple distillation.
Distilling Temperatures
A pure compound distills at a constant temperature, its boiling point. When distilling mixtures, however, the temperature does not often remain steady. This section describes why mixtures distill over a range of temperatures, and the approximate temperatures at which solutions can be expected to boil. These concepts can be understood by examination of the equation that describes a solution's vapor pressure (Equation (6)) and distillation curves.
\[P_\text{solution} = P_A + P_B = P^o_A \chi_A + P^o_B \chi_B \label{6}\]
Imagine an ideal solution that has equimolar quantities of compound "A" (b.p. = \(50^\text{o} \text{C}\)) and compound "B" (b.p. = \(100^\text{o} \text{C}\)). A common misconception is that this solution will boil at \(50^\text{o} \text{C}\),at the boiling point of the lower-boiling compound. The solution does not boil at A's boiling point because A has a reduced partial pressure when part of a solution. In fact, the partial pressure of A at \(50^\text{o} \text{C}\) would be half its normal boiling pressure, as shown in Equation \ref{7}:
\[\text{At } 50^\text{o} \text{C}: \: \: \: \: \: P_A = \left( 760 \: \text{torr} \right) \left( 0.50 \right) = 380 \: \text{torr} \label{7}\]
Since the partial pressures of A and B are additive, the vapor contribution of B at \(50^\text{o} \text{C}\) is also important. The partial pressure of B at \(50^\text{o} \text{C}\) would equal its vapor pressure at this temperature multiplied by its mole fraction (0.50). Since B is below its boiling point \(\left( 100^\text{o} \text{C} \right)\), its vapor pressure would be smaller than \(760 \: \text{torr}\), and the exact value would need to be looked up in a reference book. Let's say that the vapor pressure of B at \(50^\text{o} \text{C}\) is 160 torr.
At 50ºC:
\[ P_B = \left( 160 \: \text{torr} \right) \left( 0.50 \right) = 80 \: \text{torr} \label{8}\]
The solution boils when the combined pressure of the components equals the atmospheric pressure (let's assume \(760 \: \text{torr}\) for this calculation). This equimolar solution would therefore not boil at \(50^\text{o} \text{C}\), as its combined pressure is less than the external pressure of \(760 \: \text{torr}\) (Equation \ref{9}).
At 50 ºC:
\[\begin{align} P_\text{solution} &= P_A + P_B \\[4pt] &= \left( 380 \: \text{torr} \right) + \left( 80 \: \text{torr} \right) \\[4pt] &= 460 \: \text{torr} \label{9} \end{align}\]
The initial boiling point of this solution is \(66^\text{o} \text{C}\), which is the temperature where the combined pressure matches the atmospheric pressure (Equation \ref{10}, note: all vapor pressures would have to be found in a reference book).
At 66 ºC:
\[\begin{align} P_\text{solution} &= P^o_A \chi_A + P^o_B \chi_B \\[4pt] &= \left( 1238 \: \text{torr} \right) \left( 0.5 \right) + \left( 282 \: \text{torr} \right) \left( 0.5 \right) \\[4pt] &= \left( 619 \: \text{torr} \right) + \left( 141 \: \text{torr} \right) = 760 \: \text{torr} \label{10} \end{align}\]
These calculations demonstrate that a solution's boiling point occurs at a temperature between the component's boiling points.\(^5\).
This can also be seen by examination of the distillation curve for this system, where the solution boils when the temperature reaches position a in Figure 5.15a, a temperature between the boiling point of the components.
It is important to realize that mixtures of organic compounds containing similar boiling points (\(\Delta\) b.p. \(< 100^\text{o} \text{C}\)) boil together. They do not act as separate entities (not "A boils at \(50^\text{o} \text{C}\), then B boils at \(100^\text{o} \text{C}\)").
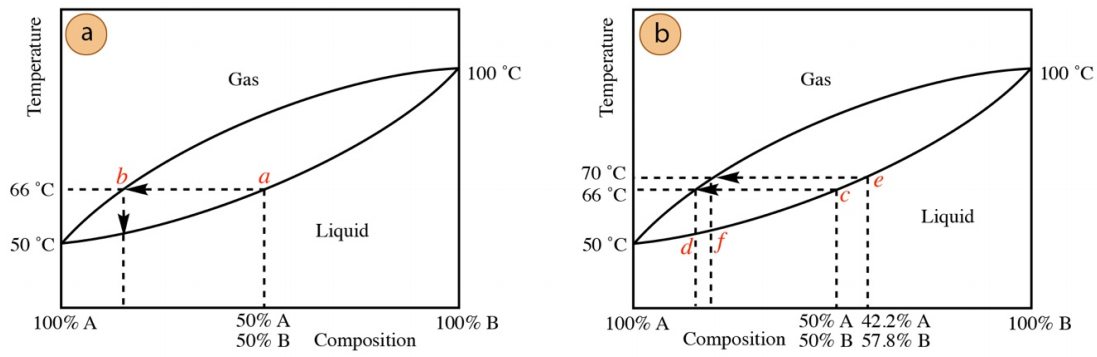
The mixture in this example begins boiling at \(66^\text{o} \text{C}\), but after a period of time boiling would cease if maintained at this temperature. This happens because the composition of the distilling pot changes over time. Since distillation removes more of the lower boiling A, the higher boiling B will increase percentagewise in the distilling pot. Imagine that after some material has distilled, the distilling pot is now \(42.2\%\) A and \(57.8\%\) B. This solution no longer boils at \(66^\text{o} \text{C}\), as shown in Equation \ref{11}.
At 66 ºC:
\[\begin{align} P_\text{solution} &= P^o_A \chi_A + P^o_B \chi_B \\[4pt] &= \left( 1238 \: \text{torr} \right) \left( 0.422 \right) + \left( 282 \: \text{torr} \right) \left( 0.578 \right) \\[4pt] &= \left( 522 \: \text{torr} \right) + \left( 163 \: \text{torr} \right) \\[4pt] &= 685 \: \text{torr} \label{11} \end{align}\]
At the new composition in this example, the temperature must be raised to 70 ºC to maintain boiling, as shown in Equation \ref{12}. This can also be shown by examination of the distillation curve in Figure 5.15b, where boiling commences at temperature c, but must be raised to temperature e as the distilling pot becomes more enriched in the higher boiling component (shifts to the right on the curve).
At 70 ºC:
\[\begin{align} P_\text{solution} &= P^o_A \chi_A + P^o_B \chi_B \\[4pt] &= \left( 1370 \: \text{torr} \right) \left( 0.422 \right) + \left( 315 \: \text{torr} \right) \left( 0.578 \right) \\[4pt] &= \left( 578 \: \text{torr} \right) + \left( 182 \: \text{torr} \right) \\[4pt] &= 760 \: \text{torr} \label{12} \end{align} \]
These calculations and analysis of distillation curves demonstrate why a mixture distills over a range of temperatures:\(^6\) as the composition in the distilling pot changes with time (which affects the mole fraction or the x-axis on the distillation curve), the temperature must be adjusted to compensate. These calculations also imply why a pure compound distills at a constant temperature: the mole fraction is one for a pure liquid, and the distilling pot composition remains unchanged during the distillation.
The calculations and distillation curves in this section enable discussion of another aspect of distillation. The partial pressures of each component at the boiling temperatures can be directly correlated to the composition of the distillate. Therefore, the distillate at \(66^\text{o} \text{C}\) is \(81 \: \text{mol}\%\) A (\(100\% \times 619 \: \text{torr}/760 \: \text{torr}\), see Equation \ref{10}) while the distillate at \(70^\text{o} \text{C}\) is \(76 \: \text{mol}\%\) A (\(100\% \times 578 \: \text{torr}/760 \: \text{torr}\), see Equation \ref{12}). The initial distillate contains the greatest quantity of the lowest boiling component, and the distillate degrades in purity as the distillation progresses. This can also be seen in the distillation curve in Figure 5.15b, where the initial distillate composition corresponds to position d (purer), while the distillate at \(70^\text{o} \text{C}\) corresponds to position f (less pure).
Azeotropes
Pure compounds distill at a constant temperature, while most solutions containing more than one volatile component distill over a range of temperatures. There is an exception to this statement, as some mixtures of certain composition also distill at a constant temperature. These mixtures are called "azeotropes", which display non-ideal behavior and do not follow Raoult's law (Equation \ref{13}).
One of the best-known azeotropes is a mixture containing \(95.6\%\) ethanol and \(4.4\%\) water. When distilling a mixture containing ethanol and water (for example when concentrating fermented grains to produce hard liquor), \(95.6\%\) is the highest percentage of ethanol possible in the distillate. It is impossible to distill \(100\%\) pure ethanol when water is in the distilling pot, as the ethanol and water co-distill acting as a pure substance. For this reason, most ethanol used by chemists is the \(95\%\) version as it can be purified through distillation and is the least expensive. "Absolute ethanol" can also be purchased (which is \(> 99\%\) ethanol), but is more expensive as further methods (e.g. drying agents) are required to remove residual water before or after distillation.
Many compounds form azeotropes, which are specific ratios of compounds that co-distill at a constant composition and temperature. Lange's Handbook of Chemistry\(^7\) lists roughly 860 known azeotropes composed of two or three components each. A selection of these azeotropic mixtures are in Table 5.5.
| Azeotropic Composition | Boiling Point (ºC) |
|---|---|
| 91% Benzene, 9% Water | 69 |
| 92% Cyclohexane, 8% Water | 70 |
| 96% Ethanol, 4% Water | 78 |
| 80% Toluene, 20% Water | 84 |
| 59% Acetone, 41% Hexane | 50 |
| 74% Benzene, 19% Ethanol, 7% Water | 65 |
| 69% Cyclohexane, 31% Ethanol | 65 |
| 68% Benzene, 32% Ethanol | 68 |
| 32% Toluene, 68% Ethanol | 77 |
| 61% Benzene, 39% Methanol | 58 |
| 29% Toluene, 71% Methanol | 64 |
| 83% Ethyl acetate, 9% Water, 8% Ethanol | 70 |
Azeotropes form when a solution deviates from Raoult's law (Equation (13)), meaning that the vapor pressure produced from an azeotropic solution does not directly correlate to its mole fraction. Deviations occur when the components are either attracted to or repelled by one another, namely if they have significantly different strengths of intermolecular forces (IMF's) to themselves (e.g. A-A or B-B) as they do with the other components (e.g. A-B). In other words, a solution will deviate from Raoult's law if the enthalpy of mixing is not zero.
\[P_A = P^o_A \chi_A \label{13}\]
Azeotropic mixtures come in two forms: one whose boiling point is lower than any of its constituents (called a "minimum boiling azeotrope") or ones whose boiling point is higher than any of its constituents (called a "maximum boiling azeotrope"). For example, the \(61\%\) benzene/\(39\%\) methanol azeotrope is a minimum boiling azeotrope as its boiling point is \(58^\text{o} \text{C}\), which is lower than the boiling point of benzene \(\left( 80^\text{o} \text{C} \right)\) and methanol \(\left( 65^\text{o} \text{C} \right)\). Minimum boiling azeotropes are much more common than maximum boiling azeotropes.
Minimum boiling azeotropes occur when the component's IMF's are stronger to themselves (A-A/B-B) than to each other (A-B). This generally occurs with components that lack an affinity for one another, for example when one component can hydrogen bond but the other cannot. In these situations, the components aggregate somewhat in solution, which decreases the solution's entropy and makes it more favorable to become a gas. These solutions therefore have a higher vapor pressure than predicted by Raoult's law, leading to a lower boiling temperature. Maximum boiling azeotropes have the opposite effect, resulting from attractions in the solution that lead to lower vapor pressures than predicted, and thus higher boiling temperatures.
A distillation curve for an ethanol-water mixture is shown in Figure 5.16 (not drawn to scale in order to show detail). The minimum boiling azeotrope is represented by the intersection of the two tear-drop shapes, as indicated in Figure 5.16a. this curve demonstrates why an ethanol-water mixture cannot be distilled to produce a distillate with greater than \(95.6\%\) ethanol. Imagine distilling an ethanol/water mixture of the composition at position a in Figure 5.16b). After each vaporization-condensation event (a to b to c, then c to d to e in Figure 5.16b), the ethanol concentration increases. However, material is always funneled toward the lowest point on the curve, the azeotropic composition. Concentrations higher than \(95.6\%\) ethanol can only be distilled when there is no longer any water in the system (at which point the phase diagram in Figure 5.16 is no longer applicable). Since water forms minimum-boiling azeotropes with many organic compounds, it should be clear why water must always be carefully removed with drying agents before distillation: failure to remove trace water will result in a wet distillate.

\(^3\)Recall that vapor pressure is the partial pressure of a compound formed by evaporation of a pure liquid into the headspace of a closed container.
\(^4\)Data from Handbook of Chemistry and Physics, 50\(^\text{th}\) ed., CRC Press, Cleveland, 1970, p. 148.
\(^5\)Azeotropic solutions do not follow this same pattern, as they do not obey Raoult's law.
\(^6\)Azeotropic solutions again do not follow this generalization, and instead boil at a constant temperature.
\(^7\)J. A. Dean, Lange's Handbook of Chemistry, 15\(^\text{th}\) ed., McGraw-Hill, 1999, Sect. 5.78 and 5.79.


