6.1: Overview of Spectroscopy
- Page ID
- 220541
The focus of this chapter is on the interaction of ultraviolet, visible, and infrared radiation with matter. Because these techniques use optical materials to disperse and focus the radiation, they often are identified as optical spectroscopies. For convenience we will use the simpler term spectroscopy in place of optical spectroscopy; however, you should understand we will consider only a limited piece of what is a much broader area of analytical techniques.
Despite the difference in instrumentation, all spectroscopic techniques share several common features. Before we consider individual examples in greater detail, let’s take a moment to consider some of these similarities. As you work through the chapter, this overview will help you focus on the similarities between different spectroscopic methods of analysis. You will find it easier to understand a new analytical method when you can see its relationship to other similar methods.
What is Electromagnetic Radiation?
Electromagnetic radiation—light—is a form of energy whose behavior is described by the properties of both waves and particles. Some properties of electromagnetic radiation, such as its refraction when it passes from one medium to another (Figure \(\PageIndex{1}\)), are explained best when we describe light as a wave. Other properties, such as absorption and emission, are better described by treating light as a particle. The exact nature of electromagnetic radiation remains unclear, as it has since the development of quantum mechanics in the first quarter of the 20th century [Home, D.; Gribbin, J. New Scientist 1991, 2 Nov. 30–33]. Nevertheless, this dual model of wave and particle behavior provide a useful description for electromagnetic radiation.

Wave Properties of Electromagnetic Radiation
Electromagnetic radiation consists of oscillating electric and magnetic fields that propagate through space along a linear path and with a constant velocity. In a vacuum, electromagnetic radiation travels at the speed of light, c, which is \(2.99792 \times 10^8\) m/s. When electromagnetic radiation moves through a medium other than a vacuum, its velocity, v, is less than the speed of light in a vacuum. The difference between v and c is sufficiently small (<0.1%) that the speed of light to three significant figures, \(3.00 \times 10^8\) m/s, is accurate enough for most purposes.
The oscillations in the electric field and the magnetic field are perpendicular to each other and to the direction of the wave’s propagation. Figure \(\PageIndex{2}\) shows an example of plane-polarized electromagnetic radiation, which consists of a single oscillating electric field and a single oscillating magnetic field.
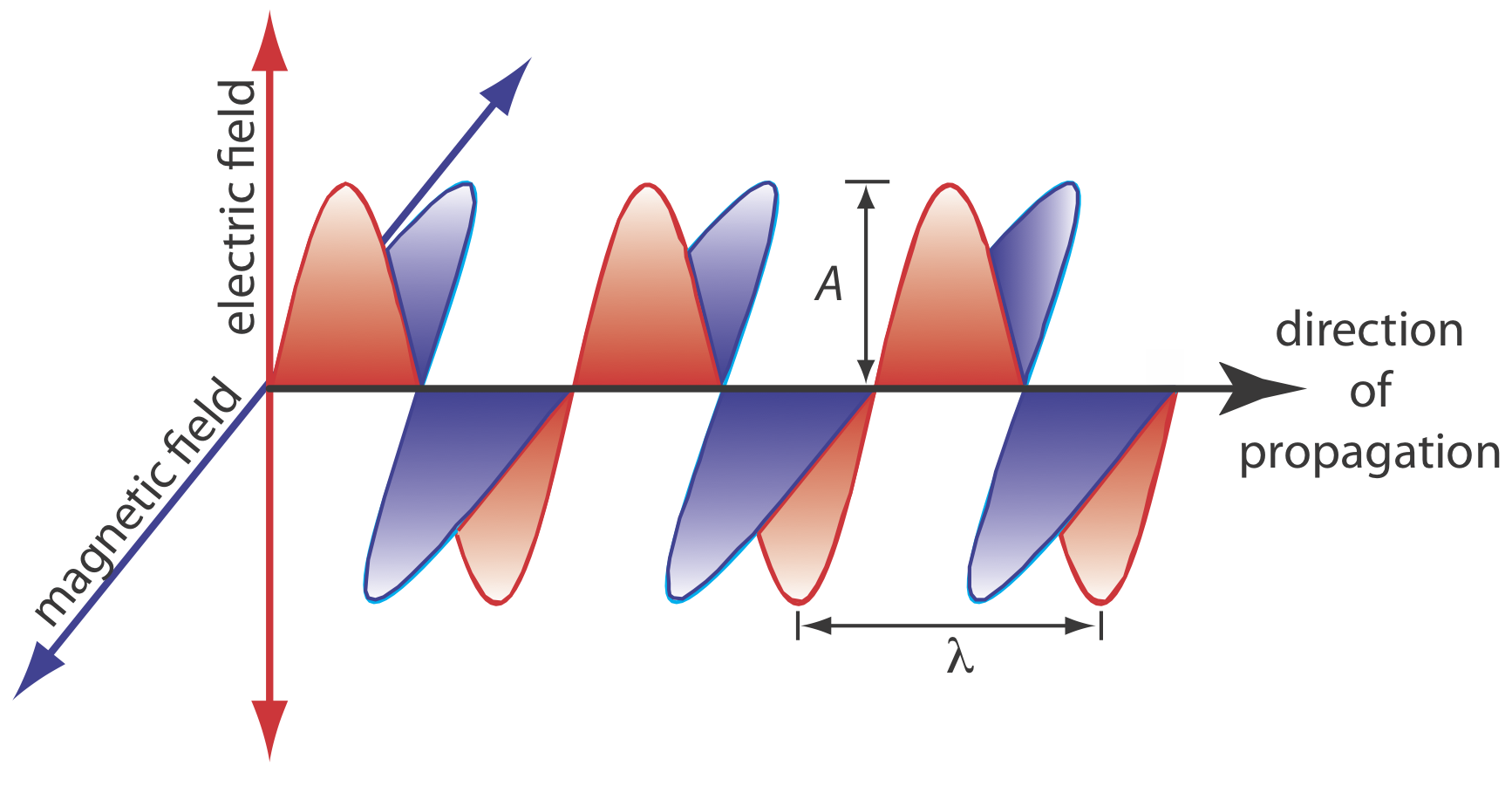
An electromagnetic wave is characterized by several fundamental properties, including its velocity, amplitude, frequency, phase angle, polarization, and direction of propagation [Ball, D. W. Spectroscopy 1994, 9(5), 24–25]. For example, the amplitude of the oscillating electric field at any point along the propagating wave is
\[A_{t}=A_{e} \sin (2 \pi \nu t+\Phi) \nonumber\]
where At is the magnitude of the electric field at time t, Ae is the electric field’s maximum amplitude, \(\nu\) is the wave’s frequency—the number of oscillations in the electric field per unit time—and \(\Phi\) is a phase angle that accounts for the fact that At need not have a value of zero at t = 0. The identical equation for the magnetic field is
\[A_{t}=A_{m} \sin (2 \pi \nu t+\Phi) \nonumber\]
where Am is the magnetic field’s maximum amplitude.
Other properties also are useful for characterizing the wave behavior of electromagnetic radiation. The wavelength, \(\lambda\), is defined as the distance between successive maxima (see Figure \(\PageIndex{2}\)). For ultraviolet and visible electromagnetic radiation the wavelength usually is expressed in nanometers (1 nm = 10–9 m), and for infrared radiation it is expressed in microns (1 mm = 10–6 m). The relationship between wavelength and frequency is
\[\lambda = \frac {c} {\nu} \nonumber\]
Another unit useful unit is the wavenumber, \(\overline{\nu}\), which is the reciprocal of wavelength
\[\overline{\nu} = \frac {1} {\lambda} \nonumber\]
Wavenumbers frequently are used to characterize infrared radiation, with the units given in cm–1.
When electromagnetic radiation moves between different media—for example, when it moves from air into water—its frequency, \(\nu\), remains constant. Because its velocity depends upon the medium in which it is traveling, the electromagnetic radiation’s wavelength, \(\lambda\), changes. If we replace the speed of light in a vacuum, c, with its speed in the medium, \(v\), then the wavelength is
\[\lambda = \frac {v} {\nu} \nonumber\]
This change in wavelength as light passes between two media explains the refraction of electromagnetic radiation shown in Figure \(\PageIndex{1}\).
In 1817, Josef Fraunhofer studied the spectrum of solar radiation, observing a continuous spectrum with numerous dark lines. Fraunhofer labeled the most prominent of the dark lines with letters. In 1859, Gustav Kirchhoff showed that the D line in the sun’s spectrum was due to the absorption of solar radiation by sodium atoms. The wavelength of the sodium D line is 589 nm. What are the frequency and the wavenumber for this line?
Solution
The frequency and wavenumber of the sodium D line are
\[\nu=\frac{c}{\lambda}=\frac{3.00 \times 10^{8} \ \mathrm{m} / \mathrm{s}}{589 \times 10^{-9} \ \mathrm{m}}=5.09 \times 10^{14} \ \mathrm{s}^{-1} \nonumber\]
\[\overline{\nu}=\frac{1}{\lambda}=\frac{1}{589 \times 10^{-9} \ \mathrm{m}} \times \frac{1 \ \mathrm{m}}{100 \ \mathrm{cm}}=1.70 \times 10^{4} \ \mathrm{cm}^{-1} \nonumber\]
Another historically important series of spectral lines is the Balmer series of emission lines from hydrogen. One of its lines has a wavelength of 656.3 nm. What are the frequency and the wavenumber for this line?
- Answer
-
The frequency and wavenumber for the line are
\[\nu=\frac{c}{\lambda}=\frac{3.00 \times 10^{8} \ \mathrm{m} / \mathrm{s}}{656.3 \times 10^{-9} \ \mathrm{m}}=4.57 \times 10^{14} \ \mathrm{s}^{-1} \nonumber\]
\[\overline{\nu}=\frac{1}{\lambda}=\frac{1}{656.3 \times 10^{-9} \ \mathrm{m}} \times \frac{1 \ \mathrm{m}}{100 \ \mathrm{cm}}=1.524 \times 10^{4} \ \mathrm{cm}^{-1} \nonumber\]
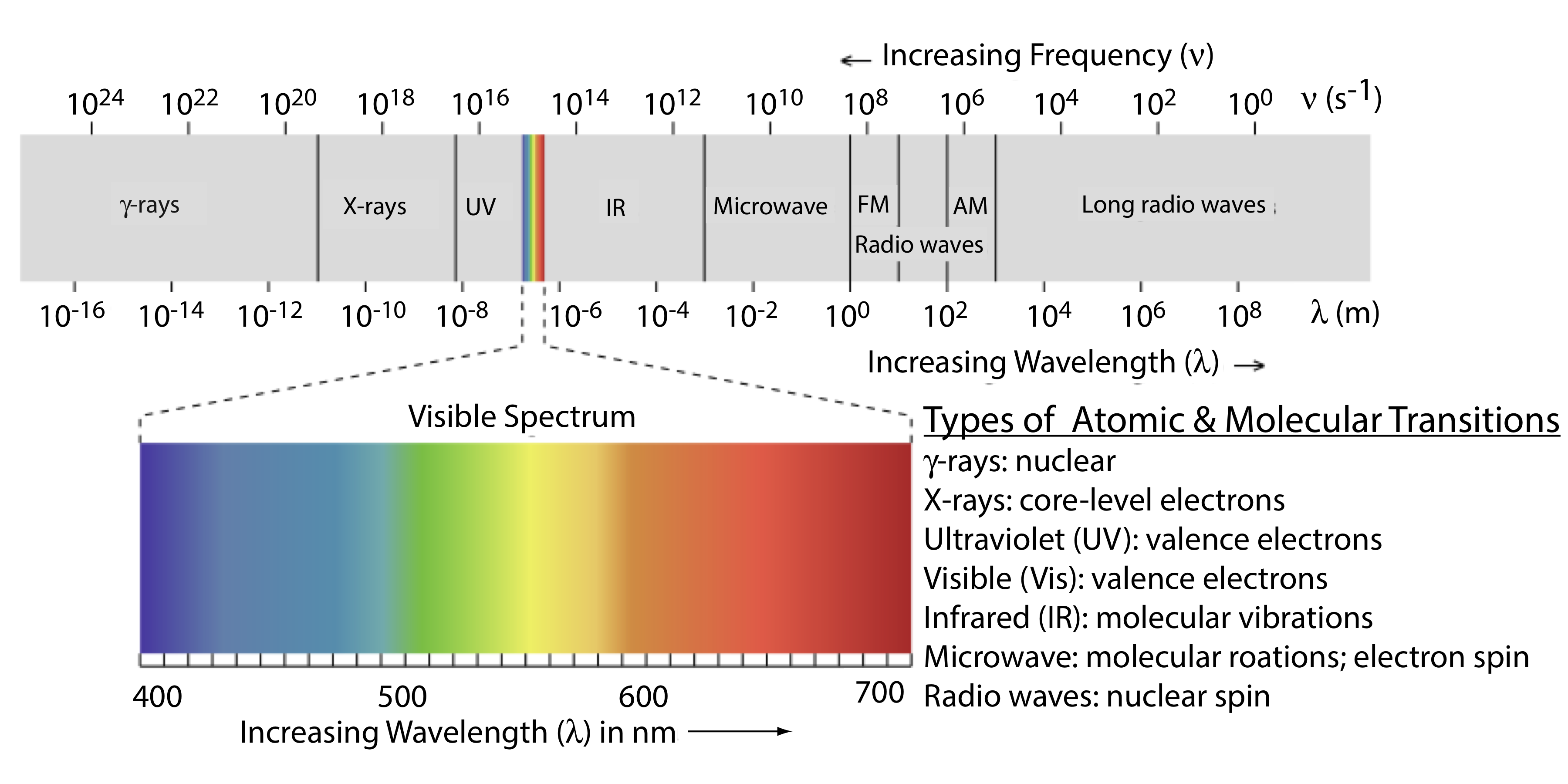
The Electromagnetic Spectrum
The frequency and the wavelength of electromagnetic radiation vary over many orders of magnitude. For convenience, we divide electromagnetic radiation into different regions—the electromagnetic spectrum—based on the type of atomic or molecular transitions that gives rise to the absorption or emission of photons (Figure \(\PageIndex{3}\)). The boundaries between the regions of the electromagnetic spectrum are not rigid and overlap between spectral regions is possible.