6.5: The Sₙ2 Reaction
- Last updated
- Save as PDF
- Page ID
- 182904
Learning Objectives
- determine the rate law & predict the mechanism based on its rate equation or reaction data for SN2 reactions
- propose mechanisms for SN2 reactions
- draw and interpret Reaction Energy Diagrams for SN2 reactions
Introduction
In many ways, the proton transfer process of a Brønsted-Lowry acid-base reaction can be thought of as simply a special kind of nucleophilic substitution reaction, one in which the electrophile is a hydrogen rather than a carbon.
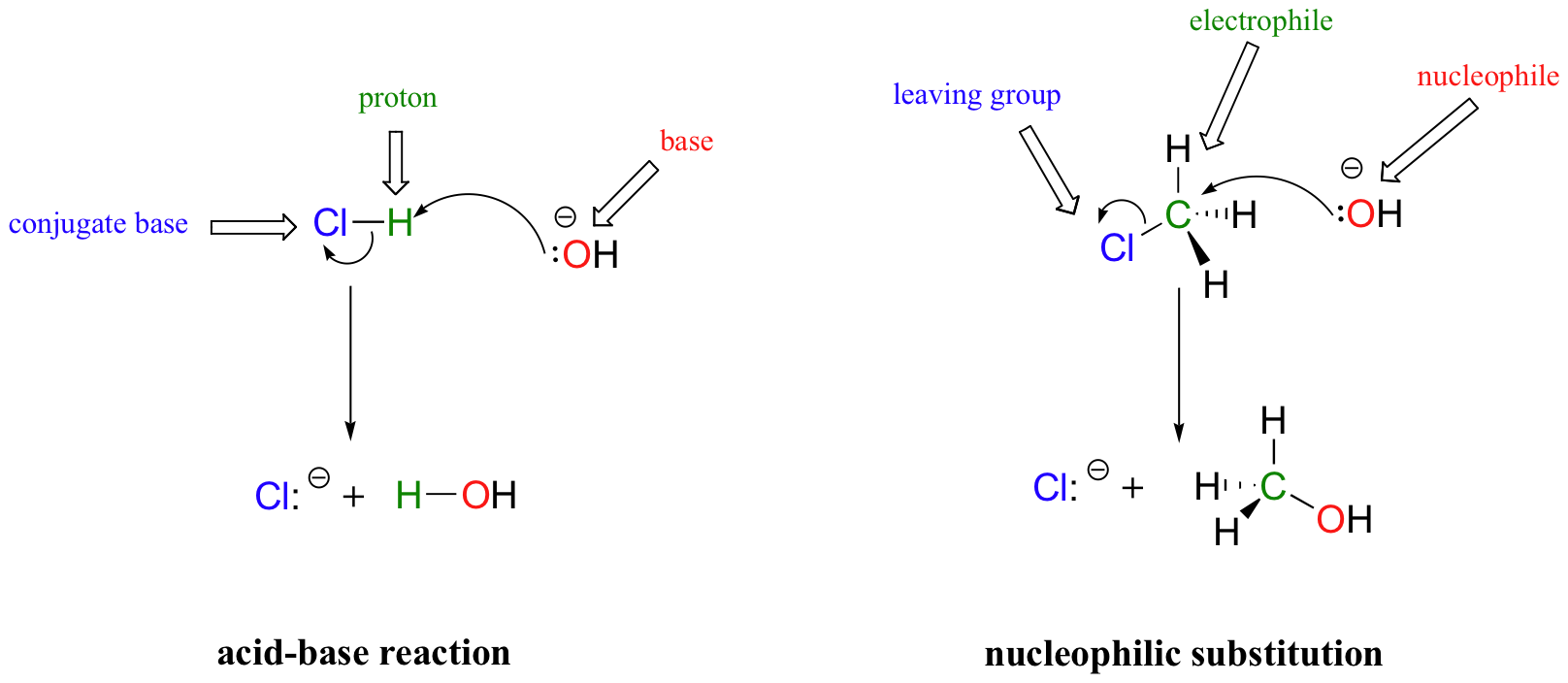
In both reaction types, we are looking at very similar players: an electron-rich species (the nucleophile/base) reacts with an electron-poor species (the electrophile/proton), driving off the leaving group/conjugate base.
In the next few sections, we are going to be discussing some general aspects of nucleophilic substitution reactions, and in doing so it will simplify things greatly if we can use some abbreviations and generalizations before we dive into real examples.
What is a nucleophile (Nu)?
Instead of showing a specific nucleophile like hydroxide, we will simply refer to the nucleophilic reactant as 'Nu'. Nucleophilic functional groups are those which have electron-rich atoms able to donate a pair of electrons to form a new covalent bond. Nucleophiles can be negatively charged and some that are neutral with lone pair electrons. In both laboratory and biological organic chemistry, the most relevant nucleophilic atoms are oxygen, nitrogen, and sulfur, and the most common nucleophilic functional groups are water, alcohols, phenols, amines, thiols, and occasionally carboxylates.
More specifically in laboratory reactions, halide and azide (N3-) anions are commonly seen acting as nucleophiles.
When thinking about nucleophiles, the first thing to recognize is that, for the most part, the same quality of 'electron-richness' that makes a something nucleophilic also makes it basic: nucleophiles can be bases, and bases can be nucleophiles. It should not be surprising, then, that most of the trends in basicity that we have already discussed also apply to nucleophilicity.
Some confusion in distinguishing basicity (base strength) and nucleophilicity (nucleophile strength) is inevitable. Since basicity is a less troublesome concept; it is convenient to start with it. Basicity refers to the ability of a base to accept a proton. Basicity may be related to the pKa of the corresponding conjugate acid, as shown below. The strongest bases have the weakest conjugate acids and vice versa. The range of basicities included in the following table is remarkable, covering over fifty powers of ten!
In an acid-base equilibrium the weakest acid and the weakest base will predominate (they will necessarily be on the same side of the equilibrium). Learning the pKa values for common compounds provides a useful foundation on which to build an understanding of acid-base factors in reaction mechanisms.
| Base | I (–) | Cl (–) | H2O | CH3CO2(–) | RS(–) | CN(–) | RO(–) | NH2(–) | CH3(–) |
|---|---|---|---|---|---|---|---|---|---|
| Conj. Acid | HI | HCl | H3O(+) | CH3CO2H | RSH | HCN | ROH | NH3 | CH4 |
| pKa | -9 | -7 | -1.7 | 4.8 | 8 | 9.1 | 16 | 33 | 48 |

Nucleophilicity is a more complex property. It commonly refers to the rate of substitution reactions at the halogen-bearing carbon atom of a reference alkyl halide, such as CH3-Br. Thus the nucleophilicity of the Nu:(–) reactant in the following substitution reaction varies as shown in the chart below:
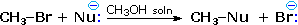 |
|---|
|
Nucleophilicity: CH3CO2 (–) < Cl(–) < Br(–) < N3(–) < CH3O(–) < CN(–) < I(–) < CH3S(–) |
 |
What is a Leaving Group (X or LG)?
In a similar fashion, we will call the leaving group 'X' for halogens as is customary. For other reactions, it will be more accurate to abbreviate the leaving group as "LG". The context of the reaction will dictate the abbreviation. Leaving groups are sometimes negatively charged, sometimes neutral, and sometimes positively charged. Therefore, in this general picture we will not include a charge designation on the 'X' or 'LG' species. In referring to the comparison between acid-base chemistry and substitution reactions, the stability of the leaving group is evaluated the same way we evaluate the stability of conjugate bases.
When comparing the reactivity of electrophiles that vary only in their leaving groups, then leaving group stability plays a dominant role. The electrophile with the more stable leaving group will be favored. The lower the electron density of the leaving group, the more stable it is. Neutral leaving groups are favoring over charged leaving groups. When comparing charged leaving groups, apply the concepts used to determine the relative stability of conjugate bases:
1) identity or identities of the atom(s) holding the charge
2) delocalization of the charge via resonance
3) inductive effects
4) orbital hybridization
What is an Electrophile (E)?
An electrophile accepts electrons analogous to a Lewis acid. Electrophiles (E) are sometimes protonated and sometimes neutral. Electrophiles can also be called "Substrates". Since nucleophiles, leaving groups, and electrons may be charged or neutral, we will not include charges on 'Nu' or 'X' (or 'LG') or 'E'.
We will generalize the three other groups bonded on the electrophilic alpha-carbon as R1, R2, and R3: these symbols could represent hydrogens as well as alkyl groups. Finally, in order to keep figures from becoming too crowded, we will use in most cases the line structure convention in which the central, electrophilic carbon is not drawn out as a 'C'.
Here, then, is the generalized picture of a concerted (single-step) nucleophilic substitution reaction:
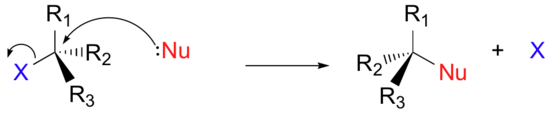
To recognize neutral electrophiles, we will need to identify polarity and/or resonance with compounds to create a partial positive charge to attract the nucleophile. The electrophilicity of alkyl halides comes from the polar carbon-halogen bond.
The common halogens being fluorine, chlorine, bromine and iodine. With the exception of iodine, these halogens have electronegativities significantly greater than carbon. Consequently, this functional group is polarized so that the carbon is electrophilic and the halogen is nucleophilic, as shown in the drawing on the right. Two characteristics other than electronegativity also have an important influence on the chemical behavior of these compounds. The first of these is covalent bond strength. The strongest of the carbon-halogen covalent bonds is that to fluorine. Remarkably, this is the strongest common single bond to carbon, being roughly 30 kcal/mole stronger than a carbon-carbon bond and about 15 kcal/mole stronger than a carbon-hydrogen bond. Because of this, alkyl fluorides and fluorocarbons in general are chemically and thermodynamically quite stable, and do not share any of the reactivity patterns shown by the other alkyl halides. The carbon-chlorine covalent bond is slightly weaker than a carbon-carbon bond, and the bonds to the other halogens are weaker still, the bond to iodine being about 33% weaker. The second factor to be considered is the relative stability of the corresponding halide anions, which is likely the form in which these electronegative atoms will be replaced. This stability may be estimated from the relative acidities of the H-X acids, assuming that the strongest acid releases the most stable conjugate base (halide anion). With the exception of HF (pKa = 3.2), all the hydrohalic acids are very strong, small differences being in the direction HCl < HBr < HI.
Exercise
1. Since everything is relative in chemistry, one reaction's nucleophile can be another reaction's leaving group. Some functional groups can only react as a nuclephile or electrophile, while other functional groups can react as either a nuclephile or electrophile depending on the reaction conditions. Classify the following compounds as nucleophiles, electrophiles, or leaving groups. More than one answer may be possible.
a) bromoethane
b) hydroxide
c) water
d) chlorocyclohexane
e) ethanol
f) bromide
- Answer
-
a) electrophile (Alkyl halides are always electrophiles - one reason they are an o-chem student's best friend.)
b) strong nucleophile
c) weak nucleophile and good leaving group
d) electrophile (Alkyl halides are always electrophiles - one reason they are an o-chem student's best friend.)
e) weak nucleophile, a poor electrophile without clever chemistry (stay tuned for future chapters), good leaving group
f) good nucleophile and a good leaving group
The SN2 mechanism
There are two mechanistic models for how an alkyl halide can undergo nucleophilic substitution, SN2 and SN1. The SN2 reaction takes place in a single step with bond-forming and bond-breaking occurring simultaneously. (In all figures in this section, 'X' indicates a halogen substituent).
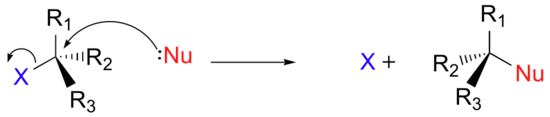
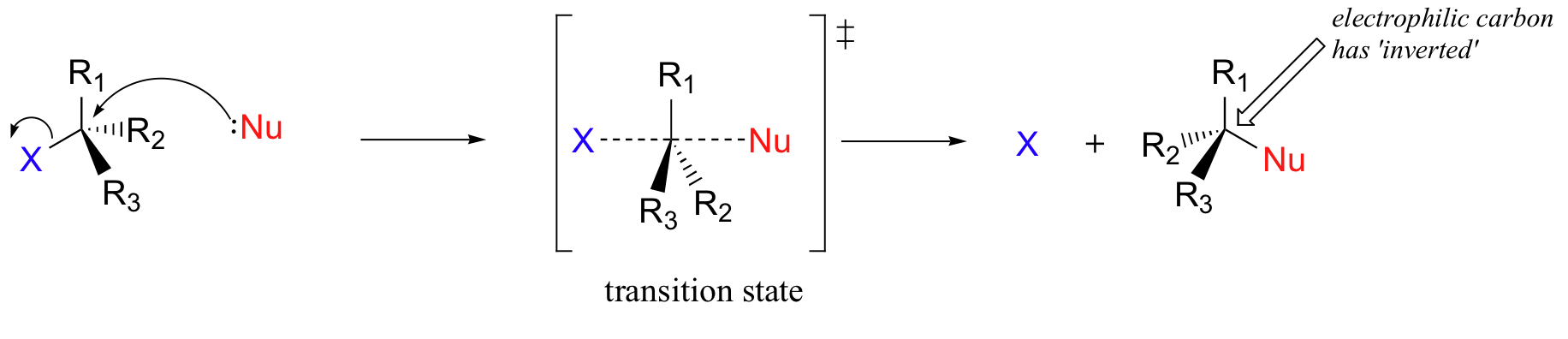
This is called an 'SN2' mechanism. In the term SN2, S stands for 'substitution', the subscript N stands for 'nucleophilic', and the number 2 refers to the fact that it is a bimolecular reaction: the overall rate depends on a step in which two separate molecules (the nucleophile and the electrophile) collide. A potential energy diagram for this reaction shows the transition state (TS) as the highest point on the pathway from reactants to products.
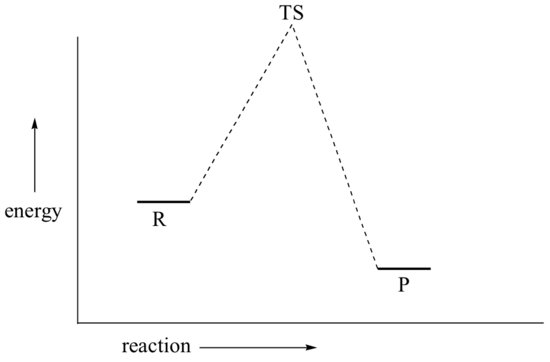
If you look carefully at the progress of the SN2 reaction, you will realize something very important about the outcome. The nucleophile, being an electron-rich species, must react with the electrophilic carbon from the back side relative to the location of the leaving group. Approach from the front side simply doesn't work: the electron rich, leaving group blocks the way with electrostatic repulsion and steric hindrance.
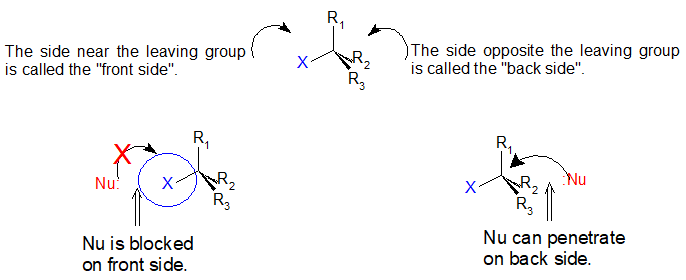
The result of this backside penetration is that the stereochemical configuration at the central carbon inverts as the reaction proceeds. In a sense, the molecule is turned inside out. At the transition state, the electrophilic carbon and the three 'R' substituents all lie on the same plane.
What this means is that SN2 reactions whether enzyme catalyzed or not, are inherently stereoselective: when the substitution takes place at a stereocenter, we can confidently predict the stereochemical configuration of the product. Below is an animation illustrating the principles we have just learned, showing the SN2 reaction between hydroxide ion and methyl iodide. Notice how backside attack by the hydroxide nucleophile results in inversion at the tetrahedral carbon electrophile.
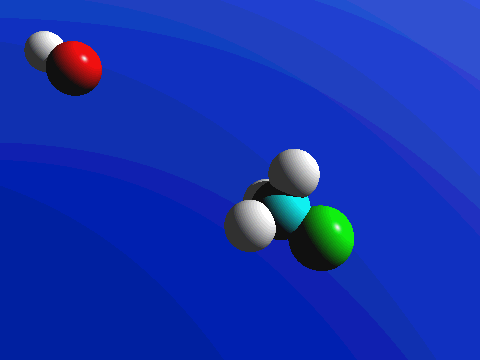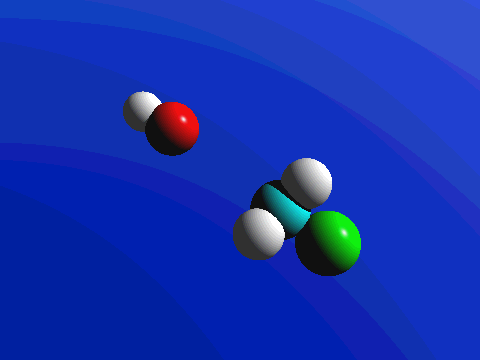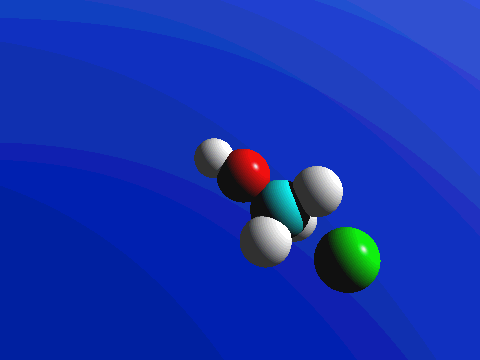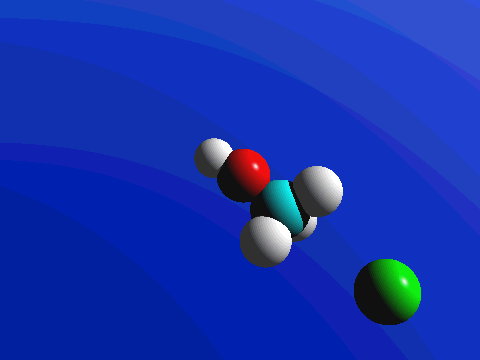
Exercise
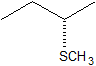
2. Predict the structure of the product in this SN2 reaction. Be sure to specify stereochemistry.
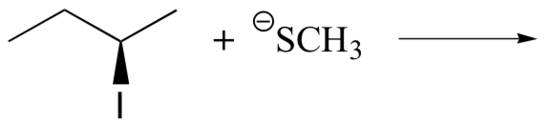
Solution
2.
SN2 Reactions Occur at sp3 Carbons with a Leaving Group
One more important point must be made before continuing: nucleophilic substitutions as a rule occur at sp3-hybridized carbons bonded to a leaving group. SN2 reactions cannot occur where the leaving group is attached to an sp2-hybridized carbon:
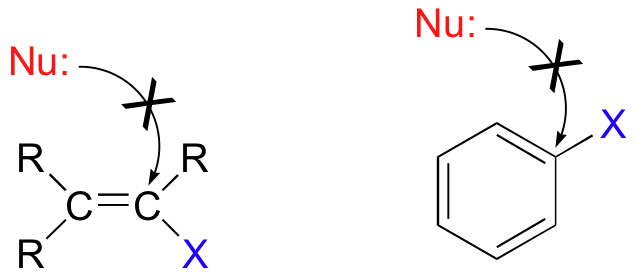
Bonds on sp2-hybridized carbons are inherently shorter and stronger than bonds on sp3-hybridized carbons, meaning that it is harder to break the C-X bond in these substrates. SN2 reactions of this type are unlikely also because the (hypothetical) electrophilic carbon is protected from nucleophilic attack by electron density in the p bond. SN1 reactions are highly unlikely, because the resulting carbocation intermediate, which would be sp-hybridized, would be very unstable (we’ll discuss the relative stability of carbocation intermediates in a later section of this module).
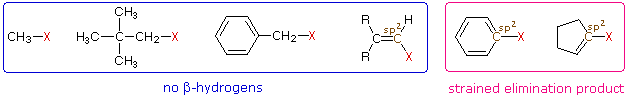
For future reference when discerning between substitution and elimination reactions, evaluating the structure of the electrophile can eliminate possible products. If the electrophilic carbon has no beta-hydrogens, then only substitution reactions can occur and elimination reactions are not possible (of course carbocation rearrangements may need to be considered). The first four halides shown on the left below do not give elimination reactions on treatment with base, because they have no β-hydrogens. The two halides on the right do not normally undergo such reactions because the potential elimination products have highly strained double or triple bonds. It is also worth noting that sp2 hybridized C–X compounds, such as the three on the right, do not normally undergo nucleophilic substitution reactions, unless other functional groups perturb the double bond(s).
Exercise
3. Predict which alkyl halides can undergo a SN2 reaction.
a) C6H5Br
b) CH3CH2CH2Br
c) CH2CHBr
d) CH3CH2CH2CHBrCH3
- Solutions
- 3.
- a) No, aryl halide.
- b) Yes, primary alkyl halide
- c) No, vinyl halide
- d) Yes, secondary alkyl halide
SN2 Reaction Kinetics
In the term SN2, the S stands for substitution, the N stands for nucleophilic, and the number two stands for bimolecular, meaning there are two molecules involved in the rate determining step. The rate of bimolecular nucleophilic substitution reactions depends on the concentration of both the haloalkane and the nucleophile. To understand how the rate depends on the concentrations of both the haloalkane and the nucleophile, let us look at the following example. The hydroxide ion is the nucleophile and methyl iodide is the haloalkane.
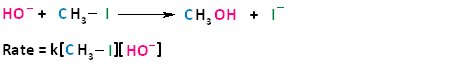
If we were to double the concentration of either the haloalkane or the nucleophile, we can see that the rate of the reaction would proceed twice as fast as the initial rate.
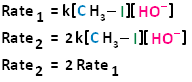
If we were to double the concentration of both the haloalkane and the nucleophile, we can see that the rate of the reaction would proceed four times as fast as the initial rate.
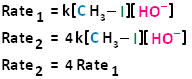
The bimolecular nucleophilic substitution reaction follows second-order kinetics; that is, the rate of the reaction depends on the concentration of two first-order reactants. In the case of bimolecular nucleophilic substitution, these two reactants are the haloalkane and the nucleophile. For further clarification on reaction kinetics, the following links may facilitate your understanding of rate laws, rate constants, and second-order kinetics
Exercise
4. The reaction below follows the SN2 mechanism.
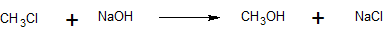
a) Write the rate law for this reaction.
b) Determine the value of the rate coefficient, k, if the initial concentrations are 0.01 M CH3Cl, 0.01 M NaOH, and the initial reaction rate is 6 x 10-10 M/s.
c) Calculate the initial reaction rate if the initial reactant concentrations are changed to 0.02 M CH3Cl and 0.0005 M NaOH.
- Solutions
-
4.
a) rate = k [CH3Cl] [OH-]
b) substitute the data into the rate expression above and apply algebra to solve for k
k = 6 x 10-6 Lmol-1s-1
c) Using the rate law above, substitute the value for k from the previous question along with the new concentrations to determine the new initial rate.
rate = 6 x 10-10 M/s
Contributors and Attributions
Dr. Dietmar Kennepohl FCIC (Professor of Chemistry, Athabasca University)
Prof. Steven Farmer (Sonoma State University)
William Reusch, Professor Emeritus (Michigan State U.), Virtual Textbook of Organic Chemistry
Organic Chemistry With a Biological Emphasis by Tim Soderberg (University of Minnesota, Morris)

