5.4 A Molecular View of Phases
- Page ID
- 218383
Skills to Develop
- To be familiar with the kinetic molecular description of liquids.
The physical properties of a substance depends upon its physical state. Water vapor, liquid water and ice all have the same chemical properties, but their physical properties are considerably different. In general Covalent bonds determine: molecular shape, bond energies, chemical properties, while intermolecular forces (non-covalent bonds) influence the physical properties of liquids and solids. The kinetic molecular theory of gases gives a reasonably accurate description of the behavior of gases. A similar model can be applied to liquids, but it must take into account the nonzero volumes of particles and the presence of strong intermolecular attractive forces.

Figure \(\PageIndex{1}\): The four fundamental states of matter. Clockwise from top left, they are solid, liquid, plasma, and gas, represented by an ice sculpture, a drop of water, electrical arcing from a tesla coil, and the air around clouds, respectively. Images used with permission from Wikipedia.
The state of a substance depends on the balance between the kinetic energy of the individual particles (molecules or atoms) and the intermolecular forces. The kinetic energy keeps the molecules apart and moving around, and is a function of the temperature of the substance and the intermolecular forces try to draw the particles together (Table \(\PageIndex{2}\)). A discussed previously, gasses are very sensitive to temperatures and pressure. However, these also affect liquids and solids too. Heating and cooling can change the kinetic energy of the particles in a substance, and so, we can change the physical state of a substance by heating or cooling it. Increasing the pressure on a substance forces the molecules closer together, which increases the effectiveness of intermolecular forces
 |
 |
 |
| (a) in the gaseous state | (b) as a liquid | (c) in solid form |
Below is an overview of the general properties of the three different phases of matter.
Properties of Gases
- A collection of widely separated molecules
- The kinetic energy of the molecules is greater than any attractive forces between the molecules
- The lack of effective attractive force between molecules allows a gas to expand to fill its container
- If attractive forces become large enough, then the gases exhibit non-ideal behavior
Properties of Liquids
- The intermolecular attractive forces are strong enough to hold molecules close together
- Liquids are more dense and less compressible than gases
- Liquids have a definite volume, independent of the size and shape of their container
- The attractive forces are not strong enough, however, to keep neighboring molecules in a fixed position and molecules are free to move past or slide over one another
Thus, liquids can be poured and assume the shape of their containers
Properties of Solids
- The intermolecular forces between neighboring molecules are strong enough to keep them locked in relatively stable positions
- Solids (like liquids) are not very compressible due to the lack of space between molecules
- If the molecules in a solid adopt a highly ordered packing arrangement, the structures are said to be crystalline
Due to the strong intermolecular forces between neighboring molecules, solids are rigid
- Cooling a gas may change the state to a liquid
- Cooling a liquid may change the state to a solid
- Increasing the pressure on a gas may change the state to a liquid
- Increasing the pressure on a liquid may change the state to a solid
Video \(\PageIndex{1}\): Video highlighting the properties for the three states of matter. Source found at https://www.youtube.com/watch?v=s-KvoVzukHo.
Physical Properties of Liquids
In a gas, the distance between molecules, whether monatomic or polyatomic, is very large compared with the size of the molecules; thus gases have a low density and are highly compressible. In contrast, the molecules in liquids are very close together, with essentially no empty space between them. As in gases, however, the molecules in liquids are in constant motion, and their kinetic energy (and hence their speed) depends on their temperature. We begin our discussion by examining some of the characteristic properties of liquids to see how each is consistent with a modified kinetic molecular description.
The properties of liquids can be explained using a modified version of the kinetic molecular theory of gases described previously This model explains the higher density, greater order, and lower compressibility of liquids versus gases; the thermal expansion of liquids; why they diffuse; and why they adopt the shape (but not the volume) of their containers. A kinetic molecular description of liquids must take into account both the nonzero volumes of particles and the presence of strong intermolecular attractive forces. Solids and liquids have particles that are fairly close to one another, and are thus called "condensed phases" to distinguish them from gases
- Density: The molecules of a liquid are packed relatively close together. Consequently, liquids are much denser than gases. The density of a liquid is typically about the same as the density of the solid state of the substance. Densities of liquids are therefore more commonly measured in units of grams per cubic centimeter (g/cm3) or grams per milliliter (g/mL) than in grams per liter (g/L), the unit commonly used for gases.
- Molecular Order: Liquids exhibit short-range order because strong intermolecular attractive forces cause the molecules to pack together rather tightly. Because of their higher kinetic energy compared to the molecules in a solid, however, the molecules in a liquid move rapidly with respect to one another. Thus unlike the ions in the ionic solids, the molecules in liquids are not arranged in a repeating three-dimensional array. Unlike the molecules in gases, however, the arrangement of the molecules in a liquid is not completely random.
- Compressibility: Liquids have so little empty space between their component molecules that they cannot be readily compressed. Compression would force the atoms on adjacent molecules to occupy the same region of space.
- Thermal Expansion: The intermolecular forces in liquids are strong enough to keep them from expanding significantly when heated (typically only a few percent over a 100°C temperature range). Thus the volumes of liquids are somewhat fixed. Notice from Table S1 (with a shorten version in Table \(\PageIndex{1}\)) that the density of water, for example, changes by only about 3% over a 90-degree temperature range.
| T (°C) | Density (g/cm3) |
|---|---|
| 0 | 0.99984 |
| 30 | 0.99565 |
| 60 | 0.98320 |
| 90 | 0.96535 |
-
Diffusion: Molecules in liquids diffuse because they are in constant motion. A molecule in a liquid cannot move far before colliding with another molecule, however, so the mean free path in liquids is very short, and the rate of diffusion is much slower than in gases.
-
Fluidity: Liquids can flow, adjusting to the shape of their containers, because their molecules are free to move. This freedom of motion and their close spacing allow the molecules in a liquid to move rapidly into the openings left by other molecules, in turn generating more openings, and so forth (Figure 11.1.3).
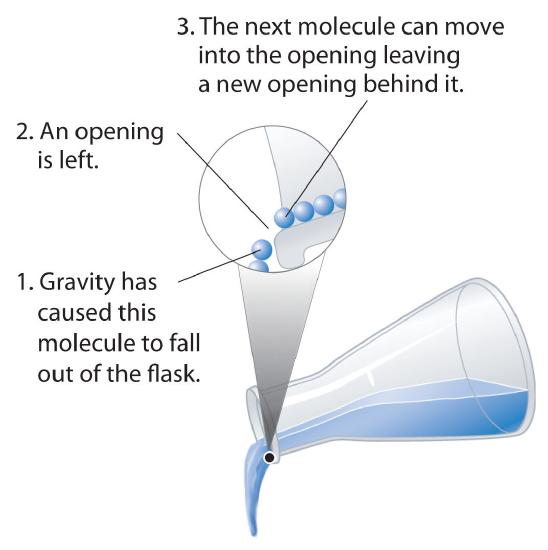
Figure \(\PageIndex{3}\): Why Liquids Flow. Molecules in a liquid are in constant motion. Consequently, when the flask is tilted, molecules move to the left and down due to the force of gravity, and the openings are occupied by other molecules. The result is a net flow of liquid out of the container.
Contributors
- Modified by Tom Neils (Grand Rapids Community College)

