12.2 Natural Radioactivity
- Page ID
- 218441
In a nuclear decay reaction, also called radioactive decay, an unstable nucleus emits radiation and is transformed into the nucleus of one or more other elements. The resulting daughter nuclei have a lower mass and are lower in energy (more stable) than the parent nucleus that decayed. We begin this section by considering the different classes of radioactive nuclei, along with their characteristic nuclear decay reactions and the radiation they emit.
Nuclear decay reactions occur spontaneously under all conditions.
Nuclear Decay Reactions
Just as we use the number and type of atoms present to balance a chemical equation, we can use the number and type of nucleons present to write a balanced nuclear equation for a nuclear decay reaction. This procedure also allows us to predict the identity of either the parent or the daughter nucleus if the identity of only one is known. Regardless of the mode of decay, the total number of nucleons is conserved in all nuclear reactions.
To describe nuclear decay reactions, chemists have extended the \(^A _Z \textrm{X}\)
| Identity | Symbol | Charge | Mass (amu) |
|---|---|---|---|
| helium nucleus | \(^4_2\alpha\) | +2 | 4.001506 |
| electron | \(^0_{-1}\beta\) or \(\beta ^-\) | −1 | 0.000549 |
| photon | \(_0^0\gamma\) | — | — |
| neutron | \(^1_0\textrm n\) | 0 | 1.008665 |
| proton | \(^1_1\textrm p \) | +1 | 1.007276 |
| positron | \(^0_{+1}\beta\) or \(\beta ^+\) | +1 | 0.000549 |
Like the notation used to indicate isotopes, the upper left superscript in the symbol for a particle gives the mass number, which is the total number of protons and neutrons. For a proton or a neutron, A = 1. Because neither an electron nor a positron contains protons or neutrons, its mass number is 0. The numbers should not be taken literally, however, as meaning that these particles have zero mass; ejection of a beta particle (an electron) simply has a negligible effect on the mass of a nucleus.
Similarly, the lower left subscript gives the charge of the particle. Because protons carry a positive charge, Z = +1 for a proton. In contrast, a neutron contains no protons and is electrically neutral, so Z = 0. In the case of an electron, Z = −1, and for a positron, Z = +1. Because γ rays are high-energy photons, both A and Z are 0. In some cases, two different symbols are used for particles that are identical but produced in different ways. For example, the symbol
There are six fundamentally different kinds of nuclear decay reactions, and each releases a different kind of particle or energy. The essential features of each reaction are shown in Figure \(\PageIndex{1}\). The most common are alpha and beta decay and gamma emission, but the others are essential to an understanding of nuclear decay reactions.
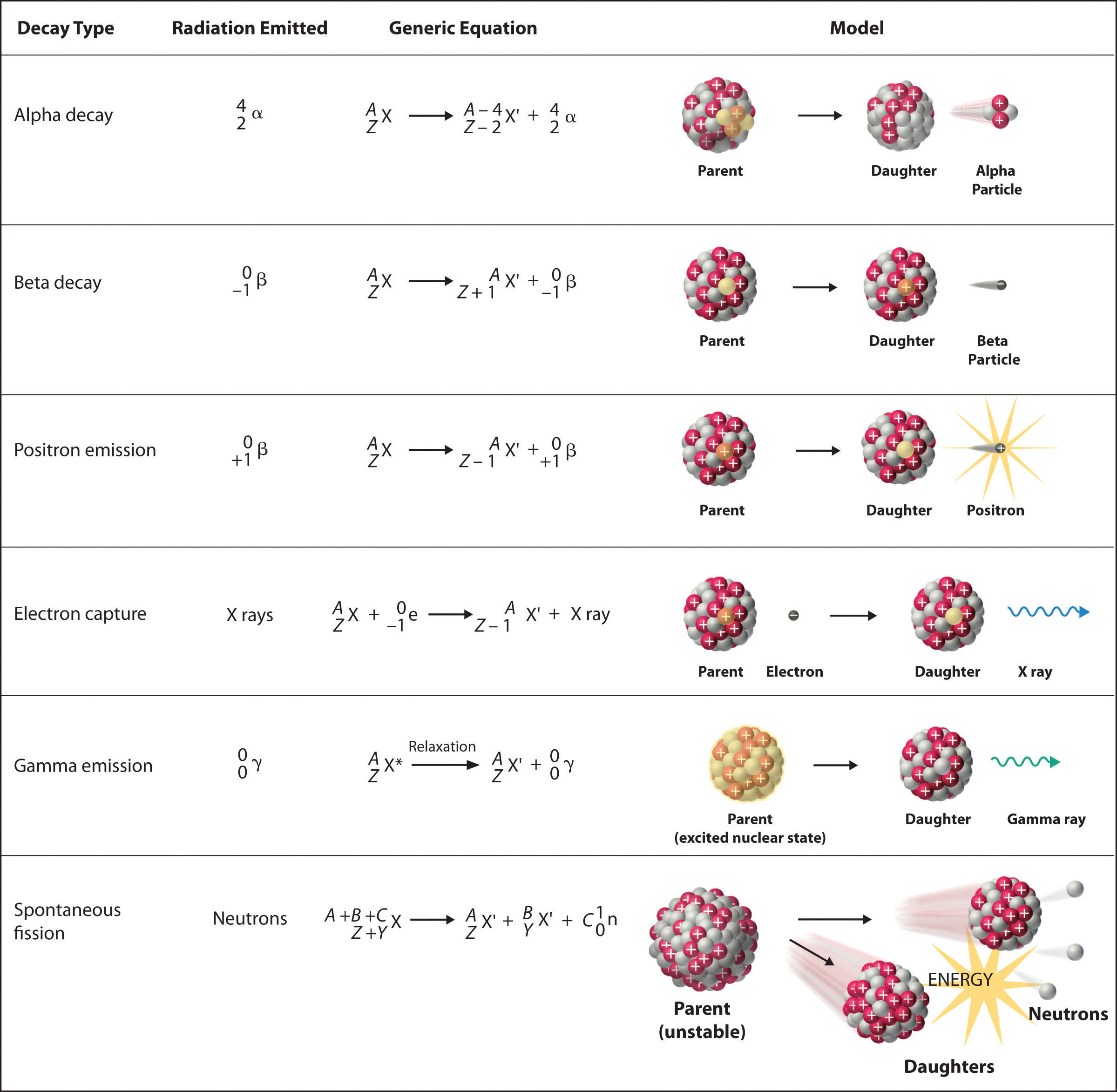
Figure \(\PageIndex{1}\): Common Modes of Nuclear Decay
Alpha \(\alpha\)Decay
Many nuclei with mass numbers greater than 200 undergo alpha (α) decay, which results in the emission of a helium-4 nucleus as an alpha (α) particle,
\[\underset{\textrm{parent}}{^A_Z \textrm X}\rightarrow \underset{\textrm{daughter}}{^{A-4}_{Z-2} \textrm X'}+\underset{\textrm{alpha}\\ \textrm{particle}}{^4_2 \alpha}\label{Eq1}\]
The daughter nuclide contains two fewer protons and two fewer neutrons than the parent. Thus α-particle emission produces a daughter nucleus with a mass number A − 4 and a nuclear charge Z − 2 compared to the parent nucleus. Radium-226, for example, undergoes alpha decay to form radon-222:
\[^{226}_{88}\textrm{Ra}\rightarrow ^{222}_{86}\textrm{Rn}+^{4}_{2}\alpha\label{Eq2}\]
Because nucleons are conserved in this and all other nuclear reactions, the sum of the mass numbers of the products, 222 + 4 = 226, equals the mass number of the parent. Similarly, the sum of the atomic numbers of the products, 86 + 2 = 88, equals the atomic number of the parent. Thus the nuclear equation is balanced.
Note
Just as the total number of atoms is conserved in a chemical reaction, the total number of nucleons is conserved in a nuclear reaction.
Beta \(\beta^-\) Decay
Nuclei that contain too many neutrons often undergo beta (β) decay, in which a neutron is converted to a proton and a high-energy electron that is ejected from the nucleus as a β particle:
\[\underset{\textrm{unstable} \\ \textrm{neutron in} \\ \textrm{nucleus}}{^1_0 \textrm n}\rightarrow \underset{\textrm{proton} \\ \textrm{retained} \\ \textrm{by nucleus}}{^{1}_{1} \textrm p}+\underset{\textrm{beta particle} \\ \textrm{emitted by} \\ \textrm{nucleus}}{^0_{-1} \beta}\label{Eq3}\]
The general reaction for beta decay is therefore
Although beta decay does not change the mass number of the nucleus, it does result in an increase of +1 in the atomic number because of the addition of a proton in the daughter nucleus. Thus beta decay decreases the neutron-to-proton ratio, moving the nucleus toward the band of stable nuclei. For example, carbon-14 undergoes beta decay to form nitrogen-14:
\[^{14}_{6}\textrm{C}\rightarrow ^{14}_{7}\textrm{N}+\,^{0}_{-1}\beta\label{Eq5}\]
Once again, the number of nucleons is conserved, and the charges are balanced. The parent and the daughter nuclei have the same mass number, 14, and the sum of the atomic numbers of the products is 6, which is the same as the atomic number of the carbon-14 parent.
Positron \(\beta^+\) Emission
Because a positron has the same mass as an electron but opposite charge, positron emission is the opposite of beta decay. Thus positron emission is characteristic of neutron-poor nuclei, which decay by transforming a proton to a neutron and emitting a high-energy positron:
\[^{1}_{1}\textrm{p}^+\rightarrow ^{1}_{0}\textrm{n}+\,^{0}_{+1}\beta^+\label{Eq6}\]
The general reaction for positron emission is therefore
\[\underset{\textrm{parent}}{^A_Z \textrm X}\rightarrow \underset{\textrm{daughter}}{^{A}_{Z-1} \textrm X'}+\underset{\textrm{positron}}{^0_{+1} \beta^+}\label{Eq7}\]
Like beta decay, positron emission does not change the mass number of the nucleus. In this case, however, the atomic number of the daughter nucleus is lower by 1 than that of the parent. Thus the neutron-to-proton ratio has increased, again moving the nucleus closer to the band of stable nuclei. For example, carbon-11 undergoes positron emission to form boron-11:
\[^{11}_{6}\textrm{C}\rightarrow ^{11}_{5}\textrm{B}+\,^{0}_{+1}\beta^+ \label{Eq8}\]
Nucleons are conserved, and the charges balance. The mass number, 11, does not change, and the sum of the atomic numbers of the products is 6, the same as the atomic number of the parent carbon-11 nuclide.
Electron Capture
A neutron-poor nucleus can decay by either positron emission or electron capture (EC), in which an electron in an inner shell reacts with a proton to produce a neutron:
\[^{1}_{1}\textrm{p} +\; ^{0}_{-1}\textrm{e}\rightarrow \, ^{1}_{0}\textrm n\label{Eq9}\]
When a second electron moves from an outer shell to take the place of the lower-energy electron that was absorbed by the nucleus, an x-ray is emitted. The overall reaction for electron capture is thus
Electron capture does not change the mass number of the nucleus because both the proton that is lost and the neutron that is formed have a mass number of 1. As with positron emission, however, the atomic number of the daughter nucleus is lower by 1 than that of the parent. Once again, the neutron-to-proton ratio has increased, moving the nucleus toward the band of stable nuclei. For example, iron-55 decays by electron capture to form manganese-55, which is often written as follows:
\[^{55}_{26}\textrm{Fe}\overset{\textrm{EC}}{\rightarrow}\, ^{55}_{25}\textrm{Mn}+\textrm{x-ray}\label{Eq11}\]
The atomic numbers of the parent and daughter nuclides differ in Equation 20.2.11, although the mass numbers are the same. To write a balanced nuclear equation for this reaction, we must explicitly include the captured electron in the equation:
\[^{55}_{26}\textrm{Fe}+\,^{0}_{-1}\textrm{e}\rightarrow \, ^{55}_{25}\textrm{Mn}+\textrm{x-ray}\label{Eq12}\]
Both positron emission and electron capture are usually observed for nuclides with low neutron-to-proton ratios, but the decay rates for the two processes can be very different.
Gamma \(\gamma\) Emission
Many nuclear decay reactions produce daughter nuclei that are in a nuclear excited state, which is similar to an atom in which an electron has been excited to a higher-energy orbital to give an electronic excited state. Just as an electron in an electronic excited state emits energy in the form of a photon when it returns to the ground state, a nucleus in an excited state releases energy in the form of a photon when it returns to the ground state. These high-energy photons are γ rays. Gamma (\(\gamma\)) emission can occur virtually instantaneously, as it does in the alpha decay of uranium-238 to thorium-234, where the asterisk denotes an excited state:
\[^{238}_{92}\textrm{U}\rightarrow \, \underset{\textrm{excited} \\ \textrm{nuclear} \\ \textrm{state}}{^{234}_{90}\textrm{Th*}} + ^{4}_{2}\alpha\xrightarrow {\textrm{relaxation}\,}\,^{234}_{90}\textrm{Th}+^{0}_{0}\gamma\label{Eq13}\]
If we disregard the decay event that created the excited nucleus, then
\[^{234}_{88}\textrm{Th*} \rightarrow\, ^{234}_{88}\textrm{Th}+^{0}_{0}\gamma\label{Eq14}\]
or more generally,
\[^{A}_{Z}\textrm{X*} \rightarrow\, ^{A}_{Z}\textrm{X}+^{0}_{0}\gamma\label{Eq15}\]
Gamma emission can also occur after a significant delay. For example, technetium-99m has a half-life of about 6 hours before emitting a γ ray to form technetium-99 (the m is for metastable). Because γ rays are energy, their emission does not affect either the mass number or the atomic number of the daughter nuclide. Gamma-ray emission is therefore the only kind of radiation that does not necessarily involve the conversion of one element to another, although it is almost always observed in conjunction with some other nuclear decay reaction.
Spontaneous Fission
Only very massive nuclei with high neutron-to-proton ratios can undergo spontaneous fission, in which the nucleus breaks into two pieces that have different atomic numbers and atomic masses. This process is most important for the transactinide elements, with Z ≥ 104. Spontaneous fission is invariably accompanied by the release of large amounts of energy, and it is usually accompanied by the emission of several neutrons as well. An example is the spontaneous fission of \(^{254}_{98}\textrm{Cf}\)
\[^{254}_{98}\textrm{Cf}\rightarrow \,^{118}_{46}\textrm{Pd}+\,^{132}_{52}\textrm{Te}+4^{1}_{0}\textrm{n}\label{Eq16}\]
Once again, the number of nucleons is conserved. Thus the sum of the mass numbers of the products (118 + 132 + 4 = 254) equals the mass number of the reactant. Similarly, the sum of the atomic numbers of the products [46 + 52 + (4 × 0) = 98] is the same as the atomic number of the parent nuclide.
Example \(\PageIndex{1}\)
Write a balanced nuclear equation to describe each reaction.
- the beta decay of \(^{35}_{16}\textrm{S}\)
- the decay of \(^{201}_{80}\textrm{Hg}\) by electron capture
- the decay of \(^{30}_{15}\textrm{P}\) by positron emission
Given: radioactive nuclide and mode of decay
Asked for: balanced nuclear equation
Strategy:
A Identify the reactants and the products from the information given.
B Use the values of A and Z to identify any missing components needed to balance the equation.
Solution:
a.
A We know the identities of the reactant and one of the products (a β particle). We can therefore begin by writing an equation that shows the reactant and one of the products and indicates the unknown product as \(^{A}_{Z}\textrm{X}\)
B Because both protons and neutrons must be conserved in a nuclear reaction, the unknown product must have a mass number of A = 35 − 0 = 35 and an atomic number of Z = 16 − (−1) = 17. The element with Z = 17 is chlorine, so the balanced nuclear equation is as follows:
A We know the identities of both reactants: \(^{201}_{80}\textrm{Hg}\) and an inner electron, \(^{0}_{-1}\textrm{e}\)
\(^{201}_{80}\textrm{Hg}+\,^{0}_{-1}\textrm e\rightarrow\,^{A}_{Z}\textrm{X}\)
B Both protons and neutrons are conserved, so the mass number of the product must be A = 201 + 0 = 201, and the atomic number of the product must be Z = 80 + (−1) = 79, which corresponds to the element gold. The balanced nuclear equation is thus
\(^{201}_{80}\textrm{Hg}+\,^{0}_{-1}\textrm e\rightarrow\,^{201}_{79}\textrm{Au}\)
c.A As in part (a), we are given the identities of the reactant and one of the products—in this case, a positron. The unbalanced nuclear equation is therefore
\(^{30}_{15}\textrm{P}\rightarrow\,^{A}_{Z}\textrm{X}+\,^{0}_{+1}\beta\)
B The mass number of the second product is A = 30 − 0 = 30, and its atomic number is Z = 15 − 1 = 14, which corresponds to silicon. The balanced nuclear equation for the reaction is as follows:
\(^{30}_{15}\textrm{P}\rightarrow\,^{30}_{14}\textrm{Si}+\,^{0}_{+1}\beta\)
Exercise \(\PageIndex{1}\)
Write a balanced nuclear equation to describe each reaction.
\(^{11}_{6}\textrm{C}\) by positron emission- the beta decay of molybdenum-99
- the emission of an α particle followed by gamma emission from \(^{185}_{74}\textrm{W}\)
- Answer a
-
\(^{11}_{6}\textrm{C}\rightarrow\,^{11}_{5}\textrm{B}+\,^{0}_{+1}\beta\)
- Answer d
-
\(^{99}_{42}\textrm{Mo}\rightarrow\,^{99m}_{43}\textrm{Tc}+\,^{0}_{-1}\beta\)
- Answer c
-
\(^{185}_{74}\textrm{W}\rightarrow\,^{181}_{72}\textrm{Hf}+\,^{4}_{2}\alpha +\,^{0}_{0}\gamma\)
Radioactive Decay Series
The nuclei of all elements with atomic numbers greater than 83 are unstable. Thus all isotopes of all elements beyond bismuth in the periodic table are radioactive. Because alpha decay decreases Z by only 2, and positron emission or electron capture decreases Z by only 1, it is impossible for any nuclide with Z > 85 to decay to a stable daughter nuclide in a single step, except via nuclear fission. Consequently, radioactive isotopes with Z > 85 usually decay to a daughter nucleus that is radiaoctive, which in turn decays to a second radioactive daughter nucleus, and so forth, until a stable nucleus finally results. This series of sequential alpha- and beta-decay reactions is called a radioactive decay series. The most common is the uranium-238 decay series, which produces lead-206 in a series of 14 sequential alpha- and beta-decay reactions (Figure \(\PageIndex{2}\)). Although a radioactive decay series can be written for almost any isotope with Z > 85, only two others occur naturally: the decay of uranium-235 to lead-207 (in 11 steps) and thorium-232 to lead-208 (in 10 steps). A fourth series, the decay of neptunium-237 to bismuth-209 in 11 steps, is known to have occurred on the primitive Earth. With a half-life of “only” 2.14 million years, all the neptunium-237 present when Earth was formed decayed long ago, and today all the neptunium on Earth is synthetic.
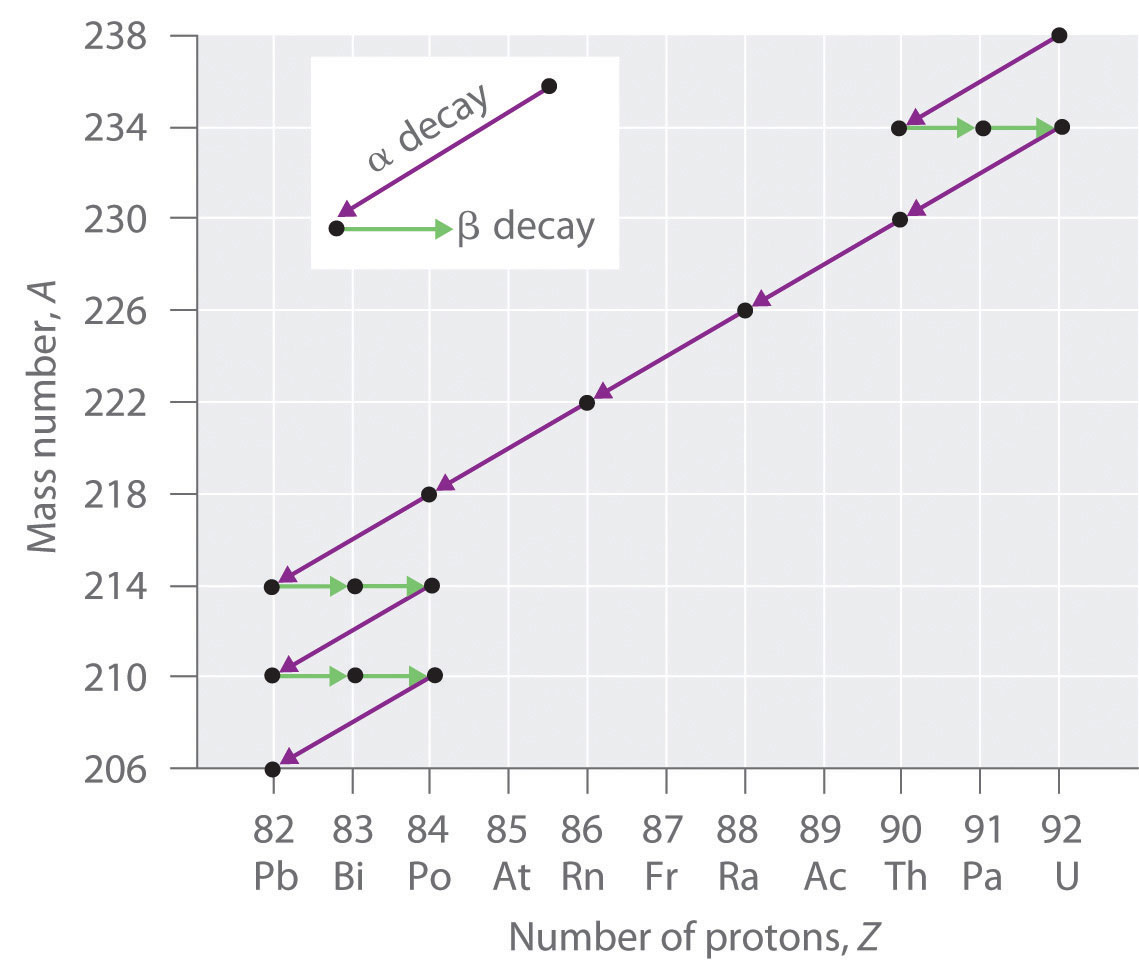
Figure \(\PageIndex{2}\) A Radioactive Decay Series. Three naturally occurring radioactive decay series are known to occur currently: the uranium-238 decay series, the decay of uranium-235 to lead-207, and the decay of thorium-232 to lead-208.
Due to these radioactive decay series, small amounts of very unstable isotopes are found in ores that contain uranium or thorium. These rare, unstable isotopes should have decayed long ago to stable nuclei with a lower atomic number, and they would no longer be found on Earth. Because they are generated continuously by the decay of uranium or thorium, however, their amounts have reached a steady state, in which their rate of formation is equal to their rate of decay. In some cases, the abundance of the daughter isotopes can be used to date a material or identify its origin.
Skills to Develop
- To know how to use half-lives to describe the rates of first-order reactions
Radioactive Decay Rates
Radioactivity, or radioactive decay, is the emission of a particle or a photon that results from the spontaneous decomposition of the unstable nucleus of an atom. The rate of radioactive decay is an intrinsic property of each radioactive isotope that is independent of the chemical and physical form of the radioactive isotope. The rate is also independent of temperature. Because there are so many unstable nuclei that decay, we need a method to describe and compare the rates at which these nuclei decay. One approach to describing reaction rates is based on the time required for the number of unstable nuclei to decrease to one-half the initial value. This period of time is called the half-life of the process, written as t1/2. Thus the half-life of a nuclear decay process is the time required for the number of unstable nuclei to decrease from [A]0 to 1/2[A]0.
| Number of Half-Lives | Percentage of Reactant Remaining | |
|---|---|---|
| 1 | \(\dfrac{100\%}{2}=50\%\) | \(\dfrac{1}{2}(100\%)=50\%\) |
| 2 | \(\dfrac{50\%}{2}=25\%\) | \(\dfrac{1}{2}\left(\dfrac{1}{2}\right)(100\%)=25\%\) |
| 3 | \(\dfrac{25\%}{2}=12.5\%\) | \(\dfrac{1}{2}\left(\dfrac{1}{2}\right )\left (\dfrac{1}{2}\right)(100\%)=12.5\%\) |
| n | \(\dfrac{100\%}{2^n}\) | \(\left(\dfrac{1}{2}\right)^n(100\%)=\left(\dfrac{1}{2}\right)^n\%\) |
As you can see from this table, the amount of reactant left after n half-lives of a first-order reaction is (1/2)n times the initial concentration.
For a first-order reaction, the concentration of the reactant decreases by a constant with each half-life and is independent of [A].
For a given number of atoms, isotopes with shorter half-lives decay more rapidly, undergoing a greater number of radioactive decays per unit time than do isotopes with longer half-lives. The half-lives of several isotopes are listed in Table 14.6, along with some of their applications.
| Radioactive Isotope | Half-Life | Typical Uses |
|---|---|---|
| *The m denotes metastable, where an excited state nucleus decays to the ground state of the same isotope. | ||
| hydrogen-3 (tritium) | 12.32 yr | biochemical tracer |
| carbon-11 | 20.33 min | positron emission tomography (biomedical imaging) |
| carbon-14 | 5.70 × 103 yr | dating of artifacts |
| sodium-24 | 14.951 h | cardiovascular system tracer |
| phosphorus-32 | 14.26 days | biochemical tracer |
| potassium-40 | 1.248 × 109 yr | dating of rocks |
| iron-59 | 44.495 days | red blood cell lifetime tracer |
| cobalt-60 | 5.2712 yr | radiation therapy for cancer |
| technetium-99m* | 6.006 h | biomedical imaging |
| iodine-131 | 8.0207 days | thyroid studies tracer |
| radium-226 | 1.600 × 103 yr | radiation therapy for cancer |
| uranium-238 | 4.468 × 109 yr | dating of rocks and Earth’s crust |
| americium-241 | 432.2 yr | smoke detectors |
Note
Radioactive decay is a first-order process.
Example \(\PageIndex{1}\)
If you have a 120 gram sample of a radioactive element, how many grams of that element will be left after 3 half-lives have passed?
Solution
Given: mass of radioactive sample of an element, number of half-lives
Asked to Solve For: mass of radioactive element after so many half-lives
Solve:
All radioactive samples lose half of their mass after each half-life. Thus, one solution is to calculate the mass after each half-life. (This method only works if you are asked to solve for a whole number of half-lives). Let the passing of time equal to one half-life be represented by and arrow, →. Then the solution is:
120 g → 60 g → 30 g → 15 g
Exercise \(\PageIndex{1}\)
If you have a 200. gram sample of a radioactive element, how many grams of that element will be left after 4 half-lives have passed?
- Answer
-
amount left = 200 → 100 g → 50 g → 25 g → 12.5 g
Exercise \(\PageIndex{2}\)
A certain radioactive nuclide has a half-life of 5.25 days. If you start with 80. grams of this nuclide, how many grams of the nuclide will be left after 21.00 days?
- Answer
-
\( 21.00 \; days \times \dfrac{1 \; half-life}{5.25 \; days} = 4.00 \; half-lives\)
amount left = 80. → 40. g → 20. g → 10. g → 5.0 g
Radioisotope Dating Techniques
In our earlier discussion, we used the half-life of a first-order reaction to calculate how long the reaction had been occurring. Because nuclear decay reactions follow first-order kinetics and have a rate constant that is independent of temperature and the chemical or physical environment, we can perform similar calculations using the half-lives of isotopes to estimate the ages of geological and archaeological artifacts. The techniques that have been developed for this application are known as radioisotope dating techniques.
The most common method for measuring the age of ancient objects is carbon-14 dating. The carbon-14 isotope, created continuously in the upper regions of Earth’s atmosphere, reacts with atmospheric oxygen or ozone to form 14CO2. As a result, the CO2 that plants use as a carbon source for synthesizing organic compounds always includes a certain proportion of 14CO2 molecules as well as nonradioactive 12CO2 and 13CO2. Any animal that eats a plant ingests a mixture of organic compounds that contains approximately the same proportions of carbon isotopes as those in the atmosphere. When the animal or plant dies, the carbon-14 nuclei in its tissues decay to nitrogen-14 nuclei by a radioactive process known as beta decay, which releases low-energy electrons (β particles) that can be detected and measured:
\[ \ce{^{14}C \rightarrow ^{14}N + \beta^{−}} \label{21.4.7}\]
The half-life for this reaction is 5700 ± 30 yr.
The 14C/12C ratio in living organisms is 1.3 × 10−12, with a decay rate of 15 dpm/g of carbon. Comparing the disintegrations per minute per gram of carbon from an archaeological sample with those from a recently living sample enables scientists to estimate the age of the artifact, as illustrated in Example 11.Using this method implicitly assumes that the 14CO2/12CO2 ratio in the atmosphere is constant, which is not strictly correct. Other methods, such as tree-ring dating, have been used to calibrate the dates obtained by radiocarbon dating, and all radiocarbon dates reported are now corrected for minor changes in the 14CO2/12CO2 ratio over time.
Summary
- The half-life of a first-order reaction is independent of the concentration of the reactants.
- The half-lives of radioactive isotopes can be used to date objects.
The rate of decay, or activity, of a sample of a radioactive substance is the rate of decrease in the number of radioactive nuclei per unit time. The half-life of a reaction is the time required for the reactant concentration to decrease to one-half its initial value. Radioactive decay reactions are first-order reactions.
Modified by Tom Neils (Grand Rapids Community College)

