Lattice energies, in addition to guiding the discovery of unknown compounds, are useful in explaining the absence (i.e., the thermodynamic instability) of non-existent compounds.[8] For example, CuF and AuF are unknown compounds, whereas CuF2, AuF3, and AuF5 are stable. In contrast AgF is a known, stable compound.
From the Born Haber cycle for CuF, the compound should be marginally stable (ΔHfo = -140 kJ/mol) with respect to the elements. Why then is CuF unknown?
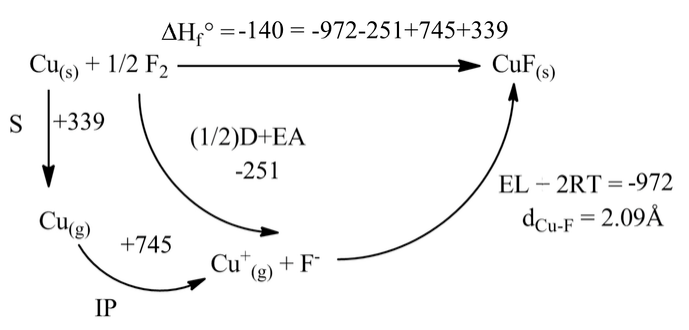
To gain insight into this question, we first construct a Born-Haber cycle for the formation of CuF2 from the elements. This compound is stable with respect to the elements by -368 kJ/mol.
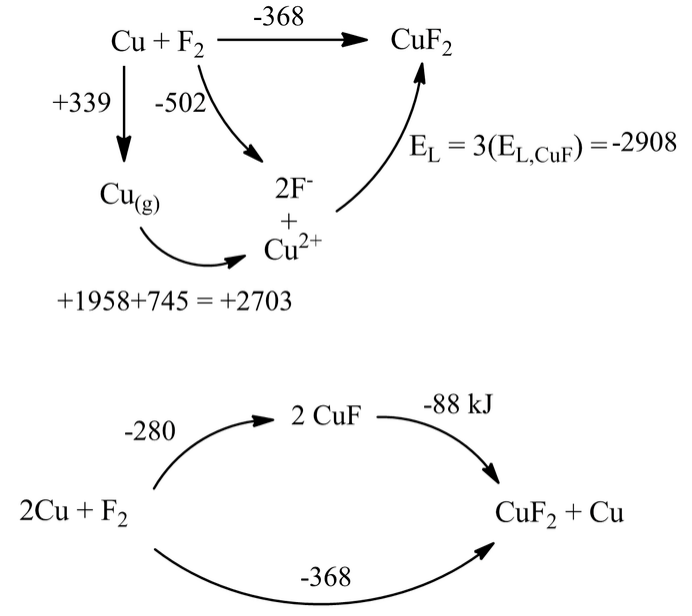
Combining the two cycles we see that the disproportionation of CuF to Cu and CuF2 is spontaneous. From similar cycles, we can also predict that the reaction 3AuF → AuF3 + 2Au should be spontaneous.
Why is the lowest oxidation state unstable for these fluorides? The key point is that the large difference in EL values (2908-972=1926 kJ in the case of copper fluorides) drives their disproportionation reactions. Note that when we use the Kapustinskii equation, we calculate that EL for CuF2 is approximately three times that of CuF. We use the same univalent radii in both calculations, but Cu has a 2+ charge in CuF2 (doubling the lattice energy relative to CuF), and contains 3/2 as many ions. The product z+z-n is thus three times larger for CuF2. The difference in EL values will thus increase as EL for the monovalent salt increases. We know that fluorides, having a small anion radius, will give larger EL values than iodides, which have larger anions. Thus the disproportionation reaction becomes more favorable for CuF than it is for CuI.
The stability of the lower vs. higher oxidation state thus depends on the size of the anion. For example, in fluorides, CuF is unstable but CuF2 is stable. However, in iodides, CuI is stable whereas CuI2 is unstable. From this we can develop a broad conclusion: small anions (O,F) tend to stabilize higher oxidation states, whereas large anions (S, Br, I...) stabilize lower oxidation states. Note that this trend has to do with the size and not with the electronegativity of the anion. Coincidentally, F and O are electronegative elements, but it is really their small size that has consequences for the lattice energy and their stabilization of higher oxidation states.
|

Cuprous iodide (CuI) is a crystalline compound used in organic synthesis and cloud seeding. This compound can be made in the laboratory by reacting soluble Cu2+ salts with a solution of sodium or potassium iodide. Because CuI2 is thermodynamicaly unstable, the reaction liberates I2 and a CuI precipitate forms.
|
Remember that the hard-soft acid-base rules could be interpreted in terms of the dominance of ionic vs. covalent interactions. Here we have put the hard-hard interaction in quantitative terms, based on (electrostatic) lattice energies.
Ag appears to buck the periodic trend. Why is AgF stable? This is because the second IP is very high (2071 kJ vs. 1958 kJ for Cu, 1979 for Au). Thus both AgF and AgF2 are known fluorides of Ag.




