9.E: Titrimetric Methods (Exercises)

Some of the problems that follow require one or more equilibrium constants or standard state potentials. For your convenience, here are hyperlinks to the appendices containing these constants.

Appendix 10: Solubility Products
Appendix 11: Acid Dissociation Constants
Appendix 12: Metal-Ligand Formation Constants
Appendix 13: Standard State Reduction Potentials

1. Calculate or sketch titration curves for the following acid–base titrations.

1. 25.0 mL of 0.100 M NaOH with 0.0500 M HCl
2. 50.0 mL of 0.0500 M HCOOH with 0.100 M NaOH
3. 50.0 mL of 0.100 M NH₃ with 0.100 M HCl
4. 50.0 mL of 0.0500 M ethylenediamine with 0.100 M HCl
5. 50.0 mL of 0.0400 M citric acid with 0.120 M NaOH
6. 50.0 mL of 0.0400 M H₃PO₄ with 0.120 M NaOH

2. Locate the equivalence point for each titration curve in problem 1. What is the stoichiometric relationship between the moles of acid and the moles of base at each of these equivalence points?

3. Suggest an appropriate visual indicator for each of the titrations in problem 1.

4. In sketching the titration curve for a weak acid we approximate the pH at 10% of the equivalence point volume as pK_a – 1, and the pH at 90% of the equivalence point volume as pK_a + 1. Show that these assumptions are reasonable.

5. Tartaric acid, H₂C₄H₄O₆, is a diprotic weak acid with a pK_a1 of 3.0 and a pK_a2 of 4.4. Suppose you have a sample of impure tartaric acid (purity > 80%), and that you plan to determine its purity by titrating with a solution of 0.1 M NaOH using an indicator to signal the end point. Describe how you will carry out the analysis, paying particular attention to how much sample to use, the desired pH range for the indicator, and how you will calculate the %w/w tartaric acid.

6. The following data for the titration of a monoprotic weak acid with a strong base were collected using an automatic titrator. Prepare normal, first derivative, second derivative, and Gran plot titration curves for this data, and locate the equivalence point for each.

Volume of NaOH (ml)	pH	Volume of NaOH (mL)	pH
0.25	3.0	49.95	7.8
0.86	3.2	49.97	8.0
1.63	3.4	49.98	8.2
2.72	3.6	49.99	8.4
4.29	3.8	50.00	8.7
6.54	4.0	50.01	9.1
9.67	4.2	50.02	9.4
13.79	4.4	50.04	9.6
18.83	4.6	50.06	9.8
24.47	4.8	50.10	10.0
30.15	5.0	50.16	10.2
35.33	5.2	50.25	10.4
39.62	5.4	50.40	10.6
42.91	5.6	50.63	10.8
45.28	5.8	51.01	11.0
46.91	6.0	51.61	11.2
48.01	6.2	52.58	11.4
48.72	6.4	54.15	11.6
49.19	6.6	56.73	11.8
49.48	6.8	61.11	12.0
49.67	7.0	68.83	12.2
49.79	7.2	83.54	12.4
49.78	7.4	116.14	12.6
49.92	7.6

7. Schwartz published the following simulated data for the titration of a 1.02 × 10^–4 M solution of a monoprotic weak acid (pK_a = 8.16) with 1.004 × 10^–3 M NaOH.¹⁰ The simulation assumes that a 50-mL pipet is used to transfer a portion of the weak acid solution to the titration vessel. A calibration of the pipet shows that it delivers a volume of only 49.94 mL. Prepare normal, first derivative, second derivative, and Gran plot titration curves for this data, and determine the equivalence point for each. How do these equivalence points compare to the expected equivalence point? Comment on the utility of each titration curve for the analysis of very dilute solutions of very weak acids.

mL of NaOH	pH	mL of NaOH	pH
0.03	6.212	4.79	8.858
0.09	6.504	4.99	8.926
0.29	6.936	5.21	8.994
0.72	7.367	5.41	9.056
1.06	7.567	5.61	9.118
1.32	7.685	5.85	9.180
1.53	7.776	6.05	9.231
1.76	7.863	6.28	9.283
1.97	7.938	6.47	9.327
2.18	8.009	6.71	9.374
2.38	8.077	6.92	9.414
2.60	8.146	7.15	9.451
2.79	8.208	7.36	9.484
3.01	8.273	7.56	9.514
3.41	8.332	7.79	9.545
3.60	8.458	8.21	9.572
3.80	8.521	8.44	9.599
3.99	8.584	8.64	9.645
4.18	8.650	8.84	9.666
4.40	8.720	9.07	9.688
4.57	8.784	9.27	9.706

8. Calculate or sketch the titration curve for a 50.0 mL solution of a 0.100 M monoprotic weak acid (pK_a = 8) with 0.1 M strong base in a nonaqueous solvent with K_s = 10^–20. You may assume that the change in solvent does not affect the weak acid’s pK_a. Compare your titration curve to the titration curve when water is the solvent.

9. The titration of a mixture of p-nitrophenol (pK_a = 7.0) and m-nitrophenol (pK_a = 8.3) can be followed spectrophotometrically. Neither acid absorbs at a wavelength of 545 nm, but their respective conjugate bases do absorb at this wavelength. The m-nitrophenolate ion has a greater absorbance than an equimolar solution of the p-nitrophenolate ion. Sketch the spectrophotometric titration curve for a 50.00-mL mixture consisting of 0.0500 M p-nitrophenol and 0.0500 M m-nitrophenol with 0.100 M NaOH. Compare your result to the expected potentiometric titration curves.

10. The quantitative analysis for aniline (C₆H₅NH₂, K_b = 3.94 × 10^–10) can be carried out by an acid–base titration using glacial acetic acid as the solvent and HClO₄ as the titrant. A known volume of sample containing 3–4 mmol of aniline is transferred to a 250-mL Erlenmeyer flask and diluted to approximately 75 mL with glacial acetic acid. Two drops of a methyl violet indicator are added, and the solution is titrated with previously standardized 0.1000 M HClO₄ (prepared in glacial acetic acid using anhydrous HClO₄) until the end point is reached. Results are reported as parts per million aniline.

(a) Explain why this titration is conducted using glacial acetic acid as the solvent instead of water.

(b) One problem with using glacial acetic acid as solvent is its relatively high coefficient of thermal expansion of 0.11%/^oC. For example, 100.00 mL of glacial acetic acid at 25^oC occupies 100.22 mL at 27^oC. What is the effect on the reported concentration of aniline if the standardization of HClO₄ is conducted at a temperature that is lower than that for the analysis of the unknown?

(c) The procedure calls for a sample containing 3–4 mmoles of aniline. Why is this requirement necessary?

11. Using a ladder diagram, explain why the presence of dissolved CO₂ leads to a determinate error for the standardization of NaOH if the end point’s pH falls between 6–10, but no determinate error if the end point’s pH is less than 6.

12. A water sample’s acidity is determined by titrating to fixed end point pHs of 3.7 and 8.3, with the former providing a measure of the concentration of strong acid, and the later a measure of the combined concentrations of strong acid and weak acid. Sketch a titration curve for a mixture of 0.10 M HCl and 0.10 M H₂CO₃ with 0.20 M strong base, and use it to justify the choice of these end points.

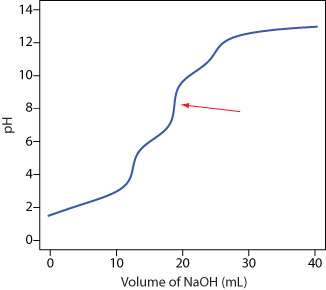
13. Ethylenediaminetetraacetic acid, H₄Y, is a weak acid with successive acid dissociation constants of 0.010, 2.19 × 10^–3, 6.92 × 10^–7, and 5.75 × 10^–11. Figure 9.46 shows a titration curve for H₄Y with NaOH. What is the stoichiometric relationship between H₄Y and NaOH at the equivalence point marked with the red arrow?

Figure 9.46 Titration curve for Problem 9.13.

14. A Gran plot method has been described for the quantitative analysis of a mixture consisting of a strong acid and a monoprotic weak acid.¹¹ A 50.00-mL mixture of HCl and CH₃COOH is transferred to an Erlenmeyer flask and titrated by using a digital pipet to add successive 1.00-mL aliquots of 0.09186 M NaOH. The progress of the titration is monitored by recording the pH after each addition of titrant. Using the two papers listed in the footnote as a reference, prepare a Gran plot for the following data, and determine the concentrations of HCl and CH₃COOH.

Volume of NaOH (mL)	pH	Volume of NaOH (mL)	pH	Volume of NaOH (mL)	pH
1.00	1.83	24.00	4.45	47.00	12.14
2.00	1.86	25.00	4.53	48.00	12.17
3.00	1.89	26.00	4.61	49.00	12.20
4.00	1.92	27.00	4.69	50.00	12.23
5.00	1.95	28.00	4.76	51.00	12.26
6.00	1.99	29.00	4.84	52.00	12.28
7.00	2.03	30.00	4.93	53.00	12.30
8.00	2.10	31.00	5.02	54.00	12.32
9.00	2.18	32.00	5.13	55.00	12.34
10.00	2.31	33.00	5.23	56.00	12.36
11.00	2.51	34.00	5.37	57.00	12.38
12.00	2.81	35.00	5.52	58.00	12.39
13.00	3.16	36.00	5.75	59.00	12.40
14.00	3.36	37.00	6.14	60.00	12.42
15.00	3.54	38.00	10.30	61.00	12.43
16.00	3.69	39.00	11.31	62.00	12.44
17.00	3.81	40.00	11.58	63.00	12.45
18.00	3.93	41.00	11.74	64.00	12.47
19.00	4.02	42.00	11.85	65.00	12.48
20.00	4.14	43.00	11.93	66.00	12.49
21.00	4.22	44.00	12.00	67.00	12.50
22.00	4.30	45.00	12.05	68.00	12.51
23.00	4.38	46.00	12.10	69.00	12.52

15. Explain why it is not possible for a sample of water to simultaneously have OH^– and HCO₃^– as sources of alkalinity.

16. For each of the following, determine the sources of alkalinity (OH^–, HCO₃^–, CO₃^2–) and their respective concentrations in parts per million. In each case a 25.00-mL sample is titrated with 0.1198 M HCl to the bromocresol green and the phenolphthalein end points.

		Volume of HCl (mL) to the phenolphthalein end point		Volume of HCl (mL) to the bromocresol green end point
a		21.36		21.38
b		5.67		21.13
c		0.00		14.28
d		17.12		34.26
e		21.36		25.69

17. A sample may contain any of the following: HCl, NaOH, H₃PO₄, H₂PO₄^–, HPO₄^2–, or PO₄^3–. The composition of a sample is determined by titrating a 25.00-mL portion with 0.1198 M HCl or 0.1198 M NaOH to the phenolphthalein and the methyl orange end points. For each of the following, determine which species are present in the sample, and their respective molar concentrations.

		Titrant		Phenolphthalein end ​​ point volume (mL)		methyl orange end point volume (mL)
a		HCl		11.54		35.29
b		NaOH		19.79		9.89
c		HCl		22.76		22.78
d		NaOH		39.42		17.48

18. The protein in a 1.2846-g sample of an oat cereal is determined by a Kjeldahl analysis. The sample is digested with H₂SO₄, the resulting solution made basic with NaOH, and the NH₃ distilled into 50.00 mL of 0.09552 M HCl. The excess HCl is back titrated using 37.84 mL of 0.05992 M NaOH. Given that the proteins in grains average 17.54% w/w N, report the %w/w protein in the sample.

19. The concentration of SO₂ in air is determined by bubbling a sample of air through a trap containing H₂O₂. Oxidation of SO₂ by H₂O₂ results in the formation of H₂SO₄, which is then determined by titrating with NaOH. In a typical analysis, a sample of air was passed through the peroxide trap at a rate of 12.5 L/min for 60 min and required 10.08 mL of 0.0244 M NaOH to reach the phenolphthalein end point. Calculate the μL/L SO₂ in the sample of air. The density of SO₂ at the temperature of the air sample is 2.86 mg/mL.

20. The concentration of CO₂ in air is determined by an indirect acid–base titration. A sample of air is bubbled through a solution containing an excess of Ba(OH)₂, precipitating BaCO₃. The excess Ba(OH)₂ is back titrated with HCl. In a typical analysis a 3.5-L sample of air was bubbled through 50.00 mL of 0.0200 M Ba(OH)₂. Back titrating with 0.0316 M HCl required 38.58 mL to reach the end point. Determine the ppm CO₂ in the sample of air given that the density of CO₂ at the temperature of the sample is 1.98 g/L.

21. The purity of a synthetic preparation of methylethyl ketone, C₃H₈O, is determined by reacting it with hydroxylamine hydrochloride, liberating HCl (see reaction in Table 9.8). In a typical analysis a 3.00-mL sample was diluted to 50.00 mL and treated with an excess of hydroxylamine hydrochloride. The liberated HCl was titrated with 0.9989 M NaOH, requiring 32.68 mL to reach the end point. Report the percent purity of the sample given that the density of methylethyl ketone is 0.805 g/mL.

22. Animal fats and vegetable oils are triesters formed from the reaction between glycerol (1,2,3-propanetriol) and three long-chain fatty acids. One of the methods used to characterize a fat or an oil is a determination of its saponification number. When treated with boiling aqueous KOH, an ester saponifies into the parent alcohol and fatty acids (as carboxylate ions). The saponification number is the number of milligrams of KOH required to saponify 1.000 gram of the fat or the oil. In a typical analysis a 2.085-g sample of butter is added to 25.00 mL of 0.5131 M KOH. After saponification is complete the excess KOH is back titrated with 10.26 mL of 0.5000 M HCl. What is the saponification number for this sample of butter?

23. A 250.0-mg sample of an organic weak acid is dissolved in an appropriate solvent and titrated with 0.0556 M NaOH, requiring 32.58 mL to reach the end point. Determine the compound’s equivalent weight.

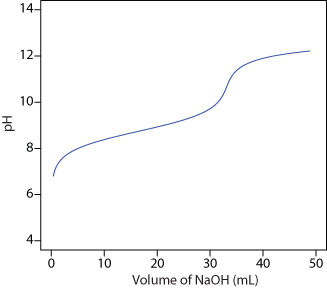
24. Figure 9.47 shows a potentiometric titration curve for a 0.4300-g sample of a purified amino acid that was dissolved in 50.00 mL of water and titrated with 0.1036 M NaOH. Identify the amino acid from the possibilities listed in the following table.

amino acid	formula weight (g/mol)	Ka
alanine	89.1	1.36 × 10^–10
glycine	75.1	1.67 × 10^–10
methionine	149.2	8.9 × 10^–10
taurine	125.2	1.8 × 10^–9
asparagine	150	1.9 × 10^–9
leucine	131.2	1.79 × 10^–10
phenylalanine	166.2	4.9 × 10^–10
valine	117.2	1.91 × 10^–10

Figure 9.47 Titration curve for Problem 9.24.

25. Using its titration curve, determine the acid dissociation constant for the weak acid in problem 9.6.

26. Where in the scale of operations do the microtitration techniques discussed in section 9B.7 belong?

27. An acid–base titration may be used to determine an analyte’s gram equivalent weight, but it can not be used to determine its gram formula weight. Explain why.

28. Commercial washing soda is approximately 30–40% w/w Na₂CO₃. One procedure for the quantitative analysis of washing soda contains the following instructions:

Transfer an approximately 4-g sample of the washing soda to a 250-mL volumetric flask. Dissolve the sample in about 100 mL of H₂O and then dilute to the mark. Using a pipet, transfer a 25-mL aliquot of this solution to a 125-mL Erlenmeyer flask, and add 25-mL of H₂O and 2 drops of bromocresol green indicator. Titrate the sample with 0.1 M HCl to the indicator’s end point.

What modifications, if any, are necessary if you want to adapt this procedure to evaluate the purity of commercial Na₂CO₃ that is >98% pure?

29. A variety of systematic and random errors are possible when standardizing a solution of NaOH against the primary weak acid standard potassium hydrogen phthalate (KHP). Identify, with justification, whether the following are systematic or random sources of error, or if they have no effect. If the error is systematic, then indicate whether the experimentally determined molarity for NaOH is too high or too low. The standardization reaction is

\[\ce{C_8H_5O_4^-}(aq)+\mathrm{OH^-}(aq)\rightarrow \mathrm{C_8H_4O_4^{2-}}(aq)+\mathrm{H_2O}(l)\]

(a) The balance used to weigh KHP is not properly calibrated and always reads 0.15 g too low.

(b) The indicator for the titration changes color between a pH of 3–4.

(c) An air bubble, which is lodged in the buret’s tip at the beginning of the analysis, dislodges during the titration.

(d) Samples of KHP are weighed into separate Erlenmeyer flasks, but the balance is only tarred with the first flask.

(e) The KHP is not dried before it was used.

(f) The NaOH is not dried before it was used.

(g) The procedure states that the sample of KHP should be dissolved in 25 mL of water, but it is accidentally dissolved in 35 mL of water.

30. The concentration of o-phthalic acid in an organic solvent, such as n-butanol, is determined by an acid–base titration using aqueous NaOH as the titrant. As the titrant is added, the o-phthalic acid is extracted into the aqueous solution where it reacts with the titrant. The titrant must be added slowly to allow sufficient time for the extraction to take place.

(a) What type of error do you expect if the titration is carried out too quickly?

(b) Propose an alternative acid–base titrimetric method that allows for a more rapid determination of the concentration of o-phthalic acid in n‑butanol.

31. Calculate or sketch titration curves for 50.00 mL of 0.0500 Mg²⁺ with 0.0500 M EDTA at a pH of 7 and 10. Locate the equivalence point for each titration curve.

32. Calculate or sketch titration curves for 25.0 mL of 0.0500 M Cu²⁺ with 0.025 M EDTA at a pH of 10, and in the presence of 10^–3 M and 10^–1 M NH₃. Locate the equivalence point for each titration curve.

33. Sketch the spectrophotometric titration curve for the titration of a mixture of 5.00 × 10^–3 M Bi³⁺ and 5.00 × 10^–3 M Cu²⁺ with 0.0100 M EDTA. Assume that only the Cu²⁺–EDTA complex absorbs at the selected wavelength.

34. The EDTA titration of mixtures of Ca²⁺ and Mg²⁺ can be followed thermometrically because the formation of the Ca²⁺–EDTA complex is exothermic and the formation of the Mg²⁺–EDTA complex is endothermic. Sketch the thermometric titration curve for a mixture of 5.00 × 10^–3 M Ca²⁺ and 5.00 × 10^–3 M Mg²⁺ with 0.0100 M EDTA. The heats of formation for CaY^2– and MgY^2– are, respectively, –23.9 kJ/mole and 23.0 kJ/mole.

35. EDTA is one member of a class of aminocarboxylate ligands that form very stable 1:1 complexes with metal ions. The following table provides logK_f values for the complexes of six such ligands with Ca²⁺ and Mg²⁺. Which ligand is the best choice for the direct titration of Ca²⁺ in the presence of Mg²⁺?

		Mg^2+	Ca^2+
EDTA	ethylenediaminetetraacetic acid	8.7	10.7
HEDTA	N-hydroxyethylenediaminetriacetic acid	7.0	8.0
EEDTA	ethyletherdiaminetetraacetic acid	8.3	10.0
DGTA	ethyleneglycol-bis(β-aminoethylether)- N,N´-tetraacetic acid	5.4	10.9
DTPA	diethylenetriaminepentaacetic acid	9.0	10.7
CyDTA	cyclohexanediaminetetraacetic acid	10.3	12.3

36. The amount of calcium in physiological fluids can be determined by a complexometric titration with EDTA. In one such analysis a 0.100-mL sample of a blood serum was made basic by adding 2 drops of NaOH and titrated with 0.00119 M EDTA, requiring 0.268 mL to reach the end point. Report the concentration of calcium in the sample as milligrams Ca per 100 mL.

37. After removing the membranes from an eggshell, the shell is dried and its mass recorded as 5.613 g. The eggshell is transferred to a 250-mL beaker and dissolved in 25 mL of 6 M HCl. After filtering, the solution containing the dissolved eggshell is diluted to 250 mL in a volumetric flask. A 10.00-mL aliquot is placed in a 125-mL Erlenmeyer flask and buffered to a pH of 10. Titrating with 0.04988 M EDTA requires 44.11 mL to reach the end point. Determine the amount of calcium in the eggshell as %w/w CaCO₃.

38. The concentration of cyanide, CN^–, in a copper electroplating bath can be determined by a complexometric titration with Ag⁺, forming the soluble Ag(CN)₂^– complex. In a typical analysis a 5.00-mL sample from an electroplating bath is transferred to a 250-mL Erlenmeyer flask, and treated with 100 mL of H₂O, 5 mL of 20% w/v NaOH and 5 mL of 10% w/v KI. The sample is titrated with 0.1012 M AgNO₃, requiring 27.36 mL to reach the end point as signaled by the formation of a yellow precipitate of AgI. Report the concentration of cyanide as parts per million of NaCN.

39. Before the introduction of EDTA most complexation titrations used Ag⁺ or CN^– as the titrant. The analysis for Cd²⁺, for example, was accomplished indirectly by adding an excess of KCN to form Cd(CN)₄^2–, and back titrating the excess CN^– with Ag⁺, forming Ag(CN)₂^–. In one such analysis a 0.3000-g sample of an ore was dissolved and treated with 20.00 mL of 0.5000 M KCN. The excess CN^– required 13.98 mL of 0.1518 M AgNO₃ to reach the end point. Determine the %w/w Cd in the ore.

40. Solutions containing both Fe³⁺ and Al³⁺ can be selectively analyzed for Fe³⁺ by buffering to a pH of 2 and titrating with EDTA. The pH of the solution is then raised to 5 and an excess of EDTA added, resulting in the formation of the Al³⁺–EDTA complex. The excess EDTA is back-titrated using a standard solution of Fe³⁺, providing an indirect analysis for Al³⁺.

(a) At a pH of 2, verify that the formation of the Fe³⁺–EDTA complex is favorable, and that the formation of the Al³⁺–EDTA complex is not favorable.

(b) A 50.00-mL aliquot of a sample containing Fe³⁺ and Al³⁺ is transferred to a 250-mL Erlenmeyer flask and buffered to a pH of 2. A small amount of salicylic acid is added, forming the soluble red-colored Fe³⁺–salicylic acid complex. The solution is titrated with 0.05002 M EDTA, requiring 24.82 mL to reach the end point as signaled by the disappearance of the Fe³⁺–salicylic acid complex’s red color. The solution is buffered to a pH of 5 and 50.00 mL of 0.05002 M EDTA is added. After ensuring that the formation of the Al³⁺–EDTA complex is complete, the excess EDTA was back titrated with 0.04109 M Fe³⁺, requiring 17.84 mL to reach the end point as signaled by the reappearance of the red-colored Fe³⁺–salicylic acid complex. Report the molar concentrations of Fe³⁺ and Al³⁺ in the sample.

41. Prada and colleagues described an indirect method for determining sulfate in natural samples, such as seawater and industrial effluents.¹² The method consists of three steps: precipitating the sulfate as PbSO₄; dissolving the PbSO₄ in an ammonical solution of excess EDTA to form the soluble PbY^2– complex; and titrating the excess EDTA with a standard solution of Mg²⁺. The following reactions and equilibrium constants are known

\(\mathrm{PbSO_4}(s)\rightleftharpoons \mathrm{Pb^{2+}}(aq)+\mathrm{SO_4^{2-}}(aq)\)	\(K_\textrm{sp}=1.6\times10^{-8}\)
\(\mathrm{Pb^{2+}}(aq)+\mathrm{Y^{4-}}(aq)\rightleftharpoons\mathrm{PbY^{2-}}(aq)\)	\(K_\textrm f=1.1\times10^{18}\)
\(\mathrm{Mg^{2+}}(aq)+\mathrm{Y^{4-}}(aq)\rightleftharpoons\mathrm{MgY^{2-}}(aq)\)	\(K_\textrm f=4.9\times10^8\)
\(\mathrm{Zn^{2+}}(aq)+\mathrm{Y^{4-}}(aq)\rightleftharpoons \mathrm{ZnY^{2-}}(aq)\)	\(K_\textrm f=3.2\times10^{16}\)

(a) Verify that a precipitate of PbSO₄ dissolves in a solution of Y^4–.

(b) Sporek proposed a similar method using Zn²⁺ as a titrant and found that the accuracy was frequently poor.¹³ One explanation is that Zn²⁺ might react with the PbY^2–complex, forming ZnY^2–. Show that this might be a problem when using Zn²⁺ as a titrant, but that it is not a problem when using Mg²⁺ as a titrant. Would such a displacement of Pb²⁺ by Zn²⁺ lead to the reporting of too much or too little sulfate?

(c) In a typical analysis, a 25.00-mL sample of an industrial effluent was carried through the procedure using 50.00 mL of 0.05000 M EDTA. Titrating the excess EDTA required 12.42 mL of 0.1000 M Mg²⁺. Report the molar concentration of SO₄^2– in the sample of effluent.

42. Table 9.10 provides values for the fraction of EDTA present as Y^4-, α_Y^4–. Values of α_Y^4– are calculated using the equation

\[\alpha_\mathrm{Y^{\large 4-}}=\dfrac{[Y^{4-}]}{C_\textrm{EDTA}}\]

where [Y⁴⁻] is the concentration of the fully deprotonated EDTA and C_EDTA is the total concentration of EDTA in all of its forms

\[C_\textrm{EDTA}=[\mathrm{H_6Y^{2+}}]+[\mathrm{H_5Y^+}]+[\mathrm{H_4Y}]+[\mathrm{H_3Y^-}]+[\mathrm{H_2Y^{2-}}]+[\mathrm{HY^{3-}}]+[\mathrm{Y^{4-}}]\]

Using the following equilibria

\(\mathrm{H_6Y^{2+}}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{H_5Y^+}(aq)\)	\(K_\textrm{a1}\)
\(\mathrm{H_5Y^+}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{H_4Y}(aq)\)	\(K_\textrm{a2}\)
\(\mathrm{H_4Y}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{H_3Y^-}(aq)\)	\(K_\textrm{a3}\)
\(\mathrm{H_3Y^-}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{H_2Y^{2-}}(aq)\)	\(K_\textrm{a4}\)
\(\mathrm{H_2Y^{2-}}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{HY^{3-}}(aq)\)	\(K_\textrm{a5}\)
\(\mathrm{HY^{3-}}(aq)+\mathrm{H_2O}(l)\rightleftharpoons\mathrm{H_3O^+}(aq)+\mathrm{Y^{4-}}(aq)\hspace{10mm}\)	\(K_\textrm{a6}\)

show that

\[\alpha_\mathrm{Y^{4-}}=\dfrac{K_\textrm{a1}K_\textrm{a2}K_\textrm{a3}K_\textrm{a4}K_\textrm{a5}K_\textrm{a6}}{d}\]

where

\[d=[\textrm H^+]^6+[\textrm H^+]^5K_\textrm{a1}+[\textrm H^+]^4K_\textrm{a1}K_\textrm{a2}+[\textrm H^+]^3K_\textrm{a1}K_\textrm{a2}K_\textrm{a3}+\]

\[[\textrm H^+]^2K_\textrm{a1}K_\textrm{a2}K_\textrm{a3}K_\textrm{a4}+[\textrm H^+]^1K_\textrm{a1}K_\textrm{a2}K_\textrm{a3}K_\textrm{a4}K_\textrm{a5}+ K_\textrm{a1}K_\textrm{a2}K_\textrm{a3}K_\textrm{a4}K_\textrm{a5}K_\textrm{a6}\]

43. Calculate or sketch titration curves for the following (unbalanced) redox titration reactions at 25^oC. Assume the analyte is initially present at a concentration of 0.0100 M and that a 25.0-mL sample is taken for analysis. The titrant, which is the underlined species in each reaction, is 0.0100 M.

\((\textrm{a})\;\mathrm{V^{2+}}(aq) + \underline{\mathrm{Ce^{4+}}}(aq)\rightarrow \mathrm V^{3+}(aq) + \mathrm{Ce^3+}(aq)\)
\((\textrm{b})\;\mathrm{Ti^{2+}}(aq) + \underline{\mathrm{Fe^{3+}}}(aq)\rightarrow \mathrm{Ti^{3+}}(aq) + \mathrm{Fe^{2+}}(aq)\)
\((\textrm{c})\;\mathrm{Fe^{2+}}(aq) + \underline{\ce{MnO_4^-}}(aq) \rightarrow \mathrm{Fe^{3+}}(aq) + \mathrm{Mn^{2+}}(aq)\;\textrm{(at pH = 1)}\)

44. What is the equivalence point for each titration in problem 43?

45. Suggest an appropriate indicator for each titration in problem 43.

46. The iron content of an ore can be determined by a redox titration using K₂Cr₂O₇ as the titrant. A sample of the ore is dissolved in concentrated HCl using Sn²⁺ to speed its dissolution by reducing Fe³⁺ to Fe²⁺. After the sample is dissolved, Fe²⁺ and any excess Sn²⁺ are oxidized to Fe³⁺ and Sn⁴⁺ using MnO₄^–. The iron is then carefully reduced to Fe²⁺ by adding a 2–3 drop excess of Sn²⁺. A solution of HgCl₂ is added and, if a white precipitate of Hg₂Cl₂ forms, the analysis is continued by titrating with K₂Cr₂O₇. The sample is discarded without completing the analysis if a precipitate of Hg₂Cl₂ does not form, or if a gray precipitate (due to Hg) forms.

(a) Explain why the analysis is not completed if a white precipitate of Hg₂Cl₂ forms, or if a gray precipitate forms.

(b) Is a determinate error introduced if the analyst forgets to add Sn²⁺ in the step where the iron ore is dissolved?

(c) Is a determinate error introduced if the iron is not quantitatively oxidized back to Fe³⁺ by the MnO₄^–?

47. The amount of Cr³⁺ in an inorganic salt can be determined by a redox titration. A portion of sample containing approximately 0.25 g of Cr³⁺ is accurately weighed and dissolved in 50 mL of H₂O. The Cr³⁺ is oxidized to Cr₂O₇^2– by adding 20 mL of 0.1 M AgNO₃, which serves as a catalyst, and 50 mL of 10%w/v (NH₄)₂S₂O₈, which serves as the oxidizing agent. After the reaction is complete the resulting solution is boiled for 20 minutes to destroy the excess S₂O₈^2–, cooled to room temperature, and diluted to 250 mL in a volumetric flask. A 50-mL portion of the resulting solution is transferred to an Erlenmeyer flask, treated with 50 mL of a standard solution of Fe²⁺, and acidified with 200 mL of 1 M H₂SO₄, reducing the Cr₂O₇^2– to Cr³⁺. The excess Fe²⁺ is then determined by a back titration with a standard solution of K₂Cr₂O₇ using an appropriate indicator. The results are reported as %w/w Cr³⁺.

(a) There are several places in the procedure where a reagent’s volume is specified (see underlined text). Which of these measurements must be made using a volumetric pipet?

(b) Excess peroxydisulfate, S₂O₈^2– is destroyed by boiling the solution. What is the effect on the reported %w/w Cr³⁺ if some of the S₂O₈^2– is not destroyed during this step?

(c) Solutions of Fe²⁺ undergo slow air oxidation to Fe³⁺. What is the effect on the reported %w/w Cr³⁺ if the standard solution of Fe²⁺ is inadvertently allowed to be partially oxidized?

48. The exact concentration of H₂O₂ in a solution that is nominally 6% w/v H₂O₂ can be determined by a redox titration with MnO₄^–. A 25-mL aliquot of the sample is transferred to a 250-mL volumetric flask and diluted to volume with distilled water. A 25-mL aliquot of the diluted sample is added to an Erlenmeyer flask, diluted with 200 mL of distilled water, and acidified with 20 mL of 25% v/v H₂SO₄. The resulting solution is titrated with a standard solution of KMnO₄ until a faint pink color persists for 30 s. The results are reported as %w/v H₂O₂.

(a) Many commercially available solutions of H₂O₂ contain an inorganic or organic stabilizer to prevent the autodecomposition of the peroxide to H₂O and O₂. What effect does the presence of this stabilizer have on the reported %w/v H₂O₂ if it also reacts with MnO₄^–?

(b) Laboratory distilled water often contains traces of dissolved organic material that may react with MnO₄^–. Describe a simple method to correct for this potential interference.

(c) What modifications to the procedure, if any, are need if the sample has a nominal concentration of 30% w/v H₂O₂.

49. The amount of iron in a meteorite was determined by a redox titration using KMnO₄ as the titrant. A 0.4185-g sample was dissolved in acid and the liberated Fe³⁺ quantitatively reduced to Fe²⁺ using a Walden reductor. Titrating with 0.02500 M KMnO₄ requires 41.27 mL to reach the end point. Determine the %w/w Fe₂O₃ in the sample of meteorite.

50. Under basic conditions, MnO₄^– can be used as a titrant for the analysis of Mn²⁺, with both the analyte and the titrant forming MnO₂. In the analysis of a mineral sample for manganese, a 0.5165-g sample is dissolved and the manganese reduced to Mn²⁺. The solution is made basic and titrated with 0.03358 M KMnO₄, requiring 34.88 mL to reach the end point. Calculate the %w/w Mn in the mineral sample.

51. The amount of uranium in an ore can be determined by a redox back titration. The analysis is accomplished by dissolving the ore in sulfuric acid and reducing the resulting UO₂²⁺ to U⁴⁺ with a Walden reductor. The resulting solution is treated with an excess of Fe³⁺, forming Fe²⁺ and U⁶⁺. The Fe²⁺ is titrated with a standard solution of K₂Cr₂O₇. In a typical analysis a 0.315-g sample of ore is passed through the Walden reductor and treated with 50.00 mL of 0.0125 M Fe³⁺. Back titrating with 0.00987 M K₂Cr₂O₇ requires 10.52 mL. What is the %w/w U in the sample?

52. The thickness of the chromium plate on an auto fender was determined by dissolving a 30.0-cm² section in acid, and oxidizing the liberated Cr³⁺ to Cr₂O₇^2– with peroxydisulfate. After removing the excess peroxydisulfate by boiling, 500.0 mg of Fe(NH₄)₂(SO₄)₂•6H₂O was added, reducing the Cr₂O₇^2– to Cr³⁺. The excess Fe²⁺ was back titrated, requiring 18.29 mL of 0.00389 M K₂Cr₂O₇ to reach the end point. Determine the average thickness of the chromium plate given that the density of Cr is 7.20 g/cm³.

53. The concentration of CO in air can be determined by passing a known volume of air through a tube containing I₂O₅, forming CO₂ and I₂. The I₂ is removed from the tube by distilling it into a solution containing an excess of KI, producing I₃^–. The I₃^– is titrated with a standard solution of Na₂S₂O₃. In a typical analysis a 4.79-L sample of air was sampled as described here, requiring 7.17 mL of 0.00329 M Na₂S₂O₃ to reach the end point. If the air has a density of 1.23 × 10^–3 g/mL, determine the parts per million CO in the air.

54. The level of dissolved oxygen in a water sample can be determined by the Winkler method. In a typical analysis a 100.0-mL sample is made basic and treated with a solution of MnSO₄, resulting in the formation of MnO₂. An excess of KI is added and the solution is acidified, resulting in the formation of Mn²⁺ and I₂. The liberated I₂ is titrated with a solution of 0.00870 M Na₂S₂O₃, requiring 8.90 mL to reach the starch indicator end point. Calculate the concentration of dissolved oxygen as parts per million O₂.

55. The analysis for Cl^– using the Volhard method requires a back titration. A known amount of AgNO₃ is added, precipitating AgCl. The unreacted Ag⁺ is determined by back titrating with KSCN. There is a complication, however, because AgCl is more soluble than AgSCN.

(a) Why do the relative solubilities of AgCl and AgSCN lead to a titration error?

(b) Is the resulting titration error a positive or a negative determinate error?

(c) How might you modify the procedure to prevent this eliminate this source of determinate error?

(d) Will this source of determinate error be of concern when using the Volhard method to determine Br^–?

56. Voncina and co-workers suggest that a precipitation titration can be monitored by measuring pH as a function of the volume of titrant if the titrant is a weak base.¹⁴ For example, when titrating Pb²⁺ with CrO₄^2– the solution containing the analyte is initially acidified to a pH of 3.50 using HNO₃. Before the equivalence point the concentration of CrO₄^2– is controlled by the solubility product of PbCrO₄. After the equivalence point the concentration of CrO₄^2– is determined by the amount of excess titrant. Considering the reactions controlling the concentration of CrO₄^2–, sketch the expected titration curve of pH versus volume of titrant.

57. Calculate or sketch the titration curve for the titration of 50.0 mL of 0.0250 M KI with 0.0500 M AgNO₃. Prepare separate titration curve using pAg and pI on the y-axis.

58. Calculate or sketch the titration curve for the titration of 25.0 mL mixture of 0.0500 M KI and 0.0500 M KSCN with 0.0500 M AgNO₃.

59. A 0.5131-g sample containing KBr is dissolved in 50 mL of distilled water. Titrating with 0.04614 M AgNO₃ requires 25.13 mL to reach the Mohr end point. A blank titration requires 0.65 mL to reach the same end point. Report the %w/w KBr in the sample.

60. A 0.1093-g sample of impure Na₂CO₃ was analyzed by the Volhard method. After adding 50.00 mL of 0.06911 M AgNO₃, the sample was back titrated with 0.05781 M KSCN, requiring 27.36 mL to reach the end point. Report the purity of the Na₂CO₃ sample.

61. A 0.1036-g sample containing only BaCl₂ and NaCl is dissolved in 50 mL of distilled water. Titrating with 0.07916 M AgNO₃ requires 19.46 mL to reach the Fajans end point. Report the %w/w BaCl₂ in the sample.

9.6.3 Solutions to Practice Exercises

Practice Exercise 9.1

The volume of HCl needed to reach the equivalence point is

\[V_\textrm{eq}=V_\textrm a=\dfrac{M_\textrm bV_\textrm b}{M_\textrm a}=\dfrac{\textrm{(0.125 M)(25.0 mL)}}{\textrm{0.0625 M}}=\textrm{50.0 mL}\]

Before the equivalence point, NaOH is present in excess and the pH is determined by the concentration of unreacted OH^–. For example, after adding 10.0 mL of HCl

\[[\mathrm{OH^-}]=\dfrac{\textrm{(0.125 M)(25.0 mL)}-\textrm{(0.0625 M)(10.0 mL)}}{\textrm{25.0 mL + 10.0 mL}}=\textrm{0.0714 M}\]

\[[\mathrm{H_3O^+}]=\dfrac{K_\textrm w}{[\textrm{OH}^-]}=\dfrac{1.0\times10^{-14}}{\textrm{0.0714 M}}=1.40\times10^{-13}\textrm{ M}\]

the pH is 12.85.

For the titration of a strong base with a strong acid the pH at the equivalence point is 7.00.

For volumes of HCl greater than the equivalence point, the pH is determined by the concentration of excess HCl. For example, after adding 70.0 mL of titrant the concentration of HCl is

\[[\textrm{HCl}]=\dfrac{\textrm{(0.0625 M)(70.0 mL)}-\textrm{(0.125 M)(25.0 mL)}}{\textrm{70.0 mL + 25.0 mL}}=\textrm{0.0132 M}\]

giving a pH of 1.88. Some additional results are shown here.

Volume of HCl (mL)	pH	Volume of HCl (mL)	pH
0	13.10	60	2.13
10	12.85	70	1.88
20	12.62	80	1.75
30	12.36	90	1.66
40	11.98	100	1.60
50	7.00

Click here to return to the chapter.

Practice Exercise 9.2

The volume of HCl needed to reach the equivalence point is

\[V_\textrm{eq}=V_\textrm a=\dfrac{M_\textrm bV_\textrm b}{M_\textrm a}=\dfrac{\textrm{(0.125 M)(25.0 mL)}}{\textrm{0.0625 M}}=\textrm{50.0 mL}\]

Before adding HCl the pH is that for a solution of 0.100 M NH₃.

\[K_\textrm b=\mathrm{\dfrac{[OH^-][NH_4^+]}{[NH_3]}}=\dfrac{(x)(x)}{0.125-x}=1.75\times10^{-5}\]

\[x=[\mathrm{OH^-}]=1.47\times10^{-3}\textrm{ M}\]

The pH at the beginning of the titration, therefore, is 11.17.

Before the equivalence point the pH is determined by an NH₃/NH₄⁺ buffer. For example, after adding 10.0 mL of HCl

\[[\mathrm{NH_3}]=\dfrac{\textrm{(0.125 M)(25.0 mL)}-\textrm{(0.0625 M)(10.0 mL)}}{\textrm{25.0 mL + 10.0 mL}}=\textrm{0.0714 M}\]

\[[\mathrm{NH_4^+}]=\dfrac{\textrm{(0.0625 M)(10.0 mL)}}{\textrm{25.0 mL + 10.0 mL}}=\textrm{0.0179 M}\]

\[\textrm{pH}=9.244+\log\dfrac{0.0714\textrm{ M}}{0.0179\textrm{ M}}=9.84\]

At the equivalence point the predominate ion in solution is NH₄⁺. To calculate the pH we first determine the concentration of NH₄⁺

\[[\mathrm{NH_4^+}]=\dfrac{\textrm{(0.125 M)(25.0 mL)}}{\textrm{25.0 mL + 50.0 mL}}=\textrm{0.0417 M}\]

and then calculate the pH

\[K_\textrm a=\mathrm{\dfrac{[H_3O^+][NH_3]}{[NH_4^+]}}=\dfrac{(x)(x)}{0.0417 - x}=5.70\times10^{-10}\]

\[x=[\mathrm{H_3O^+}]=4.88\times10^{-6}\textrm{ M}\]

obtaining a value of 5.31.

After the equivalence point, the pH is determined by the excess HCl. For example, after adding 70.0 mL of HCl

\[[\textrm{HCl}]=\dfrac{\textrm{(0.0625 M)(70.0 mL)}-\textrm{(0.125 M)(25.0 mL)}}{\textrm{25.0 mL + 70.0 mL}}=0.0132\textrm{ M}\]

and the pH is 1.88. Some additional results are shown here.

Volume of HCl (mL)	pH	Volume of HCl (mL)	pH
0	11.17	60	2.13
10	9.84	70	1.88
20	9.42	80	1.75
30	9.07	90	1.66
40	8.64	100	1.60
50	5.31

Click here to return to the chapter.

Practice Exercise 9.3

Figure 9.48 shows a sketch of the titration curve. The two points before the equivalence point (V_HCl = 5 mL, pH = 10.24 and V_HCl = 45 mL, pH = 8.24) are plotted using the pK_a of 9.244 for NH₄⁺. The two points after the equivalence point (V_HCl = 60 mL, pH = 2.13 and V_HCl = 80 mL, pH = 1.75 ) are from the answer to Practice Exercise 9.2.

Figure 9.48 Titration curve for Practice Exercise 9.3. The black dots and curve are the approximate sketch of the titration curve. The points in red are the calculations from Practice Exercise 9.2.

Click here to return to the chapter.

Practice Exercise 9.4

Figure 9.49 shows a sketch of the titration curve. The titration curve has two equivalence points, one at 25.0 mL (H₂A → HA⁻) and one at 50.0 mL (HA⁻ → A²⁻). In sketching the curve, we plot two points before the first equivalence point using the pK_a of 3 for H₂A

\[\mathrm{\mathit V_{HCl} = 2.5\;mL,\;pH = 2\;and\;\mathit V_{HCl} = 22.5\;mL,\;pH = 4}\]

two points between the equivalence points using the pK_a of 5 for HA^–

\[\mathrm{\mathit V_{HCl} = 27.5\;mL,\;pH = 3,\;and\;\mathit V_{HCl} = 47.5\;mL,\;pH = 5}\]

and two points after the second equivalence point

\[\mathrm{\mathit V_{HCl} = 70\;mL,\;pH = 12.22\;and\;\mathit V_{HCl} = 90\;mL,\;pH = 12.46}\]

Drawing a smooth curve through these points presents us with the following dilemma—the pH appears to increase as the titrant’s volume approaches the first equivalence point and then appears to decrease as it passes through the first equivalence point. This is, of course, absurd; as we add NaOH the pH cannot decrease. Instead, we model the titration curve before the second equivalence point by drawing a straight line from the first point (V_HCl = 2.5 mL, pH = 2) to the fourth (V_HCl = 47.5 mL, pH = 5), ignoring the second and third points. The results is a reasonable approximation of the exact titration curve.

Figure 9.49 Titration curve for Practice Exercise 9.4. The black points and curve are the approximate titration curve, and the red curve is the exact titration curve.

Click here to return to the chapter.

Practice Exercise 9.5

The pH at the equivalence point is 5.31 (see Practice Exercise 9.2) and the sharp part of the titration curve extends from a pH of approximately 7 to a pH of approximately 4. Of the indicators in Table 9.4, methyl red is the best choice because its pK_a value of 5.0 is closest to the equivalence point’s pH and because the pH range of 4.2–6.3 for its change in color will not produce a significant titration error.

Click here to return to the chapter.

Practice Exercise 9.6

Because salicylic acid is a diprotic weak acid, we must first determine to which equivalence point it is being titrated. Using salicylic acid’s pK_a values as a guide, the pH at the first equivalence point is between a pH of 2.97 and 13.74, and the second equivalence points is at a pH greater than 13.74. From Table 9.4, phenolphthalein’s end point falls in the pH range 8.3–10.0. The titration, therefore, is to the first equivalence point for which the moles of NaOH equal the moles of salicylic acid; thus

\[\dfrac{\textrm{0.1354 mol NaOH}}{\mathrm{L}}\times0.02192\textrm{ L}=2.968\times10^{-3}\textrm{ mol NaOH}\]

\[\mathrm{2.968\times10^{-3}\;mol\;NaOH\times\dfrac{1\;mol\;C_7H_6O_3}{mol\;NaOH}\times\dfrac{138.12\;g\;C_7H_6O_3}{mol\;C_7H_6O_3}=0.4099\;g\;C_7H_6O_3}\]

\[\mathrm{\dfrac{0.4099\;g\;C_7H_6O_3}{0.4208\;g\;sample}\times100=97.41\%\;w/w\;C_7H_6O_3}\]

Because the purity of the sample is less than 99%, we reject the shipment.

Click here to return to the chapter.

Practice Exercise 9.7

The moles of HNO₃ produced by pulling the air sample through the solution of H₂O₂ is

\[\mathrm{\dfrac{0.01012\;mol\;NaOH}{L}\times0.00914\;L\times\dfrac{1\;mol\;HNO_3}{mol\;NaOH}=9.25\times10^{-5}\;mol\;HNO_3}\]

A conservation of mass on nitrogen requires that each mole of NO₂ in the sample of air produces one mole of HNO₃; thus, the mass of NO₂ in the sample is

\[\mathrm{9.25\times10^{-5}\;mol\;HNO_3\times\dfrac{1\;mol\;NO_2}{mol\;HNO_3}\times\dfrac{46.01\;g\;NO_2}{mol\;NO_2}=4.26\times10^{-3}\;g\;NO_2}\]

and the concentration of NO₂ is

\[\mathrm{\dfrac{4.26\times10^{-3}\;g\;NO_2}{5\;L\;air}\times\dfrac{1000\;mg}{g}=0.852\;mg\;NO_2/L\;air}\]

Click here to return to the chapter.

Practice Exercise 9.8

The total moles of HCl used in this analysis is

\[\mathrm{\dfrac{1.396\;mol\;NaOH}{L}\times0.01000\;L=1.396\times10^{-2}\;mol\;HCl}\]

Of this,

\[\mathrm{\dfrac{0.1004\;mol\;NaOH}{L}\times0.03996\;L\times\dfrac{1\;mol\;HCl}{mol\;NaOH}=4.012\times10^{-3}\;mol\;HCl}\]

are consumed in the back titration with NaOH, which means that

\[\mathrm{1.396\times10^{-2}\;mol\;HCl-4.012\times10^{-3}\;mol\;HCl=9.95\times10^{-3}\;mol\;HCl}\]

react with the CaCO₃. Because CO₃^2– is dibasic, each mole of CaCO₃ consumes two moles of HCl; thus

\[\mathrm{9.95\times10^{-3}\;mol\;HCl\times\dfrac{1\;mol\;CaCO_3}{2\;mol\;HCl}\times\dfrac{100.09\;g\;CaCO_3}{mol\;CaCO_3}=0.498\;g\;CaCO_3}\]

\[\mathrm{\dfrac{0.498\;g\;CaCO_3}{0.5143\;g\;sample}\times100=96.8\%\;w/w\;CaCO_3}\]

Click here to return to the chapter.

Practice Exercise 9.9

Of the two analytes, 2-methylanilinium is the stronger acid and is the first to react with the titrant. Titrating to the bromocresol purple end point, therefore, provides information about the amount of 2-methylanilinium in the sample.

\[\mathrm{\dfrac{0.200\;mol\;NaOH}{L}\times0.01965\;L\times\dfrac{1\;mol\;C_7H_{10}NCl}{mol\;NaOH}\times\dfrac{143.61\;g\;C_7H_{10}NCl}{mol\;C_7H_{10}NCl}=0.564\;g\;C_7H_{10}NCl}\]

\[\mathrm{\dfrac{0.564\;g\;C_7H_{10}NCl}{2.006\;g\;sample}\times100=28.1\%\;w/w\;C_7H_{10}NCl}\]

Titrating from the bromocresol purple end point to the phenolphthalein end point, a total of 48.41 mL – 19.65 mL, or 28.76 mL, gives the amount of NaOH reacting with 3-nitrophenol. The amount of 3-nitrophenol in the sample, therefore, is

\[\mathrm{\dfrac{0.200\;mol\;NaOH}{L}\times0.02876\;L\times\dfrac{1\;mol\;C_6H_5NO_3}{mol\;NaOH}\times\dfrac{139.11\;g\;C_6H_5NO_3}{mol\;C_6H_5NO_3}=0.800\;g\;C_6H_5NO_3}\]

\[\mathrm{\dfrac{0.800\;g\;C_6H_5NO_3}{2.006\;g\;sample}\times100=38.8\%\;w/w\;C_6H_5NO_3}\]

Click here to return to the chapter.

Practice Exercise 9.10

The first of the two visible end points is approximately 37 mL of NaOH. The analyte’s equivalent weight, therefore, is

\[\mathrm{\dfrac{0.1032\;mol\;NaOH}{L}\times0.037\;L\times\dfrac{1\;equivalent}{mol\;NaOH}=3.8\times10^{-3}\;equivalents}\]

\[EW=\mathrm{\dfrac{0.5000\;g}{3.8\times10^{-3}\;equivalents}=1.3\times10^2\;g/equivalent}\]

Click here to return to the chapter.

Practice Exercise 9.11

At ½V_eq, or approximately 18.5 mL, the pH is approximately 2.2; thus, we estimate that the analyte’s pK_a is 2.2.

Click here to return to the chapter.

Practice Exercise 9.12

Let’s begin with the calculations at a pH of 10. At a pH of 10 some of the EDTA is present in forms other than Y^4–. To evaluate the titration curve, therefore, we need the conditional formation constant for CdY^2–, which, from Table 9.11 is K_f´ = 1.1 × 10¹⁶. Note that the conditional formation constant is larger in the absence of an auxiliary complexing agent.

The titration’s equivalence point requires

\[V_\textrm{eq}=V_\textrm{EDTA}=\dfrac{M_\textrm{Cd}V_\textrm{Cd}}{M_\textrm{EDTA}}=\mathrm{\dfrac{(5.00\times10^{-3}\;M)(50.0\;mL)}{0.0100\;M}=25.0\;mL}\]

of EDTA.

Before the equivalence point, Cd²⁺ is present in excess and pCd is determined by the concentration of unreacted Cd²⁺. For example, after adding 5.00 mL of EDTA, the total concentration of Cd²⁺ is

\[\begin{align}
\ce{[Cd^{2+}]}&=\mathrm{\dfrac{(5.00\times10^{-3}\;M)(50.0\;mL)-(0.0100\;M)(5.00\;mL)}{50.0\;mL+5.00\;mL}}\\
&=3.64\times10^{-3}\textrm{ M}
\end{align}\]

which gives a pCd of 2.43.

At the equivalence point all the Cd²⁺ initially in the titrand is now present as CdY^2–. The concentration of Cd²⁺, therefore, is determined by the dissociation of the CdY^2– complex. First, we calculate the concentration of CdY^2–.

\[\mathrm{[CdY^{2-}]=\dfrac{(5.00\times10^{-3}\;M)(50.0\;mL)}{50.0\;mL+25.0\;mL}=3.33\times10^{-3}\;M}\]

Next, we solve for the concentration of Cd²⁺ in equilibrium with CdY^2–.

\[K_{\textrm{f}}'=\dfrac{\mathrm{[CdY^{2-}]}}{\mathrm{[Cd^{2+}}]C_\textrm{EDTA}}=\dfrac{3.33\times10^{-3}-x}{(x)(x)}=1.1\times10^{16}\]

Solving gives [Cd²⁺] as 5.50 × 10^–10 M, or a pCd of 9.26 at the equivalence point.

After the equivalence point, EDTA is in excess and the concentration of Cd²⁺ is determined by the dissociation of the CdY^2– complex. First, we calculate the concentrations of CdY^2– and of unreacted EDTA. For example, after adding 30.0 mL of EDTA

\[\mathrm{[CdY^{2-}]=\dfrac{(5.00\times10^{-3}\;M)(50.0\;mL)}{50.0\;mL+30.0\;mL}=3.13\times10^{-3}\;M}\]

\[\begin{align}
C_\textrm{EDTA}&=\mathrm{\dfrac{(0.0100\;M)(30.0\;mL)-(5.00\times10^{-3}\;M)(50.0\;mL)}{50.0\;mL+30.0\;mL}}\\
&=6.25\times10^{-4}\textrm{ M}
\end{align}\]

Substituting into the equation for the conditional formation constant and solving for [Cd²⁺] gives

\[\mathrm{\dfrac{3.13\times10^{-3}\;M}{[Cd^{2+}](6.25\times10^{-4}\;M)}=1.1\times10^{16}}\]

[Cd²⁺] as 4.55× 10^–16 M, or a pCd of 15.34.

The calculations at a pH of 7 are identical, except the conditional formation constant for CdY^2– is 1.5 × 10¹³ instead of 1.1 × 10¹⁶. The following table summarizes results for these two titrations as well as the results from Table 9.13 for the titration of Cd²⁺ at a pH of 10 in the presence of 0.0100 M NH₃ as an auxiliary complexing agent.

Volume of EDTA (mL)	pCd at pH 10	pCd at pH 10 w/ 0.0100 M NH3	pCd at pH 7
0	2.30	3.36	2.30
5.00	2.43	3.49	2.43
10.0	2.60	3.66	2.60
15.0	2.81	3.87	2.81
20.0	3.15	4.20	3.15
23.0	3.56	4.62	3.56
25.0	9.26	9.77	7.83
27.0	14.94	14.95	12.08
30.0	15.34	15.33	12.48
35.0	15.61	15.61	12.78
40.0	15.76	15.76	12.95
45.0	15.86	15.86	13.08
50.0	15.94	15.94	13.18

Examining these results allows us to draw several conclusions. First, in the absence of an auxiliary complexing agent the titration curve before the equivalence point is independent of pH (compare columns 2 and 4). Second, for any pH, the titration curve after the equivalence point is the same regardless of whether or not an auxiliary complexing agent is present (compare columns 2 and 3). Third, the largest change in pH through the equivalence point occurs at higher pHs and in the absence of an auxiliary complexing agent. For example, from 23.0 mL to 27.0 mL of EDTA the change in pCd is 11.38 at a pH of 10, 10.33 at a pH of 10 and in the presence of 0.0100 M NH₃, and 8.52 at a pH of 7.

Click here to return to the chapter.

Practice Exercise 9.13

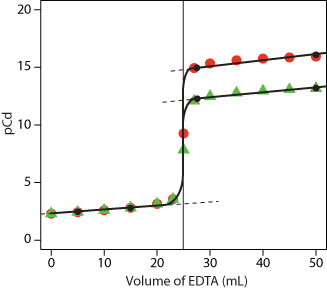
Figure 9.50 shows a sketch of the titration curves. The two points before the equivalence point (V_EDTA = 5 mL, pCd = 2.43 and V_EDTA = 15 mL, pCd = 2.81) are the same for both pHs and are taken from the results of Practice Exercise 9.12. The two points after the equivalence point for a pH of 7 (V_EDTA = 27.5 mL, pCd = 12.2 and V_EDTA = 50 mL, pCd = 13.2 ) are plotted using the logK_f´ of 13.2 for CdY^2-. The two points after the equivalence point for a pH of 10 (V_EDTA = 27.5 mL, pCd = 15.0 and V_EDTA = 50 mL, pCd = 16.0) are plotted using the logK_f´ of 16.0 for CdY^2-.

Figure 9.50 Titration curve for Practice Exercise 9.13. The black dots and curve are the approximate sketches of the two titration curves. The points in red are the calculations from Practice Exercise 9.12 for a pH of 10, and the points in green are the calculations from Practice Exercise 9.12 for a pH of 7.

Click here to return to the chapter.

Practice Exercise 9.14

In an analysis for hardness we treat the sample as if Ca²⁺ is the only metal ion reacting with EDTA. The grams of Ca²⁺ in the sample, therefore, is

\[\mathrm{\dfrac{0.0109\;mol\;EDTA}{L}\times0.02363\;L\times\dfrac{1\;mol\;Ca^{2+}}{mol\;EDTA}=2.58\times10^{-4}\;mol\;Ca^{2+}}\]

\[\mathrm{2.58\times10^{-4}\;mol\;Ca^{2+}\times\dfrac{1\;mol\;CaCO_3}{mol\;Ca^{2+}}\times\dfrac{100.09\;g\;CaCO_3}{mol\;CaCO_3}=0.0258\;g\;CaCO_3}\]

and the sample’s hardness is

\[\mathrm{\dfrac{0.0258\;g\;CaCO_3}{0.1000\;L}\times\dfrac{1000\;mg}{g}=258\;mg\;CaCO_3/L}\]

Click here to return to the chapter.

Practice Exercise 9.15

The titration of CN^– with Ag⁺ produces a metal-ligand complex of Ag(CN)₂^2–; thus, each mole of AgNO₃ reacts with two moles of NaCN. The grams of NaCN in the sample is

\[\mathrm{\dfrac{0.1018\;mol\;AgNO_3}{L}\times0.03968\;L\times\dfrac{2\;mol\;NaCN}{mol\;AgNO_3}\times\dfrac{49.01\;g\;NaCN}{mol\;NaCN}=0.3959\;g\;NaCN}\]

and the purity of the sample is

\[\mathrm{\dfrac{0.3959\;g\;NaCN}{0.4482\;g\;sample}\times100=88.33\%\;w/w\;NaCN}\]

Click here to return to the chapter.

Practice Exercise 9.16

The total moles of EDTA used in this analysis is

\[\mathrm{\dfrac{0.02011\;mol\;EDTA}{L}\times0.02500\;L = 5.028\times10^{-4}\;mol\;EDTA}\]

Of this,

\[\mathrm{\dfrac{0.01113\;mol\;Mg^{2+}}{L}\times0.00423\;L\times\dfrac{1\;mol\;EDTA}{mol\;Mg^{2+}}=4.708\times10^{-5}\;mol\;EDTA}\]

are consumed in the back titration with Mg²⁺, which means that

\[\mathrm{5.028\times10^{-4}\;mol\;EDTA-4.708\times10^{-5}\;mol\;EDTA=4.557\times10^{-4}\;mol\;EDTA}\]

react with the BaSO₄. Each mole of BaSO₄ reacts with one mole of EDTA; thus

\[\mathrm{4.557\times10^{-4}\;mol\;EDTA\times\dfrac{1\;mol\;BaSO_4}{mol\;EDTA}\times\dfrac{1\;mol\;Na_2SO_4}{mol\;BaSO_4}\times\dfrac{142.04\;g\;Na_2SO_4}{mol\;Na_2SO_4}=0.06473\;g\;Na_2SO_4}\]

\[\mathrm{\dfrac{0.06473\;g\;Na_2SO_4}{0.1557\;g\;sample}\times100=41.23\%\;w/w\;Na_2SO_4}\]

Click here to return to the chapter.

Practice Exercise 9.17

The volume of Tl³⁺ needed to reach the equivalence point is

\[V_\textrm{eq}=V_\textrm{Tl}=\dfrac{M_\textrm{Sn}V_\textrm{Sn}}{M_\textrm{Tl}}=\mathrm{\dfrac{(0.050\;M)(50.0\;mL)}{0.100\;M}=25.0\;mL}\]

Before the equivalence point, the concentration of unreacted Sn²⁺ and the concentration of Sn⁴⁺ are easy to calculate. For this reason we find the potential using the Nernst equation for the Sn⁴⁺/Sn²⁺ half-reaction.For example, the concentrations of Sn²⁺ and Sn⁴⁺ after adding 10.0 mL of titrant are

\[\mathrm{[Sn^{2+}]=\dfrac{(0.050\;M)(50.0\;mL)-(0.100\;M)(10.0\;mL)}{50.0\;mL+10.0\;mL}=0.0250\;M}\]

\[\mathrm{[Sn^{4+}]=\dfrac{(0.100\;M)(10.0\;mL)}{50.0\;mL+10.0\;mL}=0.0167\;M}\]

and the potential is

\[E=\mathrm{+0.139\;V-\dfrac{0.05916}{2}\log\dfrac{0.0250\;M}{0.0167\;M}=+0.134\;V}\]

After the equivalence point, the concentration of Tl⁺ and the concentration of excess Tl³⁺ are easy to calculate. For this reason we find the potential using the Nernst equation for the Tl³⁺/Tl⁺ half-reaction. For example, after adding 40.0 mL of titrant, the concentrations of Tl⁺ and Tl³⁺ are

\[\mathrm{[Tl^+]=\dfrac{(0.0500\;M)(50.0\;mL)}{50.0\;mL+40.0\;mL}=0.0278\;M}\]

\[\mathrm{[Tl^{3+}]=\dfrac{(0.100\;M)(40.0\;mL)-(0.0500\;M)(50.0\;mL)}{50.0\;mL+40.0\;mL}=0.0167\;M}\]

and the potential is

\[E=\mathrm{+0.77\;V-\dfrac{0.05916}{2}\log\dfrac{0.0278\;M}{0.0167\;M}=+0.76\;V}\]

At the titration’s equivalence point, the potential, E_eq, potential is

\[E_\textrm{eq}=\mathrm{\dfrac{0.139\;V+0.77\;V}{2}=0.45\;V}\]

Some additional results are shown here.

Volume of Tl^3^+ (mL)	E (V)	Volume of Tl^3^+ (mL)	E (V)
5	0.121	30	0.75
10	0.134	35	0.75
15	0.144	40	0.76
20	0.157	45	0.76
25	0.45	50	0.76

Click here to return to the chapter.

Practice Exercise 9.18

Figure 9.51 shows a sketch of the titration curve. The two points before the equivalence point

\[\mathrm{\mathit V_{Tl} = 2.5\;mL,\;\mathit E = +0.109\;V\;and\;\mathit V_{Tl} = 22.5\; mL,\;\mathit E = +0.169\;V}\]

are plotted using the redox buffer for Sn⁴⁺/Sn²⁺, which spans a potential range of +0.139 ± 0.5916/2. The two points after the equivalence point

\[\mathrm{\mathit V_{Tl} = 27.5\;mL,\;\mathit E = +0.74\;V\;and\;\mathit V_{EDTA} = 50\;mL,\;\mathit E = +0.77\;V}\]

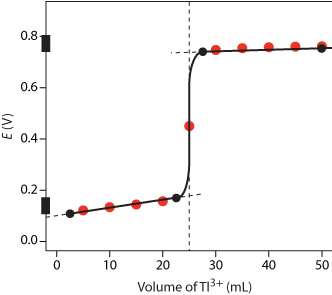
are plotted using the redox buffer for Tl³⁺/Tl⁺, which spans the potential range of +0.139 ± 0.5916/2.

Figure 9.51 Titration curve for Practice Exercise 9.18. The black dots and curve are the approximate sketch of the titration curve. The points in red are the calculations from Practice Exercise 9.17.

Click here to return to the chapter.

Practice Exercise 9.19

The two half reactions are

\[\textrm{Ce}^{4+}(aq)+e^-\rightarrow \textrm{Ce}^{3+}(aq)\]

\[\mathrm{U^{4+}}(aq)+\mathrm{2H_2O}\rightarrow \mathrm{UO_2^{2+}}(aq)+\mathrm{4H^+}(aq)+2e^-\]

for which the Nernst equations are

\[E=E^{\circ}\mathrm{_{Ce^{4+}/Ce^{3+}}-\dfrac{0.05916}{1}\log\dfrac{[Ce^{3+}]}{[Ce^{4+}]}}\]

\[E=E^{\circ}\mathrm{_{UO_2^{2+}/U^{4+}}-\dfrac{0.05916}{2}\log\dfrac{[U^{4+}]}{[UO_2^{2+}][H^+]^4}}\]

Before adding these two equations together we must multiply the second equation by 2 so that we can combine the log terms; thus

\[3E=E^{\circ}\mathrm{_{Ce^{4+}/Ce^{3+}}}+2E^{\circ}\mathrm{_{UO_2^{2+}/U^{4+}}-0.05916\log\dfrac{[Ce^{3+}][U^{4+}]}{[Ce^{4+}][UO_2^{2+}][H^+]^4}}\]

At the equivalence point we know that

\[\mathrm{[Ce^{3+}]=2\times[UO_2^{2+}]}\]

\[\mathrm{[Ce^{4+}]=2\times[U^{4+}]}\]

Substituting these equalities into the previous equation and rearranging gives us a general equation for the potential at the equivalence point.

\[3E=E^{\circ}\mathrm{_{Ce^{4+}/Ce^{3+}}}+2E^{\circ}\mathrm{_{UO_2^{2+}/U^{4+}}-0.05916\log\dfrac{2[UO_2^{2+}][U^{4+}]}{2[U^{4+}][UO_2^{2+}][H^+]^4}}\]

\[E=\dfrac{E^{\circ}_\mathrm{Ce^{4+}/Ce^{3+}}+2E^{\circ}_\mathrm{UO_2^{2+}/U^{4+}}}{3}-\dfrac{0.05916}{3}\log\dfrac{1}{[\textrm H^+]^4}\]

\[E=\dfrac{E^{\circ}_\mathrm{Ce^{4+}/Ce^{3+}}+2E^{\circ}_\mathrm{UO_2^{2+}/U^{4+}}}{3}+\dfrac{0.05916\times4}{3}\log[\textrm H^+]\]

\[E=\dfrac{E^{\circ}_\mathrm{Ce^{4+}/Ce^{3+}}+2E^{\circ}_\mathrm{UO_2^{2+}/U^{4+}}}{3}-0.07888\textrm{ pH}\]

At a pH of 1 the equivalence point has a potential of

\[E_\textrm{eq}=\dfrac{1.72 + 2\times0.327}{3}-0.07888\times1=0.712\textrm{ V}\]

Click here to return to the chapter.

Practice Exercise 9.20

Because we have not been provided with a balanced reaction, let’s use a conservation of electrons to deduce the stoichiometry. Oxidizing C₂O₄^2–, in which each carbon has a +3 oxidation state, to CO₂, in which carbon has an oxidation state of +4, requires one electron per carbon, or a total of two electrons for each mole of C₂O₄^2–. Reducing MnO₄^–, in which each manganese is in the +7 oxidation state, to Mn²⁺ requires five electrons. A conservation of electrons for the titration, therefore, requires that two moles of KMnO₄ (10 moles of e^-) reacts with five moles of Na₂C₂O₄ (10 moles of e^-).

The moles of KMnO₄ used in reaching the end point is

\[\mathrm{(0.0400\;M\;KMnO_4)\times(0.03562\;L\;KMnO_4)=1.42\times10^{-3}\;mol\;KMnO_4}\]

which means that the sample contains

\[\mathrm{1.42\times10^{-3}\;mol\;KMnO_4\times\dfrac{5\;mol\;Na_2C_2O_4}{2\;mol\;KMnO_4}=3.55\times10^{-3}\;mol\;Na_2C_2O_4}\]

Thus, the %w/w Na₂C₂O₄ in the sample of ore is

\[\mathrm{3.55\times10^{-3}\;mol\;Na_2C_2O_4\times\dfrac{134.00\;g\;Na_2C_2O_4}{mol\;Na_2C_2O_4}=0.476\;g\;Na_2C_2O_4}\]

\[\mathrm{\dfrac{0.476\;g\;Na_2C_2O_4}{0.5116\;g\;sample}\times100=93.0\%\;w/w\;Na_2C_2O_4}\]

Click here to return to the chapter.

Practice Exercise 9.21

For a back titration we need to determine the stoichiometry between Cr₂O₇^2– and the analyte, C₂H₆O, and between Cr₂O₇^2– and the titrant, Fe²⁺. In oxidizing ethanol to acetic acid, the oxidation state of carbon changes from –2 in C₂H₆O to 0 in C₂H₄O₂. Each carbon releases two electrons, or a total of four electrons per C₂H₆O. In reducing Cr₂O₇^2–, in which each chromium has an oxidation state of +6, to Cr³⁺, each chromium loses three electrons, for a total of six electrons per Cr₂O₇^2–. Oxidation of Fe²⁺ to Fe³⁺ requires one electron. A conservation of electrons requires that each mole of K₂Cr₂O₇ (6 moles of e^-) reacts with six moles of Fe²⁺ (6 moles of e^-), and that four moles of K₂Cr₂O₇ (24 moles of e^-) react with six moles of C₂H₆O (24 moles of e^-).

The total moles of K₂Cr₂O₇ reacting with C₂H₆O and with Fe²⁺ is

\[\mathrm{(0.0200\;M\;K_2Cr_2O_7)\times(0.05000\;L\;\ce{I_3^-})=1.00\times10^{-3}\;mol\;K_2Cr_2O_7}\]

The back titration with Fe²⁺ consumes

\[\mathrm{0.02148\;L\;Fe^{2+}\times\dfrac{0.1014\;mol\;Fe^{2+}}{L\;Fe^{2+}}\times\dfrac{1\;mol\;K_2Cr_2O_7}{6\;mol\;Fe^{2+}}=3.63\times10^{-4}\;mol\;K_2Cr_2O_7}\]

Subtracting the moles of K₂Cr₂O₇ reacting with Fe²⁺ from the total moles of K₂Cr₂O₇ gives the moles reacting with the analyte.

\[\mathrm{1.00\times10^{-3}\;K_2Cr_2O_7-3.63\times10^{-4}\;mol\;K_2Cr_2O_7=6.37\times10^{-4}\;mol\;K_2Cr_2O_7}\]

The grams of ethanol in the 10.00-mL sample of diluted brandy is

\[\mathrm{6.37\times10^{-4}\;mol\;K_2Cr_2O_7\times\dfrac{6\;mol\;C_2H_6O}{4\;mol\;K_2Cr_2O_7}\times\dfrac{46.50\;g\;C_2H_6O}{mol\;C_2H_6O}=0.0444\;g\;C_2H_6O}\]

The %w/v C₂H₆O in the brandy is

\[\mathrm{\dfrac{0.0444\;g\;C_2H_6O}{10.00\;mL\;dilute\;brandy}\times\dfrac{500.0\;mL\;dilute\;brandy}{5.00\;mL\;brandy}\times100=44.4\%\;w/v\;C_2H_6O}\]

Click here to return to the chapter.

Practice Exercise 9.22

The first task is to calculate the volume of NaCl needed to reach the equivalence point; thus

\[V_\textrm{eq}=V_\textrm{NaCl}=\dfrac{M_\textrm{Ag}V_\textrm{Ag}}{M_\textrm{NaCl}}=\mathrm{\dfrac{(0.0500\;M)(50.0\;mL)}{(0.100\;M)}=25.0\;mL}\]

Before the equivalence point the titrand, Ag⁺, is in excess. The concentration of unreacted Ag⁺ after adding 10.0 mL of NaCl, for example, is

\[\mathrm{[Ag^+]=\dfrac{(0.0500\;M)(50.0\;mL)-(0.100\;M)(10.0\;mL)}{50.0\;mL+10.0\;mL}=2.50\times10^{-2}\;M}\]

which corresponds to a pAg of 1.60. To find the concentration of Cl^– we use the K_sp for AgCl; thus

\[[\mathrm{Cl^-}]=\dfrac{K_\textrm{sp}}{[\mathrm{Ag^+}]}=\dfrac{1.8\times10^{-10}}{2.50\times10^{-2}}=7.2\times10^{-9}\textrm{ M}\]

or a pCl of 8.14.

At the titration’s equivalence point, we know that the concentrations of Ag⁺ and Cl^– are equal. To calculate their concentrations we use the K_sp expression for AgCl; thus

\[K_\textrm{sp}=\mathrm{[Ag^+][Cl^-]}=(x)(x)=1.8\times10^{-10}\]

Solving for x gives a concentration of Ag⁺ and the concentration of Cl^– as 1.3 × 10^–5 M, or a pAg and a pCl of 4.89.

After the equivalence point, the titrant is in excess. For example, after adding 35.0 mL of titrant

\[\mathrm{[Cl^-]=\dfrac{(0.100\;M)(35.0\;mL)-(0.0500\;M)(50.0\;mL)}{50.0\;mL+35.0\;mL}=1.18\times10^{-2}\;M}\]

or a pCl of 1.93. To find the concentration of Ag⁺ we use the K_sp for AgCl; thus

\[[\mathrm{Ag^+}]=\dfrac{K_\textrm{sp}}{[\mathrm{Cl^-}]}=\dfrac{1.8\times10^{-10}}{1.18\times10^{-2}}=1.5\times10^{-8}\textrm{ M}\]

or a pAg of 7.82. The following table summarizes additional results for this titration.

Volume of NaCl (mL)	pAg	pCl
0	1.30	–
5.00	1.44	8.31
10.0	1.60	8.14
15.0	1.81	7.93
20.0	2.15	7.60
25.0	4.89	4.89
30.0	7.54	2.20
35.0	7.82	1.93
40.0	7.97	1.78
45.0	8.07	1.68
50.0	8.14	1.60

Click here to return to the chapter.

Practice Exercise 9.23

The titration uses

\[\mathrm{\dfrac{0.1078\;M\;KSCN}{L}\times0.02719\;L=2.931\times10^{-3}\;mol\;KSCN}\]

The stoichiometry between SCN^– and Ag⁺ is 1:1; thus, there are

\[\mathrm{2.931\times10^{-3}\;mol\;Ag^+\times\dfrac{107.87\;g\;Ag}{mol\;Ag}=0.3162\;g\;Ag}\]

in the 25.00 mL sample. Because this represents ¼ of the total solution, there are 0.3162 × 4 or 1.265 g Ag in the alloy. The %w/w Ag in the alloy is

\[\mathrm{\dfrac{1.265\;g\;Ag}{1.963\;g\;sample}\times100=64.44\%\;w/w\;Ag}\]

Click here to return to the chapter.

Contributors

David Harvey (DePauw University)