7.36: Bosonic and Fermionic Photon Behavior at Beam Splitters- A Tensor Algebra Analysis

Photons from separate sources, u and d, arrive simultaneously at the beam splitter shown in the figure below.

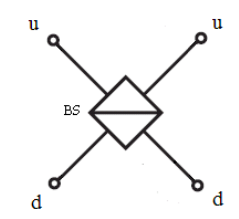
Because these photons are indistinguishable they donʹt possess separate identities, and we are forced by quantum mechanical principles to represent their collective state at the beam splitter (BS) by the following entangled wave function. The plus sign in this superposition indicates that photons are bosons; their wave functions are symmetric with respect to the interchange of the photon labels.

\[ | \Psi _B \rangle = \frac{1}{ \sqrt{2}} \left[ |u \rangle_1 |d \rangle_2 + |d \rangle_1 |u \rangle_2 \right] \nonumber \]

The following vector representations are used for the photon states.

\[ |u \rangle = \begin{pmatrix} 1 \\ 0 \end{pmatrix} \nonumber \]

\[ |d \rangle = \begin{pmatrix} 0 \\ 1 \end{pmatrix} \nonumber \]

In tensor format this entangled state becomes,

\[ \Psi_B \rangle = \frac{1}{ \sqrt{2}} \left[ \begin{pmatrix} 1 \\ 0 \end{pmatrix} \otimes \begin{pmatrix} 0 \\ 1 \end{pmatrix} + \begin{pmatrix} 0 \\ 1 \end{pmatrix} \otimes \begin{pmatrix} 1 \\ 0 \end{pmatrix} \right] = \frac{1}{ \sqrt{2}} \left[ \begin{pmatrix} 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} + \begin{pmatrix} 0 \\ 0 \\ 1 \\ 0 \end{pmatrix} \right] = \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \nonumber \]

Transmission and reflection occur at the 50-50 beam splitters. By convention, reflection is assigned a π/2 phase shift relative to transmission. The matrix representing a 50-50 beam splitter operating on an individual photon is given below, along with its effect on the u and d photon states.

\[ \frac{1}{ \sqrt{2}} \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \nonumber \]

\[ \frac{1}{ \sqrt{2}} \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \begin{pmatrix} 1 \\ 0 \end{pmatrix} \rightarrow \begin{pmatrix} \frac{ \sqrt{2}}{2} \\ \frac{ \sqrt{2} i}{2} \end{pmatrix} \nonumber \]

\[ \frac{1}{ \sqrt{2}} \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \begin{pmatrix} 0 \\ 1 \end{pmatrix} \rightarrow \begin{pmatrix} \frac{ \sqrt{2} i}{2} \\ \frac{ \sqrt{2}}{2} \end{pmatrix} \nonumber \]

This result clearly shows that the beam splitter converts pure states (u and d) into superpositions of u and d.

In this experiment the beam splitter operates on both photons, and in tensor format the operator becomes the following 4x4 matrix operator.

\[ \hat{BS} = \hat{BS}_1 \otimes \hat{BS}_2 = \frac{1}{ \sqrt{2}} \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \otimes \frac{1}{ \sqrt{2}} \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} = \frac{1}{2} \begin{pmatrix} 1 \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} & i \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \\ i \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} & 1 \begin{pmatrix} 1 & i \\ i & 1 \end{pmatrix} \end{pmatrix} = \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \nonumber \]

Temporarily thinking of the photon as generic quantum particle (quon to use Nick Herbertʹs phrase), we can identify four possible photon states after the beam splitter, which are shown below in tensor format.

\[ |uu \rangle = \begin{pmatrix} 1 \\ 0 \end{pmatrix} \otimes \begin{pmatrix} 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 1 \\ 0 \\ 0 \\ 0 \end{pmatrix} \nonumber \]

\[ |ud \rangle = \begin{pmatrix} 1 \\ 0 \end{pmatrix} \otimes \begin{pmatrix} 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 1 \\ 0 \\ 0 \end{pmatrix} \nonumber \]

\[ |du \rangle = \begin{pmatrix} 0 \\ 1 \end{pmatrix} \otimes \begin{pmatrix} 1 \\ 0 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 1 \\ 0 \end{pmatrix} \nonumber \]

\[ |dd \rangle = \begin{pmatrix} 0 \\ 1 \end{pmatrix} \otimes \begin{pmatrix} 0 \\ 1 \end{pmatrix} = \begin{pmatrix} 0 \\ 0 \\ 0 \\ 1 \end{pmatrix} \nonumber \]

The actual result of the interaction of the initial entangled state with the beam splitter is,

\[ \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \rightarrow \begin{pmatrix} \frac{ \sqrt{2} i}{2} \\ 0 \\ 0 \\ \frac{ \sqrt{2} i}{2} \end{pmatrix} \nonumber \]

By inspection we can see that the photons are always observed in the same output channel, either |uu > or |dd >, with equal probabilities of 0.5. Not surprisingly we see bosonic behavior by the photons.

\[ \left[ \left| \begin{pmatrix} 1 & 0 & 0 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow \frac{1}{2} \nonumber \]

\[ \left[ \left| \begin{pmatrix} 0 & 0 & 0 & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow \frac{1}{2} \nonumber \]

Naturally this means they are never observed in different output channels, |ud > or |du>. The photons are not exhibiting fermionic behavior (so far).

\[ \left[ \left| \begin{pmatrix} 0 & 1 & 0 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow 0 \nonumber \]

\[ \left[ \left| \begin{pmatrix} 0 & 0 & 1 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow 0 \nonumber \]

However, recombining the photon beams at a second beam splitter appears to invest them with fermionic character.

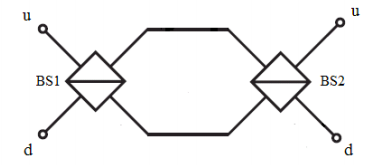
As is shown below, the addition of a second beam splitter is easily implemented in the tensor format.

\[ \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \rightarrow \begin{pmatrix} 0 \\ - \frac{ \sqrt{2}}{2} \\ - \frac{ \sqrt{2}}{2} \\ 0 \end{pmatrix} \nonumber \]

Now we see (by inspection or calculation) fermionic behavior. The photons never appear in the same output channel.

\( |uu \rangle\)

\[ \left[ \left| \begin{pmatrix} 1 & 0 & 0 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow 0 \nonumber \]

\( |dd \rangle\)

\[ \left[ \left| \begin{pmatrix} 0 & 0 & 0 & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow 0 \nonumber \]

They always appear in different output channels, now behaving like fermions.

\( |ud \rangle\)

\[ \left[ \left| \begin{pmatrix} 0 & 1 & 0 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow \frac{1}{2} \nonumber \]

\( |du \rangle\)

\[ \left[ \left| \begin{pmatrix} 0 & 0 & 1 & 0 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{2} \begin{pmatrix} 1 & i & i & -1 \\ i & 1 & -1 & i \\ i & -1 & 1 & i \\ -1 & i & i & 1 \end{pmatrix} \frac{1}{ \sqrt{2}} \begin{pmatrix} 0 \\ 1 \\ 1 \\ 0 \end{pmatrix} \right| \right]^2 \rightarrow \frac{1}{2} \nonumber \]