1.19: Basic Quantum Mechanics in Coordinate, Momentum and Phase Space

The purpose of this paper is to use calculations on the harmonic oscillator to illustrate the relationship between the coordinate, momentum and phase space representations of quantum mechanics.

First, the ground‐state coordinate space eigenfunction for the harmonic oscillator is used for several traditional quantum mechanical calculations. Then the coordinate wave function is Fourier transformed into the momentum representation, and the calculations repeated showing that the same results are obtained. Next, the coordinate (and subsequently the momentum) wave function is used to generate the Wigner phase‐space distribution function. It is then used to repeat the quantum mechanical calculations done in the coordinate and momentum representations, yielding the same results. Finally, a variational calculation is carried out in all three representations for the V = |x| potential energy function using a Gaussian trial wave function. As might be expected the three calculations yield identical results.

All calculations are carried out in atomic units (h = 2\(\pi\)) with the effective mass and force constant set to unity (\(\mu\) = k = 1) for the sake of computational convenience.

The first three harmonic oscillator eigenfunctions are given below. While the ground‐state eigenfunction is used in the example calculations, it is easy for the interested reader to edit the companion Mathcad file to repeat the calculations for the other eigenfunctions.

\[
\Psi_{0}(x) :=\pi^{-\frac{1}{4}} \cdot \exp \left(-\frac{x^{2}}{2}\right) \qquad \Psi_{1}(x) :=\left(\frac{4}{\pi}\right)^{\frac{1}{4}} \cdot x \cdot \exp \left(-\frac{x^{2}}{2}\right) \qquad \Psi_{2}(x) :=(4 \cdot \pi)^{-\frac{1}{4}} \cdot\left(2 \cdot x^{2}-1\right) \cdot \exp \left(-\frac{x^{2}}{2}\right)
\nonumber \]

As is well‐known, in coordinate space the position operator is multiplicative and the momentum operator is differential. In momentum space it is the reverse, while in phase space, both position and momentum are multiplicative operators. In Appendix A Dirac notation is used to derive the position and momentum operators in coordinate and momentum space. Case (1) uses the Weyl transform to show that both the position and momentum operators are multiplicative in phase space. In Appendix B a deductive rationalization for the multiplicative character of the position operator in phase space is presented. The extension to the multiplicative character of the momentum operator is straightforward.

Coordinate Space Calculations

Coordinate space integral: \(\int_{-\infty}^{\infty} \Box\; d x\)

Position operator: \(x \cdot \Box\)

Potential energy operator: \(\frac{x^{2}}{2} \cdot \Box\)

Momentum operator: \(\frac{1}{i} \cdot \frac{d}{d x} \Box\)

Kinetic energy operator: \(-\frac{1}{2} \cdot \frac{d^{2}}{d x^{2}} \Box\)

Display the v = 0 coordinate distribution function.

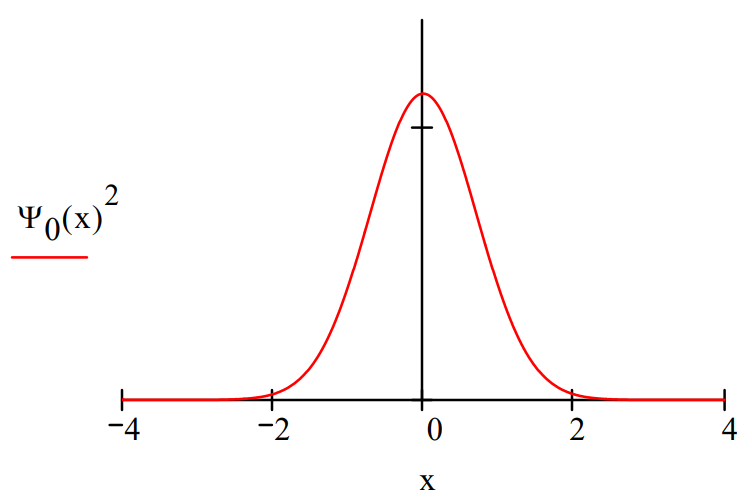
Demonstrate that the wave function is normalized and calculate <x>, <x²>,

, and <p²>. Then use these results to demonstrate that the uncertainty principle is satisfied.

\[
\int_{-\infty}^{\infty} \Psi_{0}(x)^{2} d x=1 \qquad x_{\text { ave }}=\int_{-\infty}^{\infty} x \cdot \Psi_{0}(x)^{2} d x \rightarrow 0 \qquad \mathrm{x} 2_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \mathrm{x}^{2} \cdot \Psi_{0}(\mathrm{x})^{2} \mathrm{d} \mathrm{x} \rightarrow \frac{1}{2}
\nonumber \]

\[
\mathrm{p}_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \Psi_{0}(\mathrm{x}) \cdot \frac{1}{\mathrm{i}} \cdot \frac{\mathrm{d}}{\mathrm{dx}} \Psi_{0}(\mathrm{x}) \mathrm{d} \mathrm{x} \rightarrow 0 \qquad \mathrm{p} 2_{\text { ave }} :=\int_{-\infty}^{\infty} \Psi_{0}(\mathrm{x}) \cdot \frac{\mathrm{d}^{2}}{\mathrm{dx}^{2}} \Psi_{0}(\mathrm{x}) \mathrm{d} \mathrm{x} \rightarrow \frac{1}{2}
\nonumber \]

In the coordinate representation the expectation values involving position appear to be calculated classically. The average value is the sum over each value of x weighted by its probability of occurring, \(\Psi(x)^{2}\). This is clearly not the case for the momentum expectation values in coordinate space. Quantum weirdness is manifest in the momentum calculations and hidden in the coordinate calculations. As mentioned above, Appendix A shows the origin of this computational difference between position and momentum expectation values in coordinate space. Basically it comes down to the fact that in its own (eigen) space an operator has special privileges, it appears to operate multiplicatively

The uncertainty principle requires that \(\Delta x \cdot \Delta p \geq \frac{1}{2}\) (in atomic units). The expectation values from above show that the harmonic oscillator is in compliance.

\[
\Delta x :=\sqrt{x 2_{\text { ave }}-x_{\text { ave }}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \qquad \Delta \mathrm{p} :=\sqrt{\mathrm{p} 2_{\mathrm{ave}}-\mathrm{p}_{\mathrm{ave}}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \qquad \Delta \mathrm{x} \cdot \Delta \mathrm{p} \rightarrow \frac{1}{2}
\nonumber \]

Demonstrate that \(\Psi_{0}(x)\) is an eigenfunction of the energy operator and use the expectation values from above to calculate the expectation value for energy.

\[
\frac{-1}{2} \cdot \frac{\mathrm{d}^{2}}{\mathrm{dx}^{2}} \Psi_{0}(\mathrm{x})+\frac{1}{2} \cdot \mathrm{x}^{2} \cdot \Psi_{0}(\mathrm{x})=\mathrm{E} \cdot \Psi_{0}(\mathrm{x}) \text { solve, } \mathrm{E} \rightarrow \frac{1}{2} \qquad \frac{\mathrm{p} 2_{\mathrm{ave}}}{2}+\frac{\mathrm{x} 2_{\mathrm{ave}}}{2} \rightarrow \frac{1}{2}
\nonumber \]

Momentum Space Calculations

The energy operator for the harmonic oscillator is,

\[
\hat{H}=\frac{\hat{p}^{2}}{2 m}+\frac{1}{2} k \hat{x}^{2}
\nonumber \]

Most quantum mechanical problems are easier to solve in coordinate space. Because of its symmetry, the harmonic oscillator is as easy to solve in momentum space as it is in coordinate space. However, we generate the momentum wave function by Fourier transform of the coordinate‐space wave function. It is then shown that it gives the same results as the wave function in the position basis.

\[
\Phi_{0}(\mathrm{p}) :=\frac{1}{\sqrt{2 \cdot \pi}} \cdot \int_{-\infty}^{\infty} \exp (-\mathrm{i} \cdot \mathrm{p} \cdot \mathrm{x}) \Psi_{0}(\mathrm{x}) \mathrm{dx} \; \text { simplify } \rightarrow \frac{\frac{\mathrm{e}^{2}}{2} \cdot \mathrm{p}^{2}}{\pi^{\frac{1}{4}}}
\nonumber \]

First, we demonstrate that the Fourier transform of this momentum wave function returns the coordinate space wave function.

\[
\frac{1}{\sqrt{2 \cdot \pi}} \cdot \int_{-\infty}^{\infty} \exp (\mathrm{i} \cdot \mathrm{p} \cdot \mathrm{x}) \Phi_{0}(\mathrm{p}) \text { dp simplify } \rightarrow \frac{1}{\pi^{\frac{1}{4}}} \cdot \mathrm{e}^{\frac{-1}{2} \cdot \mathrm{x}^{2}}
\nonumber \]

Display the v = 0 momentum distribution function.

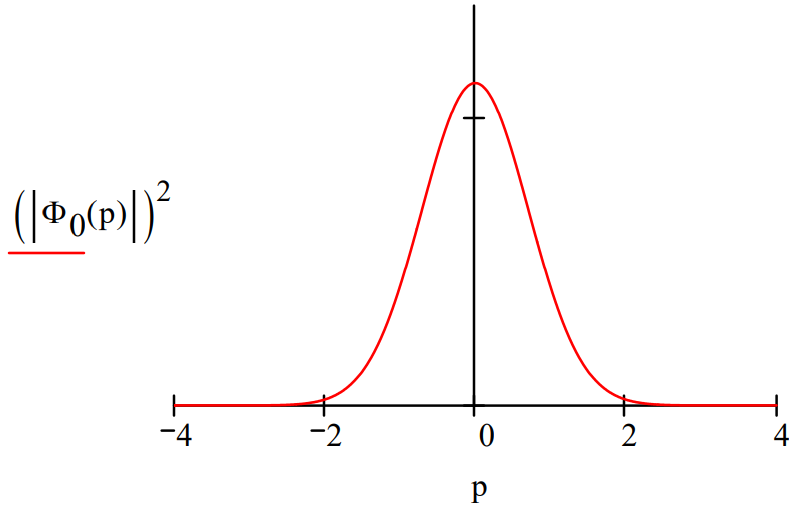
Notice that the coordinate and momentum distribution functions are identical given the parameterization of the calculation (\(\mu\) = k = 1).

Momentum space integral: \(\int_{-\infty}^{\infty} \Box\; d p\)

Momentum operator: \(p \cdot \Box\)

Kinetic energy operator: \(\frac{p^{2}}{2} \cdot \Box\)

Position operator: \(i \cdot \frac{d}{d p} \Box\)

Potential energy operator: \(\frac{-1}{2} \cdot \frac{d^{2}}{d p^{2}} \Box\)

Demonstrate that the wave function is normalized and calculate <x>, <x²>,

, and <p²>. Then use these results to demonstrate that the uncertainty principle is satisfied.

\[
\int_{-\infty}^{\infty}\left(\left|\Phi_{0}(\mathrm{p})\right|\right)^{2} \mathrm{d} \mathrm{p}=1 \quad \mathrm{p}_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \mathrm{p} \cdot \Phi_{0}(\mathrm{p})^{2} \mathrm{d} \mathrm{p} \rightarrow 0 \quad \mathrm{p} 2_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \mathrm{p}^{2} \cdot\left(\left|\Phi_{0}(\mathrm{p})\right|\right)^{2} \mathrm{dp} \rightarrow \frac{1}{2}
\nonumber \]

\[
\mathrm{x}_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \Phi_{0}(\mathrm{p}) \cdot \mathrm{i} \cdot \frac{\mathrm{d}}{\mathrm{d} \mathrm{p}} \Phi_{0}(\mathrm{p}) \mathrm{dp} \rightarrow 0 \qquad \mathrm{x}_{2 \mathrm{ave}} :=\int_{-\infty}^{\infty} \overline{\Phi_{0}(\mathrm{p})} \cdot -\frac{\mathrm{d}^{2}}{\mathrm{d} \mathrm{p}^{2}} \Phi_{0}(\mathrm{p}) \mathrm{dp} \rightarrow \frac{1}{2}
\nonumber \]

In momentum space, it is the momentum operator that appears to behave classically, and the position operator that manifests quantum weirdness.

These momentum space calculations are in compliance with the uncertainty principle.

\[
\Delta x :=\sqrt{x 2_{\text { ave }}-x_{\text { ave }}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \qquad \Delta \mathrm{p} :=\sqrt{\mathrm{p} 2_{\mathrm{ave}}-\mathrm{p}_{\mathrm{ave}}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \qquad \Delta \mathrm{x} \cdot \Delta \mathrm{p} \rightarrow \frac{1}{2}
\nonumber \]

Demonstrate that \(\Phi_{0}(p)\) is an eigenfunction of the energy operator and use the expectation values from above to calculate the expectation value for energy.

\[
\frac{\mathrm{p}^{2}}{2} \cdot \Phi_{0}(\mathrm{p})-\frac{1}{2} \cdot \frac{\mathrm{d}^{2}}{\mathrm{d} \mathrm{p}^{2}} \Phi_{0}(\mathrm{p})=\mathrm{E} \cdot \Phi_{0}(\mathrm{p}) \text { solve, } \mathrm{E} \rightarrow \frac{1}{2} \qquad \frac{\mathrm{p} 2_{\mathrm{ave}}}{2}+\frac{\mathrm{x} 2_{\mathrm{ave}}}{2} \rightarrow \frac{1}{2}
\nonumber \]

In summary, we see that coordinate and momentum space calculations give the same results. However, the coordinate wave function does not tell us anything about the distribution of momentum states, only the average value. Likewise, the momentum wave function does not provide detail on the spatial distribution of the particle it represents, only the average position.

Phase Space Calculations

Phase‐space calculations require a phase‐space distribution, such as the Wigner function. Because this approach to quantum mechanics is not as familiar as the Schrödinger formulation, several important equations will be deconstructed using Dirac notation. Expressed in Dirac notation, the Wigner function resembles a classical trajectory.

\[
W(x, p)=\int_{-\infty}^{\infty}\left\langle\Psi | x+\frac{s}{2}\right\rangle\left\langle x+\frac{s}{2} | p\right\rangle\left\langle p | x-\frac{s}{2}\right\rangle\left\langle x-\frac{s}{2} | \Psi\right\rangle d s
\nonumber \]

The four Dirac brackets are read from right to left as follows: (1) is the amplitude that a particle in the state \(\Psi\) has position (x ‐ s/2); (2) is the amplitude that a particle with position (x ‐ s/2) has momentum p; (3) is the amplitude that a particle with momentum p has position (x + s/2); (4) is the amplitude that a particle with position (x + s/2) is (still) in the state \(\Psi\). The Wigner function is the integral of the product of these probability amplitudes over all values of s.

We get the traditional form of the Wigner distribution function by recognizing that the middle brackets, which function as a propagator between the initial and final positional states, can be combined as follows,

\[
\left\langle x+\frac{s}{2} | p\right\rangle\left\langle p | x-\frac{s}{2}\right\rangle=\frac{1}{\sqrt{2 \pi}} \mathrm{e}^{i p\left(x+\frac{\Delta}{2}\right)} \frac{1}{\sqrt{2 \pi}} \mathrm{e}^{-i p\left(x-\frac{\delta}{2}\right)}=\frac{1}{2 \pi} \mathrm{e}^{i p s}
\nonumber \]

Now we can generate the Wigner function for the v = 0 harmonic oscillator state using the coordinate eigenfunction.

\[
\mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) :=\frac{1}{2 \pi} \cdot \int_{-\infty}^{\infty} \Psi_{0}\left(\mathrm{x}+\frac{\mathrm{s}}{2}\right) \cdot \exp (\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{p}) \cdot \Psi_{0}\left(\mathrm{x}-\frac{\mathrm{s}}{2}\right) \text { ds simplify } \rightarrow \frac{1}{\pi} \cdot \mathrm{e}^{\left(-\mathrm{x}^{2}\right)-\mathrm{p}^{2}}
\nonumber \]

Now we can generate the Wigner function for the v = 0 harmonic oscillator state using the coordinate eigenfunction.

\[
\mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) :=\frac{1}{2 \pi} \cdot \int_{-\infty}^{\infty} \Psi_{0}\left(\mathrm{x}+\frac{\mathrm{s}}{2}\right) \cdot \exp (\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{p}) \cdot \Psi_{0}\left(\mathrm{x}-\frac{\mathrm{s}}{2}\right) \text { ds simplify } \rightarrow \frac{1}{\pi} \cdot \mathrm{e}^{\left(-\mathrm{x}^{2}\right)-\mathrm{p}^{2}}
\nonumber \]

In coordinate space, momentum is represented by a differential operator, the first derivative with respect to position. In momentum space, position is represented by the first derivative with respect to momentum. Part of the appeal of the phase‐space approach to quantum mechanics is that both position and momentum are represented by multiplicative operators (1). Thus phase‐space quantum mechanics, at first glance, appears to more closely resemble classical mechanics than the traditional Schrödinger formulation with its differential operators.

Phase space integral: \(\int_{-\infty}^{\infty} \Box\; dx\; d p\)

Position operator: \(x \cdot \Box\)

Potential energy operator: \(\frac{x^{2}}{2} \cdot \Box\)

Momentum operator: \(p \cdot \Box\)

Kinetic energy operator: \(\frac{p^{2}}{2} \cdot \Box\)

Demonstrate that the Wigner function is normalized over phase space and calculate <x>, <x²>,

, and <p²>. Then use these results to demonstrate that the uncertainty principle is satisfied. In Appendix B Dirac notation is used to deconstruct (unpack) the first two phase‐space calculations below and show that they are equivalent to the traditional quantum mechanical calculations carried out previously.

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \mathrm{d} \mathrm{x} \text { dp simplify } \rightarrow 1
\nonumber \]

$$ x_{\text { ave }} :=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} W_{0}(x, p) \cdot x \text { dx dp simplify } \rightarrow 0 $$	$$ \mathrm{x} 2_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \cdot \mathrm{x}^{2} \mathrm{dx} \text { dp simplify } \rightarrow \frac{1}{2} $$
$$ \mathrm{p}_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \cdot \mathrm{p} \mathrm{dx} \text { dp simplify } \rightarrow 0 $$	$$ \mathrm{p} 2_{\mathrm{ave}} :=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \cdot \mathrm{p}^{2} \mathrm{dx} \text { dp simplify } \rightarrow \frac{1}{2} $$

\[
\Delta x :=\sqrt{x 2_{\text { ave }}-x_{\text { ave }}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \quad \Delta p :=\sqrt{p 2_{\text { ave }}-p_{\text { ave }}^{2}} \rightarrow \frac{1}{2} \cdot 2^{\frac{1}{2}} \quad \Delta x \cdot \Delta p \rightarrow \frac{1}{2}
\nonumber \]

Calculate the expectation value for the total energy

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \cdot\left(\frac{\mathrm{p}^{2}}{2}+\frac{\mathrm{x}^{2}}{2}\right) \mathrm{dx} \text { dp simplify } \rightarrow \frac{1}{2}
\nonumber \]

In summary, the phase‐space calculations based on the Wigner function give the same results as the calculations carried out in coordinate and momentum space.

Next, we demonstrate that integrating the Wigner function over momentum space yields the coordinate distribution function. (See the Appendix C for a deconstruction of this integral using Dirac notation.)

\[
\int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \mathrm{dp} \text { simplify } \rightarrow \frac{\mathrm{e}^{-\mathrm{x}^{2}}}{\pi^{\frac{1}{2}}} \qquad \Psi_{0}(x)^{2} \text { simplify } \rightarrow \frac{e^{-x^{2}}}{\pi^{\frac{1}{2}}}
\nonumber \]

Likewise, integrating the Wigner function over coordinate space yields the momentum distribution function. (See the Appendix C for a deconstruction of this integral using Dirac notation.)

\[
\int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \mathrm{dx} \text { simplify } \rightarrow \frac{\mathrm{e}^{-\mathrm{p}^{2}}}{\pi^{\frac{1}{2}}} \qquad \Phi_{0}(\mathrm{p})^{2} \text { simplify } \rightarrow \frac{\mathrm{e}^{-\mathrm{p}^{2}}}{\pi^{\frac{1}{2}}}
\nonumber \]

Just as we have previous graphed the coordinate and momentum distribution functions, we now display the Wigner distribution function.

\[
\mathrm{N} :=60 \qquad \mathrm{i} :=0 \ldots \mathrm{N} \qquad \mathrm{x}_{\mathrm{i}} :=-3+\frac{6 \cdot \mathrm{i}}{\mathrm{N}} \qquad \mathrm{j} :=0 \ldots \mathrm{N} \mathrm{p}_{\mathrm{j}} :=-5+\frac{10 \cdot \mathrm{j}}{\mathrm{N}} \qquad \text {Wigner}_{\text{i, j}} :=\mathrm{W}_{0}\left(\mathrm{x}_{\mathrm{i}}, \mathrm{p}_{\mathrm{j}}\right)
\nonumber \]

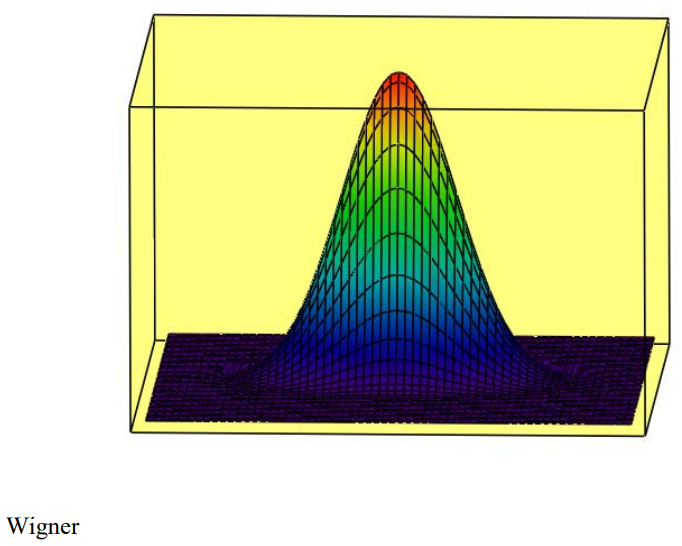
In these phase‐space calculations W(x,p) appears to behave like a classical probability function. By eliminating the need for differential operators, it seems to have removed some of the weirdness from quantum mechanics. However, we will now see that the Wigner function, phase‐space approach only temporarily hides the weirdness. This shouldnʹt come as a surprise because, after all, the Wigner function was generated using a Schrödinger wave function.

To see how the weirdness is hidden we generate the Wigner function for the v = 1 harmonic oscillator state.

\[
\mathrm{W}_{1}(\mathrm{x}, \mathrm{p}) :=\frac{1}{2 \pi} \int_{-\infty}^{\infty} \Psi_{1}\left(\mathrm{x}+\frac{\mathrm{s}}{2}\right) \cdot \exp (\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{p}) \cdot \Psi_{1}\left(\mathrm{x}-\frac{\mathrm{s}}{2}\right) \mathrm{ds} \; \text{simplify} \\ \rightarrow \mathrm{e}^{\left(-\mathrm{x}^{2}\right)-\mathrm{p}^{2} }\cdot \frac{2 \cdot \mathrm{x}^{2}+2 \cdot \mathrm{p}^{2}-1}{\pi}
\nonumber \]

Next, it is demonstrate that the Wigner functions for the ground and excited harmonic oscillator states are orthogonal over phase space.

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}_{0}(\mathrm{x}, \mathrm{p}) \cdot \mathrm{W}_{1}(\mathrm{x}, \mathrm{p}) \mathrm{d} \mathrm{x} \mathrm{dp} \rightarrow 0
\nonumber \]

This result indicates that W₁(x,p) must be negative over some part of phase space, because the graph of W₀(x,p) shows that it is positive for all values of position and momentum. To explore further we display the Wigner distribution for the v = 1 harmonic oscillator state.

This graphic shows that the Wigner function is indeed negative for certain regions of phase space. This makes it impossible to interpret it as a probability distribution function. For this reason the Wigner function is frequently referred to as a quasiprobability distribution.

The Variation Method

As a final example of the equivalence of the three approaches to quantum mechanics presented, we look at a variation method calculation on a potential function that resembles (somewhat) the harmonic oscillator.

\[
\mathrm{V}(\mathrm{x}) :=|\mathrm{x}|
\nonumber \]

A Gaussian trial wave function is chosen for the coordinate space calculation.

\[
\Psi(x, \beta) :=\left(\frac{2 \cdot \beta}{\pi}\right)^{\frac{1}{4}} \cdot \exp \left(-\beta \cdot x^{2}\right)
\nonumber \]

The variational energy integral is evaluated.

\[
\mathrm{E}(\beta) :=\int_{-\infty}^{\infty} \Psi(\mathrm{x}, \beta) \cdot -\frac{1}{2} \cdot \frac{\mathrm{d}^{2}}{\mathrm{dx}^{2}} \Psi(\mathrm{x}, \beta) \mathrm{d} \mathrm{x} \ldots \Bigg|^{\text { assume, } \beta>0}_{\text { simplify }} \\ \rightarrow \frac{1}{2} \cdot \frac{\beta^{2} \cdot \pi+2^{\frac{1}{2}} \cdot(\beta \cdot \pi)^{\frac{1}{2}}}{\beta \cdot \pi} +\int_{-\infty}^{\infty} \mathrm{V}(\mathrm{x}) \cdot \Psi(\mathrm{x}, \beta)^{2} \mathrm{d} \mathrm{x}
\nonumber \]

The momentum wave function is obtained from the coordinate wave function by Fourier transform.

\[
\Phi(\mathrm{p}, \beta) :=\frac{1}{\sqrt{2 \cdot \pi}} \cdot \int_{-\infty}^{\infty} \exp (-\mathrm{i} \cdot \mathrm{p} \cdot \mathrm{x}) \cdot \Psi(\mathrm{x}, \beta) \mathrm{d} \mathrm{x} \Bigg|^{\text { assume, } \beta>0}_{\text { simplify}} \rightarrow \frac{1}{2} \cdot \frac{\pi^{\frac{1}{2}} \cdot \beta^{\frac{3}{2}}+2^{\frac{1}{2}}}{\pi^{\frac{1}{2}} \cdot \beta^{\frac{1}{2}}} +\int_{-\infty}^{\infty} \overline{\Phi(p, \beta)} \cdot\left|i \frac{d}{d p} \Phi(p, \beta)\right| d p
\nonumber \]

The Wigner function is calculated using the coordinate wave function (the momentum wave function will yield the same result).

\[
\mathrm{W}(\mathrm{x}, \mathrm{p}, \beta) :=\frac{1}{2 \pi} \int_{-\infty}^{\infty} \Psi\left(\mathrm{x}+\frac{\mathrm{s}}{2}, \beta\right) \cdot \exp (\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{p}) \cdot \Psi\left(\mathrm{x}-\frac{\mathrm{s}}{2}, \beta\right) \mathrm{ds}\Bigg|^{\text { simplify }}_{\text { assume, } \beta>0}\rightarrow \frac{1}{\pi} \cdot e^{\frac{-1}{2} \cdot \frac{4 \cdot \beta^{2} \cdot x^{2}+p^{2}}{\beta}}
\nonumber \]

The variational energy integral in phase space is evaluated.

\[
\mathrm{E}(\beta) :=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} \mathrm{W}(\mathrm{x}, \mathrm{p}, \beta) \cdot\left(\frac{\mathrm{p}^{2}}{2}+\mathrm{V}(\mathrm{x})\right) \mathrm{d} \mathrm{x} \mathrm{dp}\Bigg|^{\text { assume, } \beta>0}_{\text { simplify }} \rightarrow \frac{1}{2} \cdot \frac{\pi^{\frac{1}{2}} \cdot \beta^{\frac{3}{2}}+2^{\frac{1}{2}}}{\pi^{\frac{1}{2}} \cdot \beta^{\frac{1}{2}}}
\nonumber \]

As is to be expected, the three methods yield the same expression for the variational energy. Minimization of the energy with respect to the variational parameter, \(\beta\), yields \(\beta\) = 0.542 and E(\(\beta\)) = 0.813. This is in good agreement with the result obtained by numerical integration of Schrödingerʹs equation for this potential, E = 0.809.

Appendix A

In coordinate space, the position eigenvalue equation can be written in two equivalent forms. In both cases the operator, in its home space, extracts the eigenvalue and returns the eigenfunction. It thus appears to be a multiplicative operator.

\[
\hat{x} | x \rangle=x | x \rangle \quad\langle x|\hat{x}=x\langle x|
\nonumber \]

Using the form on the right we demonstrate that the position operator in the coordinate representation operates multiplicatively on an arbitrary state function.

\[
\langle x|\hat{x}| \Psi\rangle= x\langle x | \Psi\rangle= x \Psi(x)
\nonumber \]

Making use of the coordinate space completeness relation,

\[
\int | x \rangle\langle x|d x=1
\nonumber \]

we can illuminate the position expectation value in the coordinate representation.

\[
\langle x\rangle=\langle\Psi|\hat{x}| \Psi\rangle=\int\langle\Psi | x\rangle\langle x|\hat{x}| \Psi\rangle d x=\int\langle\Psi | x\rangle x\langle x | \Psi\rangle d x=\int x|\Psi(x)|^{2} d x
\nonumber \]

In momentum space, the momentum eigenvalue equation can also be written in two equivalent forms. As in coordinate space, the home‐space operator extracts the eigenvalue and returns the eigenfunction.

\[
\hat{p} | p \rangle=p | p \rangle \quad\langle p|\hat{p}=p\langle p|
\nonumber \]

Using the form on the right we demonstrate that the momentum operator in momentum space operates multiplicatively on an arbitrary state function.

\[
\langle p|\hat{p}| \Phi\rangle= p\langle p | \Phi\rangle= p \Phi(p)
\nonumber \]

To proceed to a justification of the differential form of the momentum operator in coordinate space and the differential form of the position operator in momentum space requires the following Dirac bracket between position and momentum.

\[
\langle x | p\rangle=\langle p | x\rangle^{*}=\exp \left(\frac{i p x}{\hbar}\right)
\nonumber \]

This relation is obtained by substitution of the deBroglie wave equation into the Euler equation for a plane wave (2).

\[
\lambda=\frac{h}{m v}=\frac{h}{p} \qquad\langle x | \lambda\rangle=\exp \left(i 2 \pi \frac{x}{\lambda}\right)
\nonumber \]

The Dirac brackets, <x|p> and <p|x> are ubiquitous in quantum mechanics and are, essentially, a dictionary for translating from momentum language to position language and vice versa. In other words, they are momentum‐position Fourier transforms.

The momentum operator in coordinate space is obtained by projecting the momentum eigenvalue expression onto coordinate space.

\[
\langle x|\hat{p}| p\rangle= p\langle x | p\rangle= p \exp \left(\frac{i p x}{\hbar}\right)=\frac{\hbar}{i} \frac{d}{d x}\langle x | p\rangle
\nonumber \]

Comparing the first and the last terms, gives the momentum operator in position space.

\[
\langle x|\hat{p}=\frac{\hbar}{i} \frac{d}{d x}\langle x|
\nonumber \]

Using this form we demonstrate the momentum operator in coordinate space operating on an arbitrary state function.

\[
\langle x|\hat{p}| \Psi\rangle=\frac{\hbar}{i} \frac{d}{d x}\langle x | \Psi\rangle=\frac{\hbar}{i} \frac{d}{d x} \Psi(x)
\nonumber \]

Using the coordinate completeness relation, we derive the mathematical structure of the calculation of the momentum expectation value in the coordinate representation.

\[
\langle p\rangle=\langle\Psi|\hat{p}| \Psi\rangle=\int\langle\Psi | x\rangle\langle x|\hat{p}| \Psi\rangle d x=\int\langle\Psi | x\rangle \frac{\hbar}{i} \frac{d\langle x | \Psi\rangle}{d x} d x=\int \Psi^{*}(x) \frac{\hbar}{i} \frac{d \Psi(x)}{d x} d x
\nonumber \]

The position operator in momentum space is obtained by projecting the position eigenvalue expression onto momentum space.

\[
\langle p|\hat{x}| x\rangle= x\langle p | x\rangle= x \exp \left(\frac{-i p x}{\hbar}\right)=-\frac{\hbar}{i} \frac{d}{d p}\langle p | x\rangle
\nonumber \]

Comparing the first and the last terms, gives the position operator in momentum space.

\[
\langle p|\hat{x}=-\frac{\hbar}{i} \frac{d}{d p}\langle p|
\nonumber \]

Using this form we demonstrate the position operator in momentum space operating on an arbitrary state function.

\[
\langle p|\hat{x}| \Phi\rangle=-\frac{\hbar}{i} \frac{d}{d p}\langle p | \Phi\rangle=-\frac{\hbar}{i} \frac{d}{d p} \Phi(p)
\nonumber \]

Illustrating the origin of the quantum mechanical calculations for the expectation values for momentum and position in momentum space are similar to the calculations in coordinate space, except that the completeness relation in momentum space is required.

\[
\int | p \rangle\langle p|d p=1
\nonumber \]

In the text it was noted that in phase space, both position and momentum are multiplicative operators (1). The following table summarizes the operator notation for the three spaces.

\[\begin{pmatrix} \text{Operator} & \text{CoordinateSpace} & \text{MomentumSpace} & \text{PhaseSpace} \\ \text{position} & x & \frac{-1}{\mathrm{i}} \cdot \frac{\mathrm{d}}{\mathrm{d} \mathrm{p}} \Box & x \\ \text{momentum} & \frac{1}{\mathrm{i}} \cdot \frac{\mathrm{d}}{\mathrm{d} \mathrm{x}} \Box & p & p \end{pmatrix} \nonumber \]

Appendix B

In Dirac notation the phase‐space normalization condition is,

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} W(x, p) d x d p=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty} \int_{-\infty}^{\infty}\langle\Psi | x+\frac{s}{2}\rangle\left\langle x+\frac{s}{2} | p\right\rangle\langle p | x-\frac{s}{2}\rangle\left\langle x-\frac{s}{2} | \Psi\right\rangle d s d x d p
\nonumber \]

Utilizing the momentum completeness relation,

\[
\int_{-\infty}^{\infty} | p \rangle\langle p|d p=1
\nonumber \]

yields,

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty}\left\langle\Psi | x+\frac{s}{2}\right\rangle\left\langle x+\frac{s}{2} | x-\frac{s}{2}\right\rangle\left\langle x-\frac{s}{2} | \Psi\right\rangle d s d x
\nonumber \]

However, the integral over s is zero unless s = 0. Thus, for a normalized coordinate wave function we arrive at,

\[
\int_{-\infty}^{\infty}\langle\Psi | x\rangle\langle x | \Psi\rangle d x=1
\nonumber \]

Using similar arguments it is easy to show that,

\[
\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} W(x, p) x d x d p=\int_{-\infty}^{\infty}\langle\Psi | x\rangle x\langle x | \Psi\rangle d x=\langle x\rangle
\nonumber \]

Appendix C

To show that integrating the Wigner function over momentum space yields the coordinate distribution function, we proceed as shown below.

\[
\int_{-\infty}^{\infty} W(x, p) d p=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty}\left\langle\Psi | x+\frac{s}{2}\right\rangle\left\langle x+\frac{s}{2} | p\right\rangle\left\langle p | x-\frac{s}{2}\right\rangle\left\langle x-\frac{s}{2} | \Psi\right\rangle d s d p
\nonumber \]

Using the momentum completeness relation (see above) on the right side gives,

\[
\int_{-\infty}^{\infty} W(x, p) d p=\int_{-\infty}^{\infty}\left\langle\Psi | x+\frac{s}{2}\right\rangle\left\langle x+\frac{s}{2} | x-\frac{s}{2}\right\rangle\left\langle x-\frac{s}{2} | \Psi\right\rangle d s
\nonumber \]

However, the right side is zero unless s = 0, yielding

\[
\int_{-\infty}^{\infty} W(x, p) d p=\langle\Psi | x\rangle\langle x | \Psi\rangle=\Psi^{*}(x) \Psi(x)
\nonumber \]

To facilitate the demonstration that integrating the Wigner function over coordinate space yields the momentum distribution function, we first show that the Wigner function can also be generated using the momentum wave function.

\[
\mathrm{Wp}_{0}(\mathrm{x}, \mathrm{p}) :=\frac{1}{2 \cdot \pi} \cdot \int_{-\infty}^{\infty}\Phi_{0}\left(\mathrm{p}+\frac{\mathrm{s}}{2}\right) \cdot \exp (-\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{x}) \cdot \Phi_{0}\left(\mathrm{p}-\frac{\mathrm{s}}{2}\right) \mathrm{ds}\; \text{simplify} \rightarrow \frac{1}{\pi} \cdot \mathrm{e}^{\left(-\mathrm{x}^{2}\right)-\mathrm{p}^{2}}
\nonumber \]

\[
\int_{-\infty}^{\infty} W(x, p) d x=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty}\langle\Phi | p+\frac{s}{2}\rangle\left\langle p+\frac{s}{2} | x\right\rangle\langle x | p-\frac{s}{2}\rangle\left\langle p-\frac{s}{2} | \Phi\right\rangle d s d x
\nonumber \]

Employing the coordinate completeness relation

\[
\int_{-\infty}^{\infty} | x \rangle\langle x|d x=1
\nonumber \]

yields

\[
\int_{-\infty}^{\infty} W(x, p) d x=\int_{-\infty}^{\infty}\left\langle\Phi | p+\frac{s}{2}\right\rangle\left\langle p+\frac{s}{2} | p-\frac{c}{2}\right\rangle\left\langle p-\frac{s}{2} | \Phi\right\rangle d s
\nonumber \]

However, the right side is zero unless s = 0, yielding

\[
\int_{-\infty}^{\infty} W(x, p) d x=\langle\Phi | p\rangle\langle p | \Phi\rangle=\Phi^{*}(p) \Phi(p)
\nonumber \]

References

1. Case, W. B. Wigner functions and Weyl transforms for pedestrians. Am. J. Phys. 2008, 76 937‐946.
2. Rioux, F.; Johnson, B. J. Using Optical Transforms to Teach Quantum Mechanics. Chem. Educator. 2004, 9, 12‐16.