2.15: Quantum Mechanical Calculations for the Hydrogen Atom

Full kinetic energy operator in spherical coordinates:

Kinetic energy operator for s states:

\[ - \frac{1}{2r} \frac{d^2}{dr^2} \blacksquare \nonumber \]

Kinetic energy operator for p states:

\[ \begin{matrix} - \frac{1}{2r} \frac{d^2}{dr^2} r \blacksquare ... \\ + \frac{-1}{2r^2 \sin ( \theta )} \left[ \frac{d}{d \theta} \left( \sin ( \theta ) \frac{d}{d \theta} \blacksquare \right) \right] ... \\ + \frac{-1}{2r^2 \sin ( \theta)^2} \frac{d^2}{d \phi^2} \blacksquare \end{matrix} \nonumber \]

Position operator: r

Potential energy operator: \( - \frac{1}{r}\)

Triple integral with volume element: \( \int_0^{ \infty} \int_0^{ \pi} \int_0^{ 2 \pi} \blacksquare r^2 \sin ( \theta ) d \phi d \theta dr\)

Orbitals:

\[ \begin{matrix} \Psi_{1s} (r) = \frac{1}{ \sqrt{ \pi}} \text{exp(-r)} & \Psi_{2s} (r) = \frac{1}{ \sqrt{32 \pi}} (2 - r) \text{exp} \left( - \frac{r}{2} \right) \\ \Psi_{2pz} (r,~ \theta) = \frac{1}{ \sqrt{32 \pi}} \text{r exp} \left( - \frac{r}{2} \right) \cos \theta & \Psi_{2py} (r,~ \theta,~ \phi ) = \frac{1}{ \sqrt{32 \pi}} \text{r exp} \left( - \frac{r}{2} \right) \sin ( \theta ) \sin ( \phi ) \end{matrix} \nonumber \]

Plot the wave functions on the same graph:

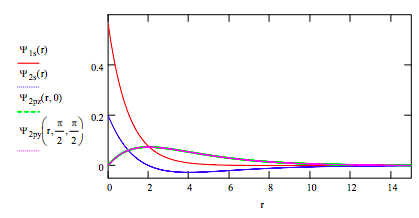
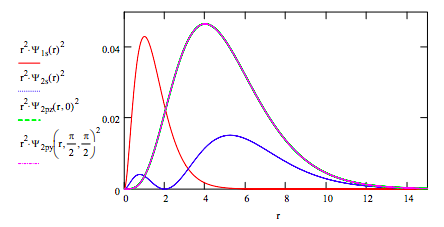
Plot the radial distribution functions for each orbital on the same graph:

Demonstrate that the 1s orbital is normalized:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s} (r)^2 r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 1 \nonumber \]

Demonstrate that the 1s orbital is normalized:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2s} (r)^2 r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 1 \nonumber \]

Demonstrate that the 2pz orbital is normalized:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2pz} (r,~ \theta)^2 r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 1 \nonumber \]

Demonstrate that the 1s and the 2pz orbitals are orthogonal:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s}(r) \Psi_{2pz} (r,~ \theta)^2 r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 0 \nonumber \]

Demonstrate the 1s and 2s orbitals are orthogonal:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s}(r) \Psi_{2s} (r)^2 r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 0 \nonumber \]

Demonstrate that the 2p_y and the 2p_z orbitals are orthogonal:

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2py} (r,~ \theta,~ \phi ) \Psi_{2pz} (r,~ \theta ) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 0 \nonumber \]

Determine the most probable value for r using the Trace function and calculus:

\[ \frac{d}{dr} r^2 \Psi_{1s}(r)^2 = 0 \text{ solve, r} \rightarrow \begin{pmatrix} 0 \\ 1 \end{pmatrix} \nonumber \]

Calculate the probability that an electron in the 1s orbital will be found within one Bohr radius of the nucleus.

\[ \int_0^1 \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s} (r)^2 r^2 \sin ( \theta ) d \phi d \theta dr \text{ float, 3} \rightarrow .325 \nonumber \]

Find the distance from the nucleus for which the probability of finding a 1s electron is 0.75.

\[ \begin{matrix} a = 2 & \text{Given} & \int_0^a \Psi_{1s} (r)^2 4 \pi r^2 dr = /75 & \text{Find(a) = 1.96} \end{matrix} \nonumber \]

Calculate <T>, <V>, and <r> for the 1s orbital. Is the virial theorem obeyed? Explain.

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s} (r) - \frac{1}{2r} \frac{d^2}{dr^2} r \left( \Psi_{1s} (r) \right) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{1}{2} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s} (r) - \frac{1}{r} \Psi_{1s} (r) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow -1 \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{1s} (r) r \Psi_{1s} (r) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{3}{2} \nonumber \]

Calculate <T>, <V>, and <r> for the 2s orbital. Is the virial theorem obeyed? Explain.

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2s} (r) - \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{2s} (r) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{1}{8} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2s} (r) - \frac{1}{r} \Psi_{2s} (r) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow - \frac{1}{4} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2s} (r) r \Psi_{2s} (r) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 6 \nonumber \]

Calculate <T>, <V>, and <r> for the 2p_y orbital. Is the virial theorem obeyed? Explain.

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2py} (r, ~ \theta,~ \phi ) \begin{bmatrix} - \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{2py} (r,~ \theta,~ \phi ) ... \\ + \frac{-1}{2r^2 \sin ( \theta)} \left[ \frac{d}{d \theta} \left( \sin ( \theta ) \frac{d}{d \theta} \Psi_{2py} (r,~ \theta,~ \phi ) \right) \right] ... \\ + \frac{-1}{2r^2 \sin ( \theta )^2} \frac{d^2}{d \phi^2} \Phi_{2py} (r,~ \theta,~ \phi) \end{bmatrix} r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{1}{8} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2py} (r,~ \theta,~ \phi) - \frac{1}{r} \Psi_{2py} (r,~ \theta,~ \phi) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{-1}{4} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2py} (r,~ \theta,~ \phi) r \Psi_{2py} (r,~ \theta,~ \phi) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 5 \nonumber \]

Calculate <T>, <V>, and <r> for the 2pz orbital. Is the virial theorem obeyed? Explain.

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2pz} (r, ~ \theta ) \begin{bmatrix} - \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{2pz} (r,~ \theta ) ... \\ + \frac{-1}{2r^2 \sin ( \theta)} \left[ \frac{d}{d \theta} \left( \sin ( \theta ) \frac{d}{d \theta} \Psi_{2pz} (r,~ \theta ) \right) \right] ... \\ + \frac{-1}{2r^2 \sin ( \theta )^2} \frac{d^2}{d \phi^2} \Phi_{2pz} (r,~ \theta ) \end{bmatrix} r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{1}{8} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2pz} (r,~ \theta ) - \frac{1}{r} \Psi_{2pz} (r,~ \theta ) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow \frac{-1}{4} \nonumber \]

\[ \int_0^{ \infty} \int_0^{ \pi} \int_0^{2 \pi} \Psi_{2pz} (r,~ \theta ) r \Psi_{2pz} (r,~ \theta ) r^2 \sin ( \theta ) d \phi d \theta dr \rightarrow 5 \nonumber \]

Summarize your results in the following table:

\[ \begin{pmatrix} \Psi & \text{T} & \text{V} & \text{E} & \text{r} \\ \text{1s} & 0.5 & -1 & -0.5 & 1.5 \\ \text{2s} & 0.125 & -0.25 & -0.125 & 6 \\ \text{2pz} & 0.125 & -0.25 & -0.125 & 5 \\ \text{2py} & 0.125 & -0.25 & -0.125 & 5 \end{pmatrix} \nonumber \]

Demonstrate that the 1s orbital is an eigenfunction of the energy operator. What is the eigenvalue?

\[ \begin{matrix} T = - \frac{1}{2r} \frac{d^2}{dr^2} r \blacksquare & V = - \frac{1}{r} & H = T + V & \Psi (r) = \frac{1}{ \sqrt{ \pi}} \text{exp} (-r) \end{matrix} \nonumber \]

\[ \frac{- \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{1s} (r) - \frac{1}{r} \Psi_{2s} (r)}{ \Psi_{1s} (r)} \text{ simplify } \rightarrow \frac{-1}{2} \nonumber \]

Demonstrate that the 2s orbital is an eigenfunction of the energy operator. What is the eigenvalue?

\[ \frac{- \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{2s} (r) - \frac{1}{r} \Psi_{2s} (r)}{ \Psi_{2s} (r)} \text{ simplify } \rightarrow \frac{-1}{8} \nonumber \]

Demonstrate that the 2py orbital is an eigenfunction of the energy operator. What is the eigenvalue?

\[ \frac{ \begin{bmatrix} - \frac{1}{2r} \frac{d^2}{dr^2} r \Psi_{2py} (r,~ \theta,~ \phi ) ... \\ + \frac{-1}{2r^2 \sin ( \theta)} \left[ \frac{d}{d \theta} \left( \sin ( \theta ) \frac{d}{d \theta} \Psi_{2py} (r,~ \theta,~ \phi ) \right) \right] ... \\ + \frac{-1}{2r^2 \sin ( \theta )^2} \frac{d^2}{d \phi^2} \Phi_{2py} (r,~ \theta, ~ \phi ) \end{bmatrix} - \frac{1}{r} \Psi_{2py} (r,~ \theta,~ \phi)}{ \Psi_{2py} (r,~ \theta,~ \phi)} \text{ simplify } \rightarrow \frac{-1}{8} \nonumber \]