6.2.2: Changing Reaction Rates with Temperature
- Page ID
- 1449
The vast majority of reactions depend on thermal activation, so the major factor to consider is the fraction of the molecules that possess enough kinetic energy to react at a given temperature. According to kinetic molecular theory, a population of molecules at a given temperature is distributed over a variety of kinetic energies that is described by the Maxwell-Boltzman distribution law.
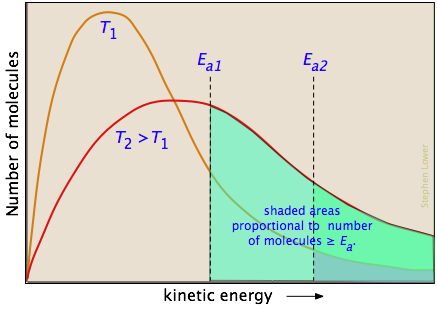
The two distribution plots shown here are for a lower temperature T1 and a higher temperature T2. The area under each curve represents the total number of molecules whose energies fall within particular range. The shaded regions indicate the number of molecules which are sufficiently energetic to meet the requirements dictated by the two values of Ea that are shown.
It is clear from these plots that the fraction of molecules whose kinetic energy exceeds the activation energy increases quite rapidly as the temperature is raised. This the reason that virtually all chemical reactions (and all elementary reactions) proceed more rapidly at higher temperatures.
Temperature is considered a major factor that affects the rate of a chemical reaction. It is considered a source of energy in order to have a chemical reaction occur. Svante Arrhenius, a Swedish chemist, believed that the reactants in a chemical reaction needed to gain a small amount of energy in order to become products. He called this type of energy the activation energy. The amount of energy used in the reaction is known to be greater than the activation energy in the reaction. Arrhenius came up with an equation that demonstrated that rate constants of different kinds of chemical reactions varied with temperature. This equation indicates a rate constant that has a proportional relationship with temperature. For example, as the rate constant increases, the temperature of the chemical reaction generally also increases. The result is given below:
\[\ln \frac{k_2}{k_1} = \frac{E_a}{R}\left(\frac{1}{T_1} - \frac{1}{T_2}\right) \nonumber \]
This equation is known as Arrhenius' equation. T1and T2are temperature variables expressed in Kelvin. T1 can be expressed as the initial or lower temperature of the reaction, while T2 is the final or higher temperature of the reaction. Rate constants, k1and k2, are values at T1 and T2. Ea is the activation energy expressed in (Joules/mole)=(J/mol). R is the gas constant expressed as 8.3145 (Joules/mole × Kelvin)=(J/mol×K)
Some may ask how the temperature actually affects the chemical reaction rate. The answer to this is that this phenomenon is related to the collision theory. Molecules only react if they have sufficient energy for a reaction to take place. When the temperature of a solution increases, the molecular energy levels also increase, causing the reaction to proceedfaster.
The graph of ln K vs. 1/T is linear, allowing the calculation of the activation energy needed for the reaction. An alternate form of the Arrhenius equation is given below:
\[k = A_e^{-\frac{E_a}{RT}} \nonumber \]
Some interesting examples:
- Salt or food coloring is added to cold water, room temperature water, and hot water. When the substance mixes with the hot water, the high temperatures allow it become a homogeneous mixture. This is because due to water molecules moving faster when the temperature is higher and speeding up the dissolution reaction.
- Another form of energy is light. One example of the effect of temperature on chemical reaction rates is the use of lightsticks or glowsticks. The lightstick undergoes a chemical reaction that is called chemiluminescence; but this reaction does not require or produce heat. Its rate, however, is influenced by temperature. If the lightstick is put in a cold environment, the chemical reaction slows down, allowing it to give off light longer. If the lightstick is in a hot environment, the reaction speeds up causing the light to wear out faster. (This example is from "How Things Work—Lightsticks" from chemistry.about.com)
Contributors and Attributions
- Andrea B.
Stephen Lower, Professor Emeritus (Simon Fraser U.) Chem1 Virtual Textbook


