Home
Bookshelves
Physical & Theoretical Chemistry
Quantum Tutorials (Rioux)
1: Quantum Fundamentals
1.73: Time‐dependent Wigner Function for Harmonic Oscillator Transitions

1.73: Time‐dependent Wigner Function for Harmonic Oscillator Transitions

Last updated
Save as PDF

Page ID: 156463

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Initial state:

\[
\mathrm{m} :=0 \qquad \mathrm{E}_{\mathrm{m}} :=\mathrm{m}+\frac{1}{2}
\nonumber \]

Final state:

\[
\mathrm{n} :=1 \qquad \mathrm{E}_{\mathrm{n}} :=\mathrm{n}+\frac{1}{2} \qquad t : = \text{FRAME}
\nonumber \]

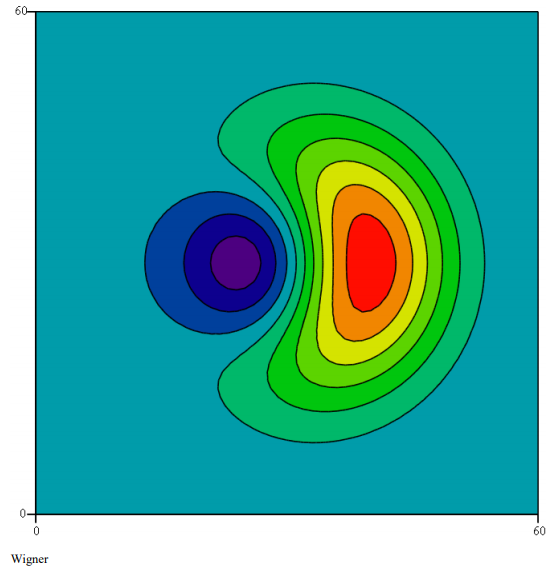
Define Wigner distribution function for a linear superposition of the initial and final harmonic oscillator state.

\[
W(x,p) : = \frac{1}{\pi^{\frac{3}{2}}}\int_{-\infty}^{\infty}\left[\frac{1}{\sqrt{2^{\mathrm{n}} \cdot \mathrm{n} ! \sqrt{\pi}}} \cdot \operatorname{Her}\left(\mathrm{n}, x+\frac{s}{2}\right) \cdot \exp \left[-\frac{\left(\mathrm{x}+\frac{\mathrm{s}}{2}\right)^{2}}{2}\right] \cdot \exp \left(\mathrm{i} \cdot \mathrm{E}_{\mathrm{n}} \cdot \mathrm{t}\right)+\frac{1}{\sqrt{2^{\mathrm{m}} \cdot \mathrm{m} ! \cdot \sqrt{\pi}}} \cdot \operatorname{Her}\left(\mathrm{m}, \mathrm{x}+\frac{\mathrm{s}}{2}\right) \cdot \exp \left[-\frac{\left(\mathrm{x}+\frac{\mathrm{s}}{2}\right)^{2}}{2}\right] \cdot \exp \left(\mathrm{i} \cdot \mathrm{E}_{\mathrm{m}} \cdot \mathrm{t}\right)\right] \\ \cdot \exp (\mathrm{i} \cdot \mathrm{s} \cdot \mathrm{p}) \cdot \left[\frac{1}{\sqrt{2^{\mathrm{n}} \cdot n ! \cdot \sqrt{\pi}}} \cdot \operatorname{Her}\left(\mathrm{n}, \mathrm{x}-\frac{\mathrm{s}}{2}\right) \cdot \exp \left[-\frac{\left(\mathrm{x}-\frac{\mathrm{s}}{2}\right)^{2}}{2}\right] \cdot \exp \left(-\mathrm{i} \cdot \mathrm{E}_{\mathrm{n}} \cdot \mathrm{t}\right)\\+\frac{1}{\sqrt{2^{\mathrm{m}} \cdot \mathrm{m} ! \cdot \sqrt{\pi}}} \cdot \operatorname{Her}\left(\mathrm{m}, \mathrm{x}-\frac{\mathrm{s}}{2}\right)\cdot\exp\left[-\frac{\left(\mathrm{x}-\frac{\mathrm{s}}{2}\right)^{2}}{2}\right]\cdot \exp\left(\mathrm{-i}\cdot\mathrm{E}_{\mathrm{m}}\cdot\mathrm{t}\right)\right]\mathrm{ds}
\nonumber \]

Display Wigner distribution:

\[
\mathrm{N}=60 \qquad \mathrm{i} :=0 \ldots \mathrm{N} \qquad \mathrm{x}_{\mathrm{i}} :=-2.5+\frac{5 \cdot \mathrm{i}}{\mathrm{N}} \\ \mathrm{j} :=0 \ldots \mathrm{N} \qquad \mathrm{P}_{\mathrm{j}} :=-2.5+\frac{5 \mathrm{j}}{\mathrm{N}} \qquad \text{Wigner}_{i,j}: = W(x_{i},p_{j})
\nonumber \]