3.1: Selecting signals by wavevector
- Page ID
- 298957
The question is how to select particular contributions to the signal. It won’t be possible to uniquely select particular diagrams. However, you can use the properties of the incident and detected fields to help with selectivity. Here is a strategy for describing a particular experiment:
- Start with the wavevector and frequency of the signal field of interest.
- (a) Time-domain: Define a time-ordering along the incident wavevectors or (b) Frequency domain: Define the frequencies along the incident wavevectors.
- Sum up diagrams for correlation functions that will scatter into the wave-vector matched direction, keeping only resonant terms (rotating wave approximation). In the frequency domain, use ladder diagrams to determine which correlation functions yield signals that pass through your filter/monochromator.
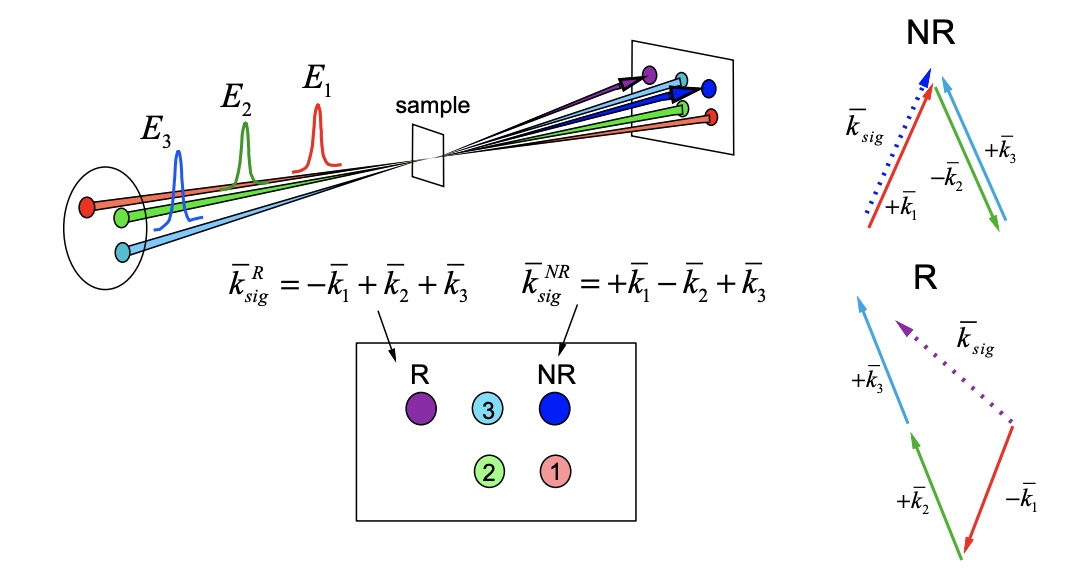
Let’s start by discussing how one can distinguish a rephasing signal from a non-rephasing signal. Consider two degenerate third-order experiments \((\omega_1 = \omega_2 = \omega_3 = \omega_{sig})\) distinguished by the signal wave-vector for a particular time-ordering. We choose a box geometry, where the three incident fields (a,b,c) are crossed in the sample, incident from three corners of the box, as shown. (Colors in these figures are not meant to represent the frequency of the incident fields – which are all the same – but rather is just there to distinguish them for the picture).

Since the frequencies are the same, the length of the wavevector \(|k|=2\pi n/\lambda\) is equal for each field, only its direction varies. Vector addition of the contributing terms from the incident fields indicates that the signal \(\bar k_{sig}\) will be radiated in the direction of the last corner of the box when observed after the sample. (Colors in the figure don’t represent frequency, but serve to distinguish beams):
\[\bar k_{sig} = +\bar k_a − \bar k_b + \bar k_c\]
Comparing the wavevector matching condition for this signal with those predicted by the third-order Feynman diagrams, we see that we can select non-rephasing signals R1 and R4 by setting the time ordering of pulses such that a = 1, b = 2, and c = 3. The rephasing signals R2 and R3 are selected with the time-ordering a = 2, b = 1, and c = 3.
Here the wave-vector matching for the rephasing signal is imperfect. The vector sum of the incident fields \(\bar k_{sig}\) dictates the direction of propagation of the radiated signal (momentum conservation), whereas the magnitude of the signal wavevector \(\bar k_{sig}'\) is dictated by the radiated frequency (energy conservation). The efficiency of radiating the signal field falls of with the wave-vector mismatch
\[\Delta k=\bar k_{sig}-\bar k_{sig}'\]
as
\[|\bar E_{sig}(t)|\propto\bar P(t)\text{sinc} (\Delta kl/2)\]
where \(l\) is the path length (see eq. 2.10).


