8.6: Problems
- Page ID
- 107013
Given a generic equation of state \(P = P(V, T, n)\), explain how you can obtain the derivative
\[\frac{\partial V}{\partial T}_{P,n} \nonumber\]
using the properties of partial derivatives we learned in this chapter.
The thermodynamic equation:
\[\left (\frac{\partial U}{\partial V} \right )_T=T\left (\frac{\partial P}{\partial T} \right )_V-P \nonumber\]
shows how the internal energy of a system varies with the volume at constant temperature.
Prove that
- \(\left (\frac{\partial U}{\partial V} \right )_T=0\) for an ideal gas.
- \(\left (\frac{\partial U}{\partial V} \right )_T=\frac{a}{V^2}\) for one mole of van der Waals gas (Equation \ref{c2v:eq:vdw})
Consider one mole of a van der Waals gas (Equation \ref{c2v:eq:vdw}) and show that
\[\left (\frac{\partial^2 P}{\partial V\partial T}\right )=\left (\frac{\partial^2 P}{\partial T\partial V} \right) \nonumber\]
Consider a van der Waals gas (Equation \ref{c2v:eq:vdw}) and show that
\[\left (\frac{\partial V}{\partial T}\right )_{P,n}=\frac{n R}{\left( P-\frac{n^2a}{V^2}+\frac{2n^3ab}{V^3} \right)} \nonumber\]
Hint: Calculate derivatives that are easier to obtain and use the properties of partial derivatives to get the one the problem asks for. Do not use the answer in your derivation; obtain the derivative assuming you don’t know the answer and simplify your expression until it looks like the equation above.
From the definitions of expansion coefficient (\(\alpha\)) and isothermal compressibility (\(\kappa\)):
\[\alpha=\frac{1}{V}\left(\frac{\partial V}{\partial T}\right)_{P,n} \nonumber\]
and
\[\kappa=-\frac{1}{V}\left(\frac{\partial V}{\partial P}\right)_{T,n} \nonumber\]
prove that
\[\left(\frac{\partial P}{\partial T}\right)_{V,n}=\frac{\alpha}{\kappa} \nonumber\]
independently of the equation of state used.
Note: A common mistake in this problem is to assume a particular equation of state. Use the cycle rule to find the required relationship independently of any particular equation of state.
Derive an equation similar to Equation \ref{c2v:eq:calculus2v_chain1}, but that relates
\[\left ( \frac{\partial f}{\partial y} \right )_x \nonumber\]
with
\[\left ( \frac{\partial f}{\partial r} \right )_\theta \nonumber\]
and
\[\left ( \frac{\partial f}{\partial \theta} \right )_r \nonumber\]
(Extra-credit level)
The expression:
\[\nabla^2=\frac{\partial^2}{\partial x^2}+\frac{\partial^2}{\partial y^2} \nonumber\]
is known as the Laplacian operator in two dimensions.
When applied to a function \(f(x,y)\), we get:
\[\nabla^2f(x,y)=\frac{\partial^2f}{\partial x^2}+\frac{\partial^2f}{\partial y^2} \nonumber\]
Express \(\nabla^2\) in polar coordinates (2D) assuming the special case where \(r=a\) is a constant.
Calculate \(\int_{0}^{1}\int_{1}^{2}\int_{0}^{2}{\left( x^2+yz \right)\, dx\, dy\, dz}.\) Try three different orders of integration an verify you always get the same result.
Calculate \(\int_{0}^{2\pi}\int_{0}^{\pi}\int_{0}^{\infty}{e^{-r}r^5\sin{\theta}\, dr\, d\theta\, d\phi}.\) Use only the formula sheet.
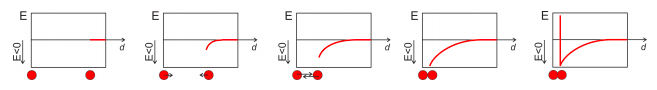
How would Figure \(8.5.2\), reproduced below, look like for an ideal gas? Sketch the potential energy as a function of the distance between the atoms.
From everything we learned in this chapter, and without doing any math, we should be able to calculate the sign (>0, <0, or 0) of the following derivatives:
For an ideal gas:
\[\left(\frac{\partial U}{\partial T}\right)_{V,n} \nonumber\]
\[\left(\frac{\partial U}{\partial V}\right)_{T,n} \nonumber\]
For a van der Vaals gas:
\[\left(\frac{\partial U}{\partial T}\right)_{V,n} \nonumber\]
\[\left(\frac{\partial U}{\partial V}\right)_{T,n} \nonumber\]
Be sure you can write a short sentence explaining your answers.
The critical point is the state at which the liquid and gas phases of a substance first become indistinguishable. A gas above the critical temperature will never condense into a liquid, no matter how much pressure is applied. Mathematically, at the critical point:
\[\left(\frac{\partial P}{\partial V} \right)_{T,n}=0 \nonumber\]
and
\[ \left(\frac{\partial^2 P}{\partial V^2} \right)_{T,n}=0 \nonumber\]
Obtain the critical constants of a van der Waals gas (Equation \ref{c2v:eq:vdw}) in terms of the parameters \(a\) and \(b\).
Hint: obtain the first and second derivatives of \(P\) with respect to \(V\), make them equal to zero, and obtain \(T_c\) and \(V_c\) from these equations. Finally, replace these expressions in Equation \ref{c2v:eq:vdw} to obtain \(P_c\).
As derived in Section 8.3,
\[\label{c2v:eq:calculus2v_chain1} \left(\dfrac{\partial f}{\partial x}\right)_y=\cos{\theta}\left(\dfrac{\partial f}{\partial r}\right)_\theta-\dfrac{\sin{\theta}}{r}\left(\dfrac{\partial f}{\partial \theta}\right)_r\]
As defined in Section 8.5, the Van der Waals is defined as:
\[\label{c2v:eq:vdw} P=\frac{nRT}{V-nb}-a \left(\frac{n}{V}\right)^2\]
- If you are not familiar with this you need to read about it before moving on


