17.5: Natural Radioactivity and Half-Life
- Page ID
- 47587
- Describe what is meant by the term half-life and what factors affect half-life.
- Calculate the amount of radioactive material that will remain after an integral number of half-lives.
- Find the half-life of an isotope given graphical or other data.
- Describe how carbon-14 is used to determine the age of carbon-containing objects.
Rate of Radioactive Decay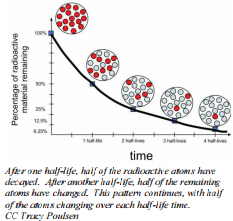
During natural radioactive decay, not all atoms of an element are instantaneously changed to atoms of another element. The decay process takes time and there is value in being able to express the rate at which a process occurs. A useful concept is half-life, which is the time required for half of the starting material to change or decay. Half-lives can be calculated from measurements on the change in mass of a nuclide and the time it takes to occur. The only thing we know is that in the time of that substance's half-life, half of the original nuclei will disintegrate. Although chemical changes are sped up or slowed down by changing factors such as temperature and concentration, these factors have no effect on half-life. Each radioactive isotope will have its own unique half-life that is independent of any of these factors.
The half-lives of many radioactive isotopes have been determined; they have been found to range from extremely long half-lives of 10 billion years, to extremely short half-lives of fractions of a second.
| Element | Mass Number (A) | Half-life | Element | Mass Number (A) | Half Life |
|---|---|---|---|---|---|
| Uranium | 238 | 4.5 Billion years | Californium | 251 | 800 years |
| Neptunium | 240 | 1 hour | Nobelium | 254 | 3 seconds |
| Plutonium | 243 | 5 hours | Carbon | 14 | 5730 years |
| Americium | 245 | 25 minutes | Carbon | 16 | 740 milliseconds |
The quantity of radioactive nuclei at any given time will decrease to half as much in one half-life. For example, if there are \(100 \: \text{g}\) of \(\ce{Cf}\)-251 in a sample at some time, after 800 years, there will be \(50 \: \text{g}\) of \(\ce{Cf}\)-251 remaining; after another 800 years (1600 years total), there will only be \(25 \: \text{g}\) remaining.
Remember, the half-life is the time it takes for half of your sample—no matter how much you have—to remain. Each half-life will follow the same general pattern as \(\ce{Cf}\)-251. The only difference is the length of time it takes for half of a sample to decay.
What is the half-life of an isotope that produces the following graph of decay over time?
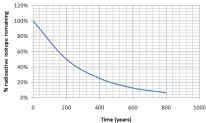
Solution
We know that the half-life is the time it takes for half of a sample to change. How long did it take for half of our isotope to change? It took approximately 200 years for \(100\%\) of our sample to leave only \(50\%\) (half of the original amount) remaining. The half-life is 200 years.
*Notice that after another 200 years (400 years total), \(25\%\) remains (half of \(50\%\)).
Look carefully at the graph in the previous example. All types of radioactive decay make a graph of the same general shape. The only difference is the scale and units of the \(x\)-axis, as the half-life time will be different.
If there are 60 grams of \(\ce{Np}\)-240 present, how much \(\ce{Np}\)-240 will remain after 4 hours? (\(\ce{Np}\)-240 has a half-life of 1 hour.)
Solution
We create a table based on \(\ce{Np}\)-240's half-life of 1 hour.

After 4 hours, only \(3.75 \: \text{g}\) of our original \(60 \: \text{g}\) sample would remain of the radioactive isotope \(\ce{Np}\)-240.
A sample of \(\ce{Ac}\)-225 originally contained 80 grams, and after 50 days only 2.55 grams of the original \(\ce{Ac}\)-225 remain. What is the half life of \(\ce{Ac}\)-225?
Solution
We will tackle this problem similarly to the last problem. The difference is that we are looking for the half-life time. Let's set up a similar table, though:
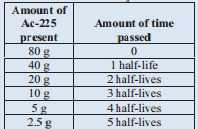
We know that 50 days is the same as 5 half-lives. Therefore, 1 half-life is 10 days. The half-life of \(\ce{Ac}\)-225 is 10 days.
Radioactive Dating
An ingenious application of half-life studies established a new science of determining ages of materials by half-life calculations. For geological dating, the decay of \(\ce{U}\)-238 can be used. The half-life of \(\ce{U}\)-238 is \(4.5 \times 10^9\) years. The end product of the decay of \(\ce{U}\)-238 is \(\ce{Pb}\)-206. After one half-life, a 1.00 gram sample of uranium will have decayed to 0.50 grams of \(\ce{U}\)-238 and 0.43 grams of \(\ce{Pb}\)-206. By comparing the amount of \(\ce{U}\)-238 to the amount of \(\ce{Pb}\)-206 in a sample of uranium mineral, the age of the mineral can be estimated. Present day estimates for the age of the Earth's crust from this method is at 4 billion years.
Organic material (material made from things that were once living, such as paper and fabric) is radioactively dated using the long-lived nuclide of carbon, carbon-14. This method of determining the age of organic material (or once living materials) was given the name radiocarbon dating. The carbon dioxide consumed by living systems contains a certain concentration of \(\ce{^{14}CO_2}\). When an organism dies, the acquisition of carbon-14 stops, but the decay of the \(\ce{C}\)-14 in the body continues. As time goes by, the ratio of \(\ce{C}\)-14 to \(\ce{C}\)-12 decreases at a rate determined by the half-life of \(\ce{C}\)-14. Using half-life equations, the time since the organism died can be calculated. These procedures have been used to determine the age of organic artifacts and determine, for instance, whether art works are real or fake.
Summary and Vocabulary
The half-life of an isotope is used to describe the rate at which the isotope will decay and give off radiation. Using the half-life, it is possible to predict the amount of radioactive material that will remain after a given amount of time. \(\ce{C}\)-14 dating procedures have been used to determine the age of organic artifacts. Its half-life is approximately 5700 years.
- Background radiation - Radiation that comes from environmental sources including the earth's crust, the atmosphere, cosmic rays, and radioisotopes. These natural sources of radiation account for the largest amount of radiation received by most people.
- Half-life - The time interval required for a quantity of (radioactive) material to decay to half of its original value.

