2.5: The Basic Units of Measurement
- Page ID
- 47451
- State the different measurement systems used in chemistry.
- Describe how prefixes are used in the metric system and identify how the prefixes milli-, centi-, and kilo- compare to the base unit.
How long is a yard? It depends on whom you ask and when you asked the question. Today we have a standard definition of the yard, which you can see marked on every football field. If you move the ball ten yards, you get a first down and it does not matter whether you are playing in Los Angeles, Dallas, or Green Bay. But at one time that yard was arbitrarily defined as the distance from the tip of the king's nose to the end of his outstretched hand. Of course, the problem there is simple: new king, new distance (and then you have to re-mark all of those football fields).


SI Base Units
All measurements depend on the use of units that are well known and understood. The English system of measurement units (inches, feet, ounces, etc.) are not used in science because of the difficulty in converting from one unit to another. The metric system is used because all metric units are based on multiples of 10, making conversions very simple. The metric system was originally established in France in 1795. The International System of Units is a system of measurement based on the metric system. The acronym SI is commonly used to refer to this system and stands for the French term, Le Système International d'Unités. The SI was adopted by international agreement in 1960 and is composed of seven base units in Table \(\PageIndex{1}\).
| Quantity | SI Base Unit | Symbol |
|---|---|---|
| Length | meter | \(\text{m}\) |
| Mass | kilogram | \(\text{kg}\) |
| Temperature | kelvin | \(\text{K}\) |
| Time | second | \(\text{s}\) |
| Amount of a Substance | mole | \(\text{mol}\) |
| Electric Current | ampere | \(\text{A}\) |
| Luminous Intensity | candela | \(\text{cd}\) |
The first units are frequently encountered in chemistry. All other measurement quantities, such as volume, force, and energy, can be derived from these seven base units.
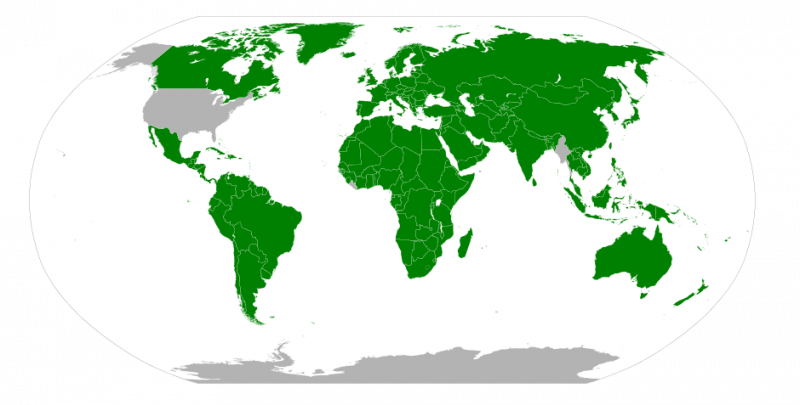
The map below shows the adoption of the SI units in countries around the world. The United States has legally adopted the metric system for measurements, but does not use it in everyday practice. Great Britain and much of Canada use a combination of metric and imperial units.
Prefix Multipliers
Conversions between metric system units are straightforward because the system is based on powers of ten. For example, meters, centimeters, and millimeters are all metric units of length. There are 10 millimeters in 1 centimeter and 100 centimeters in 1 meter. Metric prefixes are used to distinguish between units of different size. These prefixes all derive from either Latin or Greek terms. For example, mega comes from the Greek word \(\mu \varepsilon \gamma \alpha \varsigma\), meaning "great". Table \(\PageIndex{2}\) lists the most common metric prefixes and their relationship to the central unit that has no prefix. Length is used as an example to demonstrate the relative size of each prefixed unit.
| Prefix | Unit Abbreviation | Meaning | Example |
|---|---|---|---|
| giga | \(\text{G}\) | 1,000,000,000 | 1 gigameter \(\left( \text{Gm} \right)=10^9 \: \text{m}\) |
| mega | \(\text{M}\) | 1,000,000 | 1 megameter \(\left( \text{Mm} \right)=10^6 \: \text{m}\) |
| kilo | \(\text{k}\) | 1,000 | 1 kilometer \(\left( \text{km} \right)=1,000 \: \text{m}\) |
| hecto | \(\text{h}\) | 100 | 1 hectometer \(\left( \text{hm} \right)=100 \: \text{m}\) |
| deka | \(\text{da}\) | 10 | 1 dekameter \(\left( \text{dam} \right)=10 \: \text{m}\) |
| 1 | 1 meter \(\left( \text{m} \right)\) | ||
| deci | \(\text{d}\) | 1/10 | 1 decimeter \(\left( \text{dm} \right)=0.1 \: \text{m}\) |
| centi | \(\text{c}\) | 1/100 | 1 centimeter \(\left( \text{cm} \right)=0.01 \: \text{m}\) |
| milli | \(\text{m}\) | 1/1,000 | 1 millimeter \(\left( \text{mm} \right)=0.001 \: \text{m}\) |
| micro | \(\mu\) | 1/1,000,000 | 1 micrometer \(\left( \mu \text{m} \right)=10^{-6} \: \text{m}\) |
| nano | \(\text{n}\) | 1/1,000,000,000 | 1 nanometer \(\left( \text{nm} \right)=10^{-9} \: \text{m}\) |
| pico | \(\text{p}\) | 1/1,000,000,000,000 | 1 picometer \(\left( \text{pm} \right)=10^{-12} \: \text{m}\) |
There are a couple of odd little practices with the use of metric abbreviations. Most abbreviations are lowercase. We use "\(\text{m}\)" for meter and not "\(\text{M}\)". However, when it comes to volume, the base unit "liter" is abbreviated as "\(\text{L}\)" and not "\(\text{l}\)". So we would write 3.5 milliliters as \(3.5 \: \text{mL}\).
As a practical matter, whenever possible you should express the units in a small and manageable number. If you are measuring the weight of a material that weighs \(6.5 \: \text{kg}\), this is easier than saying it weighs \(6500 \: \text{g}\) or \(0.65 \: \text{dag}\). All three are correct, but the \(\text{kg}\) units in this case make for a small and easily managed number. However, if a specific problem needs grams instead of kilograms, go with the grams for consistency.
Give the abbreviation for each unit and define the abbreviation in terms of the base unit.
- kiloliter
- microsecond
- decimeter
- nanogram
Solutions
| Explanation | Answer | |
|---|---|---|
| a | The prefix kilo means “1,000 ×,” so 1 kL equals 1,000 L. | kL |
| b | The prefix micro implies 1/1,000,000th of a unit, so 1 µs equals 0.000001 s. | µs |
| c | The prefix deci means 1/10th, so 1 dm equals 0.1 m. | dm |
| d | The prefix nano means 1/1000000000, so a nanogram is equal to 0.000000001 g. | ng |
Give the abbreviation for each unit and define the abbreviation in terms of the base unit.
- kilometer
- milligram
- nanosecond
- centiliter
- Answer a:
- km
- Answer b:
- mg
- Answer c:
- ns
- Answer d:
- cL
Summary
- Metric prefixes derive from Latin or Greek terms. The prefixes are used to make the units manageable.
- The SI system is based on multiples of ten. There are seven basic units in the SI system. Five of these units are commonly used in chemistry.

