11.3: Balancing Equations
- Page ID
- 53781

Any leftovers?
When you cook a meal, quite often there are leftovers because you prepared more than people would eat at one sitting. Sometimes when you repair a piece of equipment, you end up with what are called "pocket parts"—small pieces you put in your pocket because you're not sure where they belong. Chemistry tries to avoid leftovers and pocket parts. In normal chemical processes, we cannot create or destroy matter (law of conservation of mass). If we start out with ten carbon atoms, we need to end up with ten carbon atoms. John Dalton's atomic theory said that chemical reactions basically involve the rearrangement of atoms. Chemical equations need to follow these principles in order to be correct.
Balancing Chemical Equations
A balanced equation is a chemical equation in which mass is conserved and there are equal numbers of atoms of each element on both sides of the equation. We can write a chemical equation for the reaction of carbon with hydrogen gas to form methane \(\left( \ce{CH_4} \right)\):
\[\begin{array}{ccccc} \ce{C} \left( s \right) & + & \ce{H_2} \left( g \right) & \rightarrow & \ce{CH_4} \left( g \right) \\ 2 \: \ce{C} \: \text{atoms} & & 2 \: \ce{H} \: \text{atoms} & & 1 \: \ce{C} \: \text{atom,} \: 4 \: \ce{H} \: \text{atoms} \end{array}\nonumber \]
In order to write a correct equation, you must first write the correct skeleton equation with the correct chemical formulas. Recall that hydrogen is a diatomic molecule and so is written as \(\ce{H_2}\).
When we count the number of atoms of both elements, shown under the equation, we see that the equation is not balanced. There are only 2 atoms of hydrogen on the reactant side of the equation, while there are 4 atoms of hydrogen on the product side. We can balance the above equation by adding a coefficient of 2 in front of the formula for hydrogen.
\[\ce{C} \left( s \right) + 2 \ce{H_2} \left( g \right) \rightarrow \ce{CH_4} \left( g \right)\nonumber \]
A coefficient is a small whole number placed in front of a formula in an equation in order to balance it. The 2 in front of the \(\ce{H_2}\) means that there are a total of \(2 \times 2 = 4\) atoms of hydrogen as reactants. Visually, the reaction looks like the figure below.
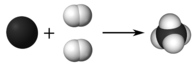
In the balanced equation, there is one atom of carbon and four atoms of hydrogen on both sides of the arrow. Below are guidelines for writing and balancing chemical equations.
- Determine the correct chemical formulas for each reactant and product.
- Write the skeleton equation.
- Count the number of atoms of each element that appears as a reactant and as a product. If a polyatomic ion is unchanged on both sides of the equation, count it as a unit.
- Balance each element one at a time by placing coefficients in front of the formulas.
- It is best to begin by balancing elements that only appear in one chemical formula on each side of the equation.
- No coefficient is written for a 1.
- NEVER change the subscripts in a chemical formula—you can only balance equations by using coefficients.
- Check each atom or polyatomic ion to be sure that they are equal on both sides of the equation.
- Make sure that all coefficients are in the lowest possible ratio. If necessary, reduce to the lowest ratio.
Example \(\PageIndex{1}\): Balancing Chemical Equations
Aqueous solutions of lead (II) nitrate and sodium chloride are mixed. The products of the reaction are an aqueous solution of sodium nitrate and a solid precipitate of lead (II) chloride. Write the balanced chemical equation for this reaction.
Solution
Step 1: Plan the problem.
Follow the steps for writing and balancing a chemical equation listed in the text.
Step 2: Solve.
Write the skeleton equation with the correct formulas.
\[\ce{Pb(NO_3)_2} \left( aq \right) + \ce{NaCl} \left( aq \right) \rightarrow \ce{NaNO_3} \left( aq \right) + \ce{PbCl_2} \left( s \right)\nonumber \]
Count the number of each atom or polyatomic ion on both sides of the equation.
\[\begin{array}{ll} \textbf{Reactants} & \textbf{Products} \\ 1 \: \ce{Pb} \: \text{atom} & 1 \: \ce{Pb} \: \text{atom} \\ 2 \: \ce{NO_3^-} \: \text{ions} & 1 \: \ce{NO_3^-} \: \text{ions} \\ 1 \: \ce{Na} \: \text{atom} & 1 \: \ce{Na} \: \text{atom} \\ 1 \: \ce{Cl} \: \text{atom} & 2 \: \ce{Cl} \: \text{atoms} \end{array}\nonumber \]
The nitrate ions and the chlorine atoms are unbalanced. Start by placing a 2 in front of the \(\ce{NaCl}\). This increases the reactant counts to 2 \(\ce{Na}\) atoms and 2 \(\ce{Cl}\) atoms. Then place a 2 in front of the \(\ce{NaNO_3}\). The result is:
\[\ce{Pb(NO_3)_2} \left( aq \right) + 2 \ce{NaCl} \left( aq \right) \rightarrow 2 \ce{NaNO_3} \left( aq \right) + \ce{PbCl_2} \left( s \right)\nonumber \]
The new count for each atom and polyatomic ion becomes:
\[\begin{array}{ll} \textbf{Reactants} & \textbf{Products} \\ 1 \: \ce{Pb} \: \text{atom} & 1 \: \ce{Pb} \: \text{atom} \\ 2 \: \ce{NO_3^-} \: \text{ions} & 2 \: \ce{NO_3^-} \: \text{ions} \\ 2 \: \ce{Na} \: \text{atom} & 2 \: \ce{Na} \: \text{atom} \\ 2 \: \ce{Cl} \: \text{atom} & 2 \: \ce{Cl} \: \text{atoms} \end{array}\nonumber \]
Step 3: Think about your result.
The equation is now balanced since there are equal numbers of atoms of each element on both sides of the equation.
Summary
- The process of balancing chemical equations is described.
Review
- What is the law of conservation of mass?
- How did Dalton describe the process of a chemical reaction?
- Why don’t we change the subscripts in order to balance an equation?

