1.8: N-dimensional cyclic systems

This lecture will provide a derivation of the LCAO eigenfunctions and eigenvalues of N total number of orbitals in a cyclic arrangement. The problem is illustrated below:

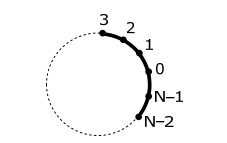
There are two derivations to this problem.

Polynomial Derivation

The Hückel determinant is given by,

\[D_{N}(x)=\left|\begin{array}{ccccccccc}
x & 1 & & & & & & & \\
1 & x & 1 & & & & & & \\
& 1 & x & \ddots & & & & & \\
& & 1 & \ddots & \ddots & & & & \\
& & & \ddots & \ddots & \ddots & & & \\
& & & & \ddots & \ddots & \ddots & & \\
& & & & & \ddots & \ddots & 1 & \\
& & & & & & \ddots & x & 1 \\
& & & & & & 1 & x
\end{array}\right|=0\]

where

\[ x=\frac{\alpha-E}{\beta}\]

From a Laplace expansion one finds,

D_N(x) = xD_n-1(x) - D_N-2(x)

Where

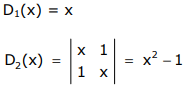
With these parameters defined, the polynomial form of D_N(x) for any value of N can be obtained,

D₃(x) = xD₂(x) – D₁(x) = x(x²–1) – x = x(x²–2)

D₄(x) = xD₃(x) – D₂(x) = x²(x²–2) – (x²–1)

\[ \vdots \nonumber \]

and so on

The expansion of DN(x) has as its solution,

\[ x={-2}\cos \dfrac{2\pi}{N}j (j= 0, 1, 2, 3...N-1) \nonumber \]

and substituting for x,

\[ E = \alpha + 2\beta\cos \dfrac{2\pi}{N}j (j= 0, 1, 2, 3...N-1) \nonumber \]

Standing Wave Derivation

An alternative approach to solving this problem is to express the wavefunction directly in an angular coordinate, θ

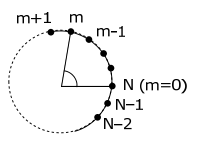
For a standing wave of λ about the perimeter of a circle of circumference c,

\[ \psi_j = \sin \dfrac{c}{\lambda} \theta \nonumber \]

The solution to the wave function must be single valued ∴ a single solution must be obtained for ψ at every 2nπ or in analytical terms,

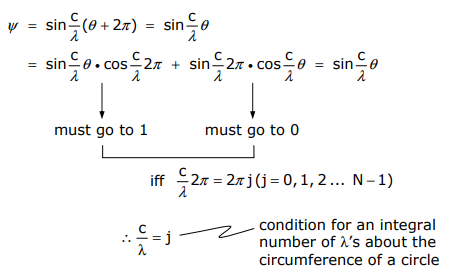
Thus the amplitude of \(ψ_j\) at atom m is, (where c/λ = j and θ = (2π/N)m)

\[ \psi_{j}(m) = \sin{2m\pi}{N}j (j= 0, 1, 2, 3...N-1) \nonumber \]

Within the context of the LCAO method, ψ_j may be rewritten as a linear combination in φ_m with coefficients c_jm. Thus the amplitude of ψ_j at m is equivalent to the coefficient of φ_m in the LCAO expansion,

\[ \psi_{j} = \displaystyle \sum_{k=1}^N C_{jm\phi m} \]

Where

\[C_{jm} = \sin{2\pi m}{N}j (j= 0, 1, 2, 3...N-1) \nonumber \]

The energy of each MO, ψ_j, may be determined from a solution of Schrödinger’s equation,

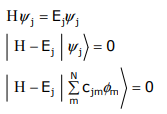
The energy of the φ_m orbital is obtained by left–multiplying by φ_m,

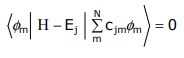
but the Hückel condition is imposed; the only terms that are retained are those involving φ_m, φ_m+1, and φ_m-1. Expanding,

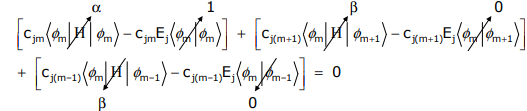
Evaluating the integrals,

Substituting for c_jm,

\[ \alpha \sin \dfrac{2\pi m}{N}j + \beta \left( \sin \dfrac{2\pi (m+1)}{N}j + \sin \dfrac{2\pi (m-1)}{N}j \right) = E_{j} \sin \dfrac{2\pi m}{N}j \nonumber \]

\[ \alpha + \dfrac{ \beta \left( \sin \dfrac{2\pi (m+1)}{N}j + \sin \dfrac{2\pi (m-1)}{N}j \right)}{ \sin \dfrac{2\pi m}{N}j} = E_{j} \nonumber \]

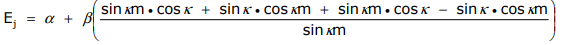
\[ E_{j} = \alpha + 2\beta \cos k \nonumber \]

\[ E_{j} = \alpha + 2\beta \cos \dfrac{2\pi}{N}j (j= 0, 1, 2, 3...N-1) \nonumber \]

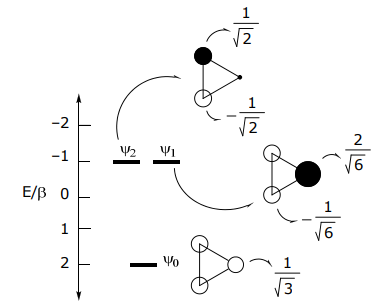
Let’s look at the simplest cyclic system, N = 3

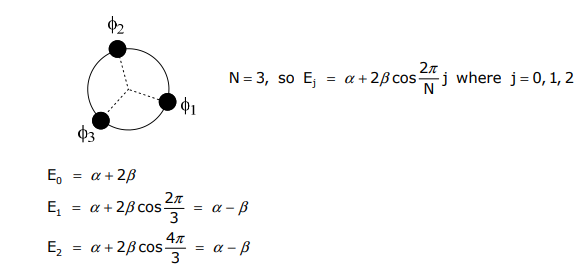
Continuing with our approach (LCAO) and using Ej to solve for the eigenfunction, we find…

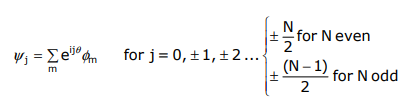
Using the general expression for ψj, the eigenfunctions are:

\[ \psi_{0} = e^{i(0)0} \phi_{1} + e^{i(0) \dfrac{2\pi}{3}} \phi_{2} + e^{i(0) \dfrac{4\pi}{3}} \phi_{3} \nonumber \]

\[ \psi_{1} = e^{i(1)0} \phi_{1} + e^{i(1) \dfrac{2\pi}{3}} \phi_{2} + e^{i(1) \dfrac{4\pi}{3}} \phi_{3} \nonumber \]

\[ \psi_{-1} = e^{i(-1)0} \phi_{1} + e^{i(-1) \dfrac{2\pi}{3}} \phi_{2} + e^{i(-1) \dfrac{4\pi}{3}} \phi_{3} \nonumber \]

Obtaining real components of the wavefunctions and normalizing,

$$
\begin{array}{ll}
\psi_{0}=\phi_{1}+\phi_{2}+\phi_{3} \rightarrow & \psi_{0}=\frac{1}{\sqrt{3}}\left(\phi_{1}+\phi_{2}+\phi_{3}\right) \\
\psi_{+1}+\psi_{-1}=2 \phi_{1}-\phi_{2}-\phi_{3} \rightarrow & \psi_{1}=\frac{1}{\sqrt{6}}\left(2 \phi_{1}-\phi_{2}-\phi_{3}\right) \\
\psi_{+1}-\psi_{-1}=\phi_{2}-\phi_{3} \rightarrow & \psi_{2}=\frac{1}{\sqrt{2}}\left(\phi_{2}-\phi_{3}\right)
\end{array}
\]

Summarizing on a MO diagram where α is set equal to 0,