3.8: Chiral Molecules
- Page ID
- 32163
Introduction
Around the year 1847, the French scientist Louis Pasteur provided an explanation for the optical activity of tartaric acid salts. when he carried out a particular reaction, Pasteur observed that two types of crystals precipitated. Patiently and carefully using tweezers, Pasteur was able to separate the two types of crystals. Pasteur noticed that the types rotated the plane polarized by the same amount but in different directions. These two compounds are called enantiomers.
What are Enantiomers?
Two compounds are enantiomers if they are non-superimposable mirror images of each other. As was mentioned, enantiomers are characterized by their ability to rotate plane-polarized light. They also have the same physical properties (e.g., melting point, etc.) relative to each other. As a result, they are also referred to as being optically active. When it comes to symmetry, there are some general rules of thumb that help determine whether a molecule is chiral or achiral. This can be very useful because sometimes molecules can have relatively complicated structures and geometries that knowing whether or not they are chiral becomes a daunting task. The goal, as a result, is to determine the point group of the molecule and the symmetry elements associated with it, then inferring the chirality of the molecule.
Using Symmetry to Determine Chirality
For a molecule to be chiral, it must lack:
- Center of inversion \(i\) and a plane of symmetry \(\sigma\).
- An improper rotation axis (rotation-reflection axis) \(S_{n}\).
However, since, by definition, an improper rotation axis is a rotation about an certain axis followed by reflection about a plane perpendicular to that axis, and an inversion center is simply \(S_{2}\), the absence of an improper axis requires, in most cases, that absence of both a plane of symmetry and an inversion center. As a result, it suffices, in most cases, to check for improper rotation axes to determine whether a molecule is chiral or not.
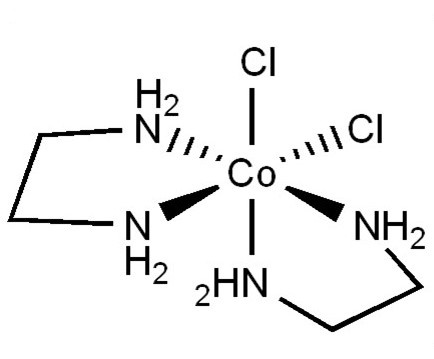
As a result of the previous discussion, there are a few classes of point groups that lack an improper axis. Those classes are \(C_{1}\), \(C_{n}\), and \(D_{n}\). Cis-dichlorobis(ethylenediamine)cobalt (III) has two enantiomers that are chiral (figure 1), but the trans compound is achiral.