14.8.1: Foods- Food Additives
- Page ID
- 50895
back to Buffer Solutions
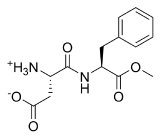
Aspartame
Soft drinks and buffers
Citric acid
So far you have learned about pH in solutions where either a single acid, such as citric acid, or a single base, such as the citrate ion have been added to water. Now, let us consider solutions prepared with both an acid and a base. The simplest case of such a solution occurs when the acid and base are conjugate to each other and also are present in comparable amounts. Solutions of this special kind are called buffer solutions because it is difficult to change their pH even when an appreciable amount of strong acid or strong base is added. Why are buffer solutions important in foods?
Buffering solutions in foods play an important role in maintaining specific pH values for optimum activity of enzymes, protein solubility, and functionality. As discussed in previous exemplars, pH may also modify the color and flavor of foods and it is a critical factor in the preservation of many processed foods. Buffering solutions are also used as reaction media in the production of food ingredients and additives. Overall pH control is a major factor in maintaining the physical, chemical, and microbiological stability of foods.
Foods contain numerous compounds able to form buffering systems. Molecules with acid-base properties naturally found in foods include amino acids, organic acids, proteins, and charged polysaccharides. Other buffering systems are intentionally added to processed foods, examples of these are the weak acids discussed in the pH of weak acids in foods and their corresponding conjugate bases.
How do we calculate the pH of buffer solutions?
As an example of a buffer solution, let us consider the solution obtained when 3.00 mol citric acid (H3C6H5O7) and 2.00 mol monosodium citrate (NaH2C6H5O7) are added to sufficient water to produce a solution of total volume 1 dm³. The stoichiometric concentration of citric acid, namely, ca, is then 3.00 mol dm–3, while the stoichiometric concentration of sodium citrate, cb, is 2.00 mol dm–3. As a result of mixing the two components, some of the citric acid, say x mol dm–3, is converted to citrate ion and hydronium ion. We can now draw up a table in order to find the equilibrium concentrations in the usual way.
| Species |
Initial Concentration mol dm–3 |
Change in Concentration mol dm–3 |
Equilibrium Concentration mol dm–3 |
| H3O+ | 10–7 (negligible) | x | x |
| H2C6H5O7– | 2.00 | x | 2.00 + x |
| H3C6H5O7 | 3.00 | (–x) | 3.00 – x |
We can now substitute concentrations in the equilibrium expression \(K_{a}=\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}][\text{H}_{3}\text{O}^{+}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\) from which we obtain \(\text{1.4}\times \text{10}^{-3}\text{ mol dm}^{-3}=\frac{\text{(2.00 + }x\text{)}x}{\text{3.00}-x}\text{ mol dm}^{-3}\)...(1) In order to solve this equation, we make the approximation that x is negligibly small compared with both 2.00 and 3.00, that is, that only a minute fraction of citric acid has converted to citrate ion. We then have \(\frac{\text{2.00}x}{\text{3.00}}=\text{1.4}\times \text{10}^{-3}\)
or \(\begin{align}x&=\frac{\text{3.00}}{\text{2.00}}\times \text{ 1.4 }\times \text{ 10}^{-3}\\ \text{ }&=\text{2.1}\times \text{10}^{-3}\end{align}\)
Since x is only 0.1 percent of 2.00 or 3.00, the approximation is valid and there is no need to obtain a second approximation by feeding x back into Eq. (1). We can thus conclude that \([\text{H}_{3}\text{O}^{+}] = \text{2.1} \times \text{10}^{-3}\text{mol dm}^{-3}\) and \(\text{pH} = \text{2.67}\,\) This example demonstrates two obvious features: 1 When the acid and its conjugate base are mixed, very little of the acid is converted to base, or vice versa. (x is small compared with 2.00 and 3.00.)
2 In a buffer mixture, the hydronium-ion concentration and the hydroxide-ion concentration are small compared with the concentrations of acid and conjugate base. ([H3O+] = 2.7 × 10–5 mol dm–3; [HO–] = 3.7 × 10–10 mol dm–3 as compared with [H2C6H5O7–] = 2.00 mol dm–3 and [H3C6H5O7] = 3.00 mol dm–3)
The Henderson-Hasselbach equation
Assuming that the above features are common to all buffer solutions, we make it very easy to handle them from a mathematical standpoint. Let us now consider the general problem of finding the pH of a buffer solution which is a mixture of a weak acid HA, of stoichiometric concentration ca, and its conjugate base A–, of stoichiometric concentration cb. We can rearrange the expression for Ka of the weak acid (Equation 2 on the pH of solutions of weak acids) as follows:
\[[\text{H}_{3}\text{O}^{+}]=K_{a}\times \frac{[\text{HA}]}{[\text{A}^{-}]}\label{6} \]
(2)
Taking negative logarithms of both sides, we obtain
\[-\text{log }[\text{H}_{3}\text{O}^{+}]=-\text{log }K_{a}-\text{log}\frac{[\text{HA}]}{[\text{A}^{-}]} \nonumber \]
\[\text{pH}=\text{p}K_{a}\text{+ log}\frac{[\text{A}^{-}]}{[\text{HA}]}\label{8} \]
(3)
Equation \(\ref{8}\) is called the Henderson-Hasselbalch equation and is often used by chemists and biologists to calculate the pH of a buffer.
As we saw in the case of the citric acid―sodium citrate buffer described earlier, the equilibrium concentrations of HA and A– are usually almost identical to the stoichiometric concentrations. That is,
\([\text{HA}] \approx \text{c}_{a}\) and \([\text{A}^{-}]\approx\text{c}_{b}\)
We can substitute these values into Eqs. (2) and (3) to obtain the very useful approximations \([\text{H}_{3}\text{O}^{+}]\approx K_{a}\times \frac{c_{a}}{c_{b}}\) (4) and \(\text{pH}\approx \text{ p}K_{a}\text{ + log}\frac{c_{a}}{c_{b}}\) (5)
Calculate the pH of a buffer containing 3.93 g of NaH2PO4 and 4.31 g of Na2HPO4 per 450 mL of solution
Solution: First, we need to calculate the concentration of both the acid (NaH2PO4) and the base (Na2HPO4) in solution. For NaH2PO4 we have that the number of moles is
\(\begin{align}\text{n}_{\text{NaH}_{2}\text{PO}_{4}} &= \frac{\text{3.93 g}}{\text{119.98 g mol}^{-1}} \\ \text{ } &= \text{3.27}\times \text{10}^{-2} \text{ mol} \end{align}\) and its concentration \(\begin{align}\left[\text{NaH}_{2}\text{PO}_{4}\right] &= \frac{\text{3.27}\times \text{10}^{-2} \text{ mol}}{\text{0.45 dm}^{3}}\\ \text{ } &= \text{7.27} \times \text{10}^{-2} \text {mol dm}^{-3} \end{align}\) and for Na2HPO4 \(\begin{align}\text{n}_{\text{Na}_{2}\text{HPO}_{4}} &= \frac{\text{4.31 g}}{\text{141.96 g mol}^{-1}} \\ \text{ } &= \text{3.04}\times \text{10}^{-2} \text{ mol} \end{align}\)\(\begin{align}\text{n}_{\text{Na}_{2}\text{HPO}_{4}} &= \frac{\text{4.31 g}}{\text{141.96 g mol}^{-1}} \\ \text{ } &= \text{3.04}\times \text{10}^{-2} \text{ mol} \end{align}\)
Which means that the concentrations of H2PO4– and HPO42– are respectively 7.27 x 10–2 mol dm–3 and 7.55 x 10–2 mol dm–3. Using these values and a pKa2= 7.21 for the pair H2PO4–/HPO42– (calculated from Ka2) in the Henderson Hasselbach equation, the pH of the buffer becomes \(\begin{align}\text{pH}&=\text{p}K_{a}\text{+ log}\frac{[\text{A}^{-}]}{[\text{HA}]}\\ \text{ }&= \text{ 7.21}\text{+ log}\frac{\text{6.75} \times \text{10}^{-2}\text {mol dm}^{-3}}{\text{7.27} \times \text{10}^{-2} \text {mol dm}^{-3}}\\ \text{ }&= \text{7.17} \end{align}\) Phosphate buffers help to control the pH of physiological fluids and are often used in carbonated soft drinks.
Buffer solutions and the stability of food additives
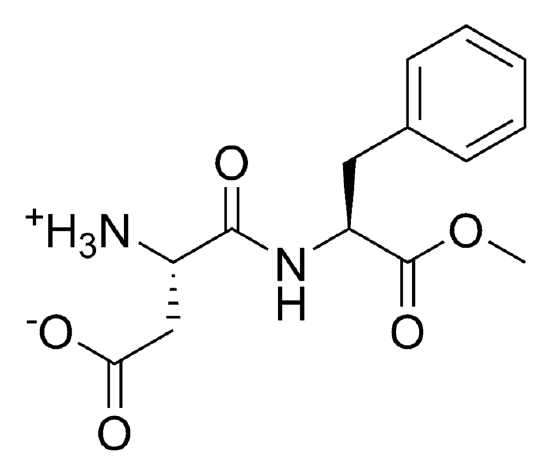
Aspartame is a high intensity sweetener composed by two amino acids L-aspartic acid and phenylalanine forming the dipeptide N-α-aspartyl-L-phenylalanine methyl ester. Aspartame is approximately 200 times sweeter than sucrose and presents synergistic interactions with other sweeteners including saccharin, cyclamate, stevioside, acesulfame K, and various sugars.[1] Aspartame is currently used as non-nutritive sweetener in various products including soft drinks, dry foods, ice cream, yogurt, fruit juices, and tabletop sweeteners.[2]
As a dipeptide, the stability of aspartame can be affected by extreme values of pH, temperature, and water activity. The degradation of aspartame generates the di-peptide aspartylphenylalanine and methanol. In some instances, aspartame can first form the cyclic compound diketopiperazine with the release of methanol. Ultimately, aspartylphenylalanine will be hydrolyzed into aspartate and phenylalanine, which posses a mayor risk for people with phenylketonuria. None of the degradation products has a sweet taste resulting in the loss of sweetness.[1]
The soft drink industry is one of the most important users of aspartame for the manufacture of diet beverages. Given that pH is a major factor affecting the stability of aspartame, it is critical to have a way to maintain its value within certain limits to ensure the sweetness expected in the product. Such control can be attained by having a buffering system in the formulation. In order to select the appropriate buffering system, it is necessary to consider not only the target pH, but also the concentration and chemical composition of the buffer as well as the potential interactions with other components in the formulation.
The concentration and the chemical composition of a buffer can affect the stability of aspartame. An study on the stability of this sweetener in solution showed that its degradation rate constants were smaller at pH 3 than at pH 7 and they increased as the concentration of the buffer was increased between 0.01 and 1.0 M. The study also investigated the effect of the composition of the buffer using phosphate and citrate acid-base pairs. At both pH 3 and 7 the degradation rates were higher in phosphate buffer. The smallest rate constant values for this buffer were registered at concentrations between 0.01 and 0.1 M. Even though these results can be explained in part by differences in the dissociation constants of the buffer components, the overall difference in the rate constants suggested that there might be additional causes affecting the degradation of aspartame in these buffers. The authors attributed such difference to the fact that phosphate buffer ions can act as bifunctional catalysts because they can both donate protons and accept groups in close proximity. This activity of the phosphate ion promotes the cyclization of aspartame to form diketopiperazine and methanol. Although citrate can also accept and donate electrons with aspartame, due to its larger size, it is not as efficient as the phosphate ions resulting in a slower degradation rate. On the other hand, the difference on the degradation rates at pH 3 and 7 can be explained considering that a pH 3, the free amino group of aspartame is protonated reducing its nucleophilic activity and preventing its interaction with the carbonyl group to form diketopiperazine. [3]
Make the calculations to prepare a buffer to be used in the manufacture of a soft drink containing aspartame and will preserve its activity as sweetener.
Solution: This buffer should have a pH of 3 and be made with citric acid/citrate with a Ka1= 1.4 x 10–3 in a concentration between concentrations 0.01 to 0.1 M.
With this information we can use the Henderson-Hasselbach equation as follows \(\begin{align}\text{pH}&=\text{p}K_{a}\text{ + log}\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\\ \text{3}&=-\text{log(1.4} \times \text{10}^{-3}\text{) + log}\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\\ \text{3}&=\text{2.85}+ \text{log}\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\\ \text{0.15}&=\text{log}\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\end{align}\) Applying the antilogarithm function in both sides of the equation we have \(\begin{align}\text{10 }^{ 0.15}&= \frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\\ \text{1.41} &= \frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}\\ \end{align}\) Now that we have the value for the ratio [H2C6H5O7–]/[H3C6H5O7], we need to determine the concentrations for each compound. Since the concentration required for the buffer is 0.1, we need to consider the following equilibrium\(\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7} + \text{H}_{2}\text{O} \rightarrow \text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-} + \text{H}_{3}\text{O}^{+}\)
and the concentration at the equilibrium for the acid an the base are
| Species |
Initial Concentration mol dm–3 |
Change in Concentration mol dm–3 |
Equilibrium Concentration mol dm–3 |
| H3O+ | 10–7 (negligible) | x | x |
| H2C6H5O7– | 0.00 | x | x |
| H3C6H5O7 | 0.10 | (–x) | 0.10 – x |
Expressing the concentration of the acid and the base in terms of x, we have
\(\begin{align}\frac{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}= \frac{\text{x}}{\text{0.1 - x}}=\text{1.41}\end{align}\) Solving for x \(\begin{align}\text{x}&=\text{1.41}\left(\text{0.1} - \text{x}\right)\\ \text{x}&=\text{0.141}-\text{1.41x}\\ \text{2.41x}&=\text{0.141}\\ \text{x}&=\frac{\text{0.141}}{\text{2.41}}=\text{0.0585} \end{align}\) Therefore,\([\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]=\text{0.0585 mol dm}^{-3}\) and
\([\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]=\text{0.1} - \text{0.0585}=\text{0.415 mol dm}^{-3}\)
To confirm that this mixture of citric acid and citrate will yield a buffer of pH 3 we can calculate \(\begin{align}\text{pH}&=-\text{log(1.4} \times \text{10}^{-3}\text{) + log}\frac{(\text{0.0585 mol dm}^{-3})}{( \text{0.0415 mol dm}^{-3})}\\ \text{ }&=\text{2.85} + \text{0.149}&\\ \text{pH}&= 2.99 \end{align}\)How do buffers work?
To better understand why a mixture of an acid and its conjugate base is resistant to a change in pH, let us go back to our first example: a mixture of citric acid (3 mol dm–3) and sodium citrate (2 mol dm–3). What would happen if we now added 0.50 mol sodium hydroxide to 1 dm3 of this mixture? The added hydroxide ion will attack both the acids present, namely, the hydronium ion and citric acid. Since the hydronium-ion concentration is so small, very little hydroxide ion will be consumed by reaction with the hydronium ion. Most of it will be consumed by reaction with citric acid. Further, since the hydroxide ion is such a strong base, the reaction
\(\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7} + \text{OH}^{-} \rightarrow \text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-} + \text{H}_{2}\text{O}\) will go virtually to completion, and 0.50 mol citric acid will be consumed. The same amount of citrate ion will be produced. In tabular form:
| Species |
Initial Concentration mol dm–3 |
Change in Concentration mol dm–3 |
Equilibrium Concentration mol dm–3 |
| H3O+ | 2.1 x 10–4 (negligible) | Small | approx. 2.1 x 10–4 |
| H2C6H5O7– | 2.00 | 0.5 | 2.00 + 0.5 = 2.5 |
| H3C6H5O7 | 3.00 | (– 0.5) | 3.00 – 0.5 = 2.5 |
Substituting the equilibrium concentrations of base (citrate ion) and conjugate acid (citric acid) into the Henderson-Hasselbalch equation, Eq. (3), we have
\(\begin{align}\text{pH}&=\text{p}K_{a}\text{ + log}\frac{[\text{A}^{-}]}{[\text{HA}]}\\ \text{ }&=-\text{log(1.4} \times \text{10}^{-3}\text{) + log}\frac{\text{(2.50 mol dm}^{-3}\text{)}}{\text{(2.50 mol dm}^{-3}\text{)}}\\ \text{ }&=-\left(\text{0.15}-\text{3} \right)+ \text{log}\left(\text{1}\right)\\ \text{ }&=\text{2.85 + 0}=\text{2.85}\end{align}\)
The addition of 0.5 mol sodium hydroxide to the buffer mixture raised its pH from 2.5 to only 2.85.
Now, what would be the pH of the solution if the 0.5 mol of sodium hydroxide would have been added to a cubic decimeter of pure water in absence of citric acid?
This amount of sodium hydroxide would produce a solution containing 0.5 M of hydroxide ions with a pOH equal to
\(\text{pOH}=-\text{log}(\text{0.5}) = \text{0.3}\,\) and \(\text{pH}= \text{14} - \text{ 0.3} = \text{13.7}\,\) This shows you that the buffer is extremely effective at resisting a change in pH because the added hydroxide ion attacks the weak acid (in very high concentration) rather than the hydronium ion (in very low concentration). The major effect of the addition of the hydroxide ion is thus to change the ratio of acid to conjugate base, i.e., to change the value of \(\frac{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}\) As long as the amount of weak acid is much larger than the amount of base added, this ratio is not altered by very much. Since the hydronium-ion concentration is governed by \([\text{H}_{3}\text{O}^{+}]=K_{a}\frac{[\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}]}{[\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}]}\) Similarly, if instead of a strong base, a strong acid such as hydrochloric was added to the buffer mixture, it would react with the citrate in solution. Although, the pH would decrease, the change would be minor again.
Find the pH of the solution obtained when 2.00 mol H2C6H5O7– and 0.80 mol H3C6H5O7 are mixed to give 2.5 dm3 of solution. Kb(H2C6H5O7–) = 7.1 × 10–12 mol dm–3.
Solution In order to use Eq. (4),we need first to have the value of
\(\begin{align}K_{a}\left(\text{H}_{3}\text{C}_{6}\text{H}_{5}\text{O}_{7}\right)&= \frac{K_{w}}{K_{b}\left(\text{H}_{2}\text{C}_{6}\text{H}_{5}\text{O}_{7}^{-}\right)}\\ \text{ }&=\frac{\text{1.00}\times \text{ 10}^{-14}\text{ mol}^{2}\text{ dm}^{-6}}{\text{7.1 }\times \text{ 10}^{-12}\text{ mol dm}^{-3}}\\ \text{ }&=\text{1.4}\times \text{ 10}^{-3}\text{ mol dm}^{-3} \end{align}\)Given that the final volume of the solution is 2.5 dm3, the concentration of the acid and base in solution are ca = 0.32 mol dm–3 and cb = 0.80 mol dm–3. Thus
\(\begin{align}\left[\text{H}_{3}\text{O}^{+}\right]&\approx K_{a}\times \frac{c_{a}}{c_{b}}\\ \text{ }&\approx \text{1.4}\times \text{ 10}^{-3}\text{ mol dm}^{3}\times \frac{\text{ 0.32 mol dm}^{-3}}{\text{0.80 mol dm}^{-3}}\\ \text{ }&\approx \text{5.6}\times \text{ 10}^{-4}\text{ mol dm}^{-3}\end{align}\)
from which
and \(\text{pH} = \text{3.25}\,\)From ChemPRIME: 14.7: Buffer Solutions
References
- Chemistry and technology of soft drinks and fruit juices. 2nd ed. 2005 Ashurst, P. R.
- Food Additives, 2nd ed. 2002, Branen, A., Davidson, M.P., Salminen, S. and Thorngate III, J.H.
- Bell, L.N. and Wetzel, C.R. 1995. Aspartame degradation in solution as impacted by buffer type and concentration. J. Agric. Food Chem. 43:2608-2612.
Contributors
Ed Vitz (Kutztown University), John W. Moore (UW-Madison), Justin Shorb (Hope College), Xavier Prat-Resina (University of Minnesota Rochester), Tim Wendorff, and Adam Hahn.


