7.7: Orbital Descriptions of Multiple Bonds
- Page ID
- 49302
The association of four sp3 hybrid orbitals with an octet can be applied to multiple bonds as well as single bonds. A simple example is ethene (ethylene), C2H4. The Lewis structure for this molecule is
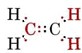
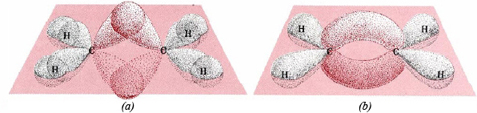
Since the orbitals which overlap are not pointing directly at each other, each of these bonds is referred to as a bent bond or (more frivolously) as a banana bond.
In ball-and-stick models of molecules, a double bond is usually represented by two springs or by curved sticks (shown in Figure \(\PageIndex{2}\) ) joining the two atoms together. In making such a model, it is necessary to bend the springs a fair amount in order to fit them into the appropriate holes in the balls. The ability to bend or stretch is characteristic of all chemical bonds—not just those between doubly bonded atoms. Thus each atom can vibrate about its most stable position. Perhaps ball-and-spring models would be more appropriate than ball-and-stick models in all cases.
The bent-bond picture makes it easy to explain several characteristics of double bonds. As noted in Chemical Bonding - Electron Pairs and Octets, the distance between two atomic nuclei connected by a double bond is shorter than if they were connected by a single bond. In the case of carbon-carbon bonds, for example, the 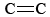 distance is 133 pm, while the C—C distance is 156 pm. This makes sense when we realize that each bent bond extends along a curved path. The distance between the ends of such a path (the C nuclei) is necessarily shorter than the path itself.
distance is 133 pm, while the C—C distance is 156 pm. This makes sense when we realize that each bent bond extends along a curved path. The distance between the ends of such a path (the C nuclei) is necessarily shorter than the path itself.
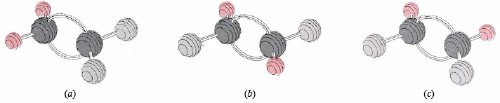
Another characteristic of double bonds is that they make it difficult to twist one end of a molecule relative to the other. This phenomenon usually is called a barrier to rotation. Such a barrier accounts for the fact that it is possible to prepare three different compounds with the formula C2H2F2. Their structures are shown in Figure 2. Structure (a) is unique because both F atoms are attached to the same C atom, but (b) and (c) differ only by a 180° flip of the right-hand 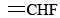 group. If there were no barrier to rotation around the double bond, structures (b) and (c) could interconvert very rapidly whenever they collided with other molecules. It would then be impossible to prepare a sample containing only type (b) molecules or only type (c) molecules.
group. If there were no barrier to rotation around the double bond, structures (b) and (c) could interconvert very rapidly whenever they collided with other molecules. It would then be impossible to prepare a sample containing only type (b) molecules or only type (c) molecules.
Since they have the same molecular formula, (a), (b) and (c) are isomers. Structure (b) in which the two F atoms are on opposite sides of the double bond is called the trans isomer, while structure (c) in which two like atoms are on the same side is called the cis isomer. It is easy to explain why there is a barrier to rotation preventing the interconversion of these cis and trans isomers in terms of our bent-bond model. Rotation of one part of the molecule about the line through the C atoms will cause one of the bent-bond electron clouds to twist around the other. Unless one-half of the double bond breaks, it is impossible to twist the molecule through a very large angle.
The jmol above allows you to view all of the molecular orbitals for ethene. By using the scroll bar, you can choose any of the molecular orbitals associated with the molecule and view them. Molecular Orbitals are discussed further in the section on Delocalized Electrons. By choosing orbital "N6", you can view a sigma bond orbital for ethene. Choosing the orbital "N8" will display a pi orbital for the molecule.


